Open Journal of Energy Efficiency
Vol.05 No.02(2016), Article ID:67338,11 pages
10.4236/ojee.2016.52005
Achievement of Laser Fusion with High Energy Efficiency Using a Mixture of D and T Ions
A. Youssef1,2*, M. Haparir2
1Physics Department, Faculty of Science, Ha’il University, Hail, Saudi Arabia
2Physics Department, Faculty of Science, Sohag University, Sohag, Egypt

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 19 March 2016; accepted 12 June 2016; published 15 June 2016
ABSTRACT
A mixture of deuterium (D) and tritium (T) is the most likely fuel for laser-driven inertial confinement fusion (ICF) reactors and hence DD and DT are the fusion reactions that will fire these reactors in the future. Neutrons produced from the two reactions will escape from the burning plasma, in the reactor core, and they are the only products possible to be measured directly. DT/DD neutron ratio is crucial for evaluation of T/D fuel ratio, burn control, tritium cycle and alpha particle self-heating power. To measure this ratio experimentally, the neutron spectra of DD and DT reactions have to be measured separately and simultaneously under high neutron counting with sufficient statistics (typically within 10% error) in a very short time and these issues are mutually contradicted. That is why it is not plausible to measure this high priority ratio for reactor performance accurately. Precise calculations of the DT/DD neutron ratio are needed. Here, we introduce such calculations using a three dimensional (3-D) Monte Carlo code at energies up to 40 MeV (the predicted maximum ion acceleration energy with the available laser systems). In addition, the fusion power ratio of DD and DT reactions is calculated for the same energy range. The study indicates that for a mixture of 50% deuterium and 50% triton, with taking into account the reactions D(d,n)3He and T(d,n)4He, the optimum energy value for achieving the most efficient laser-driven ICF is 0.08 MeV.
Keywords:
Inertial Confinement Fusion (ICF), DT Burning Plasma, DT/DD Neutron Ratio, Fusion Power Ratio

1. Introduction
Nuclear fusion is one of the few options that provide a sustainable and safe energy source for future. There are two approaches to achieve the nuclear fusion: Magnetic Confinement Fusion and laser fusion. Both of the approaches occur in burning plasma (high-energy-density plasma) to offer the very high temperature and density required to achieve the fusion. The burning plasma regime is a critically important regime of plasma physics. Investigating and understanding the physics of such plasmas are crucial in order to successfully achievement of nuclear fusion.
High power laser is a promising tool to achieving nuclear fusion as lasers can focus intense bursts of energy in a very short time onto small targets. Due to the recent progress of ultra-powerful lasers, the concept of fast ignition was introduced [1] . According to the fast ignition approach, the compressed fuel, by symmetrically laser beams irradiation, is injected by an ultra-intense laser pulse at the moment of maximum compression of the imploded plasma. The fast ignition approach takes place with accelerated ions up to a maximum value which depends on the laser properties. The question now is what is the best energy value to achieve the laser-driven ICF with the highest energy efficiency?
In principle, a mixture of hydrogen isotopes deuterium (D) and tritium (T) is the most promising fuel to fire the laser-driven ICF reactors in the future [2] - [6] . The fusion reactions D(d,n)3He and T(d,n)4He will occur in the burning DT plasma in the reactor core. Neutrons produced by the two reactions will be used to measure the fusion output and its time evolution. As it is impossible to probe the burning plasma directly, the produced neutron yield is practically the crucial factor to judge the achievement of nuclear fusion [7] . The emitted neutrons readily carry information from inside the burning plasma without any effect due to electric and/or magnetic fields generated inside or around the plasma. In particular, numerous key plasma parameters can be deduced from the neutron energy spectrum such as the fuel isotope ratio (D/T ratio), fusion power, ion temperature distributions, and the plasma density profile in a plasma core [8] - [15] . Consequently, investigating the neutron energy spectrum of the emitted neutrons from the burning plasma has been recognized as a high-priority task.
In previous works [16] - [21] , we started a series of studies both experimentally and theoretically to investigate some important aspects of laser produced burning plasma. The studies depend on the analysis of neutron spectra produced by nuclear reactions generated inside the burning plasma. For the best performance of the laser-driven ICF reactors, it is essential to carry out similar studies to measure the DT/DD neutron ratio. This ratio is crucial for controlling the isotope ratio of the fuel to be injected into the reactor. Controlling the D/T fuel ratio, and hence the D/T burning ratio, is a key parameter for determining the output power of fusion energy [2] - [15] . This study is interested in achieving a successful ICF using a mixture of D and T ions with the highest energy efficiency. To do this, the cross sections of the D(d,n)3He and T(d,n)4He reactions are calculated up to 40 MeV which is the predicted maximum acceleration energy of D ions using the laser facility for fast ignition experiment (LFEX) at the Institute of Laser Engineering (ILE), Osaka University [22] . Nuclear reactions induced by ions accelerated due to the front side acceleration [17] [18] . The maximum intensity of LFEX is 1021 W/cm2 and hence 40 MeV is the maximum energy of D ions as calculated according to the model introduced in Ref. [20] . In addition, the DT/DD neutron yield ratio over the energy range 0.01 ≤ Ed ≤ 40 MeV is calculated for the first time. As well, the fusion power ratio of the two reactions is computed for the same energy range. It is known that besides the channel D(d,n)3He, the DD reaction can occur through the other channel D(d,p)3H with the same probability i.e., 50% for each channel. The channel D(d,p)3H doesn’t give any neutrons so that it has nothing to do with the neutron ratio calculations.
Neutron spectra of DD and DT reactions have to be measured accurately to determine the DT/DD neutron yield ratio and the T/D fuel ratio. Experimentally, there many difficulties in measuring the DT/DD neutron yield ratio [23] . The neutron spectra produced by DD and DT reactions have to be measured separately and simultaneously in a very short time under high neutron counting with sufficient statistics (typically within 10% error) and these issues are mutually contradicted. The DD neutron spectrum is usually contaminated by the scattered/energy-degradation components of DT neutrons. In addition, neutron detectors suffer from a high event rate or accidental counts due to high radiation intensities. That is why, with the current experimental facilities, it is not plausible to measure this high priority ratio for reactor performance accurately. Consequently, precise calculations of the values and the behavior of DT/DD neutron yield ratio are needed. Here, we introduce such calculations for ICF using 3-D Monte Carlo code at energies up to 40 MeV. In addition, the first comparison of the fusion power, which is the final output of fusion, of DD and DT reactions is introduced for the same energy range. The rest of this paper is ordered as follows: In Section 2, the cross sections of the DD and DT reactions are calculated up to 40 MeV. In Section 3, the DT/DD neutron yield ratio over the energy range 0.01 ≤ Ed ≤ 40 MeV is introduced for the first time. In Section 4, the fusion power ratio of the two reactions is computed for the same energy range. Finally, the conclusion is given in Section 5.
2. Cross Section Calculation
To calculate both the neutron yield ratio and the fusion power ratio, the total cross section values of DD and DT reactions have to be calculated precisely. Here, the total cross sections of DD and DT reactions are calculated over the energy range 0.01 ≤ Ed ≤ 40 MeV with steps 0.01 MeV using the Drosg 2000 code [24] . To ensure that the Drosg 2000 code can reproduce the measured data, the calculated values are compared with the published data in nuclear data table [25] up to the maximum published value (Figure 1 and Figure 2). With the code we could extend the cross section values up to 40 MeV. Figures 3-5 show a comparison between calculated total cross section values of DT reaction and DD reaction up to 40 MeV.
In order to get the clearest possible picture, the comparison has been divided into three regimes. The first regime is from 0.01 MeV to 0.3 MeV (Figure 3). In this regime, the cross section of the DT reaction is much higher than that of the DD reaction and its minimum value exceeds 1000 mb. Throughout the regime, the DT cross section increases gradually to reach its maximum value (≈5000 mb) at 0.11 MeV and then it decreases
Figure 1. Total cross section values of DD reaction. The calculated values by the Drosg 2000 code (gray line) are compared with those published in nuclear data table (black line).The experimental data are a gathering of many experiments with discrepancies between them. This can explain the discrepancies between the theoretical values and the experimental data within the energy range 0.70 ≤ Ed ≤ 1.75 MeV.
Figure 2. Total cross section values of DT reaction. The calculated values by the Drosg 2000 code (gray line) are compared with those published in nuclear data table (black line).
Figure 3. Comparison between calculated total cross section values of DT fusion reaction (gray line) and DD fusion reaction (black line) by the Drosg 2000 code within the energy range 0.01 ≤ Ed ≤ 0.3 MeV for the incident deuteron.
Figure 4. Comparison between calculated total cross section values of DT fusion reaction (gray line) and DD fusion reaction (black line) by the Drosg 2000 code within the energy range 0.4 ≤ Ed ≤ 2.2 MeV for the incident deuteron.
Figure 5. Comparison between calculated total cross section values of DT fusion reaction (gray line) and DD fusion reaction (black line) by the Drosg 2000 code within the energy range 2.3 ≤ Ed ≤ 40 MeV for the incident deuteron.
to ≈ 1350 mb at 0.3 MeV. The second regime is from 0.4 MeV to 2.2 MeV (Figure 4). During this regime, the cross section of the DT reaction is less than 1000 mb and it decreases regularly to be the same as the DD reaction cross section at 2.2 MeV. In addition, this regime includes the maximum value of the DD reaction (≈107 mb) at 1.75 MeV. The third regime is from 2.3 MeV to 40 MeV (Figure 5). Through this wide regime, the DD total cross section is higher than the DT total cross section. Finally, it can be concluded that 2.2 MeV is the value at which the cross sections of DT and DD reactions are equal and before it the DT reaction cross section is higher but after it the DD reaction cross section is higher. The maximum value of the DT reaction cross is ≈5000 mb at 0.11 MeV whereas the maximum value of the DD reaction cross is ≈107 mb at 1.75 MeV.
3. Neutron Yield Ratio
DT/DD neutron yield ratio is an effective way to know the DT/DD burning ratio. This ratio will play a crucial rule for any fusion reactor in the future. It is essential for the T/D fuel ratio, burn control, estimation of tritium fuel cycle and alpha particle self-heating power [23] . Consequently, calculating of DT/DD neutron yield ratio has been considered as a critical task.
Experimentally, neutron detectors are positioned at certain angles and hence the measured neutrons are those emitted at these angles only. However, the total neutron yields of DD or DT reactions are required to determine the neutron yield ratio. In this section, the DT/DD neutron yield ratio over the energy range 0.01 ≤ Ed ≤ 40 MeV is calculated using a three dimensional (3-D) Monte Carlo code.
Monte Carlo Code
To calculate the neutron yield ratio of DD and DT reactions, numerical experiments have been performed by using 3-D Monte Carlo code [16] - [19] . This code is used to calculate neutron yields produced by the DD and DT reactions in high-energy density plasma up to 40 MeV. Neutron yield Yn of DD or DT reaction is calculated through the formula:
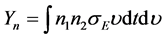 (1)
(1)
where n1 is the number density of the accelerated ions, n2 is the number of the target ions per unit volume, σE is the total cross section of the nuclear reaction for a given energy E and υ is the velocity of the accelerated ions. The total cross section σE for a given energy E is calculated by using the Drosg 2000 code. The neutron yield ratio is calculated as Yn(DT/DD) = Yn(DT)/Yn(DD) and the vice versa i.e., Yn(DD/DT) = Yn(DD)/Yn(DT).The time step, in the calculations, is taken to be enough for large number of collisions. The Monte Carlo code has been run with a large enough sample size to reduce the fluctuations in the calculated spectra.
Figures 6-9 show the results of calculations of the neutron yield ratio of DD and DT reactions in the energy range 0.01 ≤ Ed ≤ 40 MeV To get the best possible analysis of the neutron yield ratio, the total energy range has been divided into four regimes. The first regime is from 0.01 MeV to 0.14 MeV (Figure 6). In this regime, the DT/DD neutron ratio is higher than 170 with a maximum value of 334 at 0.08 MeV. The second regime is from 0.15 MeV to 0.40 MeV (Figure 7). During this regime, the DT/DD neutron ratio is higher than 10 and less than 150. The third regime is from 0.5 MeV to 2.2 MeV (Figure 8). Through this regime, the DT/DD neutron
Figure 6. DT/DD neutron yield ratio for incident deuteron energies from 0.01 to 0.14 MeV.
Figure 7. DT/DD neutron yield ratio for incident deuteron energies from 0.15 to 0.40 MeV.
Figure 8. DT/DD neutron yield ratio for incident deuteron energies from 0.5 to 2.2 MeV.
Figure 9. DD/DT neutron yield ratio for incident deuteron energies from 2.2 to 40 MeV.
ratio is less than 9.0 and it decreases to the value 1.0 at the end of the regime. The value 2.2 MeV is an inversion point as before it the DT/DD neutron ratio is more than 1.0 but after it we have the opposite situation i.e., the DD/DT neutron ratio becomes higher than 1.0. The fourth regime is from 2.2 MeV to 40 MeV (Figure 9). This is the regime that starts with the inversion point and through which the DD/DT neutron ratio is higher than one and less than two.
In summary, the DT/DD neutron ratio increases gradually from 191 at 0.01 MeV to the maximum value 334 at 0.08 MeV and then it decreases to become 1.0 at 2.2 MeV (the value at which the neutron yields of DD and DT reactions are equal). At energies higher than 2.2 MeV, the neutron yield of DD reaction becomes higher than that of the DT reaction. The energy value 0.08 MeV is the optimum energy value at which the highest DT/DD neutron yield ratio can be obtained using a mixture of D and T ions. It is worth to mention that the fast tritons produced in the DD fusion reactions may cause secondary DT reactions in flight with the D fuel ions. Neutron yield of these secondary DT reactions, in a DT mixture, is at least three orders of magnitude less than the neutron yield of the original DT reactions [2] . For this reason, the neutron yield of the secondary DT reactions is ignored in this analysis.
4. Fusion Power Ratio
The total fusion energy produced per unit volume per unit time in the burning fusion plasma is called the fusion power density of the fusion reaction. In this section, we perform a comparison between the fusion power of DD and DT reactions (achieved in high-energy density plasma relevant to laser-driven ICF) in the energy range 0.01 ≤ Ed ≤ 40 MeV. To achieve this comparison; we have derived a formula that is valid to calculate the DT/DD fusion power ratio and the DD/DT fusion power ratio using the calculated cross section values in section 2.
To calculate the fusion power density, the reaction rate (the number of fusion reactions per unit volume per unit time) has to be incorporated. The reaction rate 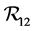 is given as:
is given as:
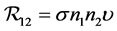 (2)
(2)
where σ is the reaction cross section, n1 is the number density of the accelerated ions, n2 is the number of the target ions per unit volume, and is the velocity of the accelerated ions. If each fusion collision generates energy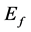 , then the fusion power density
, then the fusion power density 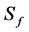 is calculated in W/m3 as:
is calculated in W/m3 as:
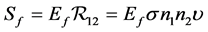 . (3)
. (3)
In the case if DT fusion fuel mixture is composite of 50% D and 50% T, then 


Consequently, the fusion power ratio 

The above formula is introduced for the first time and it is used to compare the fusion power of DD and DT fusion reactions (

Ed ≤ 40 MeV). The formula can be generalized to compare the fusion power of other fusion reactions like D-3He, D-12C, D-9Be, D-3Li, P-T and P-7Li.
For the sake of high degree of accuracy, the comparison of fusion power ratio is divided into five regimes (Figures 10-14). The first regime is from 0.01 MeV to 0.13 MeV (Figure 10). In this regime, the DT/DD fusion power ratio is more than one thousand with a maximum value of 1763 at 0.08 MeV (the same value at which the DT/DD burning ration is maximum).The second regime is from 0.14 MeV to 0.3 MeV (Figure 11). During this regime, the DT/DD fusion power ratio is higher than one hundred and less than one thousand. The third regime is from 0.4 MeV to 1 MeV (Figure 12). Through this regime, the DT/DD fusion power ratio is less than seventy and higher than ten. The fourth regime is from 1.1 MeV to 14.4 MeV (Figure 13). In this regime, the DT/DD fusion power ratio is less than ten and it decreases to the value 1.0 at the end of the regime. The value 14.4 MeV is an inversion point as before it the fusion power of the DT reaction is higher than that of the DD reaction but after it we have the opposite situation where the fusion power of the DD reaction becomes higher than that of the DT reaction. The fifth regime is from 14.4 MeV up to 40 MeV (Figure 14). This is the regime that starts with the inversion point and through which the fusion power of the DD reaction is higher than that of the DT reaction.
Figure 10. Comparison between the fusion power of DT and DD fusion reactions in the energy range 0.01 - 0.13 MeV.
Figure 11. Comparison between the fusion power of DT and DD fusion reactions in the energy range 0.14 - 0.3 MeV.
Figure 12. Comparison between the fusion power of DT and DD fusion reactions in the energy range 0.4 - 1 MeV.
Figure 13. Comparison between the fusion power of DT and DD fusion reactions in the energy range 1.1 - 14.4 MeV.
Figure 14. Comparison between the fusion power of DT and DD fusion reactions in the energy range 14.4 - 40 MeV.
5. Conclusion
In this work, an effective method for comparing the neutron yield and the fusion power of DT and DD fusion reactions is introduced. We found that the neutron yield of the DT reaction is much higher than that of the DD reaction in the energy range 0.01 - 2.2 MeV. The DT/DD neutron yield ratio reaches its maximum value at 0.08 MeV. At the energies higher than 2.2 MeV, the neutron yield of DD reaction becomes higher than that of the DT reaction but less than its double. Consequently, from the burning ratio point of view, DT reaction is the preferable fusion reaction at energies up to 2.2 MeV but the DD reaction is the preferable one at the higher energies. In addition, the fusion power of the DT reaction is higher than, but less than twice, that of the DD reaction in the energy range 0.01 - 14.3 MeV. The DT/DD fusion power ratio reaches its maximum value at 0.08 MeV. The fusion power of DD reaction becomes higher at the energies higher than 14.3 MeV. Thus, from the fusion power point of view, DT reaction is preferable fusion reaction at energies up to 14.4 MeV but the DD reaction is the preferable one at the energies higher than 14.4 MeV. From the picture presented, for a mixture of 50% deuterium and 50% triton, at 0.08 MeV both the DT/DD neutron yield ratio and the DT/DD fusion power ratio are maxima. Therefore, one can say that the energy value 0.08 MeV is the optimum value for achieving the laser-driven ICF. Now the question is whether or not the secondary fusions produced by the energetic 3H and 3He can affect this energy value. The very recent studies point out that the total probability of these secondary fusions is generally on the order of 10−2 or less [2] [26] .
Acknowledgements
The authors are very grateful to Ha’il University for funding this work.
Cite this paper
A. Youssef,M. Haparir, (2016) Achievement of Laser Fusion with High Energy Efficiency Using a Mixture of D and T Ions. Open Journal of Energy Efficiency,05,48-58. doi: 10.4236/ojee.2016.52005
References
- 1. Tabak, M., Hammer, J., Glinsky, E.M., Kruer, L.W., Wilks, C.S., Woodworth, J., Campbell, E.M. and Perry, D.M. (1994) Ignition and High Gain with Ultrapowerful Lasers. Physics of Plasmas, 1, 1626.
http://dx.doi.org/10.1063/1.870664 - 2. Munro, D.H. (2016) Interpreting Inertial Fusion Neutron Spectra. Nuclear Fusion, 56, Article ID: 036001.
http://dx.doi.org/10.1088/0029-5515/56/3/036001 - 3. Tabak, M. (1996) What Is the Role of Tritium-Poor Fuels in ICF? Nuclear Fusion, 36, 147.
http://dx.doi.org/10.1088/0029-5515/36/2/I03 - 4. Atzeni, S. and Chiampi, M.L. (1997) Burn Performance of Fast Ignited, Tritium-Poor ICF Fuels. Nuclear Fusion, 37, 1665. http://dx.doi.org/10.1088/0029-5515/37/12/i01
- 5. Feoktistov, L.P. (1998) Thermonuclear Detonation. Physics-Uspekhi, 41, 1139.
http://dx.doi.org/10.1070/PU1998v041n11ABEH000506 - 6. Shmatov, M.L. (2010) Optimum Variant of DD Fusion Ignition for Thermonuclear Power Plants. Technical Physics Letters, 36, 386.
- 7. Kodama, R., Shiraga, H., Shigemori, K., Toyama, Y., Fujioka, S., Azechi, H., Fujita, H., Habara, H., Hall, T., Izawa, Y., Jitsuno, T., Kitagawa, Y., Krushelnik, M.K., Lancaster, L.K., Mima, K., Nagai, K., Nakai, M., Nishimura, H., Norimatsu, T., Norreys, A.P., Sakabe, S., Tanaka, A.K., Youssef, A., Zepf, M. and Yamanaka, T. (2002) Fast Heating Scalable to Laser Fusion Ignition. Nature, 418, 933.
- 8. Jarvis, N.O. (2002) Neutron Spectrometry at JET (1983-1999). Nuclear Instruments and Methods in Physics Research Section A, 476, 474-484.
http://dx.doi.org/10.1016/S0168-9002(01)01493-0 - 9. Kallne, J., Gorini, G. and Ballabio, L. (1997) Feasibility of Neutron Spectrometry Diagnostic for the Fuel Ion Density in DT Tokamak Plasmas. Review of Scientific Instruments, 68, 581.
http://dx.doi.org/10.1063/1.1147658 - 10. Okada, K., Kondo, K., Sato, S., et al. (2006) Development of Neutron Measurement System for nd/nt Fuel Ratio Measurement in ITER Experiments. Review of Scientific Instruments, 77, Article ID: 10E726.
- 11. Elevant, T., Aronsson, D., Belle, V.P., et al. (1991) The JET Neutron Time-of-Flight Spectrometer. Nuclear Instruments and Methods in Physics Research Section A, 306, 331-342.
http://dx.doi.org/10.1016/0168-9002(91)90340-v - 12. Gorini, G., Kallne, J. and Ballabio, L. (1997) Neutron Spectrometry for Plasma Rotation. Review of Scientific Instruments, 68, 561.
http://dx.doi.org/10.1063/1.1147654 - 13. Giacomelli, L., Hjalmarsson, A., Sjostrand, H., et al. (2005) Advanced Neutron Diagnostics for JET and ITER Fusion Experiments. Nuclear Fusion, 45, 1191.
http://dx.doi.org/10.1088/0029-5515/45/9/019 - 14. Elevant, T., Belle, V.P., Jarvis, N.O., et al. (1995) Measurements of Fusion Neutron Energy Spectra at JET by Means of Time-of-Flight Techniques. Nuclear Instruments and Methods in Physics Research Section A, 364, 333-341.
http://dx.doi.org/10.1016/0168-9002(95)00346-0 - 15. Asai, K., Yukawa, K., Iguchi, T., Iwai, H., Naoi, N. and Kawarabayashi, J. (2008) Improvement of Multi-Scattering Time-of-Flight Neutron Spectrometer to Measure the D/T Ratio in a Fusion Experimental Reactor. Journal of Nuclear Science and Technology, 6, 69-72.
http://dx.doi.org/10.1080/00223131.2008.10875979 - 16. Youssef, A., Kodama, R., Habara, H., Tanaka, A.K., Sentoku, Y., Tampo, M. and Toyama, Y. (2005) Broad-Range Neutron Spectra Identification in Ultraintense Laser Interactions with Carbon-Deuterated Plasma. Physics of Plasmas, 12, Article ID: 110703.
http://dx.doi.org/10.1063/1.2131847 - 17. Youssef, A., Kodama, R. and Tampo, M. (2006) Investigation of Laser Ion Acceleration inside Irradiated Solid Targets by Neutron Spectroscopy. Physics of Plasmas, 13, Article ID: 030701.
http://dx.doi.org/10.1063/1.2177230 - 18. Youssef, A., Kodama, R. and Tampo, M. (2006) Study of Proton Acceleration at the Target Front Surface Laser-Solid Interactions by Neutron Spectroscopy. Physics of Plasmas, 13, Article ID: 030702.
http://dx.doi.org/10.1063/1.2183707 - 19. Youssef, A. and Kodama, R. (2010) Neutron Production in Ultraintense Laser Interactions with Carbon-Deuterated Plasma at Intensities of 1018 W/cm2. Nuclear Fusion, 50, 035010.
- 20. Youssef, A. (2013) Neutron Yields of Nuclear Reactions Induced by Ion Acceleration in Carbon-Deuterated Plasma Produced by Ultra-Intense Lasers. Physica Scripta, 87, Article ID: 015501.
http://dx.doi.org/10.1088/0031-8949/87/01/015501 - 21. Youssef, A. (2016) Counting of Ultraintense Laser-Driven Neutrons from the Pulse Height of Time-of-Flight Detector Includes Ultrafast Timing Plastic Scintillator. Journal of Instrumentation, 11, Article ID: P02009.
http://dx.doi.org/10.1088/1748-0221/11/02/P02009 - 22. Sunahara, A., Johzaki, T., Nagatomo, H., Mima, K., Shiraga, H., Azechi, H., Mori, Y. and Kitagawa, Y. (2016) Direct Heating of Imploded Plasma in the Fast Ignition. Journal of Physics: Conference Series, 688, Article ID: 012114.
http://dx.doi.org/10.1088/1742-6596/688/1/012114 - 23. Asai, K., Naoi, N., Iguchi, T., Watanabe, K., Kawarabayashi, J. and Nishitani, T. (2006) Neutron Spectrometer for DD/DT Burning Ratio Measurement in Fusion Experimental Reactor. Journal of Nuclear Science and Technology, 43, 320-324.
http://dx.doi.org/10.1080/18811248.2006.9711097 - 24. Drosg, M. (2000) International Atomic Energy Agency (IAEA)-Nuclear Data Services (NDS)-Rev. 5, January (2000), Version 2.21 (May 2005).
- 25. Liskien, H. and Paulsen, A. (1973) Neutron Production Cross Sections and Energies for the Reactions T(p, n)3He, D(d, n)3He, and T(d, n)4 He. Nuclear Data Tables, 11, 569.
http://dx.doi.org/10.1016/S0092-640X(73)80081-6 - 26. Rinderknecht, H.G., Rosenberg, M.J., Zylstra, A.B., Lahmann, B., Seguin, F.H, Frenje, J.A., Li, C.K., Gatu Johnson, M., Petrasso, R.D., Berzak Hopkins, L.F., Caggiano, J.A., Divol, L., Hartouni, E.P., Hatarik, R., Hatchett, S.P., Le Pape, S., Mackinnon, A.J., McNaney, J.M., Meezan, N.B., Moran, M.J., Bradley, P.A., Kline, J.L., Krasheninnikova, N.S., Kyrala, G.A., Murphy, T.J., Schmitt, M.J., Tregillis, I.L., Batha, S.H., Knauer, J.P. and Kilkenny, J.D. (2015) Using Multiple Secondary Fusion Products to Evaluate Fuel ρR, Electron Temperature, and Mix in Deuterium-Filled Implosions at the NIF. Physics of Plasmas, 22, Article ID: 082709.
http://dx.doi.org/10.1063/1.4928382
NOTES
*Corresponding author.
















