Received 3 January 2015; accepted 16 April 2015; published 22 April 2015

ABSTRACT

In this work, the 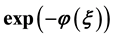-expansion method is used for the first time to investigate the exact traveling wave solutions involving parameters of nonlinear evolution equations. When these parameters are taken to be special values, the solitary wave solutions are derived from the exact traveling wave solutions. The validity and reliability of the method are tested by its applications to Nano-ionic solitons wave’s propagation along microtubules in living cells and Nano-ionic currents of MTs which play an important role in biology.

Keywords:

The 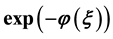-Expansion Method, Nano-Solitons of Ionic Wave’s Propagation along Microtubules in Living Cells, Nano-Ionic Currents of MTs, Traveling Wave Solutions, Kink and Anti Kink Wave Solutions

1. Introduction

The nonlinear partial differential equations of mathematical physics are major subjects in physical science [1] . Exact solutions for these equations play an important role in many phenomena in physics such as fluid mechanics, hydrodynamics, Optics, Plasma physics and so on. Recently many new approaches for finding these solutions have been proposed, for example, tanh-sech method [2] -[4] , extended tanh-method [5] -[7] , sine-cosine method [8] -[10] , homogeneous balance method [11] [12] , F-expansion method [13] -[15] , exp-function method

[16] [17] , trigonometric function series method [18] , 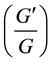expansion method [19] -[22] , Jacobi elliptic function method [23] -[26] , The exp-expansion method [27] -[29] and so on.

The objective of this article is to investigate more applications than obtained in [27] -[29] to justify and dem-

onstrate the advantages of the exp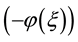-method. Here, we apply this method to Nano-solitons of ionic

waves’s propagation along microtubules in living cells and Nano-ionic currents of MTs.

2. Description of Method

Consider the following nonlinear evolution equation

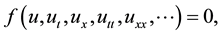(2.1)

where F is a polynomial in 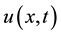and its partial derivatives in which the highest order derivatives and nonlinear terms are involved. In the following, we give the main steps of this method.

Step 1. We use the wave transformation

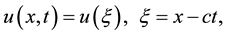(2.2)

where c is a positive constant, to reduce Equation (2.1) to the following ODE:

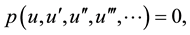(2.3)

where P is a polynomial in u(ξ) and its total derivatives.

Step 2. Suppose that the solution of ODE (2.3) can be expressed by a polynomial in 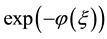as follow

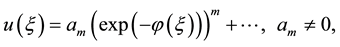(2.4)

where 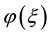satisfies the ODE in the form

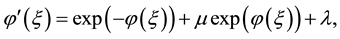(2.5)

The solutions of ODE (2.5) are

When,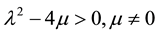

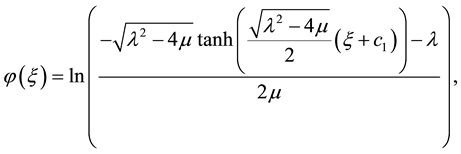(2.6)

When,

(2.7)

When

(2.8)

When

(2.9)

When

(2.10)

where are constants to be determined later,

Step 3. Substitute Equation (2.4) along Equation (2.5) into Equation (2.3) and collecting all the terms of the same power and equating them to zero, we obtain a system of algebraic equations, which can be solved by Maple or Mathematica to get the values of.

Step 4. substituting these values and the solutions of Equation (2.5) into Equation (2.3) we obtain the exact solutions of Equation (2.1).

3. Application

3.1. Example 1: Nano-Solitons of Ionic Wave’s Propagation along Microtubules in Living Cells [27]

We first consider an inviscid, incompressible and non-rotating flow of fluid of constant depth (h). We take the direction of flow as x-axis and z-axis positively upward the free surface in gravitational field. The free surface elevation above the undisturbed depth h is, so that the wave surface at height, while z = 0 is horizontal rigid bottom.

Let be the scalar velocity potential of the fluid lying between the bottom (z = 0) and free space, then we could write the Laplace and Euler equation with the boundary conditions at the surface and the bottom, respectively, as follows:

(3.1)

(3.2)

(3.3)

(3.4)

It is useful to introduce two following fundamental dimensionaless parameters:

(3.5)

where is the wave amplitude, and l is the characteristic length-like wavelength. Accordingly, we also take a complete set of new suitable non-dimensional variables:

(3.6)

where is the shallow-water wave speed, with g being gravitational acceleration. In term of (3.5) and (3.6) the initial system of Equations (3.1)-(3.4) now reads

(3.7)

(3.8)

(3.9)

(3.10)

Expanding in terms of δ

(3.11)

and using the dimensionless wave particles velocity in x-direction, by definition then substituting of (3.11) into (3.7)-(3.9), with retaining terms up to linear order of small parameters in (3.8), and second order in (3.9), we get

(3.12)

(3.13)

Making the differentiation of (3.12) with respect to, and rearranging (3.13), we get

(3.14)

(3.15)

Returning back to dimensional variables and, (3.14) now reads

(3.16)

We could define the new function unifying the velocity and displacement of water particles as follows:

(3.17)

implying that (3.16) becomes

(3.18)

We seek for traveling wave solutions with moving coordinate of the form and with wave speed, which reduces Equation (3.18) into ordinary nonlinear differential equation as follows:

(3.19)

Integrating Equation (3.19) once, and setting, we get

(3.20)

Balancing and yields,. Therefore, we can write the solution of Equation (3.20) in the form

(3.21)

(3.22)

(3.23)

Substituting (3.21) along (3.23) into (3.20), setting the coefficients of, , , , to zero, we obtain the following underdetermined system of algebraic equations for (a₀, a₁, a₂):

(3.24)

(3.25)

(3.26)

(3.27)

(3.28)

Solving the above system with the aid of Mathematica or Maple, we have the following solution:

Sothat the solution of Equation (3.20) will be in the form:

(3.29)

Consequently, the solution takes the forms:

When

(3.30)

When

(3.31)

When

(3.32)

When

(3.33)

When

(3.34)

3.2. Example 2. Nano-Ionic Currents of MTs

The Nano-ionic currents are elaborated in [27] take the form

(3.35)

where R = 0.34 × 10⁹ Ω is the resistance of the ER with length, l = 8 × 19⁻⁹ m, c₀ = 1.8× 10⁻¹⁵ F is the maximal capacitance of the ER, G₀ = 1.1 × 10⁻¹³ si is conductance of pertaining NPs and z = 5.56 ×10¹⁰ Ω is the characteristic impedance of our system parameters δ and describe nonlinearity of ER capacitor and conductance of NPs in ER, respectively. In order to solve Equation (3.35) we use the travelling wave transformations, with, to reduce Equation (3.35) to the following nonlinear ordinary differential equation:

(3.36)

Which can be written in the form

(3.37)

where

(3.38)

Thus Equation (3.37) take the form

(3.39)

Balancing and yields,. Consequently, we get

(3.40)

Where a₀, a₁, a₂ are arbitrary constants such that a₂ ≠ 0. From Equation (3.40), it is easy to see that

(3.41)

(3.42)

Substituting Equations (3.40)-(3.42) into Equation (3.39) and equating the coefficients of , , , , to zero, we obtain

(3.43)

(3.44)

(3.45)

(3.46)

(3.47)

(3.48)

Solving above system with the aid of Mathematica or Maple, we have the following solution:

a₁ = a₁, a₂ = a₂.

So that the solution of Equation (3.39) will be in the form:

(3.49)

Consequently, the solution take the forms:

When,

(3.50)

When,

(3.51)

When,

(3.52)

When,

(3.53)

When,

(3.54)

4. Results and Conclusion

In nanobiosciences the transmission line models for ionic waves propagating along microtubules in living cells play an important role in cellular signaling where ionic wave’s propagating along microtubules in living cells shaped as nanotubes that are essential for cell motility, cell division , intracellular trafficking and information processing within neuronal processes. ionic waves propagating along microtubules in living cells have been also implicated in higher neuronal functions, including memory and the emergence of consciousness and we presented an inviscid, incompressible and non-rotating flow of fluid of constant depth (h). The -ex- pansion method has been successfully used to find the exact traveling wave solutions of some nonlinear evolution equations and Figure 1 and Figure 2 show the solitary wave solution of both equations. As an application,

the traveling wave solutions for As an application, the traveling wave solutions for Nano-ionic solitons wave’s propagation along microtubules in living cells and Nano-ionic currents of MTs, which have been constructed using the -expansion method. Let us compare our results obtained in the present article with the well-known results obtained by other authors using different methods as follows: Our results of Nano-ionic solitons wave’s propagation along microtubules in living cells and Nano-ionic currents of MTs [27] . It can be concluded that this method is reliable and propose a variety of exact solutions NPDEs. The performance of this method is effective and can be applied to many other nonlinear evolution equations.

References