Open Journal of Applied Sciences
Vol.09 No.02(2019), Article ID:90726,13 pages
10.4236/ojapps.2019.92006
Development of a Time-Efficient Approach to Measure the Acoustic Impedance of Industrial Insulating Materials
Etaribo Binaebi-Soroh1*, Diogo Montalvao2
1University of Hertfordshire, Hatfield, UK
2Department of Design and Engineering, Bournemouth University, Bournemouth, UK

Copyright © 2019 by author(s) and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: January 20, 2019; Accepted: February 23, 2019; Published: February 26, 2019
ABSTRACT
This study presents a development on a time efficient approach to measure the Acoustic Impedance of Industrial Insulating materials using an impedance tube with single moveable microphone and a white noise generator. The standing wave method is used to measure the acoustic properties (absorption coefficient and acoustic impedance) of sound absorbing materials. In order to use a burst of white noise instead of individual stationary sine waves, a signal processing technique was developed. The algorithm is based on the equation of simple harmonic motion, but uses distance as a variable, instead of time. This innovative method allows measuring at frequency resolutions as low as 5 Hz in a reasonably short amount of time. This is an advantage, as the classical standing wave method uses frequency resolutions of one-third of octaves for measurements, as otherwise time would be prohibitive. Experimental results are validated with those measured with the sine wave generator using one-third of octave frequencies by comparing their behaviours.
Keywords:
Acoustic Impedance, White Noise, Sound, Absorption Coefficient

1. Introduction
The world is full of vibrating objects that order the motion of longitudinal pressure waves in the air [1] . Perceived by Humans as sound which, when unwanted and disturbing, is designated noise. Noise is a characteristic of many activities and processes and is present in most industrial areas. The uncontrolled level of noise that the human ears may be exposed to is capable of impairing human hearing abilities [2] . Industrial processes and machinery include different sources of noise such as those from gears, compressors, pumps, conveyors, rotors, fans, stators, electrical machines and internal combustion engines [3] .
In industrial surroundings sound fields are usually complex because of the presence of many sources which include propagation through solids, through air, diffraction at machinery boundaries, absorption on the surfaces and reflection from floor. Also, sound pressure level formed is dependent on the kind of noise source, the nature of the work place and the distance from which the receiver is from the source [3] .
That said, noise also influences complex task performance, causing annoyance and modifies social behaviours [4] . Standards were set for noise reduction devices, sound absorption materials and noise-related measuring procedures. Examples of such standards include: ISO 11654 and ISO 10534 for noise absorbers; ISO 11546 and ISO 11957 for noise control enclosures; ISO 7235, ISO 11691, ISO 11820, ISO 14163 for silencers; and ISO 11821 and ISO 10053 for noise baffles [3] .
The acoustic impedance (that is, the ratio between the pressure of the reflected and the transmitted/absorbed wave when hitting a medium) of sound absorption materials can be measured, for example, using an impedance tube. The transmission loss (TL) is measured at normal incidence in a traditional impedance tube experimentally to investigate the influence of granules size in silica aerogels in terms of thermal and acoustic performance characteristics, whereas the thermal conductivity (λ) is evaluated using a Hot Plate apparatus. The results show that the small granules (granules size in the 0.01 - 1.2 mm range), which have the highest density, have the best performance both in terms of thermal and acoustic properties. Depending on the granules size, λ varies in 19 - 22 mW/mK range at 10˚C, whereas a TL equal to 13 dB at about 6400 Hz for 20 mm thickness is obtained for small granules [5] .
[6] investigated the thermal and acoustic properties of innovative basalt natural fibre insulating panels by means of a Heat Flow meter apparatus: it is included in 0.030 - 0.034 W/mK range. The acoustic absorption coefficient was determined by means of Kundt’s Tube. The results were compared with traditional solutions with related chemical composition, but worse mechanical resistance. The use of 2 and 4 microphones was compared to estimate the normal incidence absorption coefficient of some fibrous porous materials [7] . The ASTM E1050-08 standard was used, in which the rear surface of the sample was placed adjacent to the hard end termination of the tube. The procedure was also simulated in Patran, and results obtained from calculation and simulated absorption plots produced similar curves. It was also found that the frequency range that is measurable depends on both the tube diameter and the microphones’ positions.
Impedance tube was used with a moveable microphone to investigate sound absorption properties of acoustic materials with different densities. Conclusion was made that the absorption coefficients of the samples investigated depend not only on the composition of the mixture, but also change with frequency up to a peak value that then decreases [8] , also experiment comparing Results between the absorption coefficient and acoustic impedance using two different methods, which are the standing wave tube and a free field method. Standing wave tube calibration is applicable for lower frequencies from 100 Hz - 4000 Hz, while the free field calibration is used for frequencies from 200 Hz - 20,000 Hz. The effect of the constraint on the tube wall on the acoustic absorption coefficient of porous material that are elastic was analysed and arrived at a conclusion that friction between the tube and material was to harden the material and proved that the impedance measurement should be carried out carefully in conditions where elasticity of the frame also aid surface impedance of the material [9] .
Generating three different layers of industrial tea leaves waste with or without a major backing layer of textile woven material to test the properties of sound absorption revealed that the sound absorption properties increased when the thickness layer of the major single backing cotton material is increased, which indicates that natural materials and renewable materials have positive sound attenuation properties, and more significantly, cause less harm to human health [10] . Also, the increase of sound absorption occurs only at low frequencies, as the material thickness is being increased, and at higher frequencies the thickness has no significant effect on sound absorption [11] . That said, [12] revealed that the sound absorption range value increases at the central and higher frequency as the density of the material is increased. The reason is because the number of the fibres increases per unit area when the supposed density is large, thus, increasing energy losses due to surface friction increases. The present study is to develop a method for impedance tubes that uses a burst of white noise instead of individual stationary sine waves. This will make the measurement of the acoustic impedance in tubes more time efficient and allow a significant increase in the frequency resolution.
2. Materials and Methods
Aglomex, Impactodan, National Instrument (NI) LabVIEW and National Instrument equipment such as NI 9263, NI 9234, NI CDAQ 9174 for data acquisition and processing, one microphone, loudspeaker, Impedance tubes, Power Amplifier, computer, meter rule, developed NI LabVIEW application for white noise generation. NI LabVIEW is “a highly productive development environment for creating custom applications that contains all the tools engineers and scientists need to design and deploy measurement and control systems” [13] .
The one microphone method has a distinct advantage in that it avoids the somewhat complicated calibration procedure that is necessary for transfer function measurements, when compared with 2 - 3 microphones method and reverberation chamber although provides a quick broadband alternative. Impedance tubes are designed for measuring the normal incidence absorption coefficient and normal specific impedance of a wide range of acoustic materials. Materials for noise control can be tested in terms of their sound absorption, reflection and transmission properties in a standing wave tube to ensure highly repeatable test conditions. This information about the material’s acoustic properties can be used, for example, for calibrating and validating computational methods used to forecast the acoustic performance of multi-layer systems.
2.1. Samples Description
Materials used in this research were sourced by Pronorma, a Portuguese company working in the field of Acoustic sampling of structures (Table 1).
The Impedance tube measurements using the standing wave ratio method (BS_EN_ISO 10534-1:2001) was used having one microphone that is moveable to probe at different position points, and the samples are circular in shape placed at one end of the tube. A picture of the experimental setup is shown in Figure 1. Using the standing wave method, a white noise sound source is placed one end of the tube, and terminate at the other end containing the test sample. Once the sound is initiated a standing wave pattern develops in the tube, and a microphone move through the length of the tube starting at the loudspeaker end and moving a 1 cm distance for each measurement and terminating at the sample end. The developed software records the location and level of the first extrema along with the following minima and maxima. From this information the sound absorption coefficient and specific acoustic impedance of samples can be calculated. This is done with multiple frequency of 5 Hz resolution from 0 Hz to 6500 Hz at 1 cm distance at a time, with a complete scan through the entire tube distance.
Frequency resolution of 5 Hz is used together with each 1 cm measurement of the sample testing, and a maximum frequency of 6500 Hz is specified. The 5 Hz resolution enables a measurement to be made for a multiple of 5 Hz to 6500 Hz
Table 1. Showing the material samples and their applications.
Figure 1. Experimental Setup showing the equipment and instruments used.
for each cm distance moved by the microphone. Thus, measurement can be done for not just one third of octaves but rather for the resolution selected for the sample testing. This implies that if a 1 Hz resolution is selected for the program, then a measurement can be taken for every 1 Hz and its increment up to maximum frequency that is to be reached. The choice of 1 cm and 5 Hz was selected to reduce any form aliasing that might influence the results.
Aliasing arises when a signal is measured at an insufficient sampling rate to capture the changes of that signal. This implies that there is the existence of some unwanted components in the reconstructed signal that were not present in the original signal that was sampled. Aliasing exist because signal frequencies overlap some times when the sampling frequency is too low or smaller than the signal being measured [14] .
2.2. Measuring the Acoustic Impedance in a Standing Wave Tube with White Noise
White noise is statistically a random signal with its power spread uniformly across the signal frequency domain. That is, it has a flat power spectral density in which signal at any frequency has the same power within a fixed bandwidth which is the difference between frequencies of lower and upper limits in a continuous set of frequencies measured in Hertz.
Figure 2 is the flowchart demonstrating the performance of the developed white noise generator using the Advanced National Instrument LabVIEW in generating the sound, acquiring the data and processing the data to calculate the acoustic absorption coefficient and acoustic impedance of samples tested.
Frequency spectrum makes the description of the signals much simpler by clearly showing these signals as harmonics. The frequency spectrum of the amplitude versus time which is also the time-domain signal can be represented as a signal in the frequency domain through the use of the Fast Fourier Transform (FFT). The FFT “breaks down” a cycle of random waveforms or signals into sine wave components of amplitude and frequency.
To measure the Acoustic Impedance in an Impedance tube, one starts by defining the Standing Wave Pressure Ratio SWR as:
(1)
where is the pressure maximum of the standing wave in the tube, is the pressure minimum and:
(2)
is the ratio coefficient between the reflected and incident wave amplitude.
In this paper, a new approach to model a standing wave in a tube is proposed. Figure 3 shows a real example of experimental data recorded along the length of an impedance tube for a harmonic signal. This wave, which resembles (and it is) a harmonic time signal, could be represented in the spatial domain instead, where it can be described as a function of distance travelled d instead of time t.
The harmonic waveform that corresponds mathematically with the sine function in the time domain is:
Figure 2. Experimental flowchart for the white noise generator.
Figure 3. Plot showing the amplitude vs distance for a standing wave with a frequency of 1700 Hz.
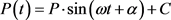 (3)
(3)
where is the angular frequency of the wave, C is the offset mean pressure and is the phase angle, where the latter two quantities cab be obtained from:
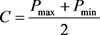 (4)
(4)
 (5)
(5)
Since each spectral component of the white noise is similar in pattern to that of the harmonic waveform (after application of the FFT), and following the earlier line of thought that harmonic spectral components can be represented as a sinusoidal function of the distance d instead of time t, the waveform shown in Figure 3 is suggested to be written in this paper as:
 (6)
(6)
Making the analogy between equations (3) and (6), we have:
The values for and are extracted with the help of the software developed in LabVIEW, where:
 (7)
(7)
(8)
(9)
Taking into account the phase angle, the distance of the first minimum from the sample being measured is determined from:
 (10)
(10)
If , then the value from Equation (10) must be amended to:
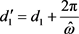 (11)
(11)
since it does not make sense to have a negative distance. The distance from the sample to the second minimum can be determined from the wavelength ; hence:
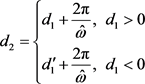 (12)
(12)
Therefore, with the above equation producing and , the real and imaginary components of the acoustic impedance can be calculated from:
 (13)
(13)
 (14)
(14)
where is the density of air, c is the speed of sound in the tube and is the phase angle between the incident and reflected sound pressure:
 (15)
(15)
With the Real and Imaginary components of the Acoustic Impedance (Equations (13) and (14)), the Acoustic Impedance in rayls can finally be determined from:
 (16)
(16)
When using white noise, the measurement is made with the impedance tube’s car (with microphone) continuously moving from 90 cm to 0 cm distance from the sample. The programme developed in LabVIEW uses the approach presented above to post-process the data and determine the Acoustic Impedance of the sample materials as a function of frequency in an automatic fashion and with a much higher frequency resolution, as shown in the Results and Analysis section.
3. Results and Analysis
The acoustic measurements were carried out by the impedance tube with the setup that contains a single microphone that converts sound pressure into electrical signals which is captured and displayed on the developed software and is analysed to view the effect and relationship of the materials tested and their acoustic impedance.
For the sine wave, software measurement of a sample at a given frequency is done twice, one is to measure the pressure minima while the second is to measure the pressure maxima, while with the white noise generator software only one set of measurement is taken for the selected frequencies from 0 Hz to 6500 Hz with 5 Hz resolution and distances from 0 to 90 cm.
The acoustic impedance at a particular frequency suggests how much sound pressure is produced at a given air vibration at that frequency. That is, a material with a low acoustic impedance will hinder the movement of sound energy less than when a high acoustic impedance is allowed to passed through a medium, this means that the higher the acoustic impedance the lower the absorption coefficient of that sample. Thus, the developed software using National Instrument LabVIEW and National Instrument equipment for acquisition of data and processing of the data easily thereby, producing the information analysed below.
Using the white noise generator and the impedance tube reveals that the frequency range of 0 Hz to 89 Hz is measurable but not with one-third of octave, also using the white noise it was observed that the chart for absorption coefficient decreases from 1 - 0.5, at frequency from 0 Hz - 295 Hz. It then gradually increases back to 0.82 for the absorption coefficient. Figure 4 and Figure 5 show absorption coefficient results for Aglomex sample using one-third of octave and white noise respectively.
The result in Figure 6 shows the sample of Aglomex being validated by overlapping the results produced with one-third of octave and that with white noise. This reveals a matching result between the sine wave generator and the white noise generator. This implies that the new method is also reliable and can give validated results.
Figure 7 reveals an improvement from Figure 4 without air gap using sine wave. This also increase steadily from 100 Hz to 500 Hz then tries to maintain a steady absorption coefficient of 0.82 from the 500 Hz to about 2000 Hz which corresponds
Figure 4. Absorption coefficient of Aglomex sample using sine wave with one-third of octave.
Figure 5. Absorption coefficient of Aglomex sample using white noise with 5 Hz resolution.
Figure 6. Overlapping of the sine wave using one-third of octave and white noise with 5 Hz resolution for Aglomex sample.
Figure 7. Absorption coefficient of Aglomex sample with 5mm air gap using sine wave with one-third of octave.
Figure 8. Absorption coefficient of Aglomex sample with 5mm air gap using white noise with 5 Hz resolution.
with the investigation executed by [11] [12] .
Figure 8 also reveals an improvement from Figure 5 without air gap using the white noise. With the introduction of air gap shows that the absorption coefficient is not increased, but rather create a steady state of absorption and reduce fluctuation.
It was also observed in this measurement also that from above 2500 Hz the analyser could no longer average the frequencies due to noise measurement, and this was observed with all the samples tested. This is because the impedance tube 4002 used is stated to measure 2000 Hz.
Figure 9 shows validation by overlapping the results in which air gap was introduced to the sample using white noise, with that of the sine wave generator. This reveals a more matching result between the sine wave generator and the white noise generator.
Figure 10 shows absorption coefficient measurement of Impactodan sample using white noise.
The measurement of the sample shown in Figure 11 reveals a very good result when the two samples are combined in the order they are mentioned. It was observed that for the absorption coefficient chart shown in Figure 11 shows a better absorption coefficient performance with a gradual steady increase in the effect of the absorption coefficient with little fluctuations when compared with the sample measured in Figure 10.
Figure 9. Overlapping of sine wave one-third of octave and white noise with 5 Hz resolution for Aglomex with air gap combined together.
Figure 10. Absorption coefficient of Impactodan sample using white noise with 5 Hz resolution.
Figure 11. Absorption coefficient of Aglomex_Impactodan sample using white noise with 5 Hz resolution.
4. Conclusions
The development of a time efficient approach to measure the Acoustic Impedance of Industrial Insulating Materials in a Standing Wave Impedance tube was presented. It was observed that the method, by significantly increasing the frequency resolution (rather than using fixed octave bandwidths as previously done), does produce a wider spread of results that are consistent with the expected outcomes. This is because unlike using harmonic time signals (i.e., at a single frequency), the white noise generator can cover a wide range of frequencies from 0 Hz to 6500 Hz with 5 Hz resolution in a test that is easy and quick to implement.
Experimental results suggest that some materials when used alone show poor insulating characteristics. This is because of their high impedance characteristic like the Impactodan sample. However, these materials can be used as backing for other samples as shown in Figure 11 to boost the insulating properties of the new samples that they are combined with; hence, improving the absorption coefficient and acoustic impedance of industrial insulating materials.
It can be concluded that this procedure of measurement of the sound absorption coefficient and acoustic impedance is a more time efficient approach of measuring the Acoustic Impedance of industrial sound insulation materials.
Conflicts of Interest
The authors declare no conflicts of interest regarding the publication of this paper.
Cite this paper
Binaebi-Soroh, E. and Montalvao, D. (2019) Development of a Time-Efficient Approach to Measure the Acoustic Impedance of Industrial Insulating Materials. Open Journal of Applied Sciences, 9, 55-67. https://doi.org/10.4236/ojapps.2019.92006
References
- 1. Cavanaugh, J.W., Tocci, C.G. and Wilkes, A.J. (2009) Architectural Acoustics: Principles and Practice. Wiley, New York.
- 2. Hellmuth, T., Classen, T., Kim, R. and Kephalopoulos, S. (2012) Methodological Guidance for Estimating the Burden of Disease from Environmental Noise. WHO Regional Office for Europe.
- 3. Sehrndt, G.A., Parthey, W. and Gerges, S.N.Y. (2019) Noise Control. http://www.who.int/occupational_health/publications/noise5.pdf
- 4. Stansfeld, S.A. and Matheson, M.P. (2003) Noise Pollution: Non-Auditory Effects on Health. British Medical Bulletin, 68, 243-257. https://doi.org/10.1093/bmb/ldg033
- 5. Moretti, E., Merli, F., Cuce, E. and Buratti, C. (2017) Thermal and Acoustic Properties of Aerogels: Preliminary Investigation of the Influence of Granule Size. Energy Procedia, 111, 472-480. https://doi.org/10.1016/j.egypro.2017.03.209
- 6. Buratti, C., Moretti, E., Belloni, E. and Agosti, F. (2015) Thermal and Acoustic Performance Evaluation of New Basalt Fibre Insulation Panels for Buildings. Energy Procedia, 78, 303-308. https://doi.org/10.1016/j.egypro.2015.11.648
- 7. Kunio, J., Yoo, T., Jou, K., Bolton, J.S. and Enok, J. (2009) A Comparison of Two and Four Microphone Standing Wave Tube Procedures for Estimating the Normal Incidence Absorption Coefficient. 38th International Congress and Exposition on Noise Control Engineering 2009, Ottawa, 23-26 August 2009, 1057-1065.
- 8. Asuquo, U.E., Obisung E.O. and Faithpraise, F.O. (2010) Sound Absorbing Properties of Different Density Local Acoustic Materials. International Research Journals, 1, 39-41.
- 9. Vigran, T.E., Kelders, L., Lauriks, W., Leclaire, P. and Johansen, T.F. (1997) Prediction and Measurements of the Influence of Boundary Conditions in a Standing Wave Tube. Acta Acustica United with Acustica, 83, 419-423.
- 10. Ersoy, S. and Küçük, H. (2009) Investigation of Industrial Tea-Leaf-Fibre Waste Material for Its Sound Absorption Properties. Applied Acoustics, 70, 215-220. https://doi.org/10.1016/j.apacoust.2007.12.005
- 11. Ibrahim, M.A. and Melik, R.W. (1978) Physical Parameters Affecting Acoustic Absorption Characteristics of Fibrous Materials. Proceedings of the Mathematical and Physical Society of Egypt, 46, 125-130.
- 12. Koizumi, T., Tsujiuchi, N. and Adachi, A. (2002) The Development of Sound Absorbing Materials Using Natural Bamboo Fibres. WIT Transactions on the Built Environment, 59, 157-166.
- 13. NI (2017) https://forums.ni.com/t5/Past-NIWeek-Sessions/NIWeek-2013-Introduction-to-NI-LabVIEW/ta-p/3502242
- 14. Norton, M. and Karczub, D. (2003) Acoustic Barriers. In: Fundamentals of Noise and Vibration Analysis for Engineers, 2nd Edition, Cambridge University Press, Cambridge, 315-316.













