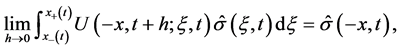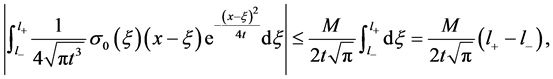ABSTRACT

There are many works (i.e. [1] ) aiming to find out numerically how positive feedback affects the formation of invadopodia and invasion of cancer cells; however, studies on the cancer cell invasion model with free boundary are fairly rare. In this paper, we study modified cancer cell invasion model with free boundary, where, free boundary stands for cancer cell membrane, so that we can more precisely describe the positive feedback affects. Firstly, we simplized the model by means of characteristic curve and semi-groups’ property, and obtained the Stefan-like problem by introducing Gaussian Kernel and Green function. Secondly, based on the classical Stefan problem, we derived the integral solution of simplified model, which could lead us a further step to find the solution of modified cancer cell invasion model.

Keywords:

Invadopodia, Cancer, Stefan Problem, Free Boundary

1. Introduction

As well known, cancer disease is one of the leading causes of death worldwide. Many natural and man-made factors (for example, smoking, car exhaust fumes, ultraviolet rays, air pollution and radiations, etc.) are the main risks for cancer disease. Metastasis is a leading death process, in which two processes are crucial from the viewpoint of cancer therapy. The first one is angiogenesis, nucleation of new blood vessels, which can provide enough nutrients to further development of tumor cells. The other one is tissue invasion. After the vascular growth of the tumor, cells become more aggressive that it can invade into the extracellular matrix, even into blood vessels, to complete the metastasis. Tissue invasion is a process in which cells can migrate and establish a new colony in new organs.

There are many studies about angiogenesis inhibition, because cancer cells have a certain size and cannot grow further without nutrients from blood vessels. Signaling molecule VEGF (vascular endothelial growth factor) can be secreted by cancer cells and can bind the normal endothelial cells to form new blood vessels. Scientists found inhibitors, such as bevacizumab, to block VEGF [2] . Bevacizumab binds to and disables VEGF to activate endothelial cells to create new blood vessels. This therapy is already applied clinically.

To reduce the ability of invasion is also one way to prevent metastasis. Cancer cells can spread by degrading ECMs. ECMs are degraded by the assembly of the actin cytoskeleton in invadopodia―the invasive feet of cancer cells. In fact, MMPs (matrix metalloproteinase), the family of ECM degrading enzymes [3] , are up-regulated by signals from growth factors [4] . Then, after up-regulation of MMPs, actin assembly delivers it to the invasive site of cancer cells [5] . At the invasive site MT1-MMP (membrane-type MMP), part of MMPs, are responsible to cut laminin-5 [6] . 2 chains of laminin-5 after cleavage can bind to receptor molecules and send signals to drive actin assembly and MMP up-regulation.

Mathematical medicine and biology have become one of the popular topics in the study of modern applied mathematics. Where, cancer cell invasion models have received much attention in recent years [1] [7] [8] [9] [10] [11] . Research [7] has introduced a PDE model to observe the tissue invasion and tumor growth. Then research [8] , based on the discrete-continuum hybrid simulation, promoted the study in [7] by considering the interactions, cell-cell adhesion and other essential functions of cells. There are many other studies relating to the growth of the tumor. For example, in [1] , the authors are aiming to find out numerically how positive feedback affects the formation of invadopodia and invasion of cancer cells. They considered a model for the formation of invadopodia and reaction between proteins, such as act in monomers, ECM (extracellular matrix), signals and MMP (matrix metalloproteinases), which are playing significant role in cancer cell invasion. In the numerical simulation, the authors examined the effects of the molecules by varying the rate constants, and successfully reproduced invadopodia-like small protrusions, which have a similar scale of the real phenomenon, eventually, investigated the leading source of invadopodia; however, they did not study a boundary for cell body, which leads actins diffused into extracellular area.

To the best of our knowledge, studies on the cancer cell invasion model with free boundary are fairly rare. For that reasons, in this paper, we study a modified cancer cell invasion model with free boundary problem. The method used in this paper is motivated by Stefan problem.

In order to obtain cancer cell invasion model with free moving boundary, we need to consider the biological background of the problem. For the reader’s convenience, we will introduce the process of invadopodia formation. Invadopodia are the invasive feet (as shown in Figure 1) of cancer cells which can degrade the surrounding matrix (mainly ECMs), and cause metastasis [12] . Invadopodia are enriched in act in filaments, which are cytoskeletal structures and pushing cell membrane to drive invadopodia.

ECM fragments are decreased by the reorganization of actin cytoskeleton indirectly [13] . Actins can transport MMPs to the invasive site of cancer cells, top of the invadopodia. MMPs can break and degrade the ECMs, broken ECM fragments in return bind with receptors on the cell membrane, and induce signals to reorganize actins and MMPs. Again, MMPs can break more ECMs, and become a positive feedback loop. We summarize the interactions as shown in Figure 2.

We improved the model considered in [1] by introduce a new variable which stands for the signal droved by the connection of ECM fragments and receptors. ECM fragments are created near the membrane by degradation of ECMs. Hence, the corresponding mathematical model is described as follows:

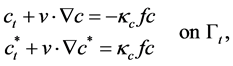(1)

where, 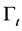stands for the free moving boundary, stands for the boundary velocity, 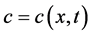, and 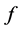are stand for the concentration of ECM, ECM fragments and MMPs, respectively. ECM fragments (laminin γ2 chains) bind to cell membrane receptors and send signals to the actin reorganization, where signals have random motility. Hence, we have

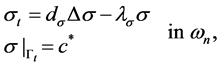(2)

where, represents signal concentration. The first equation of (2) describes the random motility and self decation of signals. The second equation of (2) describes ECM fragments bind to cell membrane and derive signals.

Free boundary 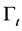is defined as

(3)

where, 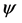is the level set function, and demands level set equation

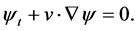(4)

Since the membrane pushed by the F-actin which is reorganized by signals from cell receptors, therefore, the velocity of the membrane depends on the gradient of signals which cause F-actin polymerization. Hence, boundary velocity defined as follows:

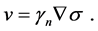(5)

Finally, we derived the modified cancer cell invasion model with cell boundary described as (1-5), our main purpose is to generalize this model into Stefan problem, then analytically discuss its solution.

The organization of this paper is as follows. In section 2 we present some basic definitions, assumptions and related properties, such as characteristic curve of the problem, Greens functions etc., to simplify the problem. In section 3, the main results, related theorem and its proof, of our paper was stated. Finally, the detailed calculation from (48) to (49) and (50) is given in Appendix A, B, C.

2. Preliminaries

The previous section, we stated the biological background and modified cancer cell invasion model with free moving boundary (1 - 5). In this section, we will introduce some basic definitions and related preliminaries, such as Gaussian Kernel, Green function and derivation of Stefan problem etc., which would be useful in proving main results and solutions of Stefan problem (27) in section 3.

2.1. Characteristic Curve

In this paper, for simplicity, we take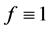, and discuss the model in 1 dimension. Hence, the level set Equation (4) is rewritten in the following form

(6)

where, stand for the right and left side boundary positions, which depends on time, stand for the velocity of right and left side boundaries (Figure 3).

Take, which satisfies

where satisfies semi-group property:

(7)

Consider the following equation of c,

One can write

Then we easily have the solution

(8)

where is arbitrary constant. We take, then we have

By (8), it follows that

(9)

By and (9), we have

When

Now we take

and finally we can get

By using an argument similar to the above, from the equation

we have the solution

Then signals on boundary satisfies

Finally we have,

(10)

with

(11)

The Equation ((10) with (11)) is our key problem for the solution of (1-5). If we can get the solution of sigma from (10) and (11), then we can easily find the solution from the following equation

(12)

2.2. Gaussian Kernel

First, consider initial condition problem

(13)

Now, we multiply to both sides of (13), and take then we have

(14)

If defined in the whole domain, then the solution of (14) would be

(15)

where,

(16)

However, (or) is defined in bounded domain therefore we cannot have the solution (15) for our case. But, at least we can see that the Gaussian function is differentiable against and, and satisfies heat equation

Thus, we can say that is can be a fundamental solution for the heat equation.

Next, consider parameters in Gaussian function. Define a new function

(17)

is differentiable for all x and t except and satisfies

(18)

If we consider is the function of with parameters, then is differentiable for all and except, and satisfies

(19)

Note that

(20)

is also be a fundamental solution for the heat equation. Next we apply this fundamental solution to express the solution for the initial value problem (14).

2.3. Green’s Function

In order to find the solution of (14), we need to introduce new function as follows

(21)

(22)

Clearly, we can see that and are differentiable for all x and t except and and satisfies

(23)

and and are differentiable for all and except, therefore satisfies

(24)

Since,

by (18), we can easily prove (23) and (24). Using the third property of in (20), we can get

(25)

(26)

where, and are called Green’s first type function and second type function, respectively.

2.4. Free Moving Boundary Problem

Now, we consider free moving boundary as in Figure 4 with the following system:

(27)

where,

Similar to (13), we have the following system

(28)

where,

Define a domain D as (Figure 4)

Take as,

(29)

Furthermore, from (24), we know

(30)

in D. Combining (29) and (30), we have

(31)

According to Green’s formula, the left side of the Equation (31) becomes

Finally, we have

(32)

since,

When, the left side of (32) equals to

(33)

3. Main Results

In this section we will state the main results, for convenience we will divide this section into two parts. In the first part, we will give three propositions and one theorem. Where, Proposition 1 and Proposition 2 are useful in proving Proposition 3, and Proposition 3 proves Theorem 1. Theorem 1 represents the solution of in free boundary problem (27). In the second part, we will derive the solution of boundary velocity and boundary position by using condition (12) and Theorem 1.

3.1. Solution for Free Boundary Problem

In order to prove Theorem 1, we need to prove following three propositions.

Proposition 1. Suppose that, is continuous for on, when, then

(34)

holds.

Proof. We consider the left side of (34),

Since,

hence,

This completes the proof of Proposition 1.

Proposition 2. Suppose that, is continuous for on, when then

(35)

holds.

Proof. We consider the left side of (35),

where,

Since is small enough, then, we have

Next, from the continuity of, for any small, there exists, for, we have

therefore,

Similarly we can get

Therefore, (35) follows.

Proposition 3. Suppose that, is continuous for on and when, then

(36)

Proof. From the assumption of Proposition 3, we have

By Proposition 1 and Proposition 2, we can prove Proposition 3. This completes the proof.

Theorem 1. Suppose that is continuous for on, then we have

(37)

Proof. Proposition 3 gives the calculation of the first term of form (33). Regarding to the second term of (33), we are using the third property of in (20),

for. Similarly to (36), we have

since not belongs to, therefore, , and the second term of (33) equivalent to 0.

The above results show that the left side of Equation (32) satisfies

(38)

Then, we have

(39)

For the right side of (39), it is clear that point is separated from, and and is continuous for on, since,

and

Moreover, note is continuous for all and on. Hence from the bounded convergence theorem we have

(40)

for. Now, take together (38) and (40), we have

(41)

Equation (41) is useful to prove Theorem 1, which can be written as,

(42)

This completes the proof of Theorem 1.

3.2. Solution for Velocity

In the above result (37) in Theorem 1, all variables are known except. From the Equation (6) and last condition of (28), we can write

(43)

(44)

where, is velocity of. To define we first differentiate the both side of (42), thereupon,

(45)

From the definition of one can easily get

(46)

then,

If we use above property, (45) becomes,

(47)

Next, from (23), we have, thus, (47) becomes

(48)

When tends to from the left,

(49)

(See appendix for specific information).

(50)

When,

(51)

Integrate both sides of (43) from 0 to t, we have

therefore, we have

(52)

Refer appendix for specific information about how (49) and (51) are followed by (48) as and, respectively.

4. Conclusions

Actin filaments are cytoskeleton in cytoplasm, which can drive cell deformation, migration [14] , and even invasion to the surrounding matrix [15] . How actin filaments are driving cancer cell invasion has been discussed in [1] . They considered four particles, actins, ECMs, MMPs and ECM fragments, where actins and MMPs act in intracellular area, ECMs and ECM fragments exist in extracellular area. Although, they had an excellent result which can describe the deformation of the cell membrane; however, they cannot control the actins, which should not be in extracellular area, diffused throughout the whole domain. To improve the work in [1] , in this paper we added a free-boundary, which is defined as (3), to separate the whole domain into two sub-domains, intracellular domain and extracellular domain. Where, the free-boundary is proportional to the cell membrane, which is considered to be pushed by actin assembly (n), and hence we took the boundary velocity depending on the assembly rate of actin proteins.

Colin et al [16] introduced a model to describe endothelial cells’ migration on bioactive micro-patterned polymers. In their model, the chemotaxis term is considered as cell-cell interaction, therefore, they considered two domains, the adhesive domain and the non-adhesive domain, where adhesive areas are surrounded by non-adhesive areas. By the motivation of the work in [16] , we divided the domain into two parts; however, because of the high complexity of the model, it is difficult to deal with the solution. Thus, we simplified the model and turn the problem into Stefan problem (27), and then consider its solution in one-dimen- sional case. As a result, integral equations and were obtained. Therefore, problem of solving system (27) was turned to the problem of solving combination of (50), (51) and (52). The results are useful because the system (27) became more suitable to apply finite difference method or other methods, for example, Picard’s successive method. On the other hand, from the biological point of view, the results, in this paper, are not enough to explain the biological meaning; however, they will lead us to further step to discuss the solution of the modified model (1 - 5).

We have more interesting topics which deserve further investigations, such as numerical simulations of the integral equations (48, 50 - 52) and how we can get the solution of the original modified model (1 - 4) based on integral solution.

Acknowledgements

This work was supported by the National Natural Science Foundation of China (Grant Nos.11401509) and the Natural Science Foundation of Xinjiang University (Starting Fund for Doctors, Grant No. BS130102).

Conflict of Interests Statements

All authors of this article declare: there is no conflict of interests regarding the publication of this article.

Cite this paper

Mahemuti, R., Muhammadhaji, A. and Suzuki, T. (2017) Research on the Solution of Cell Invasion Model with Free Boundary. Open Journal of Applied Sciences, 7, 242-261. https://doi.org/10.4236/ojapps.2017.76021

References

Appendix

This appendix provides specific information about how we get (49) from (48).

a) Confirmation of

(53)

where, is continuous in closed interval.

Proof. From the definition of, we have

Then, from the definition of,

where, is continuous in, therefore, it is clear that

is continuous on. Now, we prove there exist such that.

For further calculations, we introduce inequality

(54)

where, , and is positive constant. We can prove (54) by

(55)

then, by the definition of Function-limit, we have for any, and

then,

Therefore, applying (54) we can get

Take, from the continuity of in closed set we can say is bounded, and then there exist, such that

Hence, we have

which implies that is integrable for all

and continuous for all. Finally applying continuity of integrals theorem, it follows that

On the other hand,

Similarly, we can get

b) confirmation of

(56)

Proof. Set

if is bounded in, then,

(57)

From the definition of, we have

Take is sufficiently close to, and the absolute value of integration on the curve is

Since, is sufficiently close to, and the absolute value of is sufficiently small, hence, is uniformly converges on any point close to.

Now, prove the continuity of near. Especially, we want to prove that is continuous on. We prove this using the Definition of Continuity: if

(58)

holds for all. If we can prove there exists and (58) holds, then we can prove the continuity of. Now, we divide the integrals,

where is sufficiently close to. (is sufficiently small). Therefore, from the convergence of, we can write, for very small, it holds that

for all. Furthermore, when is sufficiently small, then is sufficiently small. Next from the continuity of,

Therefore (58) holds, which implies

c) Conformation of

where, is bounded and lipschitz continuous.

Proof. From the definition of, we have

where,. For simplicity, set, and

Suppose,

hence,

when, we have

(59)

When and, we have

(60)

next, from (59) and (60), we can get

since,

From the proof of Appendix B, one can know is continuous near, therefore,

Finally, we get

(61)

where,. From Appendix B, we have

Next, we can get

since, is away from and the integral

is continuous on.

Submit or recommend next manuscript to SCIRP and we will provide best service for you:

Accepting pre-submission inquiries through Email, Facebook, LinkedIn, Twitter, etc.

A wide selection of journals (inclusive of 9 subjects, more than 200 journals)

Providing 24-hour high-quality service

User-friendly online submission system

Fair and swift peer-review system

Efficient typesetting and proofreading procedure

Display of the result of downloads and visits, as well as the number of cited articles

Maximum dissemination of your research work

Submit your manuscript at: http://papersubmission.scirp.org/

Or contact ojapps@scirp.org