ABSTRACT

In this paper, we show that if an injective map on symmetric matrices 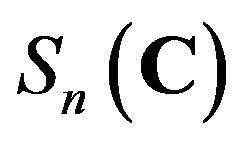satisfies 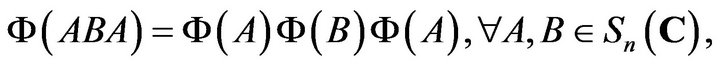then 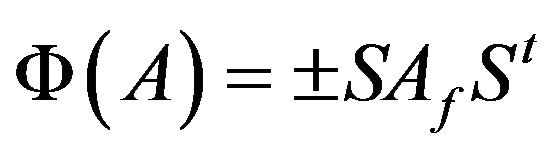for all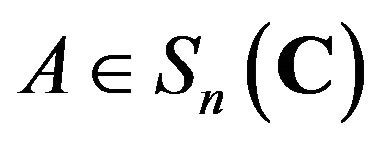, where 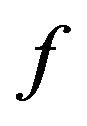is an injective homomorphism on, is a complex orthogonal matrix and is the image of under applied entrywise.

1. Introduction

It is an interesting problem to study the interrelation between the multiplicative and the additive structure of a ring or an algebra. Matindale in [1] proved that every multiplicative bijective map from a prime ring containing a nontrivial idempotent onto an arbitrary ring is additive. Thus, the multiplicative structure determines the ring structure for some rings. This result was utilized by P. Šemrl in [2] to describe the form of the semigroup isomorphisms of standard operator algebras on Banach spaces. Some other results on the additivity of multiplicative maps between operator algebras can be found in [3,4]. Besides ring homomorphisms between rings, sometimes one has to consider Jordan ring homomorphisms. Note that, Jordan operator algebras have important applications in the mathematical foundations of quantum mechanics. So, it is also interesting to ask when the Jordan multiplicative structure determines the Jordan ring structure of Jordan rings or algebras.

Let 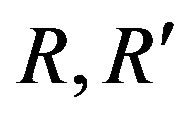be two rings and let 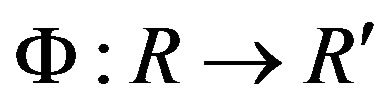be a map. Recall that is called a Jordan homomorphism if

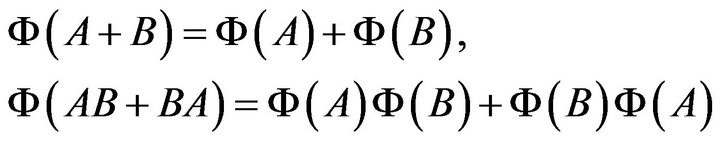
for all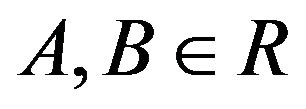. There are two basic forms of Jordan multiplicative maps, namely1) 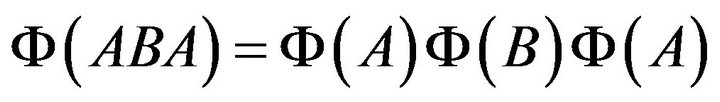(Jordan semi-triple multiplicative map) for all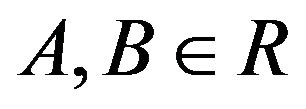2)

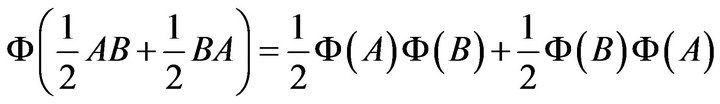
(Jordan multiplicative map) for all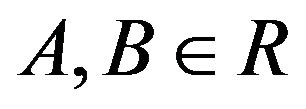. It is clear that, if is unital and additive, then these two forms of Jordan multiplicative maps are equivalent. But in general, for a unital map, we do not know whether they are still equivalent without the additivity assumption.

The question of when a Jordan multiplicative map is additive was investigated by several authors. Let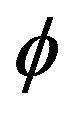be a bijective map on a standard operator algebra. Molnár showed in [5] that if 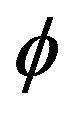satisfies

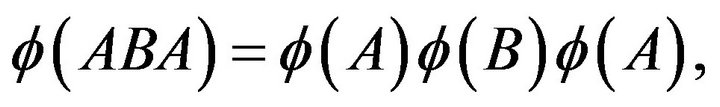
then 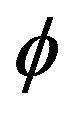is additive. Later, Molnár in [5] and then Lu in [6] considered the cases that 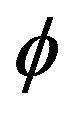preserve the operation

and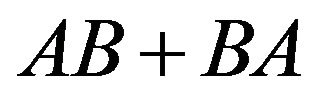, respectively, and proved that such is also additive. Thus, the Jordan multiplicative structure also determines the Jordan ring structure of the standard operator algebras. Later, in [7] we proved these Jordan multiplicative maps on the space of selfadjoint operators space are Jordan ring isomorphism and thus are equivalent. In this paper, we consider the same question and give affirmative answer for the case of Jordan multiplicative maps on the Jordan algebras of all symmetric matrices. In fact, we study injective Jordan semi-triple multiplicative maps on the symmetric matrices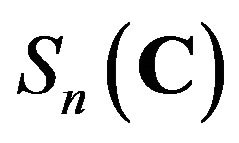, and show that such maps must be additive, and hence are Jordan ring homomorphisms.

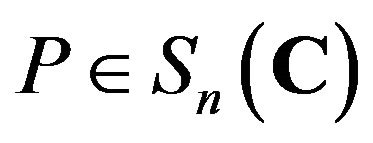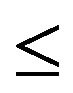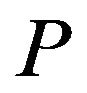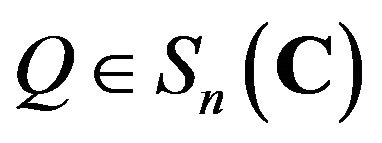Let us recall and fix some notations in this paper. Recall that is called an idempotent if. We define the orderbetween idempotents as follows: if and only if for any idempotents,. For any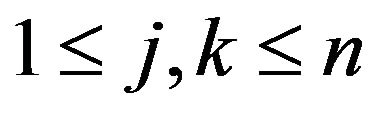, let 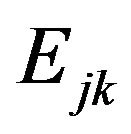be the matrix with 1 in the position 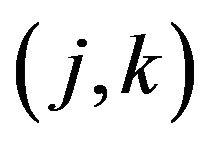and zeros elsewhere, and be the unit of.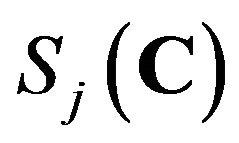

2. Main Results and Its Proof

In this section, we study injective Jordan semi-triple multiplicative maps on, the following is the main result.

Theorem 2.1. An injective map

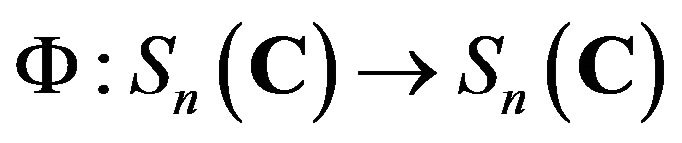
is a Jordan semi-triple multiplicative map, that is

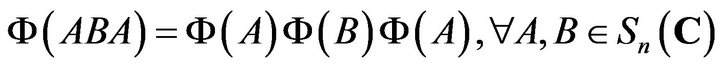(2.1)

if and only if there is an injective homomorphism 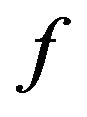of and a complex orthogonal matrix 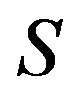such that

for all.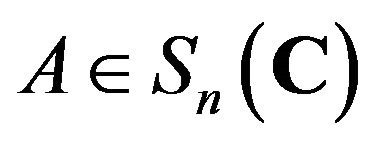

Firstly, we give some properties of injective Jordan semi-triple multiplicative maps on.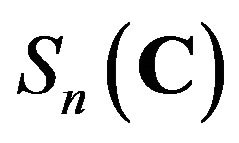

Lemma 2.2. Let be an injective Jordan semi-triple multiplicative map. Then sends idempotents to tripotents and moreover1) 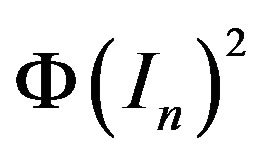is an idempotent and

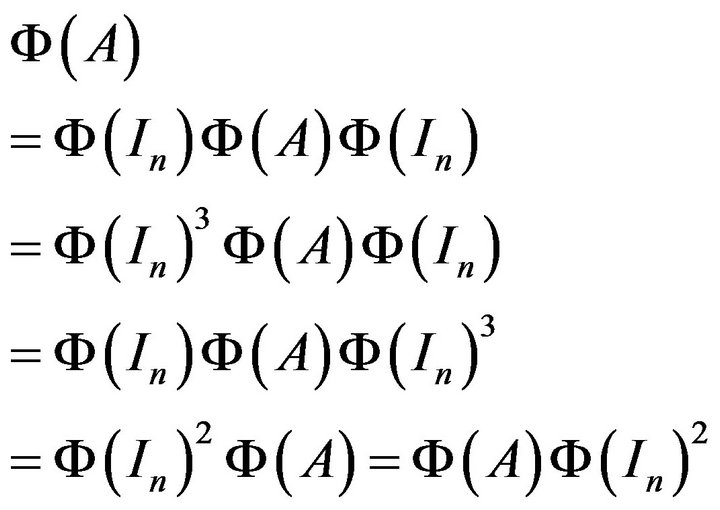
for all, in particular

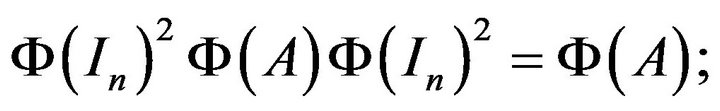
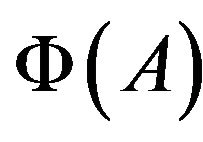2) commutes with for every;

3) 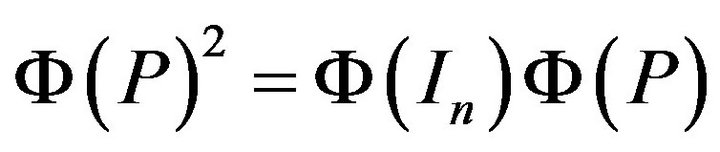is an idempotent for each idempotent;

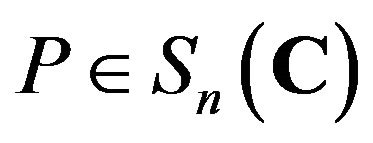4) A map 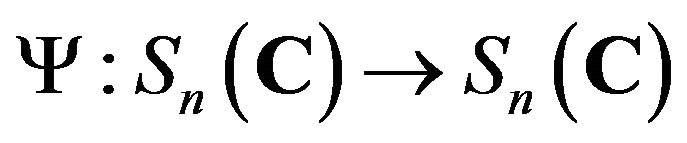defined by

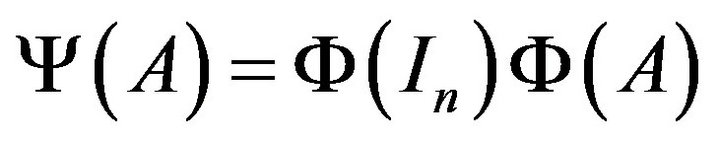
for all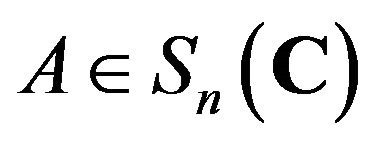, is a Jordan semi-triple multiplicative map, which is injective if and only if is injective.

For defined in Lemma 2.2, we can see that

and 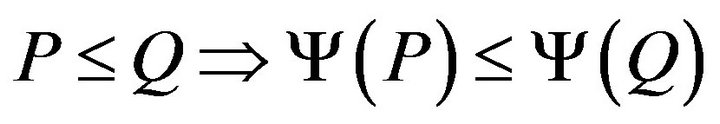for any idempotents

. Therefore, we have Corollary 2.3. Let and

be an injective Jordan semi-triple multiplicative map. Then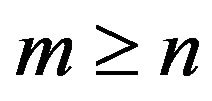. In the case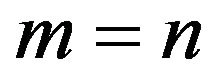, for each idempotent 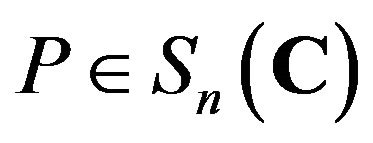the rank of 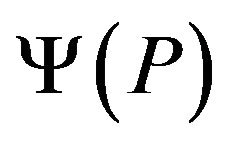is equal to the rank of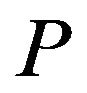. In particular,

and

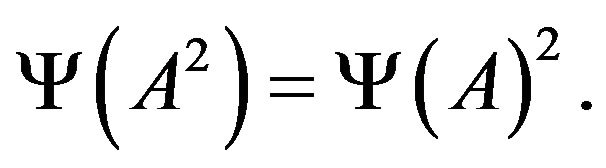
Now we give proof of Theorem 2.1. The main idea is to use the induction on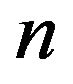, the dimension of the matrix algebra, after proving the result for 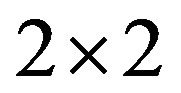matrices.

Proof of Theorem 2.1. In order to prove Theorem 2.1, it suffices to characterize. Note if

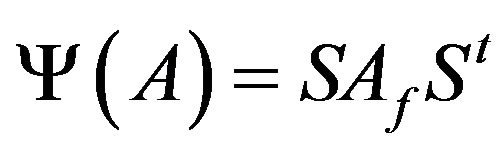
then

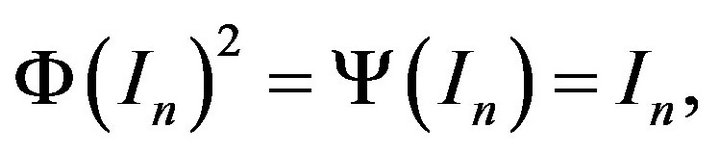
that is 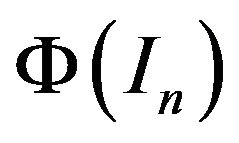is invertible and 

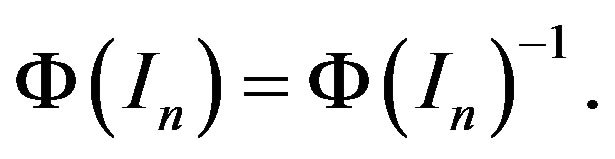
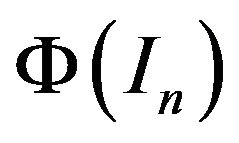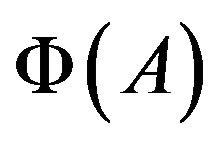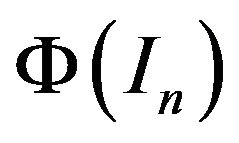By Lemma 2.1, commutes with for all. It follows that commutes with 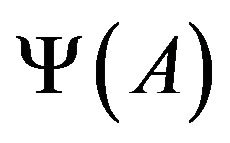for all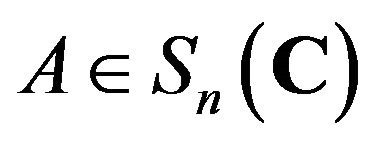. Therefore, if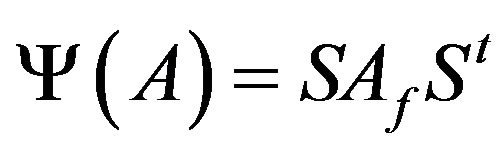, 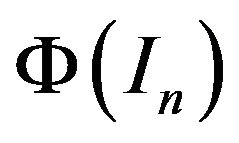must be a scalar matrix. As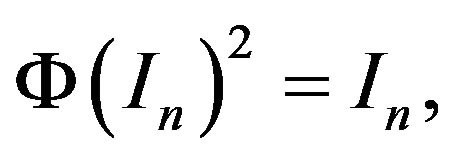 and hencehas the desired form.

Therefore, we mainly characterize. The proofs are given in two steps.

Step 1. The proof for.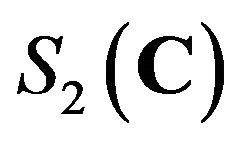

The matrix is an idempotent of rank one. By Corollary 2.3, is a rank one idempotent. It is well known that every idempotent matrix in can be diagonalizable by complex orthogonal matrix. Thus, there exists a orthogonal matrix 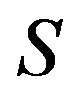such that

Without loss of generality, we may assume that 

By Corollary 2.3 and from the following fact

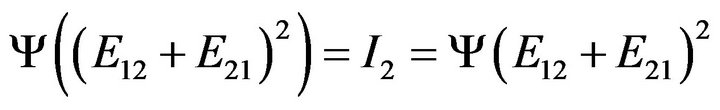
and

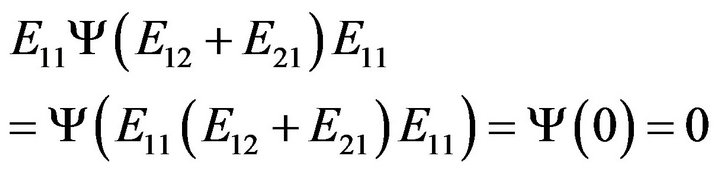
we conclude that

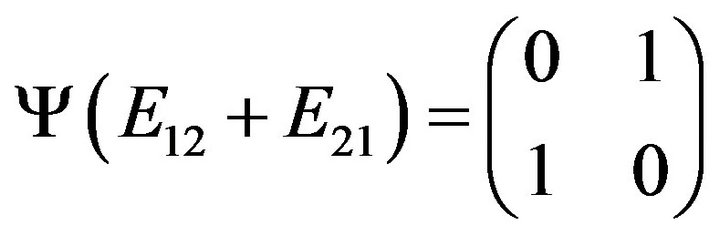
or

Let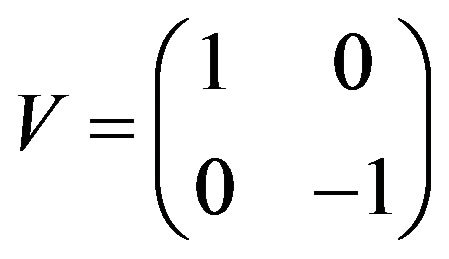, by replacing with 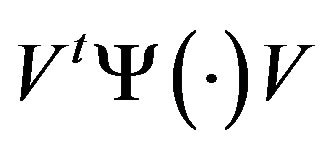if necessary, we may assume that

.

For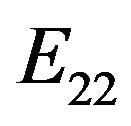, since 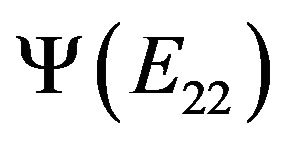is a rank one idempotent and satisfying 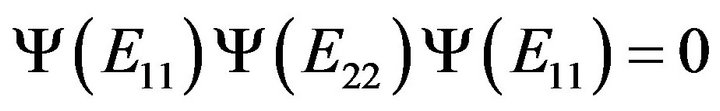and

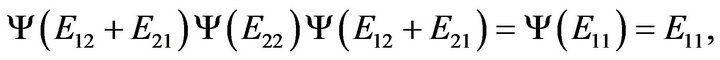
we have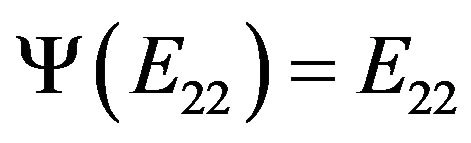. Now for any

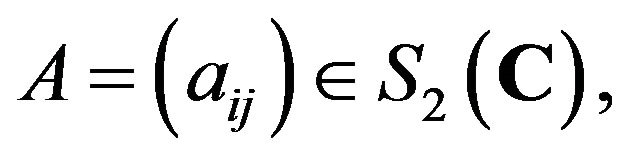
let. Then

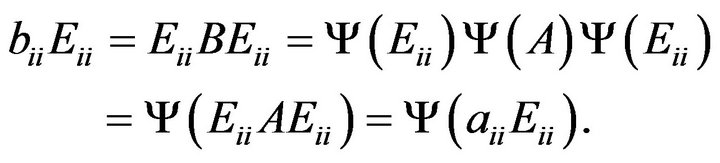
Thus, the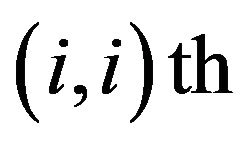 entry of depends on the entry ofonly. Therefore, there exist injective functionals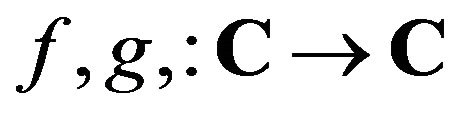 such that satisfy respectively 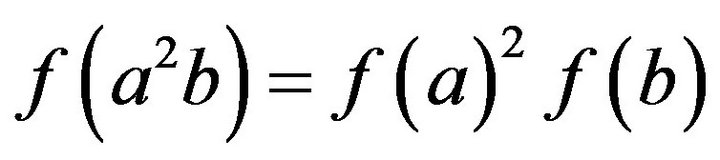and

and

.

From, it is easy to verify that is multiplicative. Next we prove tha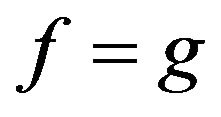t. Let

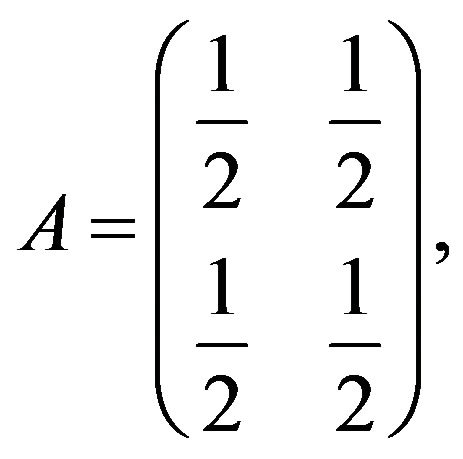since

A and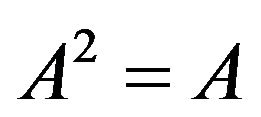, we have

and, hence or

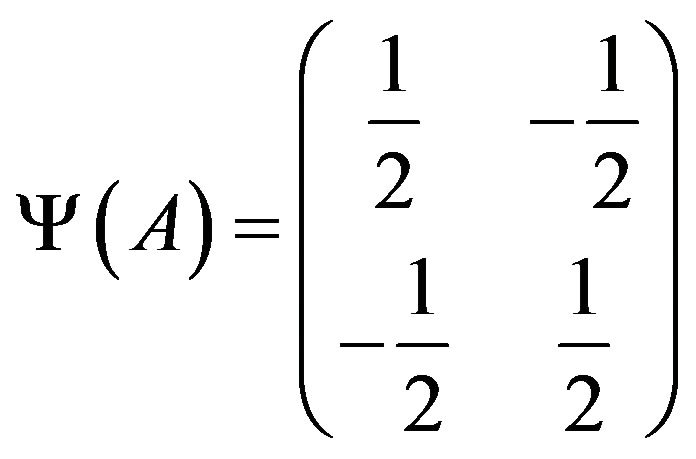with.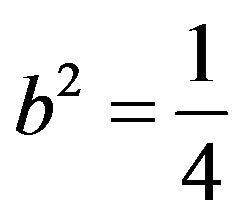

Thus, 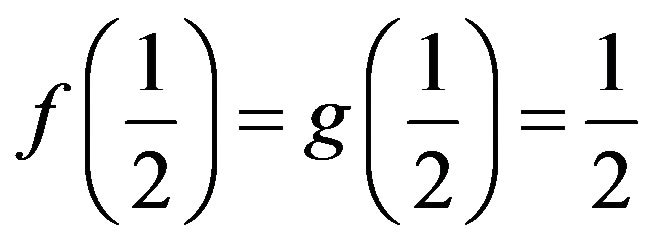and 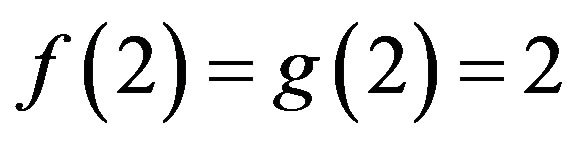since

is multiplicative. Let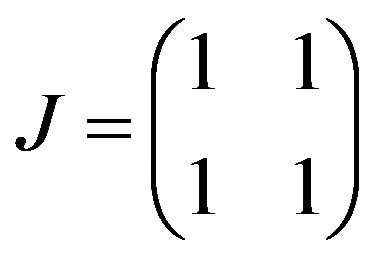, then

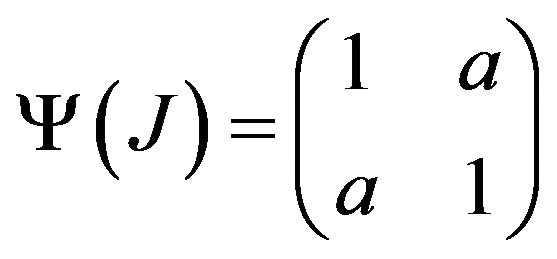. Note that 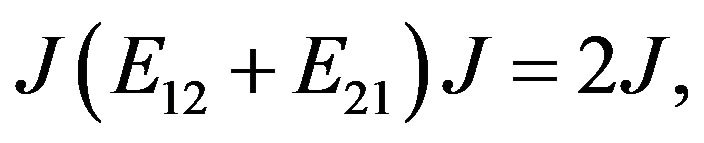and

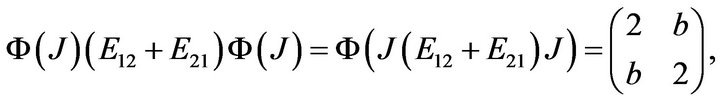
that is

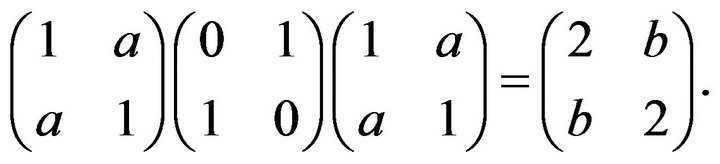
This implies and. Now by the fact and, we get. For any, since

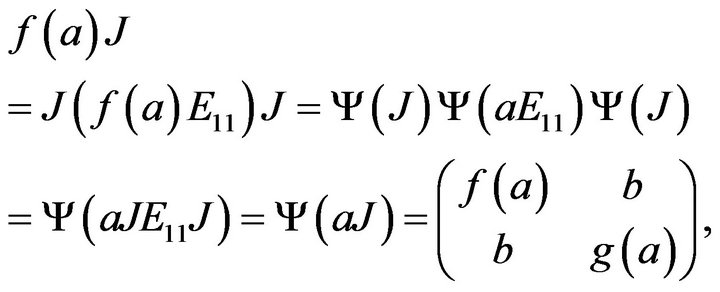
thus.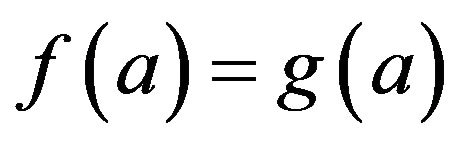

Next we prove that is additive. Since

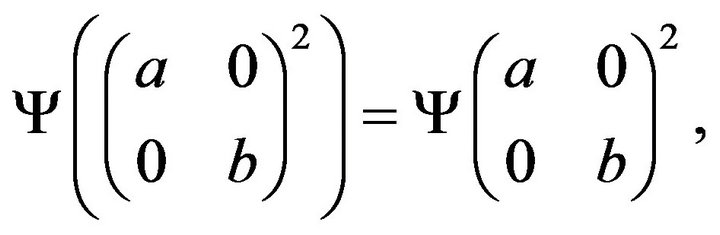and thus we have

for any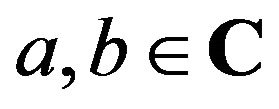. Moreover by the fact 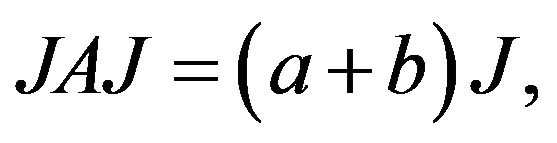one can get that

and.

Finally, we prove

for any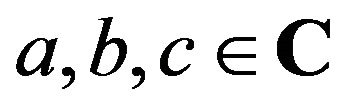. Let

.

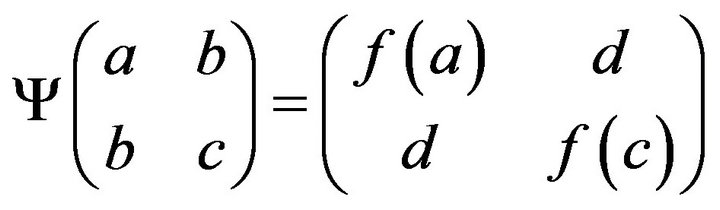By the fact that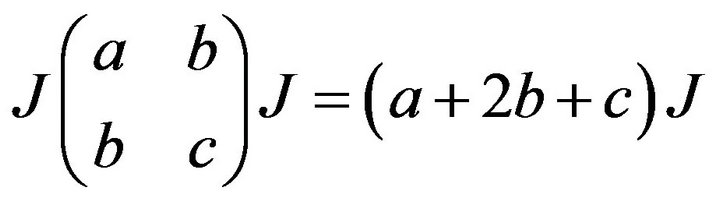

and

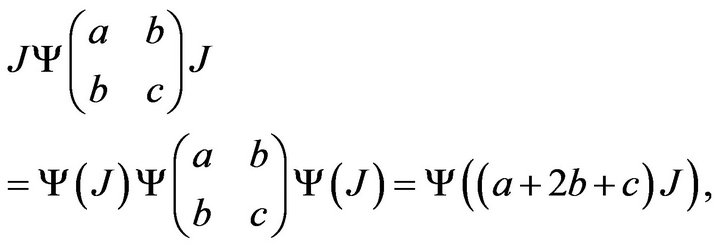
we get 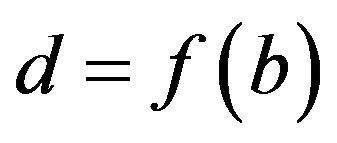and 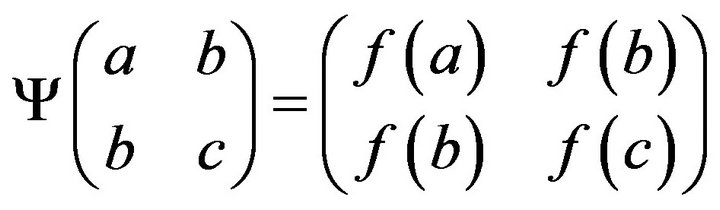for any.

Step 2. The induction.

Let

then is a rank 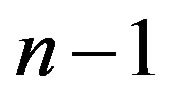idempotent, so is 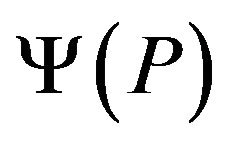by Corollary 2.3. Therefore, there exists a orthogonal matrix such that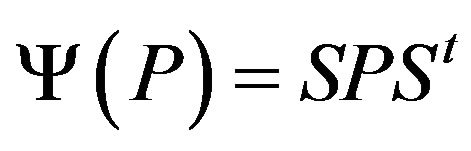. Replacing by the map 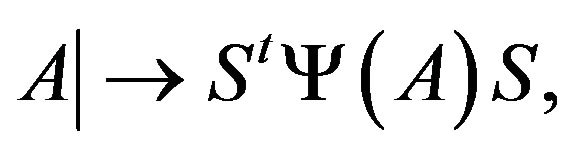we may assume that.

For any 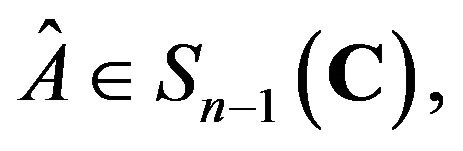let. Then implies

It follows that 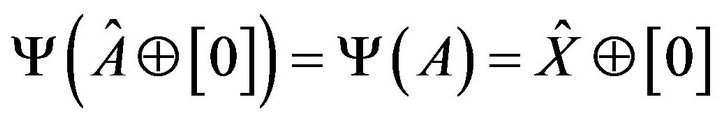for some matrix. Define the map on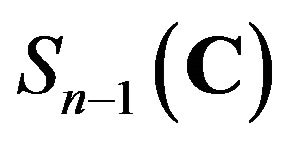

by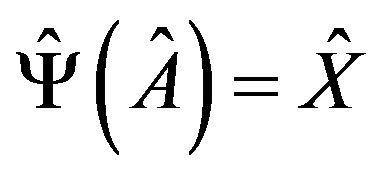. It is easy to check that is an injective Jordan semi-triple multiplicative map on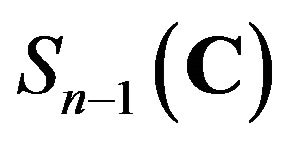. Furthermore, 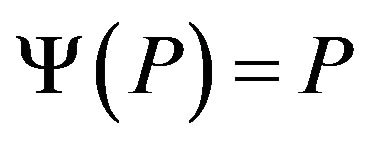implies that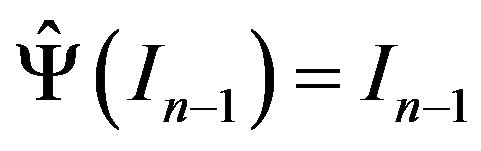. By the induction hypothesis there is a orthogonal matrix 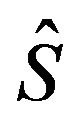and an injective homomorphism 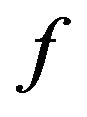on such that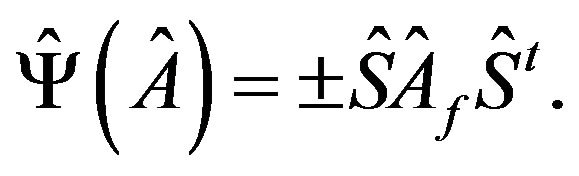

Let 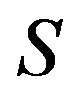be the matrix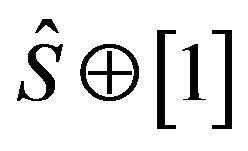. Without loss of generality, we assume that 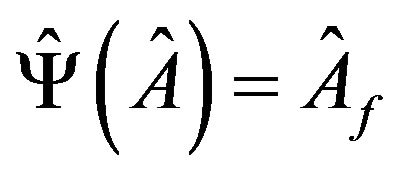for all. This is equivalent to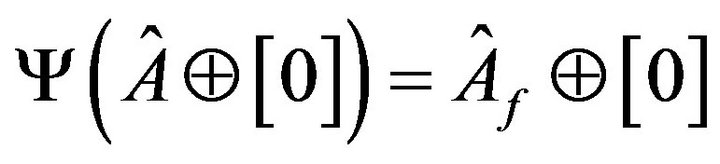. For any

with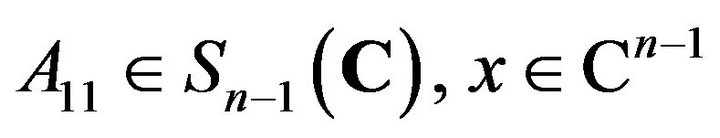

and, we have.

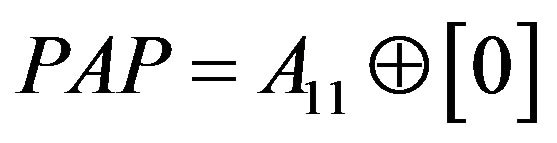Thus,

(*)

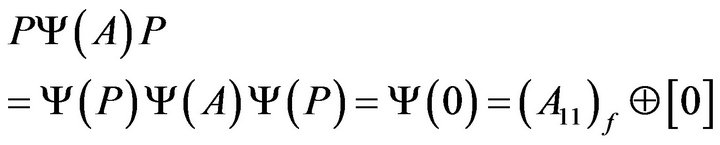Let us define matrices 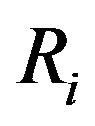for each 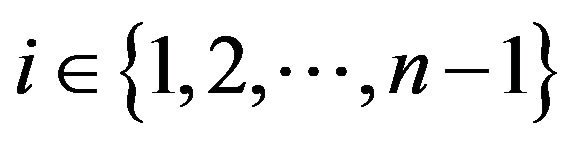by

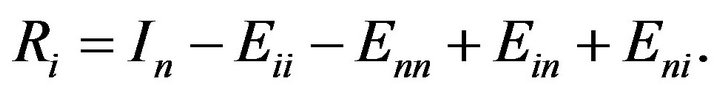
For an arbitrary, From (*) we have

Then there exists and 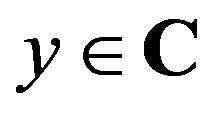such that

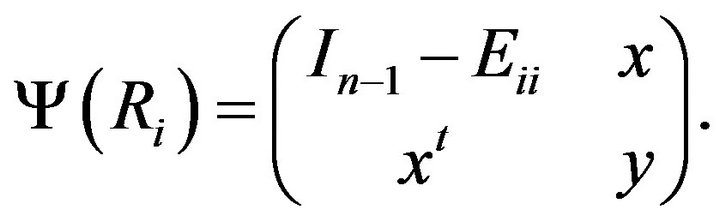
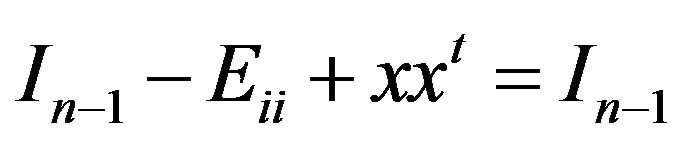From the equality we get that and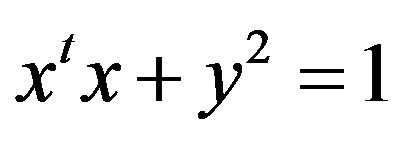. These equality implies that 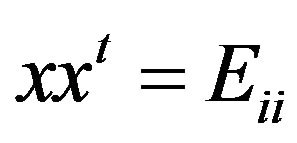and

Hence only the 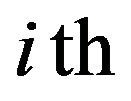entries of 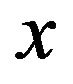are nonzero and. It follows that

Next, take any two distinct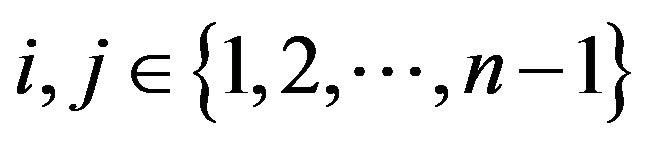. From

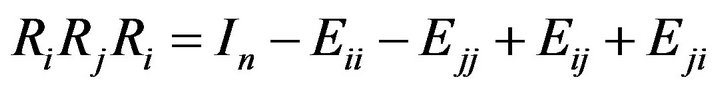
and using (*) , we get

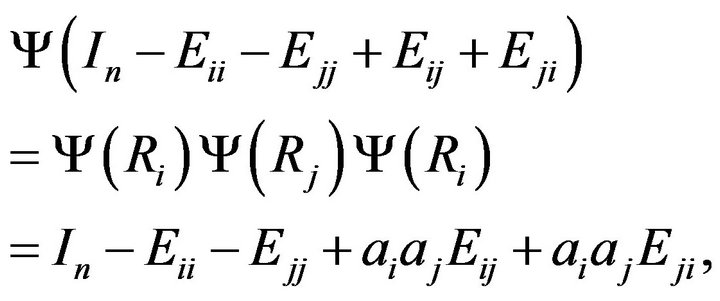
which implies that. Let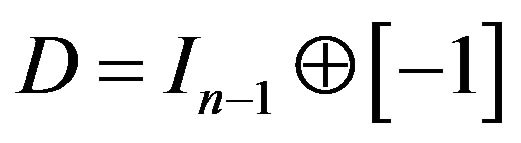, then, so we may assume that. Furthermore by the equality

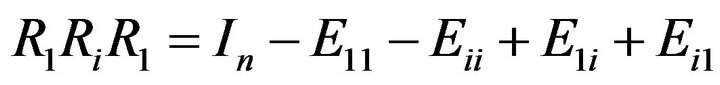
and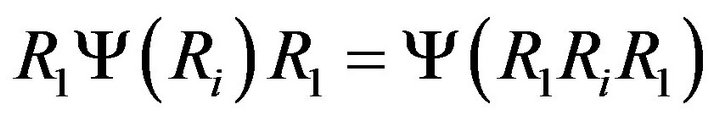, we obtain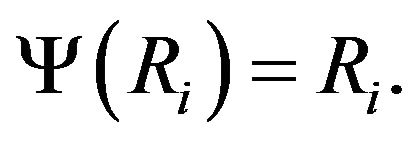

Next we prove that 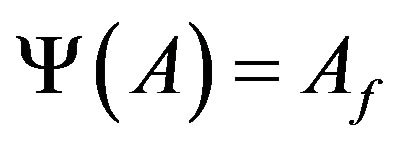for any.

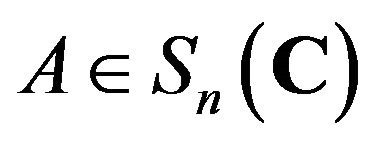Let us fix some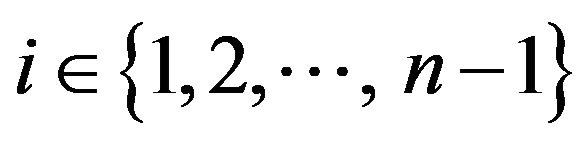. As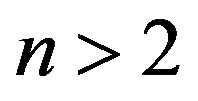, there is another such that

Then for any,

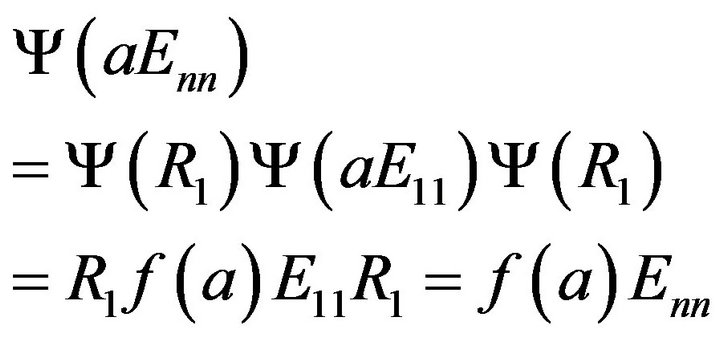
and

.

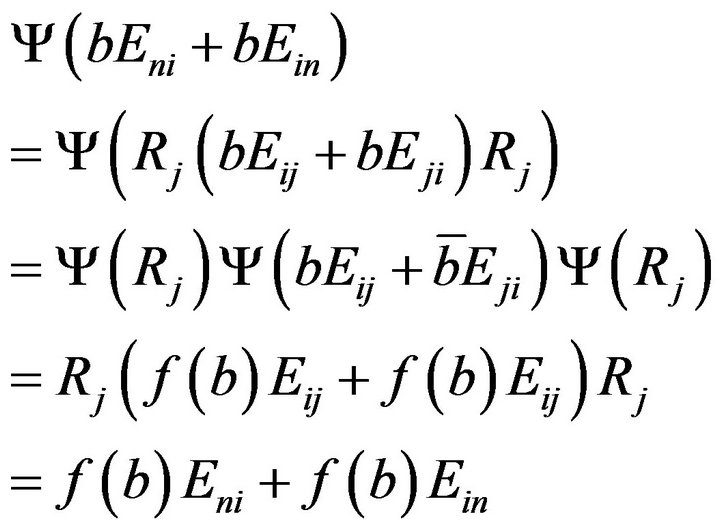Thus, for any

where 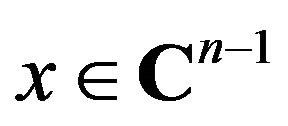has only one nonzero entry in the position, we have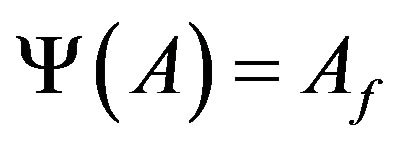. For any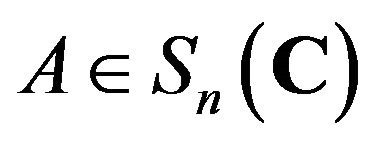, let

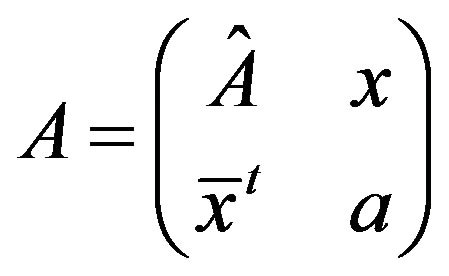
and

.

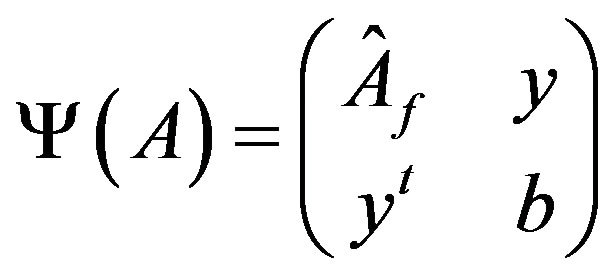From, we have

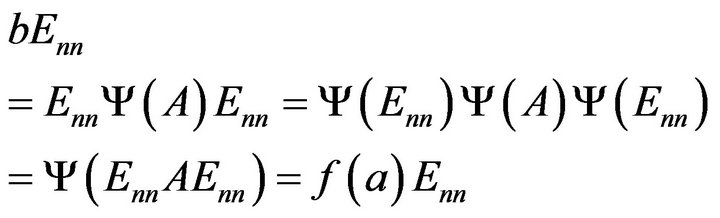
And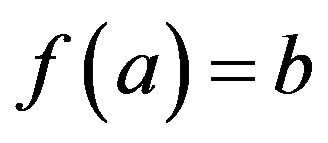. For any, since

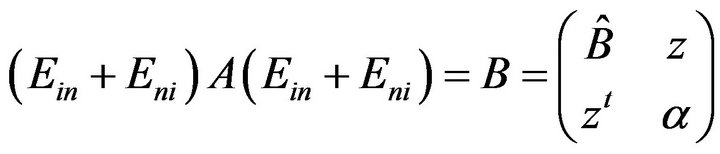
where 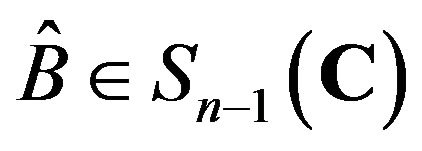and have only one nonzero entry and 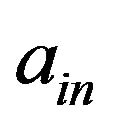in the and position respectively, is equal to the entry of, thus we have

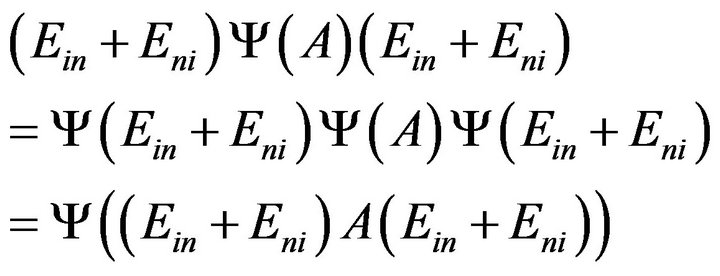
and so. The proofs are complete.

By Theorem 2.1, we can characterize another two forms of Jordan multiplicative maps on.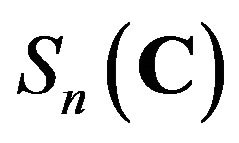

Theorem 2.4. An injective map

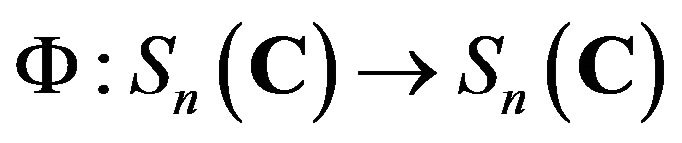
satisfies

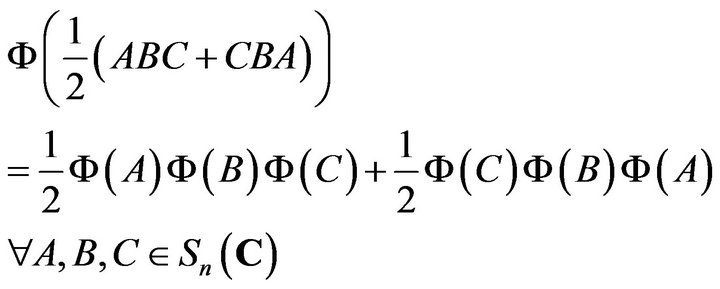(2.2)

if and only if there is an injective homomorphism on and a complex orthogonal matrix 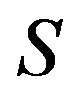such that

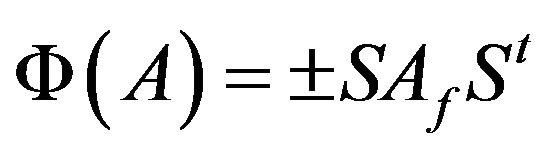for all.

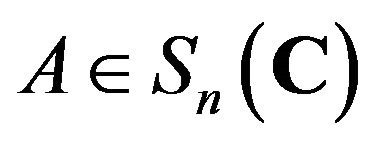Proof. Let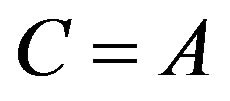 in Equation (2.2), we get

that is, is a Jordan semi-triple multiplicative map. Consequently, has the desired form by Theorem 2.1.

Since every ring homomorphism onis an identity map, thus by Theorem 2.1, Theorem 2.4, we get Corollary 2.5. Let be an injective map. Then the following condition are equivalent1)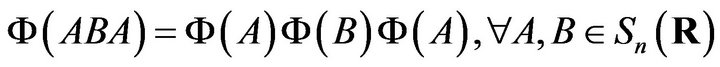

2)

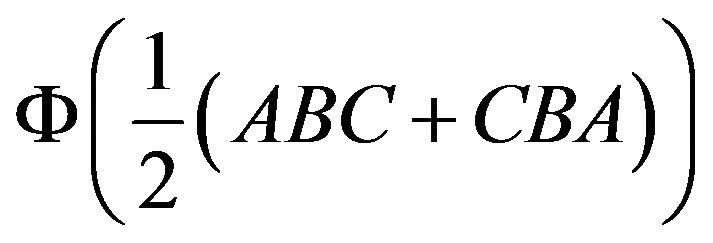
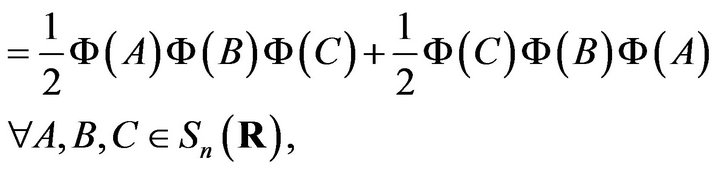
3) there is a real orthogonal matrixsuch that

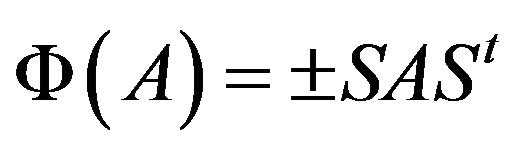for all.

At the end of this section, we characterize bijective maps on 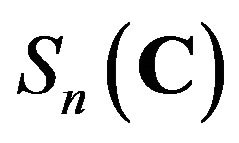preserving.

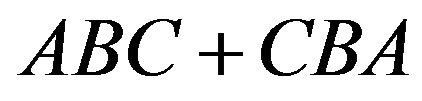Theorem 2.6. A bijective map 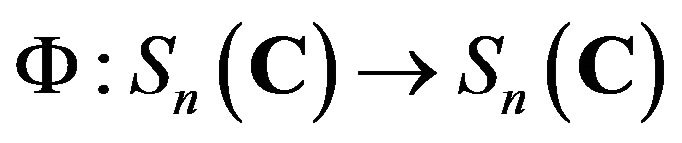satisfies

(2.3)

if and only if there is a ring isomorphism 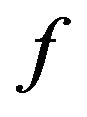on and a complex orthogonal matrix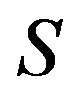such that

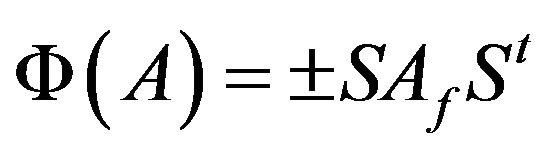for all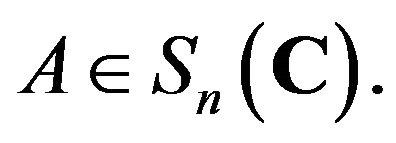

Proof. It is enough to check the “only if” part. Letting in Equation (2.3), we get

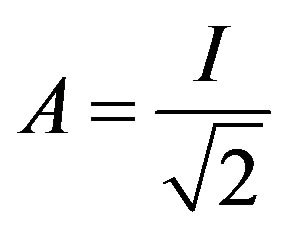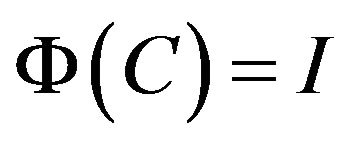Taking and, we get 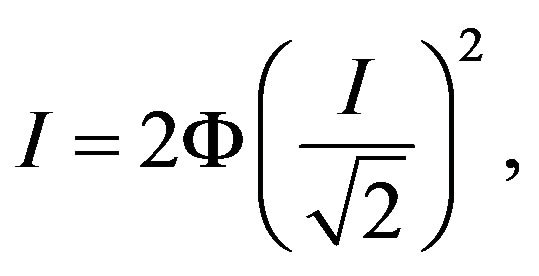and thus

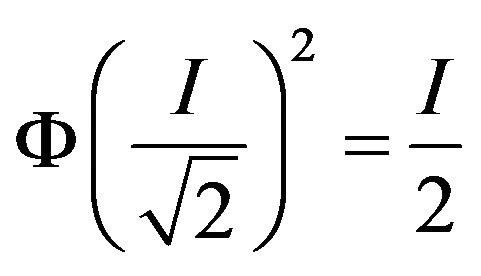(2.4)

Letting 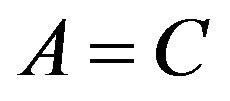in Equation (2.3), we get

.

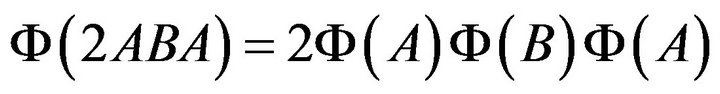Taking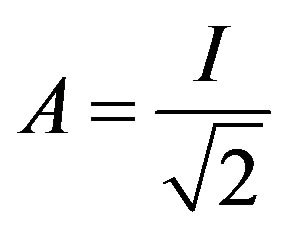, we get

.

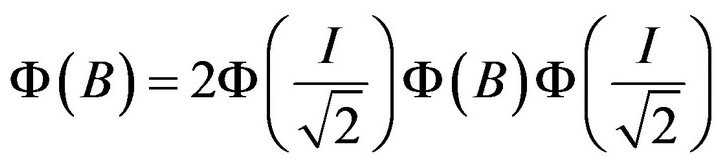Multiplying this equality by 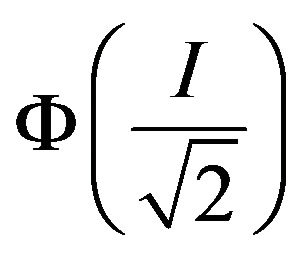from the left side, by Equation (2.4) we get

for any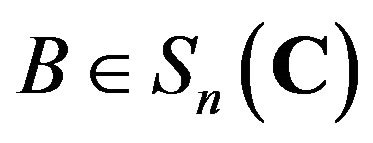, and hence 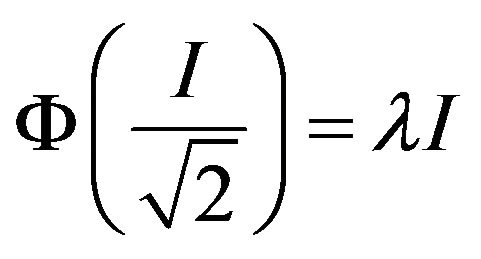for some scalar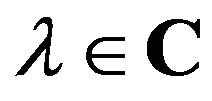. By Equation (2.4), we obtain

If, let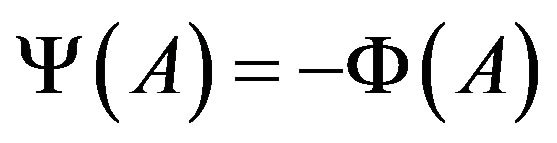, then also meets Equation (2.3) and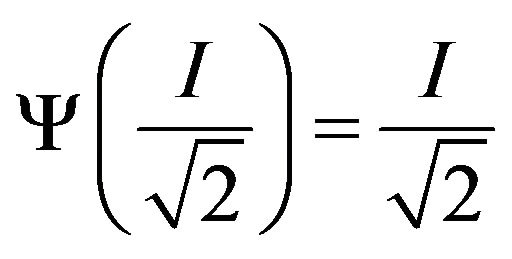. So without loss of generality, we assume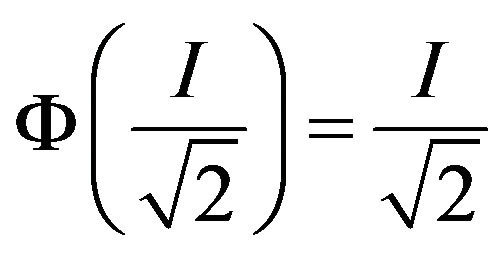. By letting 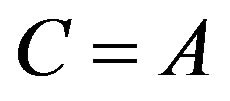and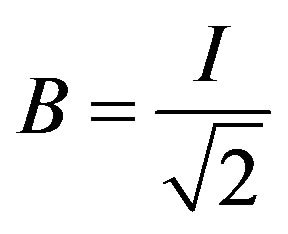 in Equation (2.3), we get

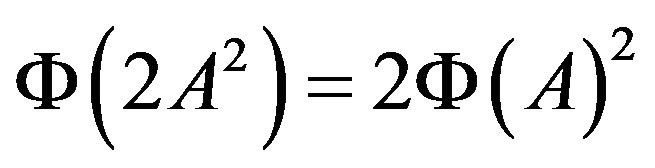and for all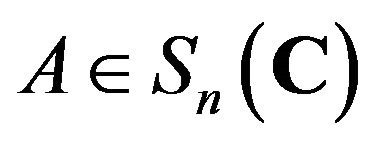. Consequently

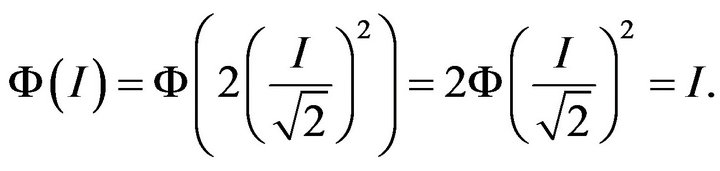
Now letting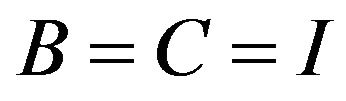in Equation (2.3) we get

.

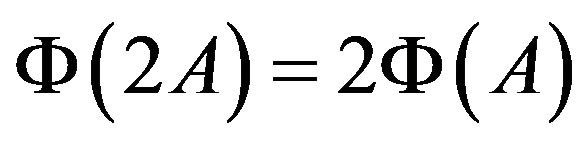Thus,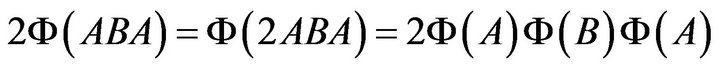

and by taking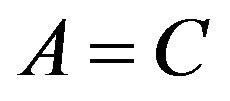in Equation (2.3). Therefore, has desired form by surjectivity of and Theorem 2.1.

In particular, we have Corollary 2.7. A bijective map 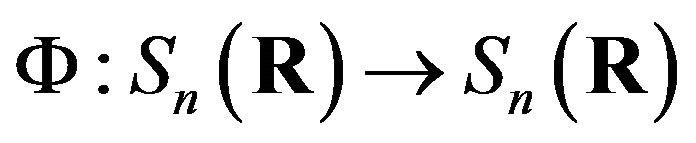satisfies

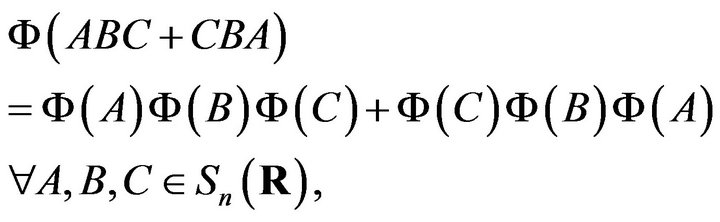
if and only if there is a real orthogonal matrix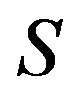such that

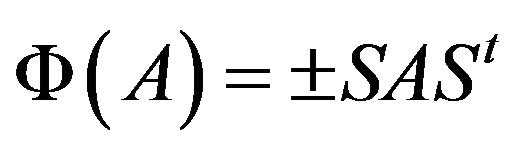for all.

Remark 2.8. We do not know whether the surjective assumption in Theorem 2.6 and Corollary 2.7 can be omitted.

REFERENCES

NOTES

^*The author is support by a grant from National Natural Foundation of China (11001194).