Open Journal of Metal
Vol.3 No.2(2013), Article ID:32778,4 pages DOI:10.4236/ojmetal.2013.32003
Technological Basics for Production of Low-Temperature Superconductors
Department Dynamics and Strength of Machines, Perm National Research Polytechnic University, Perm, Russia
Email: dpm@pstu.ru, nataly.kosheleva@gmail.com
Copyright © 2013 German Leonidovich Kolmogorov et al. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received February 3, 2013; revised March 10, 2013; accepted March 21, 2013
Keywords: Superconducting materials; Optimization; Thermoelastic state; Plastic deformation; Drawing
ABSTRACT
The technological principles of low-temperature superconductor production for the magnetic system of the International Thermonuclear Experimental Reactor (ITER) that provides continuity of a superconductor billet in the superconductor production are considered.
1. Introduction
Now, in Russia, industrial production of low-temperature superconducting materials (LTSM) for the magnetic system of the international thermonuclear experimental reactor (ITER), the construction of which started in the Atomic Center of Cadarache in France [1] is organized. This production is directly relevant to the field of modern nanotechnologies. The total number of superconducting materials for the ITER magnetic system is over 700 tons [2].
Technical superconducting cables are complex composite structures made of dissimilar materials with ultrathin fibers (fractions of microns) of the superconducting material. Figure 1 shows the structure of one of the superconductors. For the ITER, it is planned to produce low-temperature superconductors based on Nb-Ti and Nb3Sn.
Composite superconductors are transversely-isotropic medium consisting of a core which comprises superconducting fibers, and a current-stabilizing shell of ultrapure copper. The basic mechanical and thermal characteristics of the transversely-isotropic medium are defined by equations [3] of composite mechanics.
2. Technological Basics
The manufacturing technology of superconductors is the plastic deformation of metals using metal forming methods, in particular, extrusion of a combined billet and its
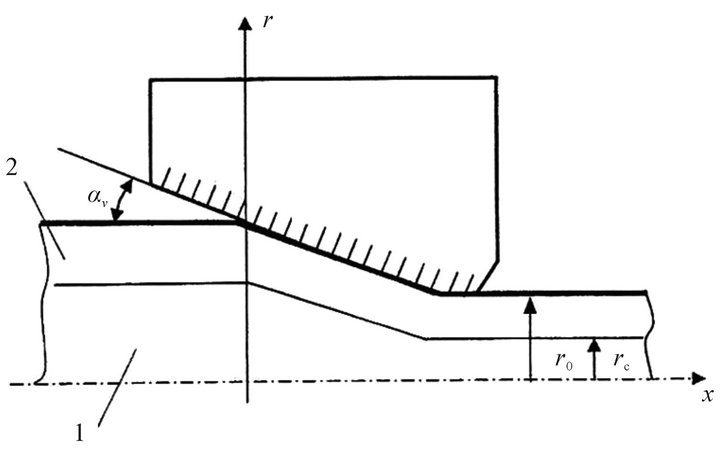
Figure 1. Schematic drawing of a superconductor bimetallic billet: 1—core; 2—shell; αv—inclination angle of a forming of the working cone to the drawing axis; r0 and rc—values of the radial coordinate for the shell and the core of the billet respectively.
repeated drawing. The highest labour input is accounted for drawing. The drawing process means drawing a billet through a conical drawing tool, and the total number of stages in superconductor production reaches several dozens.
In drawing the plastic deformation is characterized by the draw ratio that for random i-th pass is as follows:
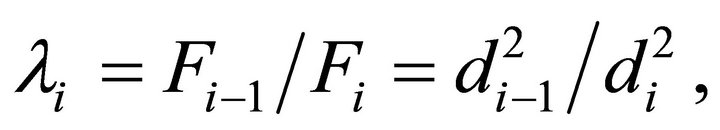 (1)
(1)
where 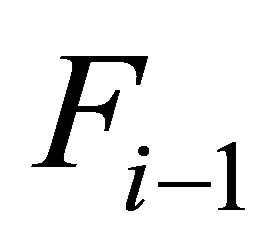 and
and  are cross-sectional area before and after the pass;
are cross-sectional area before and after the pass;  is the diameter of the billet before entering the drawing tool;
is the diameter of the billet before entering the drawing tool; 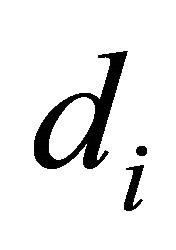 is the diameter of the billet at the output of the tool.
is the diameter of the billet at the output of the tool.
In repeated drawing the aggregate draw is determined by the area ratio of the original superconductor billet 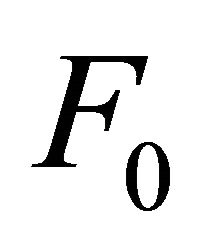 and the finished superconductor of cross Section
and the finished superconductor of cross Section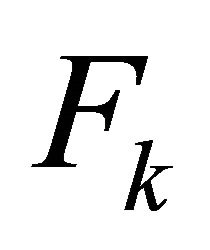 ; the aggregate draw being determined through draw ratios for individual passes by the relation:
; the aggregate draw being determined through draw ratios for individual passes by the relation:
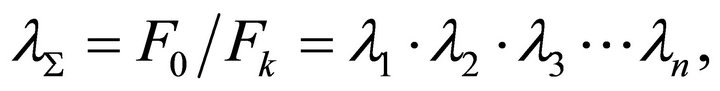 (2)
(2)
where n is the total number of passes in drawing.
To assess the manufacturing complexity of superconductor products, in the case of realization of equal draw ratios on the route of multiple deformation of the superconductor billet, the averaged around technological cycle draw ratio λav is introduced. Then from equation (2) for the averaged draw ratio the aggregate draw will be:
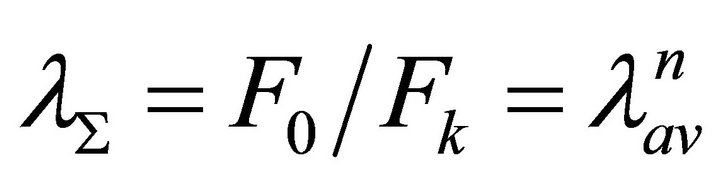 (3)
(3)
From equation (3) the number of repeated drawing passes required to produce a superconducting product is determined
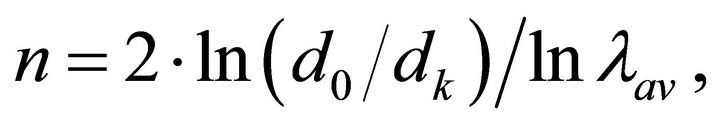 (4)
(4)
where 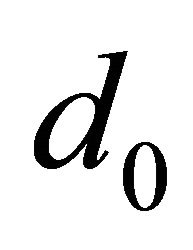 and
and  are diameters of the superconductor billet and the finished superconductor respectively.
are diameters of the superconductor billet and the finished superconductor respectively.
From (4) it follows that with the increase of single drawing the number of passes is reduced which is more technologically advanced and cost effective. However, single drawing increases danger of breakage of the billet front end to which the drawing force is applied.
Following are the calculated values of the number of passes depending on the values of single drawing by the billet deformation after pressing from the diameter d0 = 70 mm to the final diameter of the superconducting product 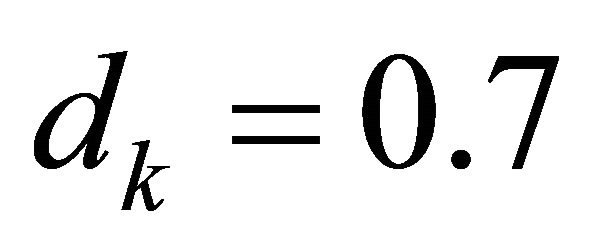 mm.
mm.
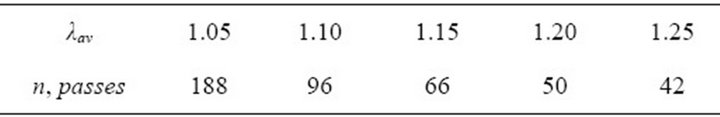
Taking into account the complex structure of the superconductor billet, the draw recommended while manufacturing is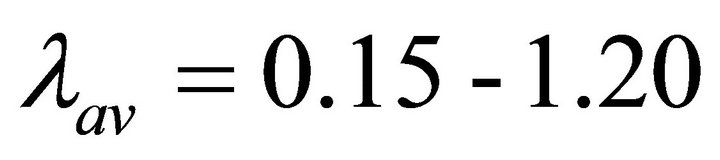 .
.
From Equation (1) for the given 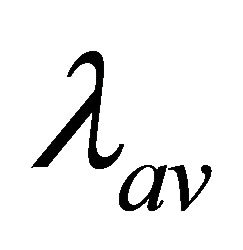 pass routes are defined:
pass routes are defined:
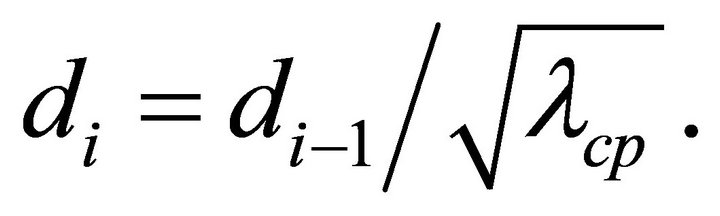 (5)
(5)
Optimization of drawing routs allows you to achieve the following objectives:
• reduce the number of the route passes;
• avoid breakage of long-length billets;
• improve quality of the surface of superconductor products;
• increase durability of the drawing tool.
In the processing of metals by pressure it is the deformation degree that largely defines energy-power and technological parameters of plastic deformation.
In [4] there is a formula proposed for determining the average over cross section deformation degree in drawing axisymmetric superconductor products:
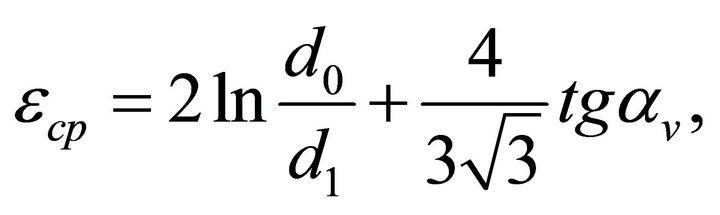 (6)
(6)
where αv is the inclination angle of a forming of the tool to the drawing axis; 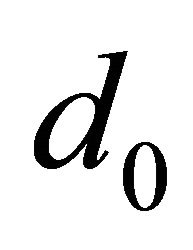 is the outer diameter before the pass;
is the outer diameter before the pass; 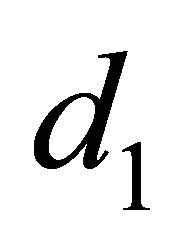 is the outer diameter after the pass.
is the outer diameter after the pass.
Realizing the technology of drawing superconductor composite billets requires knowledge of deformation temperature conditions. In repeated drawing, the billet temperature changes due to the deformation heat up in each pass and it is determined by the terms of cooling between passes. Knowledge of the temperature mode is necessary to assess the thermoelastic state of a multi-component billet and prevent possible shell detachment from the core.
To determine the heat of the metal wire under deformation it is necessary to define the work spent on the deformation, as
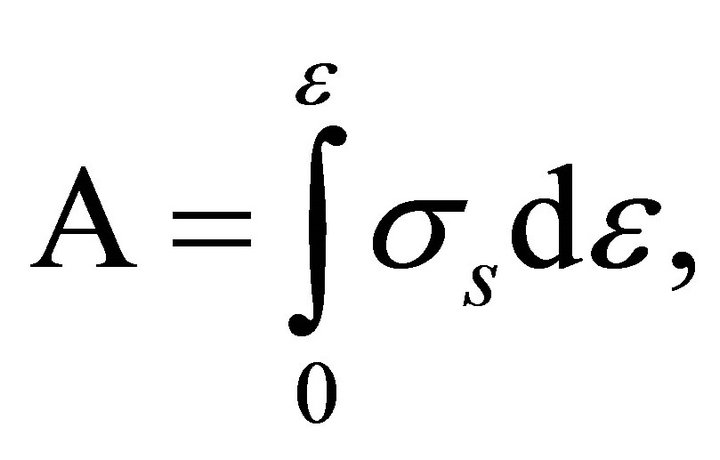 (7)
(7)
where 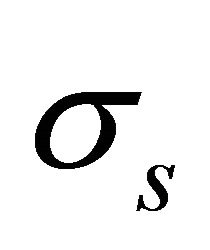 is resistance to deformation generally based on the deformation degree.
is resistance to deformation generally based on the deformation degree.
If you accept that all plastic deformation work goes into heat, then the temperature raise for a volume unit of the material element during the adiabatic process of deformation is defined by the equation:
 (8)
(8)
where c is the specific heat of the drawn metal; 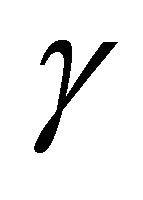 is the metal density.
is the metal density.
In deformation heat up the shell may be detached from the core due to the difference of thermophysical properties of their materials. To evaluate possible detachment and prevent it in the manufacturing process the thermoelastic state of the bimetallic superconductor billet is considered (see Figure 1).
The thermoelastic state of an axisymmetric body is described by equations of the elasticity theory [5], and the strain tensor components are as follows:
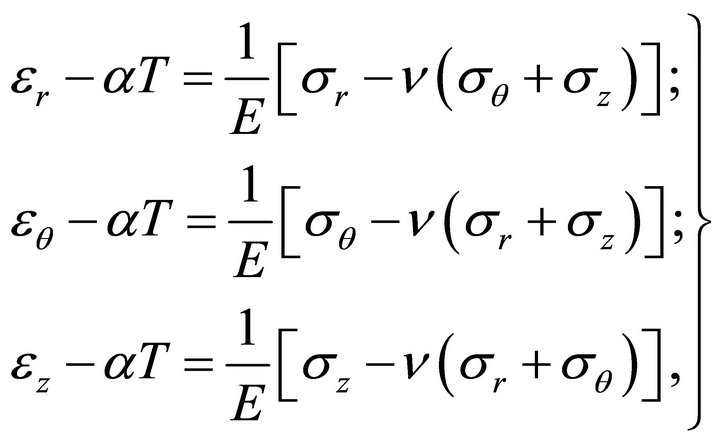 (9)
(9)
where 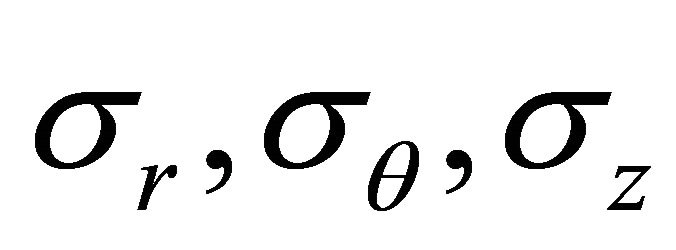 are normal stress tensor components;
are normal stress tensor components; 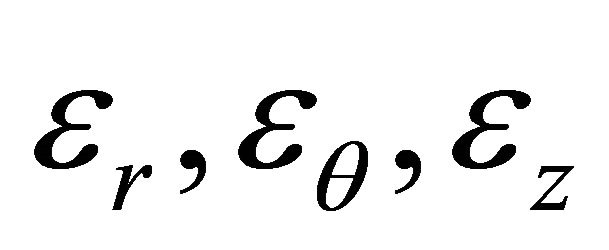 are relative deformations in the corresponding direction; α is thermal expansion coefficient; T is temperature; E is modulus of elasticity; ν is Poisson’s ratio.
are relative deformations in the corresponding direction; α is thermal expansion coefficient; T is temperature; E is modulus of elasticity; ν is Poisson’s ratio.
Because of symmetry shear strains and shear stresses equal zero. In the manufacture of long-length products axial deformation also equals zero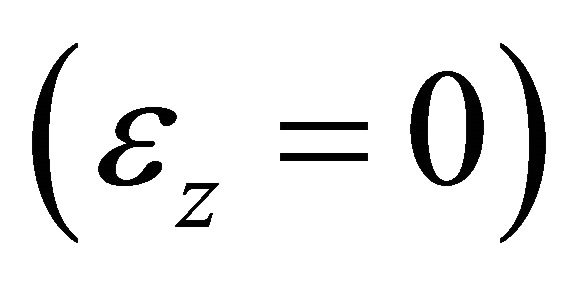 . From this condition it follows that:
. From this condition it follows that:
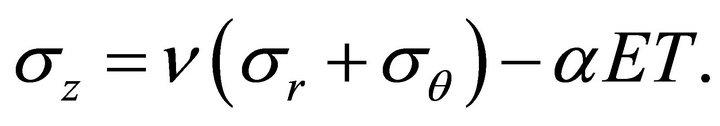 (10)
(10)
In view of equation (10) the Ratios (9) are converted to the following:
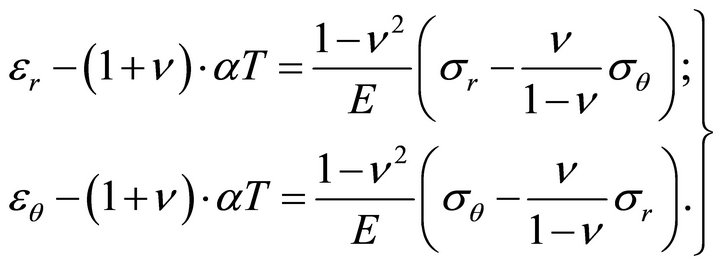 (11)
(11)
Under the action of temperature in the axisymmetrical billet radial displacements arise defining the components of the radial deformation tensor:
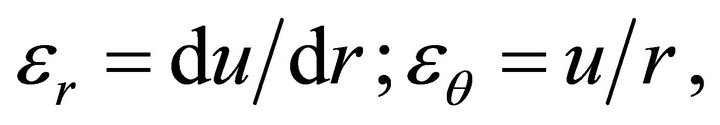 (12)
(12)
where u is displacement; r is radial coordinate.
The differential equation for radial displacements in the axisymmetrical problem of thermoelasticity [5] is as follows:
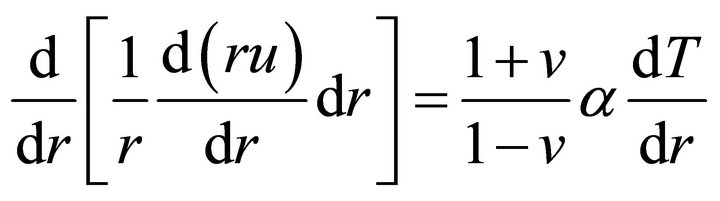 (13)
(13)
Equation (13) is addressed separately for the core, with the average temperature over the cross section Tc and for the shell, with the average temperature over thickness T0. Temperatures Tc and T0 are determined from the deformation conditions in the technological tool, with the different ratio of temperatures Tc and T0 depending on the thermal and mechanical properties of bimetallic billet components.
From Equation (13) taking into account Ratios (11) and (12) it follows that:
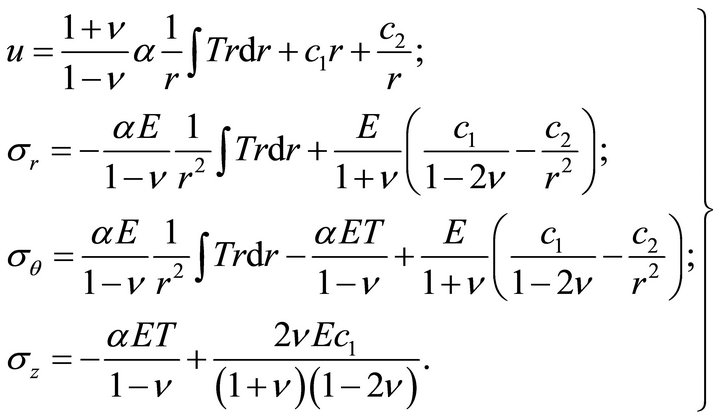 (14)
(14)
Expressions (14) are used independently for the core and for the shell. The constants of integration ![]() and
and 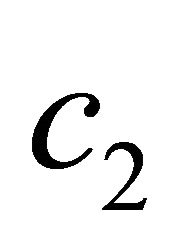 are determined from the corresponding boundary conditions. For example, for the core we believe
are determined from the corresponding boundary conditions. For example, for the core we believe 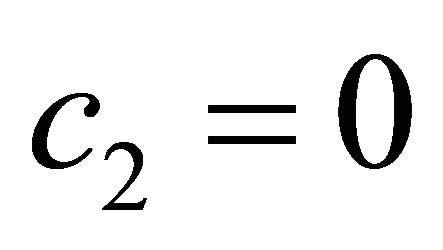 because
because 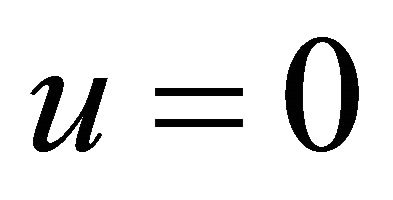 when
when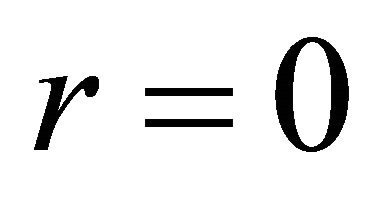 . We also assume that at the output of the drawing tool the core and the shell contact without force interaction, i.e.
. We also assume that at the output of the drawing tool the core and the shell contact without force interaction, i.e.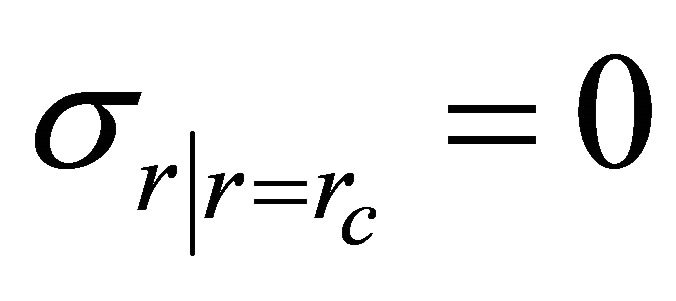 . Defining constants
. Defining constants ![]() and
and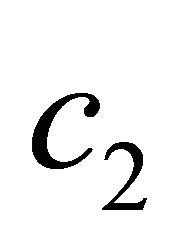 , we obtain the formula for the core:
, we obtain the formula for the core:
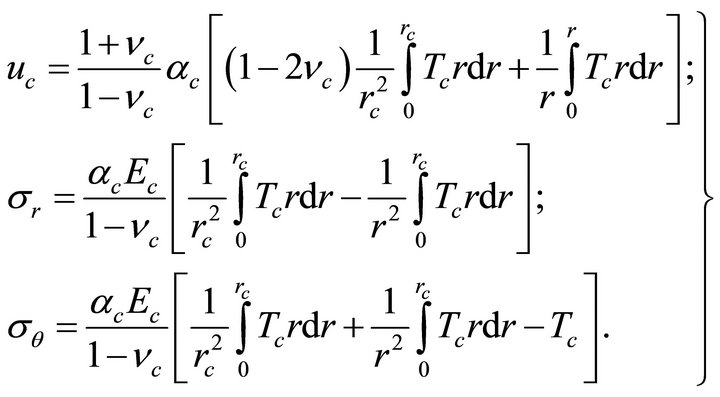 (15)
(15)
Accordingly for the shell we use boundary conditions to determine integration constants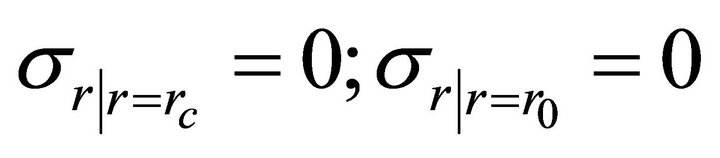 .
.
After determining the integration constants we obtain expressions for the shell:
 (16)
(16)
Possible gap between the core and the shell is determined by the size of radial displacements at the core-shell edge. From equation system (15) for r = r(c) we have
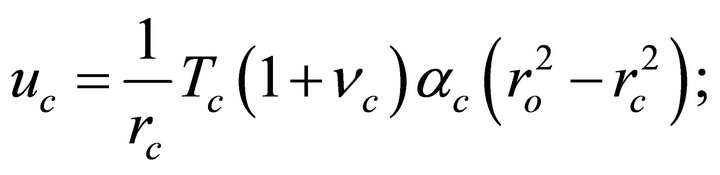 (17)
(17)
Respectively of the expressions (16) for the shell when r = r(c) we get:
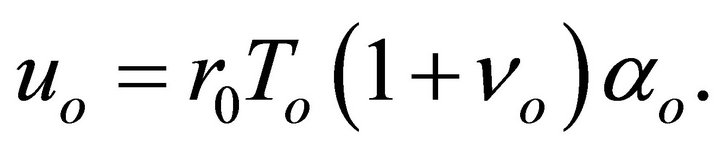 (18)
(18)
In case of equality of displacements 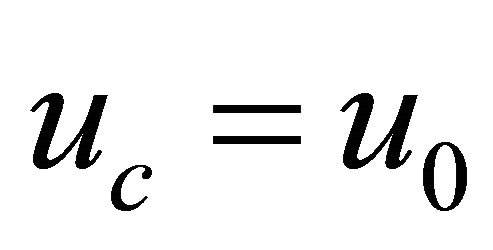 the contact in the bimetallic billet is maintained. When
the contact in the bimetallic billet is maintained. When 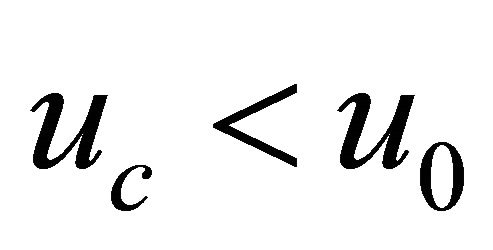 there appears a gap which is undesirable. The most favorable condition is
there appears a gap which is undesirable. The most favorable condition is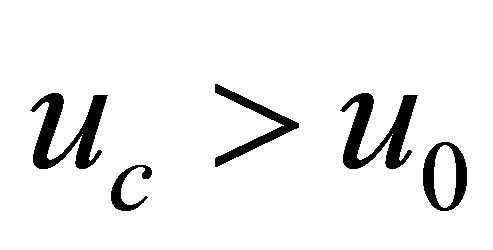 . It provides contact radial compressive stresses that help increase the metal core plasticity in deformation on subsequent passes. Thus, from the standpoint of thermoelasticity of bimetallic billets in drawing the most favorable ratio is [6].
. It provides contact radial compressive stresses that help increase the metal core plasticity in deformation on subsequent passes. Thus, from the standpoint of thermoelasticity of bimetallic billets in drawing the most favorable ratio is [6].
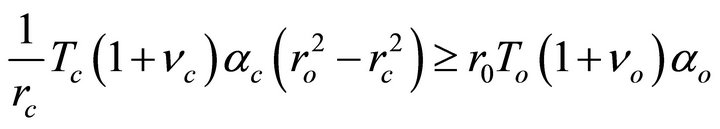 (19)
(19)
Ratio (19) is recommended for technological calculations in order to preserve the continuity of a bimetallic billet while being drawn. Equations (15) and (16) may be used to determine the stress state of bimetallic billet components.
3. Conclusion
The technological principles of low-temperature superconductor production for the magnetic system of the International thermonuclear experimental reactor (ITER) are outlined. Based on thermoelasticity equations, temperature modes in the superconductor production are established, which provides continuity of a bimetallic superconductor billet.
REFERENCES
- Chepetsky Mechanical Plant, “Superconductivity: The Experience of Organization of the High-Tech Production Capacities in JSC Chepetsky Mechanical Plant,” Nanotechnology, Environmental Science, No. 1, 2009, pp. 80- 83.
- A. K. Shikov, A. D. Nikulin and A. G. Silaev, “Development of Superconductors for ITER Magnet System in Russia,” Izvestiâ Vysših Učebnyh Zavedenij. Cvetnaâ Metallurgiâ, No. 1, 2003, pp. 36-43.
- R. Christensen, “Introduction into the Composite Mechanics,” MirPress, Moscow, 1982, 334 p.
- G. L. Kolmogorov, “Hydrodynamic Lubrication in Metal Forming,” Metallurgy, Moscow, 1986, 168 p.
- S. P. Timoshenko and J. Gud’er, “Elasticity Theory,” Nauka Publisher, Moscow, 1975, 576 p.
- G. L. Kolmogorov, V. N. Trofimov, M. G. Shtuca and T. V. Chernova, “Mechanics of Transversely-Isotropic Plastic Deformation of Composite Superconductor,” Perm National Research Polytechnic University, Perm, 2011.

