ABSTRACT

In this paper, we discuss one-dimensional optimal system and the invariant solutions of Coupled Burgers’ equations. By using Wu-differential characteristic set algorithm with the aid of Mathematica software, the classical symmetries of the Coupled Burgers’ equations are calculated, and the one-dimensional optimal system of Lie algebra is constructed. And we obtain the invariant solution of the Coupled Burgers’ equations corresponding to one element in one dimensional optimal system by using the invariant method. The results generalize the exact solutions of the Coupled Burgers’ equations.

Keywords:

Potential Symmetry, One-Dimensional Optimal System, Invariant Solution, Coupled Burgers’ Equations

1. Introduction

Lie group methods are perhaps the most powerful currently available in finding exact solutions of nonlinear partial differential equations (PDEs) [1] . This method has a profound impact on both pure and applied areas of mathematics, physics and mechanics, etc. [2] [3] [4] . Based on the symmetries of a PDEs, many important properties of the equation such as Lie algebras [5] , conservation laws [6] [7] [8] , exact solutions [9] [10] [11] , boundary value problem [12] can be considered successively.

In 1988, Bluman and Kumei [13] suggested a method to find a new class of symmetry for PDEs which can be written as conservative form. They analyzed the Lie symmetries of the system S that is obtained by introducing a potential as a further unknown function. In general, not all equations have potential symmetry, because the determining equations (DTEs) of potential symmetry are less than the DTEs of Lie symmetry. Potential symmetry can generate new symmetry and new invariant solutions which could not be obtained by Lie symmetry. Therefore, the study of potential symmetry of PDEs has become an important research topic in many fields [14] . Researchers such as Qu Changzheng [15] and Sophocleous [16] have made outstanding contributions in this field.

The plan of the paper is organized as follows. In Section 2, we obtained the potential symmetries and the one-dimensional optimal system of the Coupled Burgers’ equations based on differential characteristic set algorithm, and the corresponding Lie transformation groups are derived. In Section 3, we gave some new invariant solutions and exact solutions of the Coupled Burgers’ equations by applying Lie transformation groups on the invariant solutions. In Section 4, we give some discussions and conclusion remarks.

2. The Potential Symmetries, One-Dimensional Optimal System and Lie Transformation Groups of Coupled Burgers’ Equations

Let’s consider Coupled Burgers’ equations of Ref. [17] ,

$u_t-u_{xx}+2u{u}_x+\alpha {\left(uv\right)}_x=0,$ (1)

$v_t-v_{xx}+2v{v}_x+\beta {\left(uv\right)}_x=0.$ (2)

where $\alpha ,\beta$ are constants. The conserved form of Equations (1) (2) have the following forms

$u_t-{\left[u_x-u^2-\alpha \left(uv\right)\right]}_x=\mathrm{0,}$ (3)

$v_t-{\left[v_x-v^2-\beta \left(uv\right)\right]}_x=0.$ (4)

For obtaining the potential symmetry of Coupled Burgers’ equations, we introduce the potential variables $\varphi ,\psi$ , then Equations (3) (4) are equivalent to the potential systems

$\{\begin{array}{l}{\varphi }_x=u,\hfill \\ {\varphi }_t=u_x-u^2-\alpha \left(uv\right),\hfill \\ {\psi }_x=v,\hfill \\ {\psi }_t=v_x-v^2-\beta \left(uv\right).\hfill \end{array}$ (5)

The symmetry group of Equations (5) will be generated by the vector field of the form

$\begin{array}{c}X=\xi \left(x,t,u,v,\varphi ,\psi \right)\frac{\partial }{\partial x}+\tau \left(x,t,u,v,\varphi ,\psi \right)\frac{\partial }{\partial t}+{\eta }_1\left(x,t,u,v,\varphi ,\psi \right)\frac{\partial }{\partial u}\\ +{\eta }_2\left(x,t,u,v,\varphi ,\psi \right)\frac{\partial }{\partial v}+\gamma \left(x,t,u,v,\varphi ,\psi \right)\frac{\partial }{\partial \phi }+\delta \left(x,t,u,v,\varphi ,\psi \right)\frac{\partial }{\partial \psi },\end{array}$ (6)

where $\xi =\xi \left(x,t,u,v,\varphi ,\psi \right)$ , $\tau =\tau \left(x,t,u,v,\varphi ,\psi \right)$ , ${\eta }_1={\eta }_1\left(x,t,u,v,\varphi ,\psi \right)$ , ${\eta }_2={\eta }_2\left(x,t,u,v,\varphi ,\psi \right)$ , $\gamma =\gamma \left(x,t,u,v,\varphi ,\psi \right)$ , $\delta =\delta \left(x,t,u,v,\varphi ,\psi \right)$ are the infinitesimal functions.

Definition 1. If ${\xi }_{\varphi }^2+{\tau }_{\varphi }^2+{\eta }_{1\varphi }^2+{\eta }_{2\varphi }^2\ne 0$ , ${\xi }_{\psi }^2+{\tau }_{\psi }^2+{\eta }_{1\psi }^2+{\eta }_{2\psi }^2\ne 0$ , if and only if the infinitesimal functions $\xi ,\tau ,{\eta }_1,{\eta }_2$ have an essential dependence on the potential variables $\varphi ,\psi$ , then X is called the potential symmetry vector of Coupled Burgers’ Equations (1) (2).

2.1. The Potential Symmetries

In this section, we can divide it into three cases as following.

Case 1: $\alpha \ne 0$ , $\beta \ne 0$ .

According to the Lie algorithm, we obtain the determining equations of symmetry (6), but it is too difficult to get its solutions. However, we can obtain the following equivalent system of the determining system by corresponding to the characteristic set which is equivalent to the determining equations by using the differential characteristic set algorithm of Ref. [18]

$2{\eta }_1+u{\tau }_t=0,{\tau }_x={\tau }_u={\tau }_v={\tau }_{\varphi }={\tau }_{\psi }=0,{\eta }_{1x}={\eta }_{1t}={\eta }_{1v}={\eta }_{1\varphi }={\eta }_{1\psi }=0,$

${\gamma }_x={\gamma }_t={\gamma }_u={\gamma }_v={\gamma }_{\varphi }={\gamma }_{\psi }=0,{\delta }_x={\delta }_t={\delta }_u={\delta }_v={\delta }_{\varphi }={\delta }_{\psi }=0,$

${\xi }_t={\xi }_u={\xi }_v={\xi }_{\varphi }={\xi }_{\psi }=0,{\eta }_1+u{\eta }_{1u}=0,{\eta }_1+u{\xi }_x=0,v{\eta }_1-u{\eta }_2=0.$

By solving above equations, we obtain the infinitesimal functions as follows:

$\xi =-c_{3}x+c_5,\tau =-2c_{3}t+c_4,{\eta }_1=c_{3}u,{\eta }_2=c_{3}v,\gamma =c_1,\delta =c_2,$

where $c_i\left(i=1,\cdots ,5\right)$ are arbitrary symmetry parameters, and can give five finite symmetries of Equations (1) (2):

$X_1=\frac{\partial }{\partial \varphi },X_2=\frac{\partial }{\partial \psi },X_3=-x\frac{\partial }{\partial x}-2t\frac{\partial }{\partial t}+u\frac{\partial }{\partial u}+v\frac{\partial }{\partial v},X_4=\frac{\partial }{\partial t},X_5=\frac{\partial }{\partial x}.$

According to the definition 1, they are not potential symmetries.

Case 2: $\alpha =0$ , $\beta \ne 0$ , Equations (1) (2) have the following forms:

$u_t-u_{xx}+2u{u}_x=0,$ (7)

$v_t-v_{xx}+2v{v}_x+\beta {\left(uv\right)}_x=0,$ (8)

and Equations (5) have the following forms

$\{\begin{array}{l}{\varphi }_x=u,\hfill \\ {\varphi }_t=u_x-u^2,\hfill \\ {\psi }_x=v,\hfill \\ {\psi }_t=v_x-v^2-\beta \left(uv\right).\hfill \end{array}$ (9)

By the same manner, we can obtain the following equations:

${\xi }_t={\xi }_u={\xi }_v={\xi }_{\varphi }={\xi }_{\psi }=0,{\tau }_x={\tau }_u={\tau }_v={\tau }_{\varphi }={\tau }_{\psi }=0,$

${\eta }_{1x}={\eta }_{1t}={\eta }_{1v}={\eta }_{1\varphi }={\eta }_{1\psi }=0,{\eta }_{2x}={\eta }_{2t}={\eta }_{2uu}=0,$

${\gamma }_x={\gamma }_t={\gamma }_u={\gamma }_v={\gamma }_{\varphi }={\gamma }_{\psi }=0,{\delta }_x={\delta }_t={\delta }_u={\delta }_v=0,$

${\eta }_1-u{\eta }_{1u}=0,2{\eta }_1+u{\tau }_t=0,{\eta }_1+u{\xi }_x=0,v{\eta }_1-u{\eta }_2+u{\eta }_{2\psi }=0,$

${\eta }_2-v{\eta }_{2v}-u{\eta }_{2u}=0,{\eta }_{2\varphi }+u{\eta }_{2u}-\beta u{\eta }_{2u}-v{\eta }_{2u}=0,$

${\delta }_{\varphi }-{\eta }_{2u}=0,uv{\delta }_{\psi }+v{\eta }_1-u{\eta }_2+u^2{\eta }_{2u}=0.$

By calculating above equations, we obtain the infinitesimal functions:

$\xi =-c_{2}x+c_4,\tau =-2c_{2}t+c_3,{\eta }_1=c_{2}u,$

${\eta }_2=c_5\left(u+\frac{v}{-1+\beta }\right){\text{e}}^{\psi +\left(-1+\beta \right)\varphi }+c_{2}v+c_{6}v{\text{e}}^{\psi },$

$\gamma =c_1,\delta =\frac{c_5{\text{e}}^{\psi +\left(-1+\beta \right)\varphi }}{-1+\beta }+c_6{\text{e}}^{\psi }+c_7,$

where $c_i\left(i=1,\cdots ,7\right)$ are arbitrary symmetry parameters, and can give seven finite symmetries of Equations (7) (8):

$X_1=\frac{\partial }{\partial \varphi },X_2=-x\frac{\partial }{\partial x}-2t\frac{\partial }{\partial t}+u\frac{\partial }{\partial u}+v\frac{\partial }{\partial v},X_3=\frac{\partial }{\partial t},X_4=\frac{\partial }{\partial x},$

$X_5=\left(u+\frac{v}{-1+\beta }\right){\text{e}}^{\psi +\left(-1+\beta \right)\varphi }\frac{\partial }{\partial v}+\frac{{\text{e}}^{\psi +\left(-1+\beta \right)\varphi }}{-1+\beta }\frac{\partial }{\partial \psi },$

$X_6=v{\text{e}}^{\psi }\frac{\partial }{\partial v}+{\text{e}}^{\psi }\frac{\partial }{\partial \psi },X_7=\frac{\partial }{\partial \psi }.$

According to Definition 1, $X_5$ is the potential symmetry of Equations (7) (8).

Case 3: $\alpha \ne 0,\beta =0$ , Equations (1) (2) have the following forms:

$u_t-u_{xx}+2u{u}_x+\alpha {\left(uv\right)}_x=0,$ (10)

$v_t-v_{xx}+2v{v}_x=0.$ (11)

and Equations (5) has the following forms

$\{\begin{array}{l}{\varphi }_x=u,\hfill \\ {\varphi }_t=u_x-u^2-\alpha \left(uv\right),\hfill \\ {\psi }_x=v,\hfill \\ {\psi }_t=v_x-v^2\hfill \end{array}$ (12)

By the same manner, we can obtain the following equations:

${\xi }_t={\xi }_u={\xi }_v={\xi }_{\varphi }={\xi }_{\psi }=0,{\tau }_x={\tau }_u={\tau }_v={\tau }_{\varphi }={\tau }_{\psi }=0,$

${\eta }_{2x}={\eta }_{2t}={\eta }_{2u}={\eta }_{2\varphi }={\eta }_{2\psi }=0,{\eta }_{1x}={\eta }_{1t}={\eta }_{1uu}=0,$

${\gamma }_x={\gamma }_t={\gamma }_u={\gamma }_v=0,{\delta }_x={\delta }_t={\delta }_u={\delta }_v={\delta }_{\varphi }={\delta }_{\psi }=0,$

${\eta }_2-v{\eta }_{2v}=0,2{\eta }_2+v{\tau }_t=0,{\eta }_2+v{\xi }_x=0,$

${\eta }_1-v{\eta }_{1v}-u{\eta }_{1u}=0,v{\eta }_1-v{\eta }_{1\varphi }-u{\eta }_2=0,$

$-u{\eta }_1+v{\eta }_1-\alpha v{\eta }_1+v{\eta }_{1\psi }+u^2{\eta }_{1u}-uv{\eta }_{1u}+\alpha uv{\eta }_{1u}=0,$

$v{\gamma }_{\psi }-{\eta }_1+u{\eta }_{1u}=0,v{\gamma }_{\varphi }-v{\eta }_{1u}+{\eta }_2=0.$

By calculating above equations, we obtain the infinitesimal functions:

$\xi =-c_{2}x+c_3,\tau =-2c_{2}t+c_4,{\eta }_1=c_5\left(v+\frac{u}{-1+\alpha }\right){\text{e}}^{\varphi +\left(-1+\alpha \right)\psi }+c_{2}u+c_{6}v{\text{e}}^{\varphi },$

${\eta }_2=c_{2}v,\delta =c_1,\gamma =\frac{c_5{\text{e}}^{\varphi +\left(-1+\alpha \right)\psi }}{-1+\alpha }+c_6{\text{e}}^{\varphi }+c_7,$

where $c_i\left(i=1,\cdots ,7\right)$ are arbitrary symmetry parameters, and can give seven finite symmetries of Equations (10) (11):

$X_1=\frac{\partial }{\partial \psi },X_2=-x\frac{\partial }{\partial x}-2t\frac{\partial }{\partial t}+u\frac{\partial }{\partial u}+v\frac{\partial }{\partial v},X_3=\frac{\partial }{\partial x},X_4=\frac{\partial }{\partial t},$

$X_5=\left(v+\frac{u}{-1+\alpha }\right){\text{e}}^{\varphi +\left(-1+\alpha \right)\psi }\frac{\partial }{\partial u}+\frac{{\text{e}}^{\varphi +\left(-1+\alpha \right)\psi }}{-1+\alpha }\frac{\partial }{\partial \varphi },X_6=u{\text{e}}^{\varphi }\frac{\partial }{\partial u}+{\text{e}}^{\varphi }\frac{\partial }{\partial \varphi },X_7=\frac{\partial }{\partial \varphi }.$

According to Definition 1, $X_5$ is the potential symmetry of Equations (10) (11).

2.2. One-Dimensional Optimal System of the Coupled Burgers’ Equations

As is mentioned in process of Ref. [3] , the problem of finding an optimal of subgroups is equivalent to that of finding an optimal system of subalgebras. In this section, we will construct the optimal system of one-dimensional subalgebras of Coupled Burgers’ equations Equations (7) (8) by using the method presented in process of Ref. [3] .

In the case 2, we have obtained seven operators $X_1~X_7$ . Applying the commutator operators $\left[X_m,X_n\right]=X_m{X}_n-X_n{X}_m$ , we obtain the commutator table listed in Table 1 with the $\left(i\mathrm{,}j\right)$ -th entry indicating $\left[X_i\mathrm{,}{X}_j\right]$ . The commutation relations between these vector fields are given by the following table, the entry in row i and column j representing $\left[X_i\mathrm{,}{X}_j\right]$ .

From the above commutator table for this algebra, we use the Lie series and apply the following formula

$\begin{array}{c}Ad\left(\exp \left(\epsilon X_i\right)\right)X_j={\displaystyle \underset{n=0}{\overset{\infty }{\sum }}\frac{{\epsilon }^n}{n!}{\left(ad{X}_i\right)}^n\left(X_j\right)}\\ =X_j-\epsilon \left[X_i,X_j\right]+\frac{{\epsilon }^2}{2}\left[X_i,\left[X_i,X_j\right]\right]-\cdots \end{array}$ (13)

and compute the adjoint representation, we obtain the following table with the (i,j)-th entry indicating $Ad\left(\exp \left(\epsilon X_i\right)\right)X_j$ .

With the help of the adjoint representation Table 2, applying the adjoint action of X to $X=a_1{X}_1+a_2{X}_2+a_3{X}_3+a_4{X}_4+a_5{X}_5+a_6{X}_6+a_7{X}_7$ , we have

$\begin{array}{l}Ad\left(\exp \left({\epsilon }_1{X}_1\right)\right)X\\ =a_1{X}_1+a_2{X}_2+a_3{X}_3+a_4{X}_4+a_5{\text{e}}^{-{\epsilon }_1\left(-1+\beta \right)}{X}_5+a_6{X}_6+a_7{X}_7\\ =\left(X_1,X_2,X_3,X_4,X_5,X_6,X_7\right)\cdot Q_1\cdot {\left(a_1,a_2,a_3,a_4,a_5,a_6,a_7\right)}^{{\rm T}}.\end{array}$ (14)

It is easy to obtain $Q_1$ as follow,

$Q_1=\left(\begin{array}{ccccccc}1& 0& 0& 0& 0& 0& 0\\ 0& 1& 0& 0& 0& 0& 0\\ 0& 0& 1& 0& 0& 0& 0\\ 0& 0& 0& 1& 0& 0& 0\\ 0& 0& 0& 0& {\text{e}}^{-{\epsilon }_1\left(-1+\beta \right)}& 0& 0\\ 0& 0& 0& 0& 0& 1& 0\\ 0& 0& 0& 0& 0& 0& 1\end{array}\right)$

Similarly, the other matrices of the separate adjoint actions of the vectors $Q_2$ , $Q_3$ , $Q_4$ , $Q_5$ , $Q_6$ , $Q_7$ are found as follows:

$Q_2=\left(\begin{array}{ccccccc}1& 0& 0& 0& 0& 0& 0\\ 0& 1& 0& 0& 0& 0& 0\\ 0& 0& {\text{e}}^{-2{\epsilon }_2}& 0& 0& 0& 0\\ 0& 0& 0& {\text{e}}^{-{\epsilon }_2}& 0& 0& 0\\ 0& 0& 0& 0& 1& 0& 0\\ 0& 0& 0& 0& 0& 1& 0\\ 0& 0& 0& 0& 0& 0& 1\end{array}\right),Q_3=\left(\begin{array}{ccccccc}1& 0& 0& 0& 0& 0& 0\\ 0& 1& 0& 0& 0& 0& 0\\ 0& 2{\epsilon }_3& 1& 0& 0& 0& 0\\ 0& 0& 0& 1& 0& 0& 0\\ 0& 0& 0& 0& 1& 0& 0\\ 0& 0& 0& 0& 0& 1& 0\\ 0& 0& 0& 0& 0& 0& 1\end{array}\right),$

$Q_4=\left(\begin{array}{ccccccc}1& 0& 0& 0& 0& 0& 0\\ 0& 1& 0& 0& 0& 0& 0\\ 0& 0& 1& 0& 0& 0& 0\\ 0& {\epsilon }_4& 0& 1& 0& 0& 0\\ 0& 0& 0& 0& 1& 0& 0\\ 0& 0& 0& 0& 0& 1& 0\\ 0& 0& 0& 0& 0& 0& 1\end{array}\right),Q_5=\left(\begin{array}{ccccccc}1& 0& 0& 0& 0& 0& 0\\ 0& 1& 0& 0& 0& 0& 0\\ 0& 0& 1& 0& 0& 0& 0\\ 0& 0& 0& 1& 0& 0& 0\\ {\epsilon }_5\left(-1+\beta \right)& 0& 0& 0& 1& 0& {\epsilon }_5\\ 0& 0& 0& 0& 0& 1& 0\\ 0& 0& 0& 0& 0& 0& 1\end{array}\right),$

$Q_6=\left(\begin{array}{ccccccc}1& 0& 0& 0& 0& 0& 0\\ 0& 1& 0& 0& 0& 0& 0\\ 0& 0& 1& 0& 0& 0& 0\\ 0& 0& 0& 1& 0& 0& 0\\ 0& 0& 0& 0& 1& 0& 0\\ 0& 0& 0& 0& 0& 1& {\epsilon }_6\\ 0& 0& 0& 0& 0& 0& 1\end{array}\right),Q_7=\left(\begin{array}{ccccccc}1& 0& 0& 0& 0& 0& 0\\ 0& 1& 0& 0& 0& 0& 0\\ 0& 0& 1& 0& 0& 0& 0\\ 0& 0& 0& 1& 0& 0& 0\\ 0& 0& 0& 0& {\text{e}}^{-{\epsilon }_7}& 0& 0\\ 0& 0& 0& 0& 0& {\text{e}}^{-{\epsilon }_7}& 0\\ 0& 0& 0& 0& 0& 0& 1\end{array}\right).$

Then the general adjoint transformation matrix $Q$ of the Coupled Burgers’ equations is

$Q={\left(a_{i,j}\right)}_{7\times 7}=Q_1{Q}_2{Q}_3{Q}_4{Q}_5{Q}_6{Q}_7,$

the general adjoint transformation equation of the Coupled Burgers’ equations is

$\frac{1}{a}Q{\left({\alpha }_1\mathrm{,}{\alpha }_2\mathrm{,}{\alpha }_3\mathrm{,}{\alpha }_4\mathrm{,}{\alpha }_5\mathrm{,}{\alpha }_6\mathrm{,}{\alpha }_7\right)}^{{\rm T}}={\left({\beta }_1\mathrm{,}{\beta }_2\mathrm{,}{\beta }_3\mathrm{,}{\beta }_4\mathrm{,}{\beta }_5\mathrm{,}{\beta }_6\mathrm{,}{\beta }_7\right)}^{{\rm T}}\mathrm{,}$ (15)

where $a\ne 0$ . According to Equation (15), we got

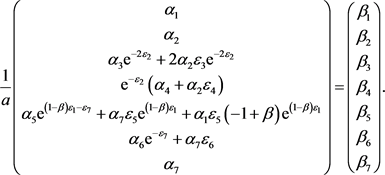(16)

Case 2.1 According to Equation (16), when ${\alpha }_7\ne 0$ , $\left({\alpha }_7=a,{\beta }_7=1\right)$ , it can also be divided into four cases:

1) When ${\alpha }_1\ne 0$ , ${\alpha }_2\ne 0$ , let ${\beta }_3={\beta }_4={\beta }_5={\beta }_6=0$ , we have ${\epsilon }_3=-\frac{{\alpha }_3}{2{\alpha }_2}$ , ${\epsilon }_4=-\frac{{\alpha }_4}{{\alpha }_2}$ , ${\epsilon }_5=-\frac{{\text{e}}^{-{\epsilon }_7}{\alpha }_5}{{\alpha }_7+{\alpha }_1\left(-1+\beta \right)}$ , ${\epsilon }_6=-\frac{{\text{e}}^{-{\epsilon }_7}{\alpha }_6}{{\alpha }_7}$ and $\overline{{\beta }_1}=\left(\lambda ,\lambda ,0,0,0,0,1\right)$ .

2) When ${\alpha }_1\ne 0$ , ${\alpha }_2\mathrm{<mo>\; =\; </mo>0}$ , Equation (16) has the follow formula

$\frac{1}{a}\left(\begin{array}{c}{\alpha }_1\\ 0\\ {\alpha }_3{\text{e}}^{-2{\epsilon }_2}\\ {\text{e}}^{-{\epsilon }_2}{\alpha }_4\\ {\alpha }_5{\text{e}}^{\left(1-\beta \right){\epsilon }_1-{\epsilon }_7}+{\alpha }_7{\epsilon }_5{\text{e}}^{\left(1-\beta \right){\epsilon }_1}+{\alpha }_1{\epsilon }_5\left(-1+\beta \right){\text{e}}^{\left(1-\beta \right){\epsilon }_1}\\ {\alpha }_6{\text{e}}^{-{\epsilon }_7}+{\alpha }_7{\epsilon }_6\\ {\alpha }_7\end{array}\right)=\left(\begin{array}{c}{\beta }_1\\ {\beta }_2\\ {\beta }_3\\ {\beta }_4\\ {\beta }_5\\ {\beta }_6\\ {\beta }_7\end{array}\right).$ (17)

It can also be divided into four cases:

a) when ${\alpha }_3\ne 0$ , ${\alpha }_4\ne 0$ , let ${\beta }_5={\beta }_6=0$ , we have ${\epsilon }_5=-\frac{{\text{e}}^{-{\epsilon }_7}{\alpha }_5}{{\alpha }_7+{\alpha }_1\left(-1+\beta \right)}$ , ${\epsilon }_6=-\frac{{\text{e}}^{-{\epsilon }_7}{\alpha }_6}{{\alpha }_7}$ and $\overline{{\beta }_2}=\left(\lambda \mathrm{,0,1,1,0,0,1}\right)$ .

b) when ${\alpha }_3\ne 0$ , ${\alpha }_4=0$ , we can obtain ${\beta }_4={\lambda }_3$ and $\overline{{\beta }_3}=\left(\lambda ,0,1,0,0,0,1\right)$ .

c) when ${\alpha }_3=0$ , ${\alpha }_4\ne 0$ , we have $\overline{{\beta }_4}=\left(\lambda ,0,0,1,0,0,1\right)$ .

d) when ${\alpha }_3=0$ , ${\alpha }_4=0$ . we have $\overline{{\beta }_5}=\left(\lambda ,0,0,0,0,0,1\right)$ .

3) When ${\alpha }_1=0$ , ${\alpha }_2\ne 0$ , let ${\beta }_3={\beta }_4={\beta }_5={\beta }_6=0$ , we have ${\epsilon }_3=-\frac{{\alpha }_3}{2{\alpha }_2}$ , ${\epsilon }_4=-\frac{{\alpha }_4}{{\alpha }_2}$ , ${\epsilon }_5=-\frac{{\text{e}}^{-{\epsilon }_7}{\alpha }_5}{{\alpha }_7}$ , ${\epsilon }_6=-\frac{{\text{e}}^{-{\epsilon }_7}{\alpha }_6}{{\alpha }_7}$ and $\overline{{\beta }_6}=\left(0,\lambda ,0,0,0,0,1\right)$ .

4) When ${\alpha }_1=0$ , ${\alpha }_2=0$ , we find that it can also be divided into four cases:

a) If ${\alpha }_3\ne 0$ , ${\alpha }_4\ne 0$ , let ${\beta }_5={\beta }_6=0$ , we have ${\epsilon }_5=-\frac{{\text{e}}^{-{\epsilon }_7}{\alpha }_5}{{\alpha }_7}$ , ${\epsilon }_6=-\frac{{\text{e}}^{-{\epsilon }_7}{\alpha }_6}{{\alpha }_7}$ and $\overline{{\beta }_7}=\left(0,0,1,1,0,0,1\right)$ ;

b) If ${\alpha }_3\ne 0$ , ${\alpha }_4=0$ , we have $\overline{{\beta }_8}=\left(0,0,1,0,0,0,1\right)$ ;

c) If ${\alpha }_3=0$ , ${\alpha }_4\ne 0$ , we have $\overline{{\beta }_9}=\left(0,0,0,1,0,0,1\right)$ ;

d) If ${\alpha }_3=0$ , ${\alpha }_4=0$ , we have $\overline{{\beta }_{10}}=\left(0,0,0,0,0,0,1\right)$ .

Case 2.2 When ${\alpha }_7=0$ , ${\alpha }_6\ne 0$ , we obtain ${\beta }_7=0$ . Equation (16) has the follow formula

$\frac{1}{a}\left(\begin{array}{c}{\alpha }_1\\ {\alpha }_2\\ {\alpha }_3{\text{e}}^{-2{\epsilon }_2}+2{\alpha }_2{\epsilon }_3{\text{e}}^{-2{\epsilon }_2}\\ {\text{e}}^{-{\epsilon }_2}\left({\alpha }_4+{\alpha }_2{\epsilon }_4\right)\\ {\alpha }_5{\text{e}}^{\left(1-\beta \right){\epsilon }_1-{\epsilon }_7}+{\alpha }_1{\epsilon }_5\left(-1+\beta \right){\text{e}}^{\left(1-\beta \right){\epsilon }_1}\\ {\alpha }_6{\text{e}}^{-{\epsilon }_7}\\ 0\end{array}\right)=\left(\begin{array}{c}{\beta }_1\\ {\beta }_2\\ {\beta }_3\\ {\beta }_4\\ {\beta }_5\\ {\beta }_6\\ {\beta }_7\end{array}\right).$ (18)

It can also be divided into four cases:

1) When ${\alpha }_1\ne 0$ , ${\alpha }_2\ne 0$ , we can obtain ${\beta }_2={\lambda }_7$ , adjust $\frac{1}{a}$ , make ${\beta }_6=1$ . Let ${\beta }_3={\beta }_4={\beta }_5=0$ , we can obtain ${\epsilon }_3=-\frac{{\alpha }_3}{2{\alpha }_2}$ , ${\epsilon }_4=-\frac{{\alpha }_4}{{\alpha }_2}$ , ${\epsilon }_5=-\frac{{\text{e}}^{-{\epsilon }_7}{\alpha }_5}{{\alpha }_1\left(-1+\beta \right)}$ , we have $\overline{{\beta }_{11}}=\left(\lambda ,\lambda ,0,0,0,1,0\right)$ .

2) When ${\alpha }_1\ne 0$ , ${\alpha }_2=0$ , it can also be divided into four cases:

a) when ${\alpha }_3\ne 0$ , ${\alpha }_4\ne 0$ , we have $\overline{{\beta }_{12}}=\left(\lambda ,0,1,1,0,1,0\right)$ ;

b) when ${\alpha }_3\ne 0$ , ${\alpha }_4=0$ , we have $\overline{{\beta }_{13}}=\left(1,0,1,0,0,1,0\right)$ ;

c) when ${\alpha }_3=0$ , ${\alpha }_4\ne 0$ , we have $\overline{{\beta }_{14}}=\left(\lambda ,0,0,1,0,1,0\right)$ ;

d) when ${\alpha }_3=0$ , ${\alpha }_4=0$ , we have $\overline{{\beta }_{15}}=\left(1,0,0,0,0,1,0\right)$ .

3) When ${\alpha }_1=0$ , ${\alpha }_2\ne 0$ , if ${\alpha }_5\ne 0$ ,

${\text{e}}^{\left(1-\beta \right){\epsilon }_1-{\epsilon }_7}{\alpha }_5=\{\begin{array}{l}1,{\alpha }_5>0\\ -1,{\alpha }_5<0\end{array}$

We have $\overline{{\beta }_{16}}=\left(0,1,0,0,\pm 1,1,0\right)$ , if ${\alpha }_5=0$ , we have $\overline{{\beta }_{17}}=\left(0,1,0,0,0,1,0\right)$ .

4) When ${\alpha }_1=0$ , ${\alpha }_2=0$ , we find that it can also be divided into eight cases:

a) when ${\alpha }_3\ne 0$ , ${\alpha }_4\ne 0$ , ${\alpha }_5\ne 0$ , we have $\overline{{\beta }_{18}}=\left(0,0,1,1,\pm 1,1,0\right)$ ;

b) when ${\alpha }_3\ne 0$ , ${\alpha }_4=0$ , ${\alpha }_5=0$ , we have $\overline{{\beta }_{19}}=\left(0,0,1,0,0,1,0\right)$ ;

c) when ${\alpha }_3=0$ , ${\alpha }_4\ne 0$ , ${\alpha }_5=0$ , we have $\overline{{\beta }_{20}}=\left(0,0,0,1,0,1,0\right)$ ;

d) when ${\alpha }_3=0$ , ${\alpha }_4=0$ , ${\alpha }_5\ne 0$ , we have $\overline{{\beta }_{21}}=\left(0,0,0,0,\pm 1,1,0\right)$ ;

e) when ${\alpha }_3\ne 0$ , ${\alpha }_4\ne 0$ , ${\alpha }_5=0$ , we have $\overline{{\beta }_{22}}=\left(0,0,1,1,0,1,0\right)$ ;

f) when ${\alpha }_3\ne 0$ , ${\alpha }_4=0$ , ${\alpha }_5\ne 0$ , we have $\overline{{\beta }_{23}}=\left(0,0,1,0,\pm 1,1,0\right)$ ;

g) when ${\alpha }_3=0$ , ${\alpha }_4\ne 0$ , ${\alpha }_5\ne 0$ , we have $\overline{{\beta }_{24}}=\left(0,0,0,1,\pm 1,1,0\right)$ ;

h) when ${\alpha }_3=0$ , ${\alpha }_4=0$ , ${\alpha }_5=0$ , we have $\overline{{\beta }_{25}}=\left(0,0,0,0,0,1,0\right)$ .

Case 2.3 When ${\alpha }_7=0$ , ${\alpha }_6=0$ , ${\alpha }_5\ne 0$ , we obtain ${\beta }_7=0$ , ${\beta }_6=0$ . Equation (16) has the follow formula

$\frac{1}{a}\left(\begin{array}{c}{\alpha }_1\\ {\alpha }_2\\ {\alpha }_3{\text{e}}^{-2{\epsilon }_2}+2{\alpha }_2{\epsilon }_3{\text{e}}^{-2{\epsilon }_2}\\ {\text{e}}^{-{\epsilon }_2}\left({\alpha }_4+{\alpha }_2{\epsilon }_4\right)\\ {\alpha }_5{\text{e}}^{\left(1-\beta \right){\epsilon }_1-{\epsilon }_7}+{\alpha }_1{\epsilon }_5\left(-1+\beta \right){\text{e}}^{\left(1-\beta \right){\epsilon }_1}\\ 0\\ 0\end{array}\right)=\left(\begin{array}{c}{\beta }_1\\ {\beta }_2\\ {\beta }_3\\ {\beta }_4\\ {\beta }_5\\ {\beta }_6\\ {\beta }_7\end{array}\right).$ (19)

We find that it can also be divided into four cases:

1) When ${\alpha }_1\ne 0$ , ${\alpha }_2\ne 0$ , let ${\beta }_3={\beta }_4={\beta }_5=0$ , we can obtain ${\epsilon }_3=-\frac{{\alpha }_3}{2{\alpha }_2}$ , ${\epsilon }_4=-\frac{{\alpha }_4}{{\alpha }_2}$ , ${\epsilon }_5=-\frac{{\text{e}}^{-{\epsilon }_7}{\alpha }_5}{{\alpha }_1\left(-1+\beta \right)}$ , we have $\overline{{\beta }_{26}}=\left(\lambda ,1,0,0,0,0,0\right).$

2) When ${\alpha }_1\ne 0$ , ${\alpha }_2=0$ , we find that it can also be divided four cases:

a) ${\alpha }_3\ne 0$ , ${\alpha }_4\ne 0$ , we have $\overline{{\beta }_{27}}=\left(\lambda ,0,1,1,0,0,0\right)$ ;

b) ${\alpha }_3\ne 0$ , ${\alpha }_4=0$ , we have $\overline{{\beta }_{28}}=\left(1,0,1,0,0,0,0\right)$ ;

c) ${\alpha }_3=0$ , ${\alpha }_4\ne 0$ , we have $\overline{{\beta }_{29}}=\left(\lambda ,0,0,1,0,0,0\right)$ ;

d) ${\alpha }_3=0$ , ${\alpha }_4=0$ , we have $\overline{{\beta }_{30}}=\left(1,0,0,0,0,0,0\right)$ .

3) When ${\alpha }_1=0$ , ${\alpha }_2\ne 0$ , we have $\overline{{\beta }_{31}}=\left(0,1,0,0,\pm 1,0,0\right)$ .

4) When ${\alpha }_1=0$ , ${\alpha }_2=0$ , we find that it can also be divided four cases:

a) ${\alpha }_3\ne 0$ , ${\alpha }_4\ne 0$ , we have $\overline{{\beta }_{32}}=\left(0,0,1,1,\pm 1,0,0\right)$ ;

b) ${\alpha }_3\ne 0$ , ${\alpha }_4=0$ , we have $\overline{{\beta }_{33}}=\left(0,0,1,0,\pm 1,0,0\right)$ ;

c) ${\alpha }_3=0$ , ${\alpha }_4\ne 0$ , we have $\overline{{\beta }_{34}}=\left(0,0,0,1,\pm 1,0,0\right)$ ;

d) ${\alpha }_3=0$ , ${\alpha }_4=0$ , we have $\overline{{\beta }_{35}}=\left(0,0,0,0,1,0,0\right)$ .

Case 2.4 When ${\alpha }_7=0$ , ${\alpha }_6=0$ , ${\alpha }_5=0$ , ${\alpha }_4\ne 0$ , we obtain ${\beta }_7=0$ , ${\beta }_6=0$ . Equation (16) has the follow formula

$\frac{1}{a}\left(\begin{array}{c}{\alpha }_1\\ {\alpha }_2\\ {\alpha }_3{\text{e}}^{-2{\epsilon }_2}+2{\alpha }_2{\epsilon }_3{\text{e}}^{-2{\epsilon }_2}\\ {\text{e}}^{-{\epsilon }_2}\left({\alpha }_4+{\alpha }_2{\epsilon }_4\right)\\ {\alpha }_1{\epsilon }_5\left(-1+\beta \right){\text{e}}^{\left(1-\beta \right){\epsilon }_1}\\ 0\\ 0\end{array}\right)=\left(\begin{array}{c}{\beta }_1\\ {\beta }_2\\ {\beta }_3\\ {\beta }_4\\ {\beta }_5\\ {\beta }_6\\ {\beta }_7\end{array}\right).$ (20)

we find that it can also be divided into four cases:

1) When ${\alpha }_1\ne 0$ , ${\alpha }_2\ne 0$ , let ${\beta }_3=0$ , we can obtain ${\epsilon }_3=-\frac{{\alpha }_3}{2{\alpha }_2}$ , ${\epsilon }_4=-\frac{{\alpha }_4}{{\alpha }_2}$ , we have $\overline{{\beta }_{36}}=\left(1,\lambda ,0,1,\pm 1,0,0\right)$ .

2) When ${\alpha }_1\ne 0$ , ${\alpha }_2=0$ , if ${\alpha }_3\ne 0$ , we have $\overline{{\beta }_{37}}=\left(\lambda ,0,1,1,\pm 1,0,0\right)$ , if ${\alpha }_3=0$ , we have $\overline{{\beta }_{38}}=\left(\lambda ,0,0,1,\pm 1,0,0\right)$ .

3) When ${\alpha }_1=0$ , ${\alpha }_2\ne 0$ , let ${\beta }_3=0$ , we obtain ${\epsilon }_3=-\frac{{\alpha }_3}{2{\alpha }_2}$ ${\epsilon }_4=-\frac{{\alpha }_4}{{\alpha }_2}$ , we have $\overline{{\beta }_{39}}=\left(0,1,0,1,0,0,0\right)$ .

4) When ${\alpha }_1=0$ , ${\alpha }_2=0$ , if ${\alpha }_3\ne 0$ , we have $\overline{{\beta }_{40}}=\left(0,0,1,1,0,0,0\right)$ , if ${\alpha }_3=0$ , we have $\overline{{\beta }_{41}}=\left(0,0,0,1,0,0,0\right)$ .

Case 2.5 When ${\alpha }_7=0$ , ${\alpha }_6=0$ , ${\alpha }_5=0$ , ${\alpha }_4=0$ , ${\alpha }_3\ne 0$ , we obtain ${\beta }_7=0$ , ${\beta }_6=0$ . Equation (16) has the follow formula

$\frac{1}{a}\left(\begin{array}{c}{\alpha }_1\\ {\alpha }_2\\ {\alpha }_3{\text{e}}^{-2{\epsilon }_2}+2{\alpha }_2{\epsilon }_3{\text{e}}^{-2{\epsilon }_2}\\ {\text{e}}^{-{\epsilon }_2}{\alpha }_2{\epsilon }_4\\ {\alpha }_1{\epsilon }_5\left(-1+\beta \right){\text{e}}^{\left(1-\beta \right){\epsilon }_1}\\ 0\\ 0\end{array}\right)=\left(\begin{array}{c}{\beta }_1\\ {\beta }_2\\ {\beta }_3\\ {\beta }_4\\ {\beta }_5\\ {\beta }_6\\ {\beta }_7\end{array}\right).$ (21)

We find that it can also be divided into four cases:

1) When ${\alpha }_1\ne 0$ , ${\alpha }_2\ne 0$ , we have $\overline{{\beta }_{42}}=\left(\lambda ,1,0,0,\pm 1,0,0\right)$ ;

2) When ${\alpha }_1\ne 0$ , ${\alpha }_2=0$ , we have $\overline{{\beta }_{43}}=\left(1,0,1,0,\pm 1,0,0\right)$ ;

3) When ${\alpha }_1=0$ , ${\alpha }_2\ne 0$ , we have $\overline{{\beta }_{44}}=\left(0,1,0,0,0,0,0\right)$ ;

4) When ${\alpha }_1=0$ , ${\alpha }_2=0$ , we have $\overline{{\beta }_{45}}=\left(0,0,1,0,0,0,0\right)$ .

Case 2.6 When ${\alpha }_7=0$ , ${\alpha }_6=0$ , ${\alpha }_5=0$ , ${\alpha }_4=0$ , ${\alpha }_3=0$ , ${\alpha }_2\ne 0$ , Equation (16) has the follow formula

$\frac{1}{a}\left(\begin{array}{c}{\alpha }_1\\ {\alpha }_2\\ 2{\alpha }_2{\epsilon }_3{\text{e}}^{-2{\epsilon }_2}\\ {\text{e}}^{-{\epsilon }_2}{\alpha }_2{\epsilon }_4\\ {\alpha }_1{\epsilon }_5\left(-1+\beta \right){\text{e}}^{\left(1-\beta \right){\epsilon }_1}\\ 0\\ 0\end{array}\right)=\left(\begin{array}{c}{\beta }_1\\ {\beta }_2\\ {\beta }_3\\ {\beta }_4\\ {\beta }_5\\ {\beta }_6\\ {\beta }_7\end{array}\right).$ (22)