Open Journal of Microphysics
Vol.3 No.3(2013), Article ID:35704,4 pages DOI:10.4236/ojm.2013.33011
Calogero Model with Different Masses
Université du Burundi, Institut de Pédagogie Appliquée, Bujumbura, Burundi
Email: nininaha@yahoo.fr
Copyright © 2013 Ancilla Nininahazwe. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received May 15, 2013; revised June 15, 2013; accepted June 22, 2013
Keywords: Multispecies One-Dimensional Calogero; Equal Masses; Different Masses; Usual Calogero Model
ABSTRACT
We study a multispecies one-dimensional Calogero model with twoand three-body interactions. Here, we factorize the ground state 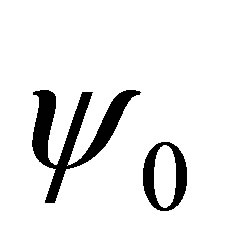 out of the Hamiltonian H in order to get the new operator
out of the Hamiltonian H in order to get the new operator  which preserves some spaces of polynomials
which preserves some spaces of polynomials 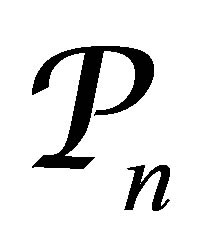 in the case of equal masses, i.e.
in the case of equal masses, i.e. 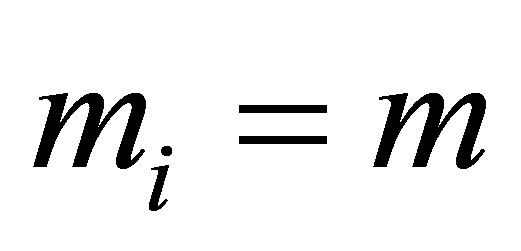 (the usual Calogero model) and in the case with different masses. The spectrum of these both cases is found easily.
(the usual Calogero model) and in the case with different masses. The spectrum of these both cases is found easily.
1. Introduction
The ordinary Cologero [1,2] model describes N indistinguishable particles on the line which interact through an inverse-square two-body interaction. The model is completely integrable in both the classical and quantum case [3]. The spectrum is known and the wave functions are given implicitly. In the present paper, which is in a sense a continuation of the investigation of the ordinary model [4], we use an algebraic method to find some of the salient features of the multispecies Calogero model on the line with twoand three-body interactions. After performing a certain transformation of the operator H, we get a new Hamiltonian  for which we find its spectrum in the both cases with equal masses and different masses.
for which we find its spectrum in the both cases with equal masses and different masses.
2. Calogero Model with Different Masses
In this section, we reconsider the “multispecies” Calogero model considered in . The Hamiltonian reads
. The Hamiltonian reads
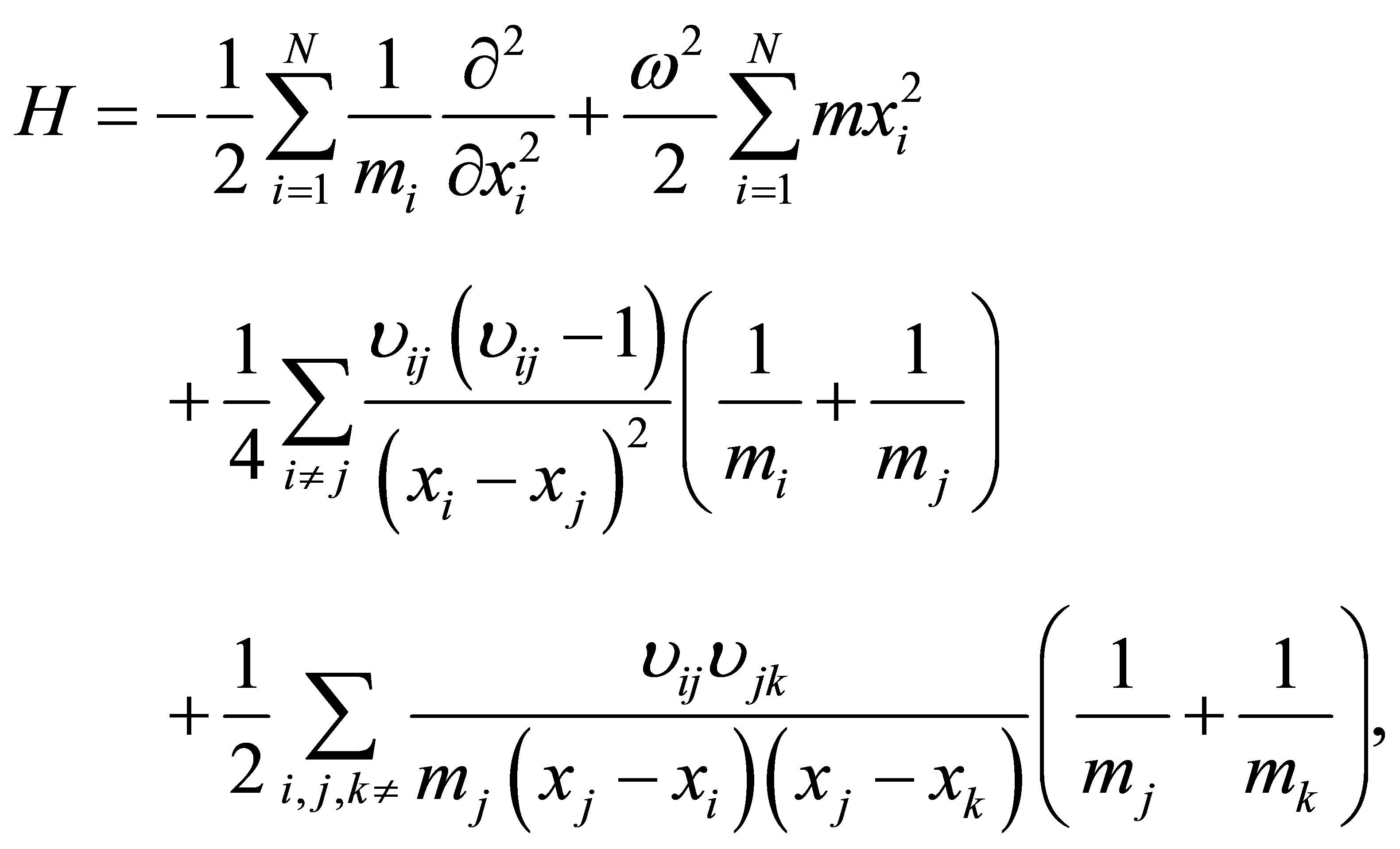 (1)
(1)
where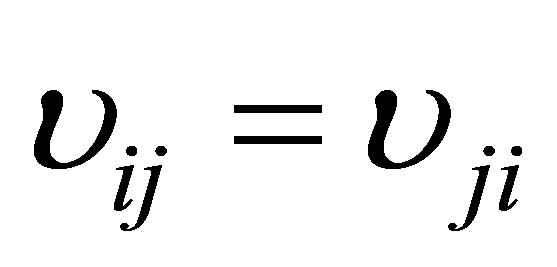 .
.
We factorize the full ground state
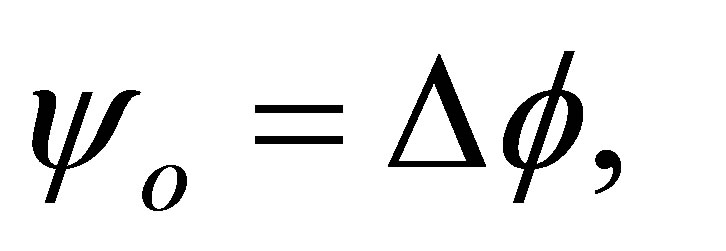 (2)
(2)
with
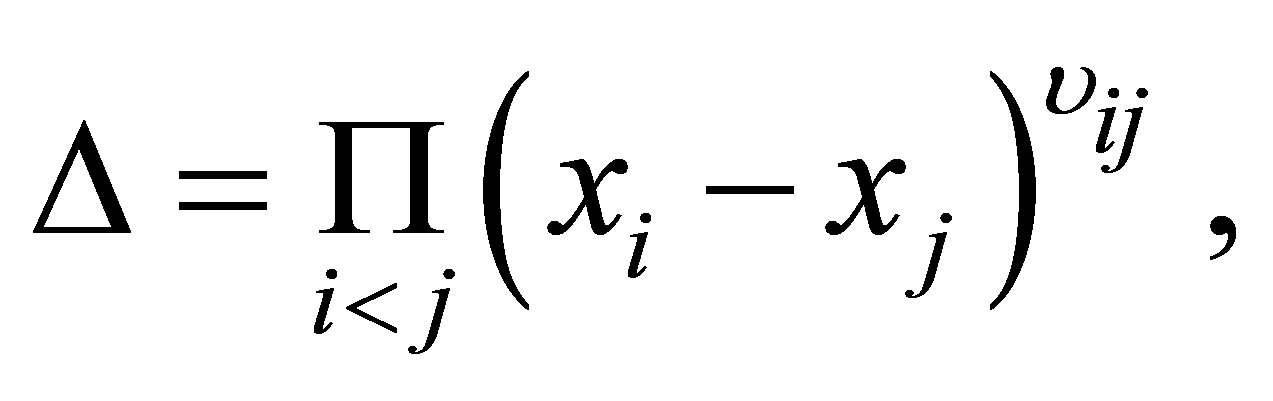 (3)
(3)
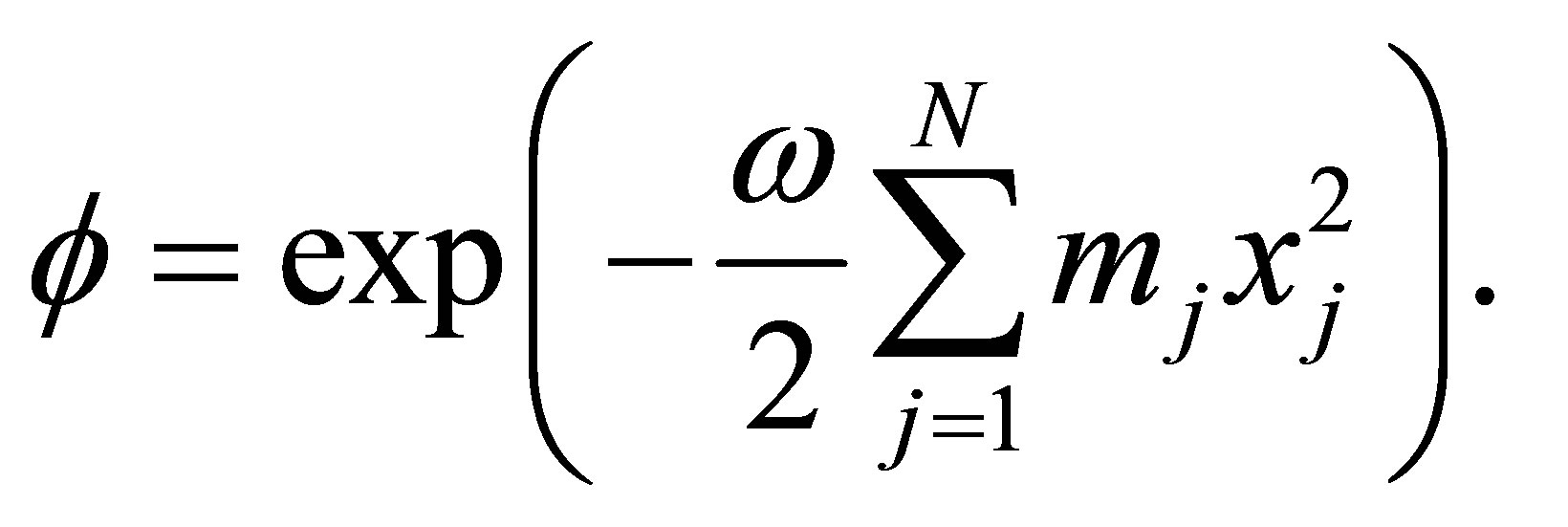 (4)
(4)
When factorizing the factor 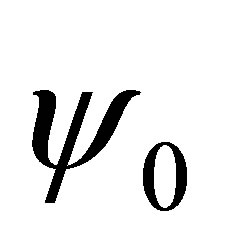 out of H, we got the new operator
out of H, we got the new operator
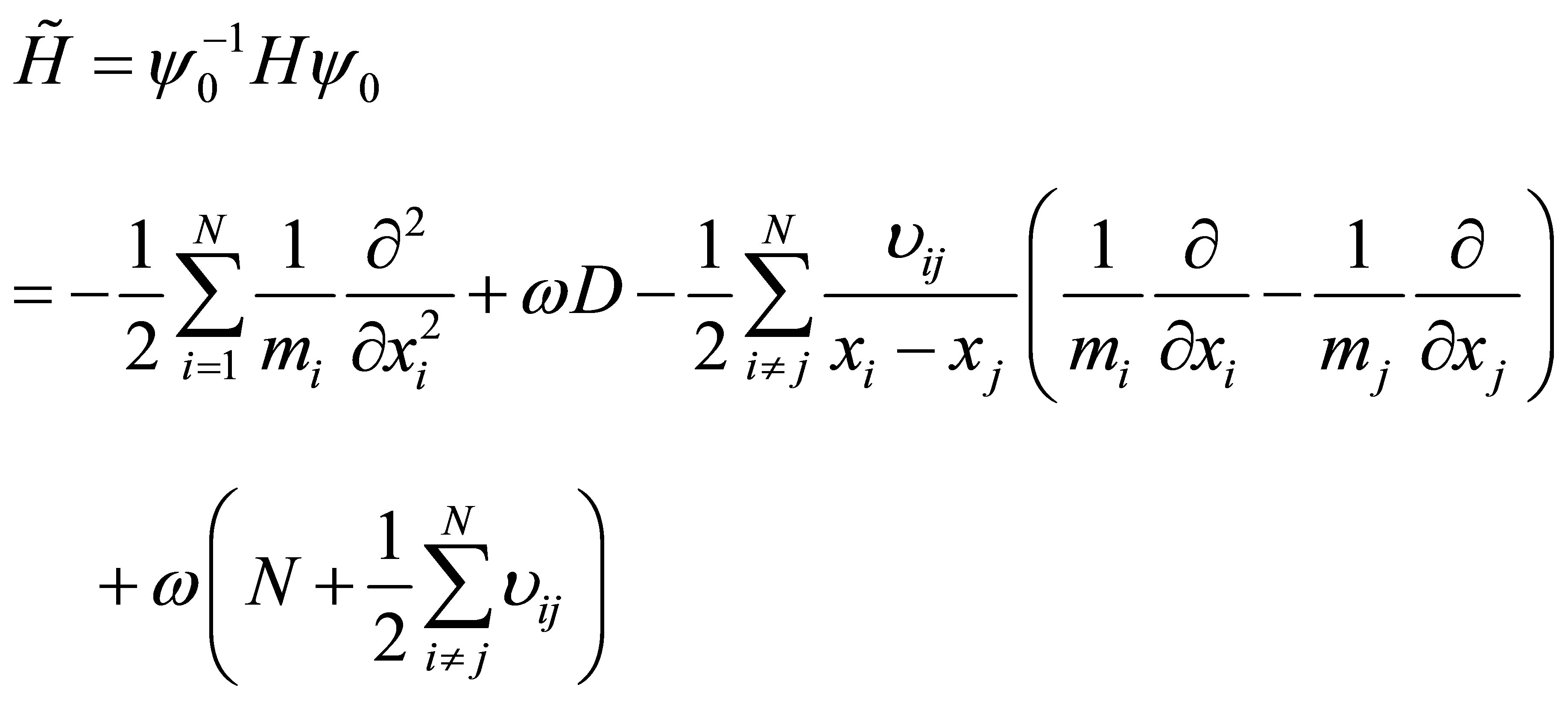 (5)
(5)
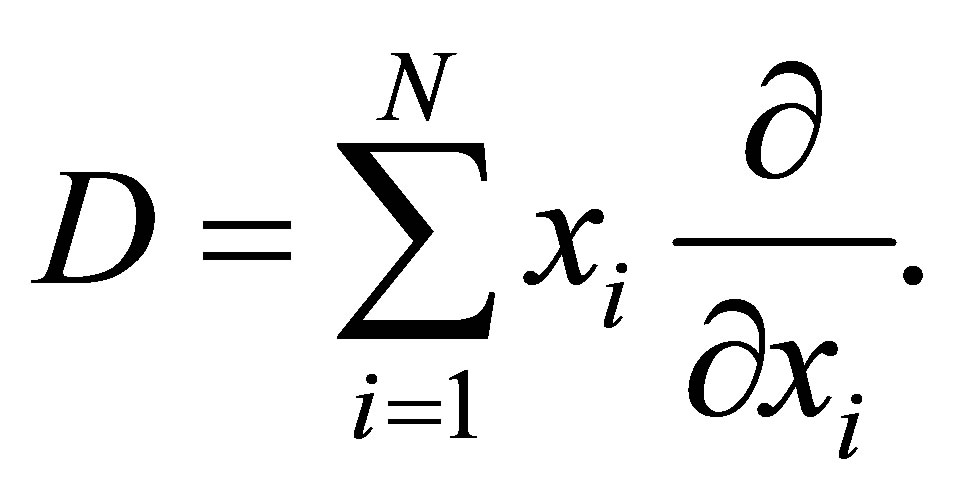 (6)
(6)
The operator  preserves some spaces of polynomials that we would like to study and compare with the invariant spaces
preserves some spaces of polynomials that we would like to study and compare with the invariant spaces 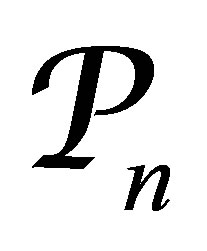 available in the case of equal masses, i.e.
available in the case of equal masses, i.e. 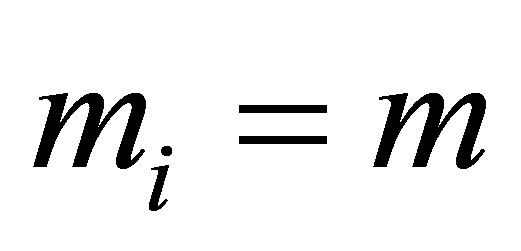 (the usual Calogero model). We first proceed with the
(the usual Calogero model). We first proceed with the 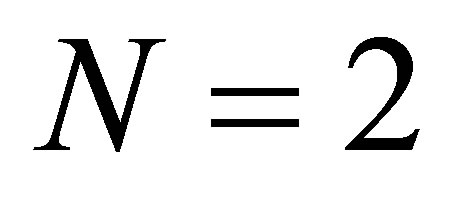 i.e. two body case. Then it is easy to check that the following vector spaces are preserved by
i.e. two body case. Then it is easy to check that the following vector spaces are preserved by :
:
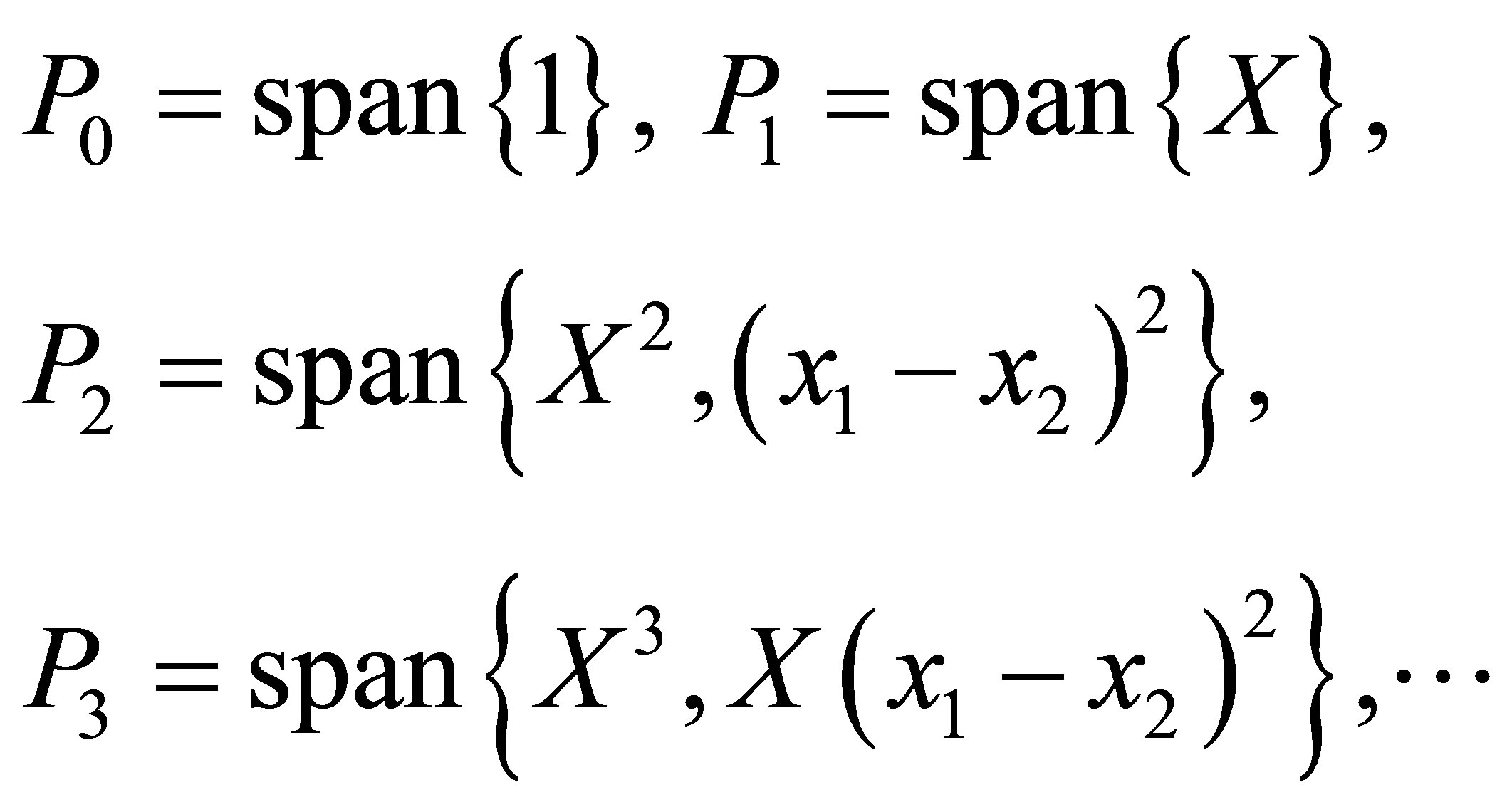 (7)
(7)
with
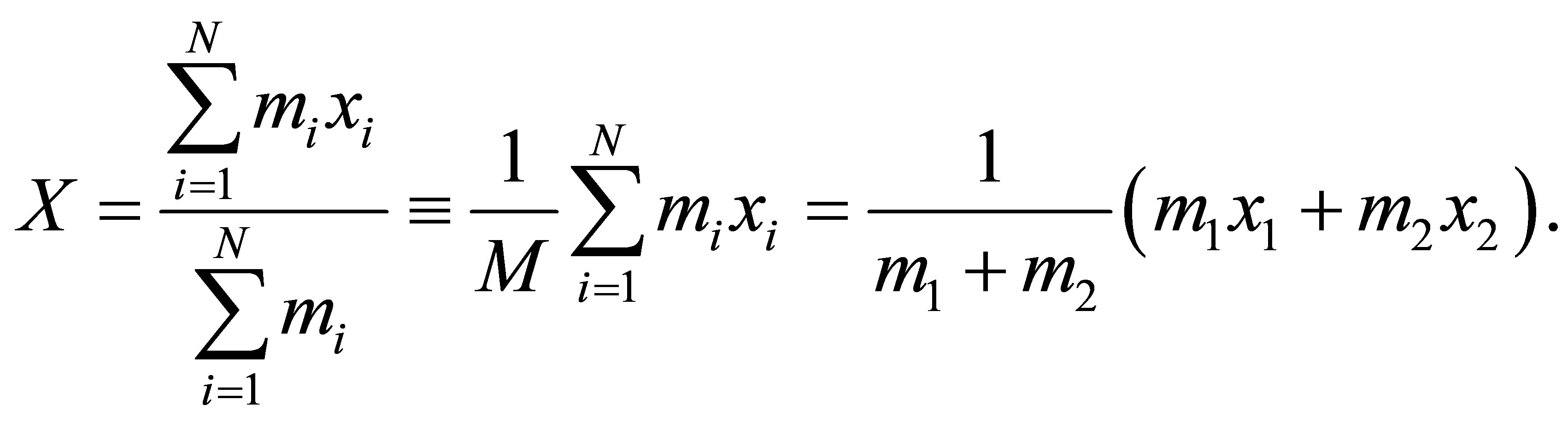 (8)
(8)
It should be stressed that the combination  has to be eliminated from
has to be eliminated from 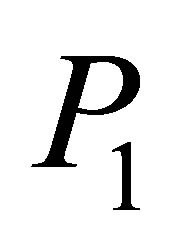 because it is not preserved by the part
because it is not preserved by the part
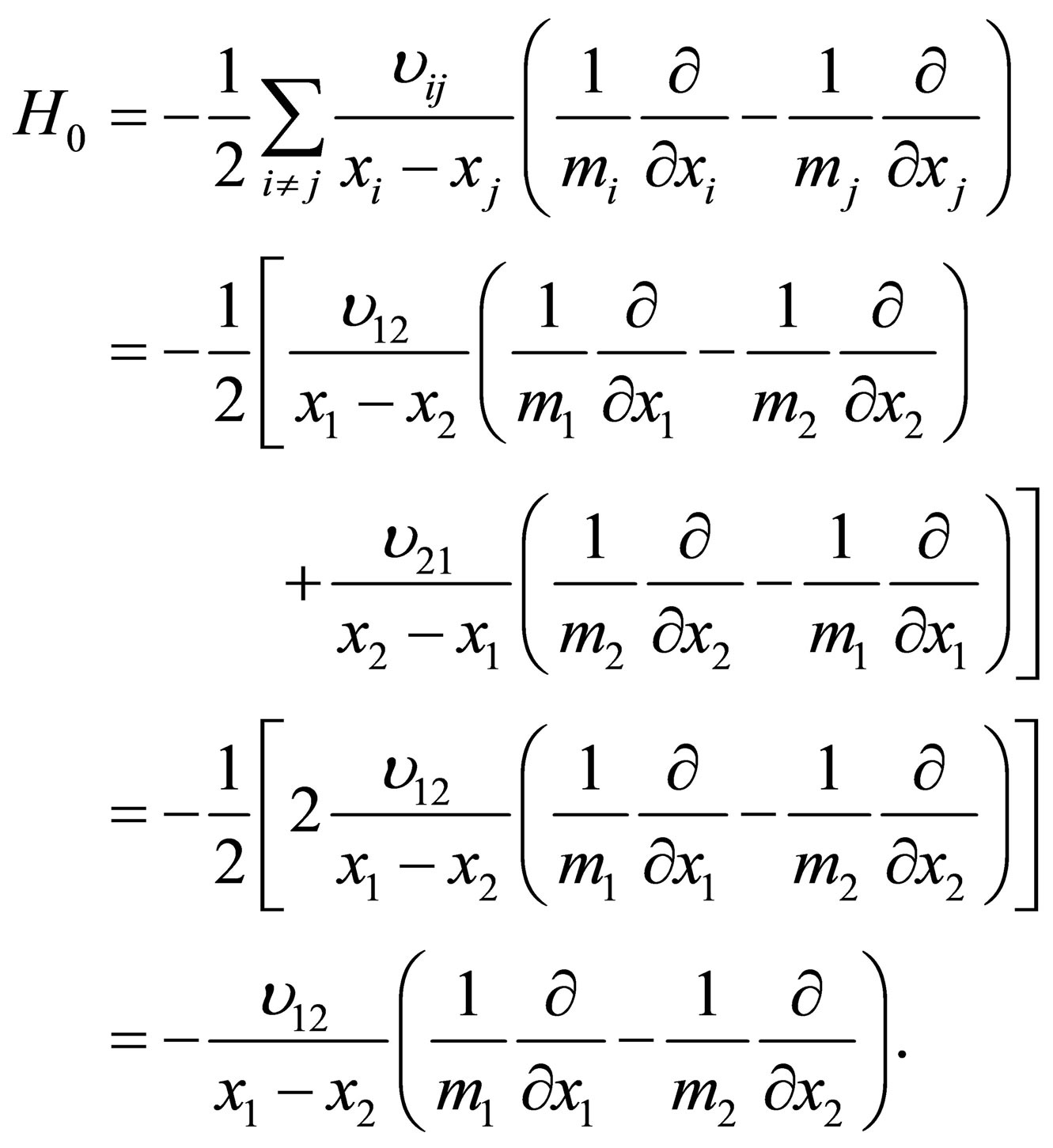 (9)
(9)
of the operator . As a consequence the monomial
. As a consequence the monomial 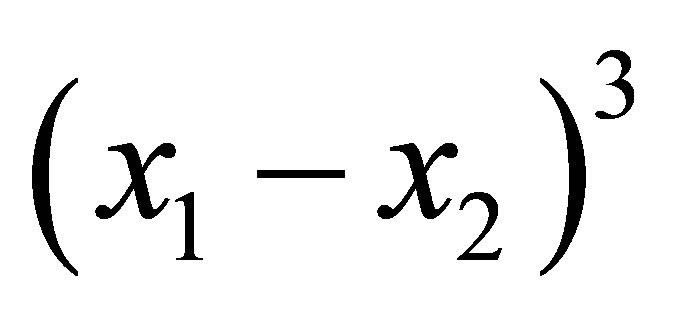 has to be discarded from P3 since
has to be discarded from P3 since 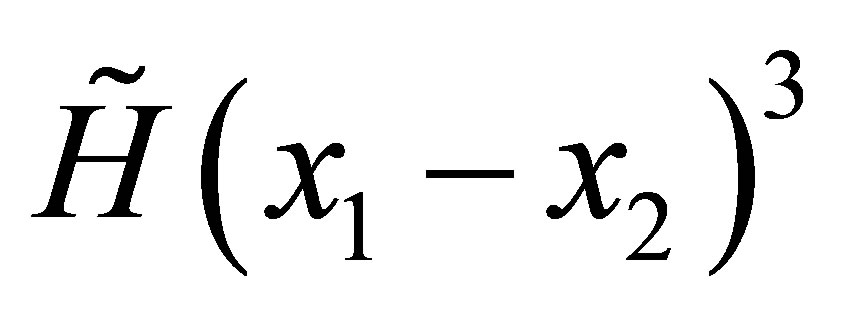 i.e, the following part of the operator
i.e, the following part of the operator 
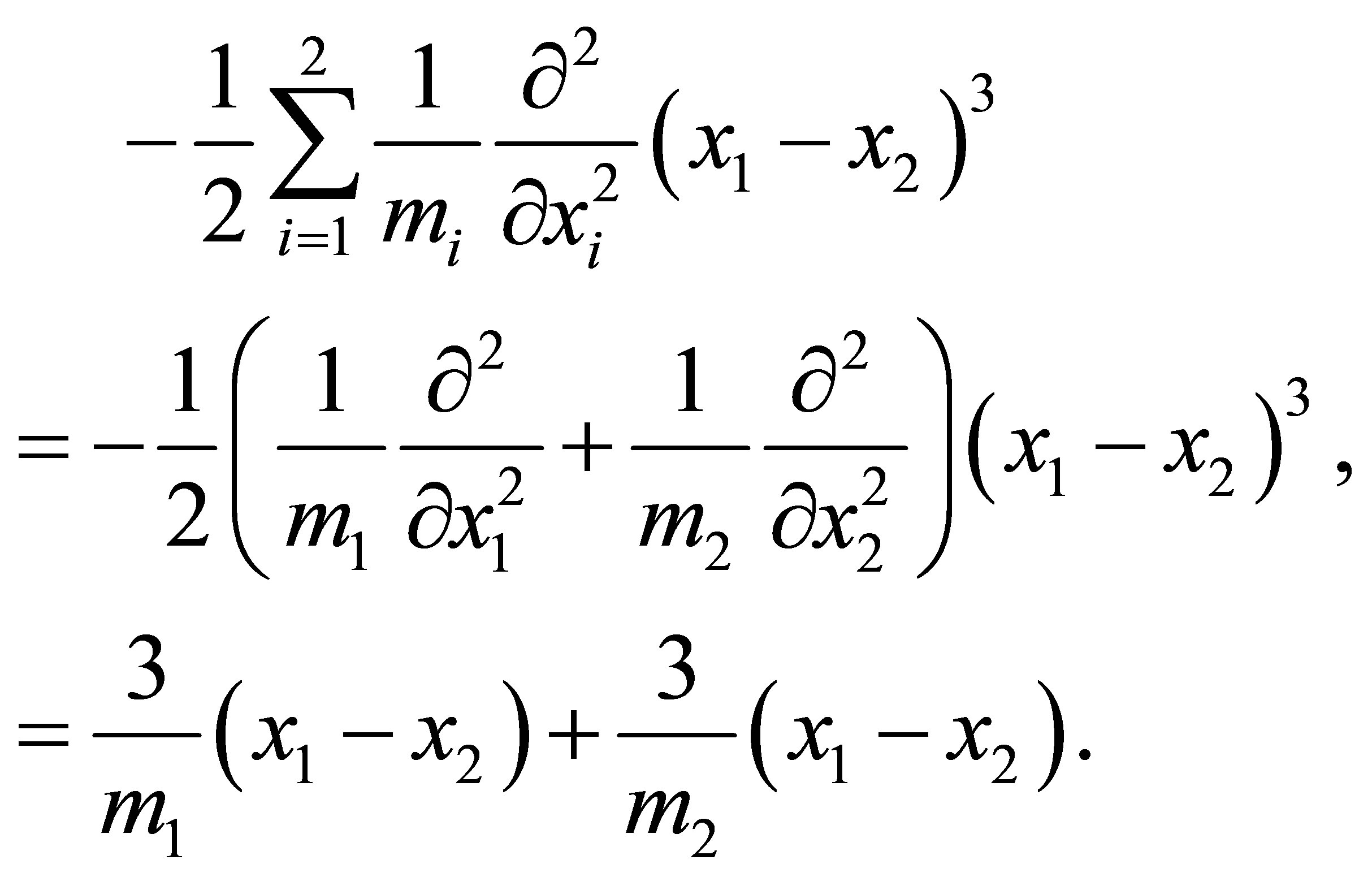 (10)
(10)
would naturally involve a term of the form 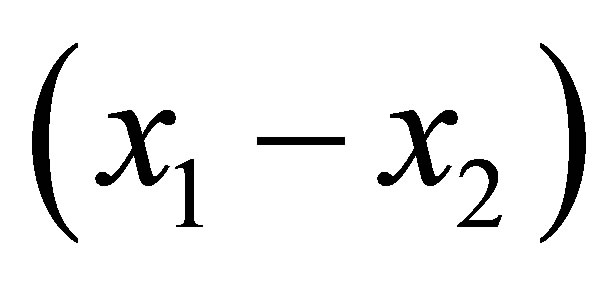 in the first order monomial which is excluded by the above argument (i.e.
in the first order monomial which is excluded by the above argument (i.e.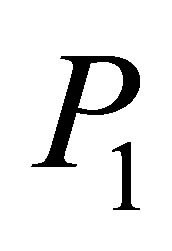 ). Proceeding along the same lines we conclude that the set of spaces
). Proceeding along the same lines we conclude that the set of spaces 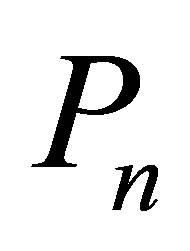 can be rephased in terms of the vector spaces
can be rephased in terms of the vector spaces 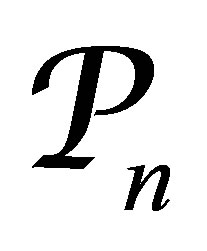 defined in
defined in  i.e.
i.e.
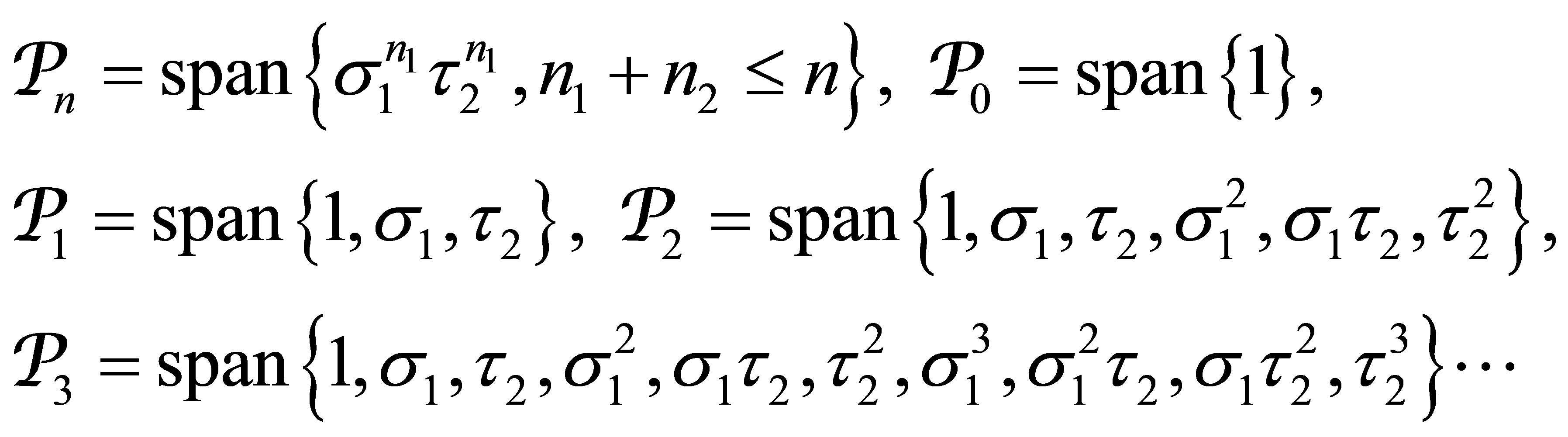
![]() (11)
(11)
with  is the center-of-mass coordinate and
is the center-of-mass coordinate and 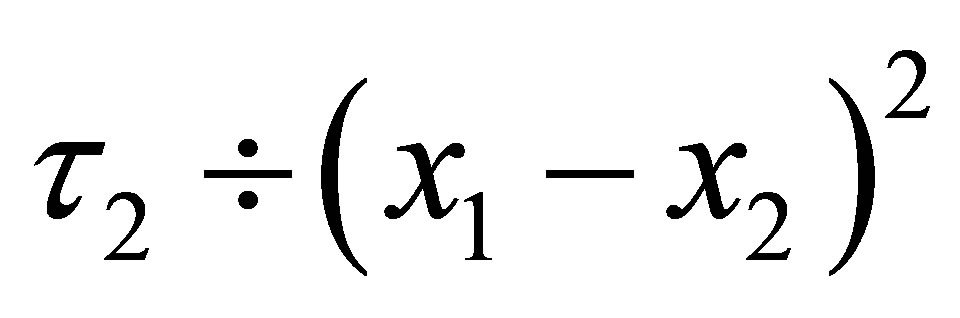 (12)
(12)
in this respect, the operator H (and then also ) is integrable and solvable for N = 2.
) is integrable and solvable for N = 2.
Notice that the space 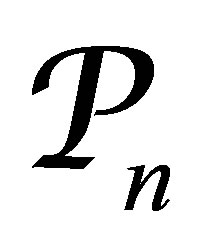 is equivalent to the ones considered by [6], apart from the fact that the variable
is equivalent to the ones considered by [6], apart from the fact that the variable 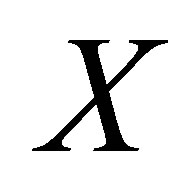 (the analogue of
(the analogue of ) is defined with the masses.
) is defined with the masses.
Let us now investigate the case N = 3. Again we can show that the following vector spaces are preserved by the operator ,
,
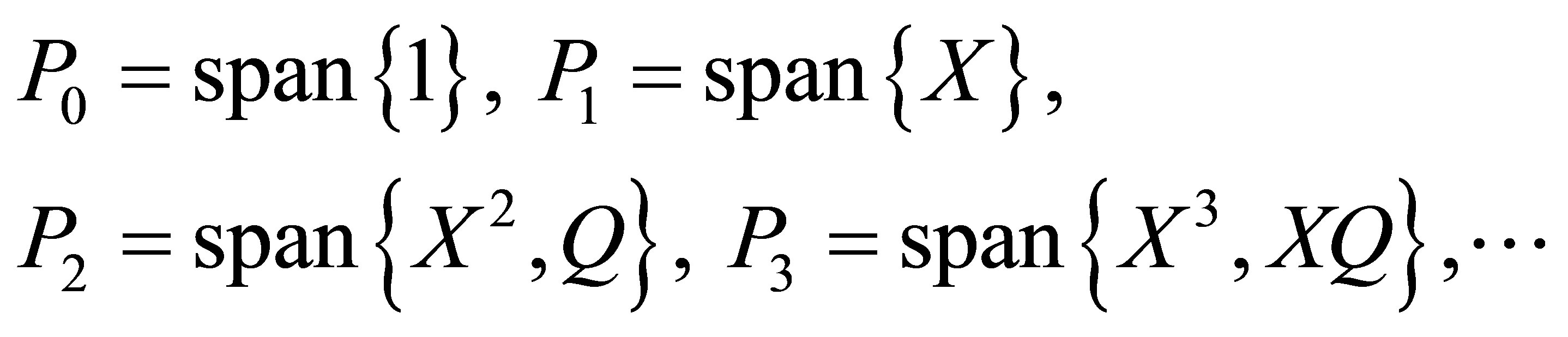 (13)
(13)
where
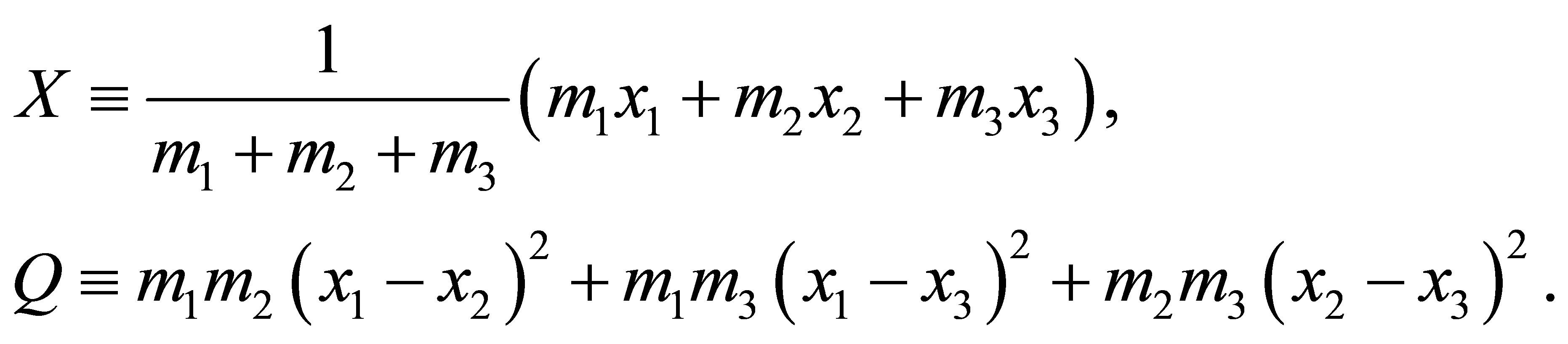 (14)
(14)
Note that 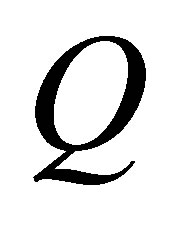 above is the generalization of the variable
above is the generalization of the variable 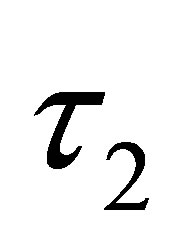 of [6]. However, it turns out to be impossible to construct a translation invariant-cubic polynomial of the form
of [6]. However, it turns out to be impossible to construct a translation invariant-cubic polynomial of the form
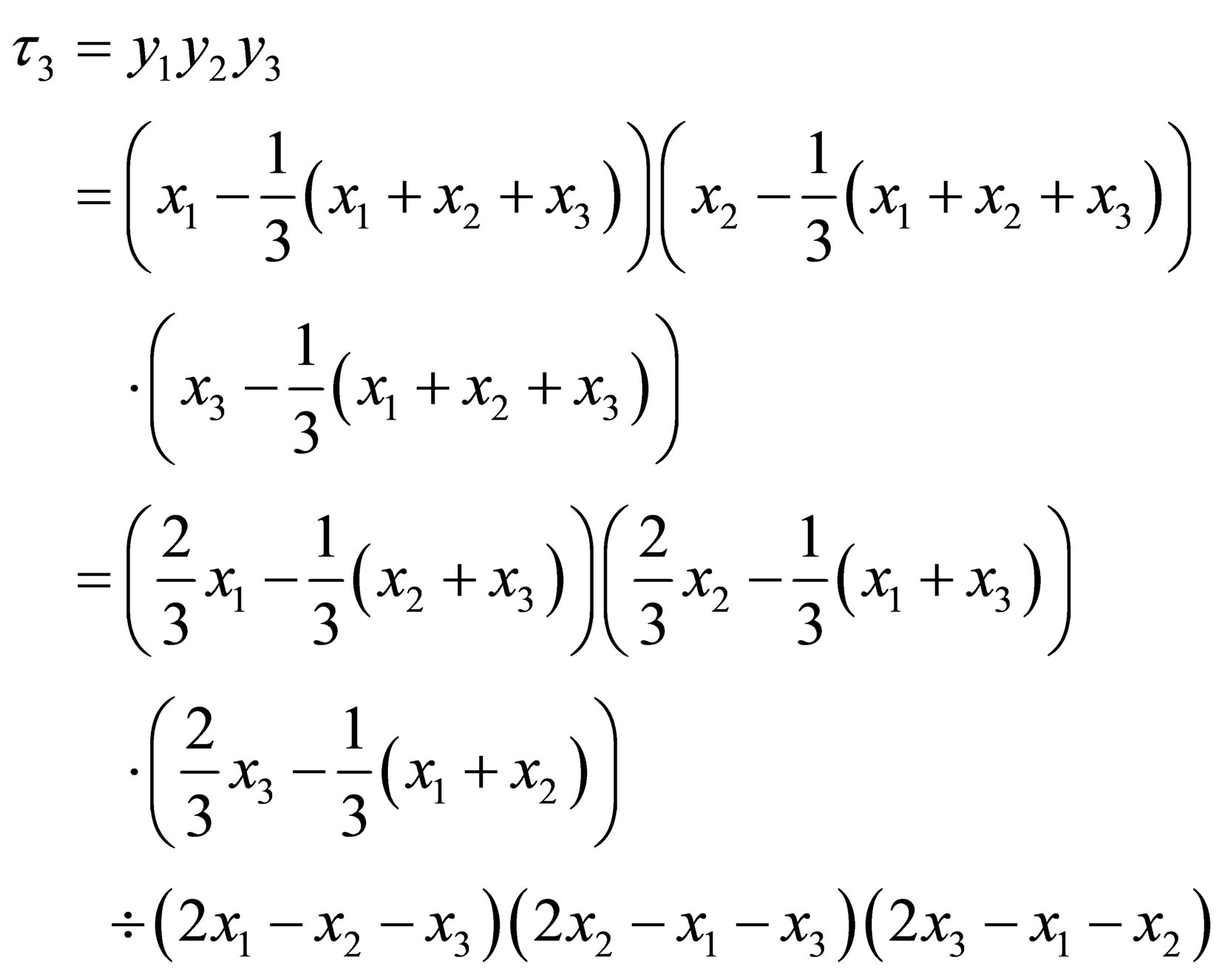 (15)
(15)
which is preserved by the operator 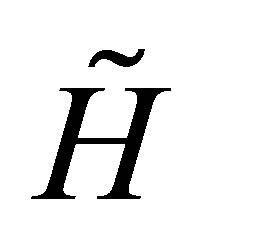 if the masses mi are generic (i.e
if the masses mi are generic (i.e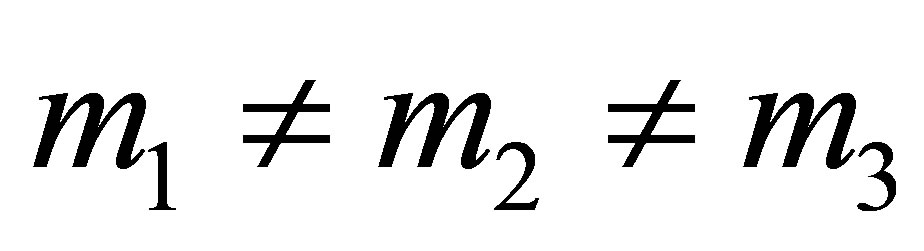 , etc). As a consequence, the dimension of the vector spaces of monomials preserved by
, etc). As a consequence, the dimension of the vector spaces of monomials preserved by 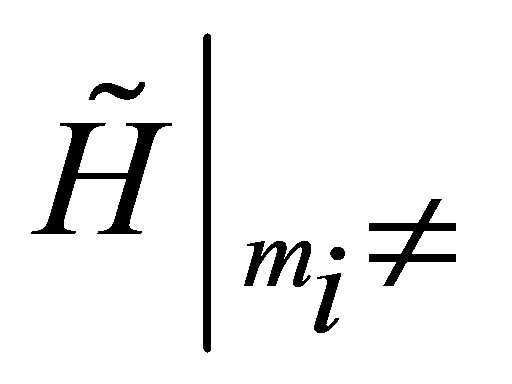 is lower than the vector spaces preserved by
is lower than the vector spaces preserved by 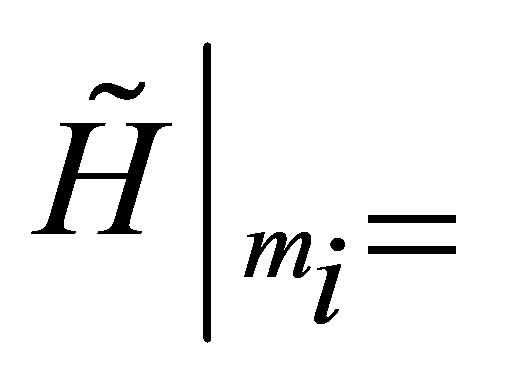 and the number of algebraic eigenvalues is lower than the usual Calogero case. In the next, this can be demonstrated easily in the particular case N = 2.
and the number of algebraic eigenvalues is lower than the usual Calogero case. In the next, this can be demonstrated easily in the particular case N = 2.
2.1. Eigenvalues for the Case with Equal Masses
We use the operator
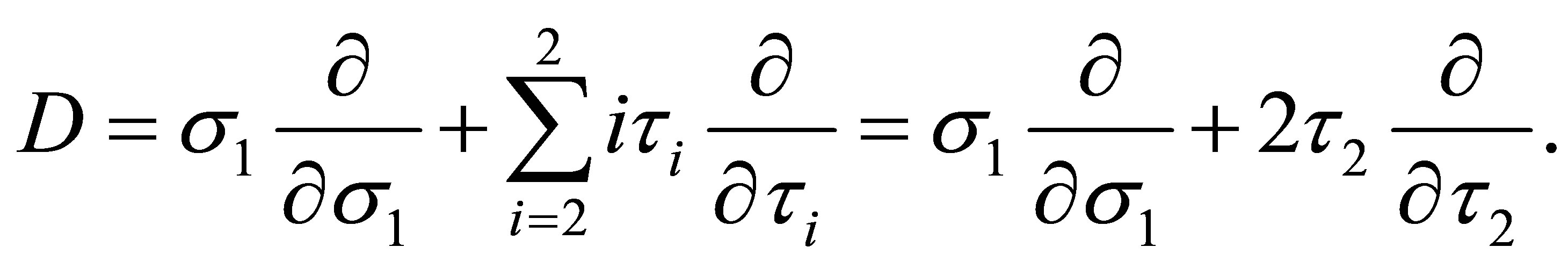 (16)
(16)
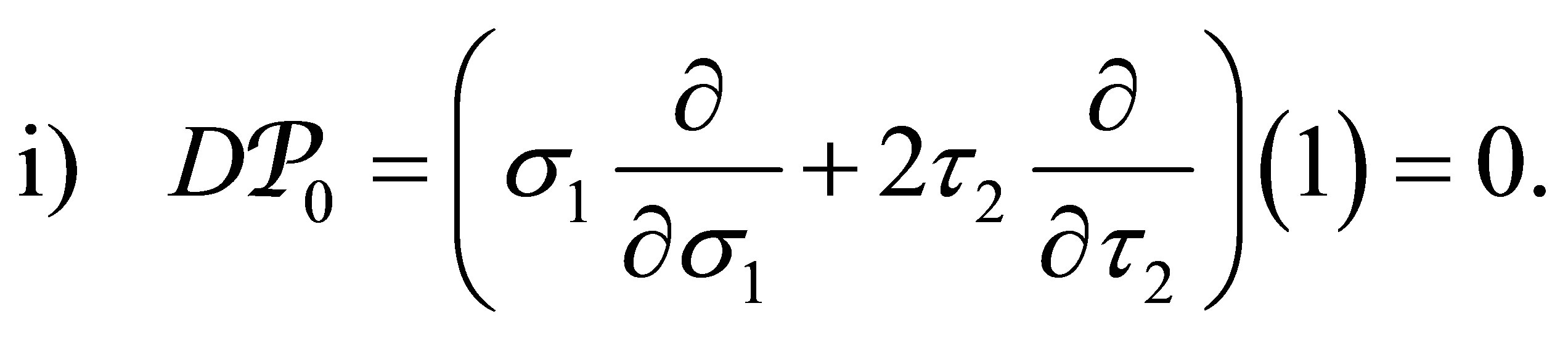 (17)
(17)
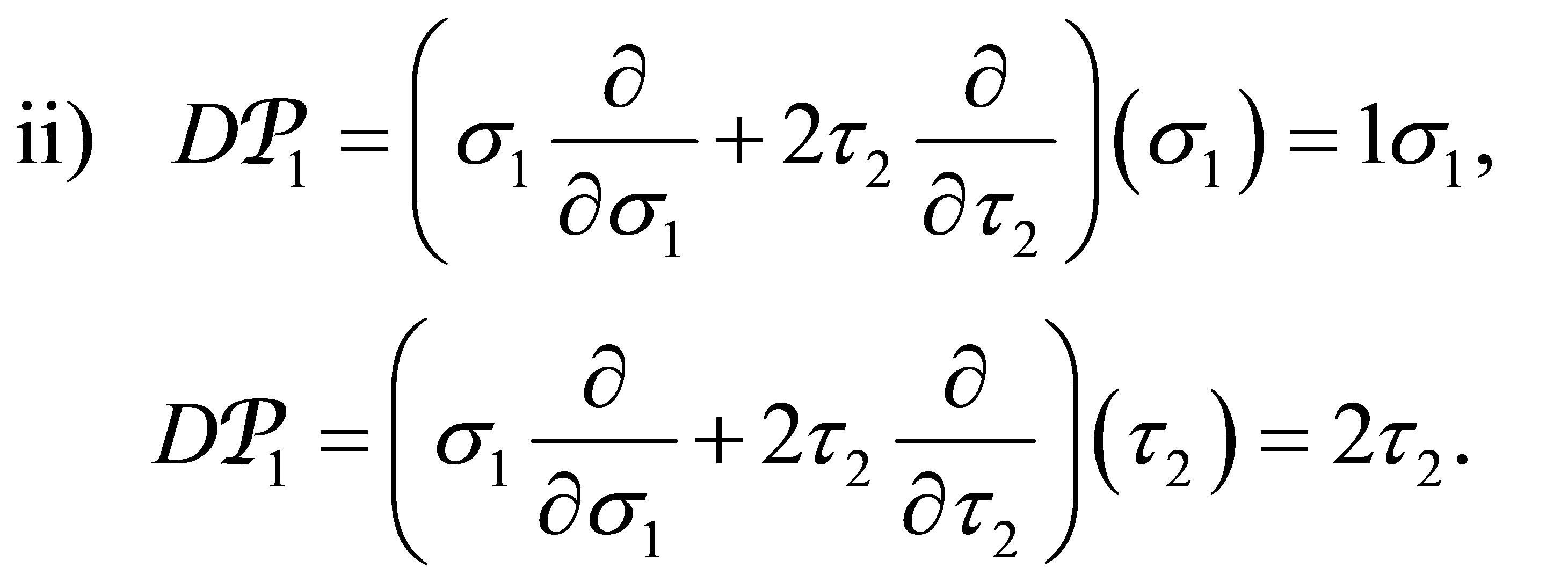 (18)
(18)
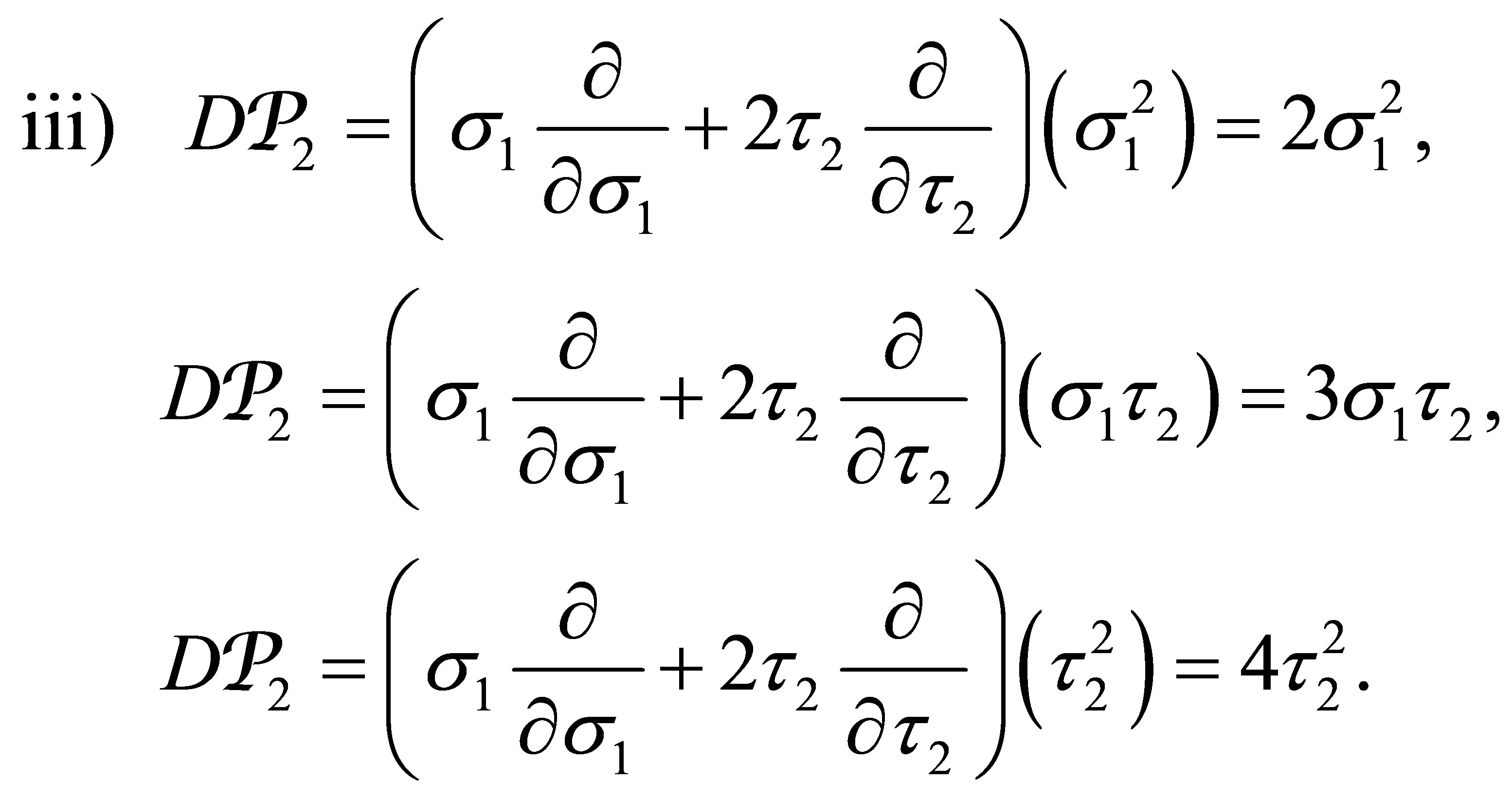 (19)
(19)
The spectrum for the above case is 0;
1, 2;
2, 3, 4.
2.2. Eigenvalues for the Case with Different Masses
In this case we apply the some procedure used in the previous case (i.e. we consider also N = 2) but the operator D has the following form
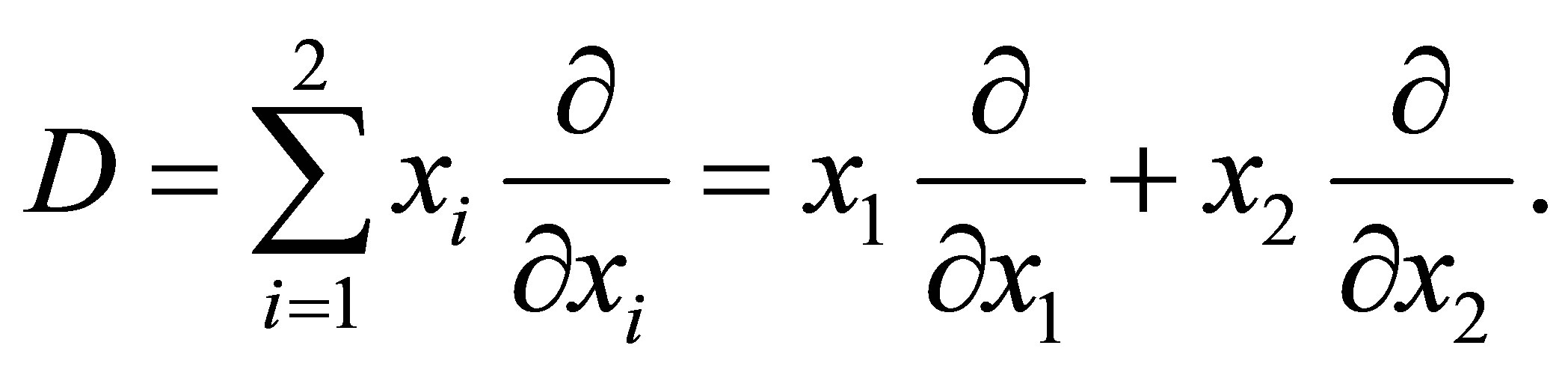 (20)
(20)
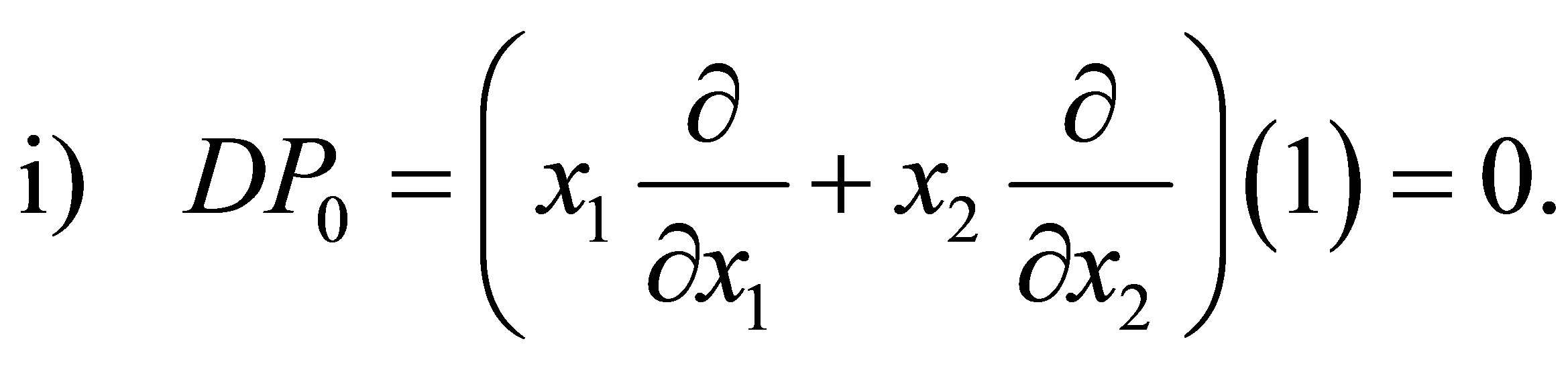 (21)
(21)
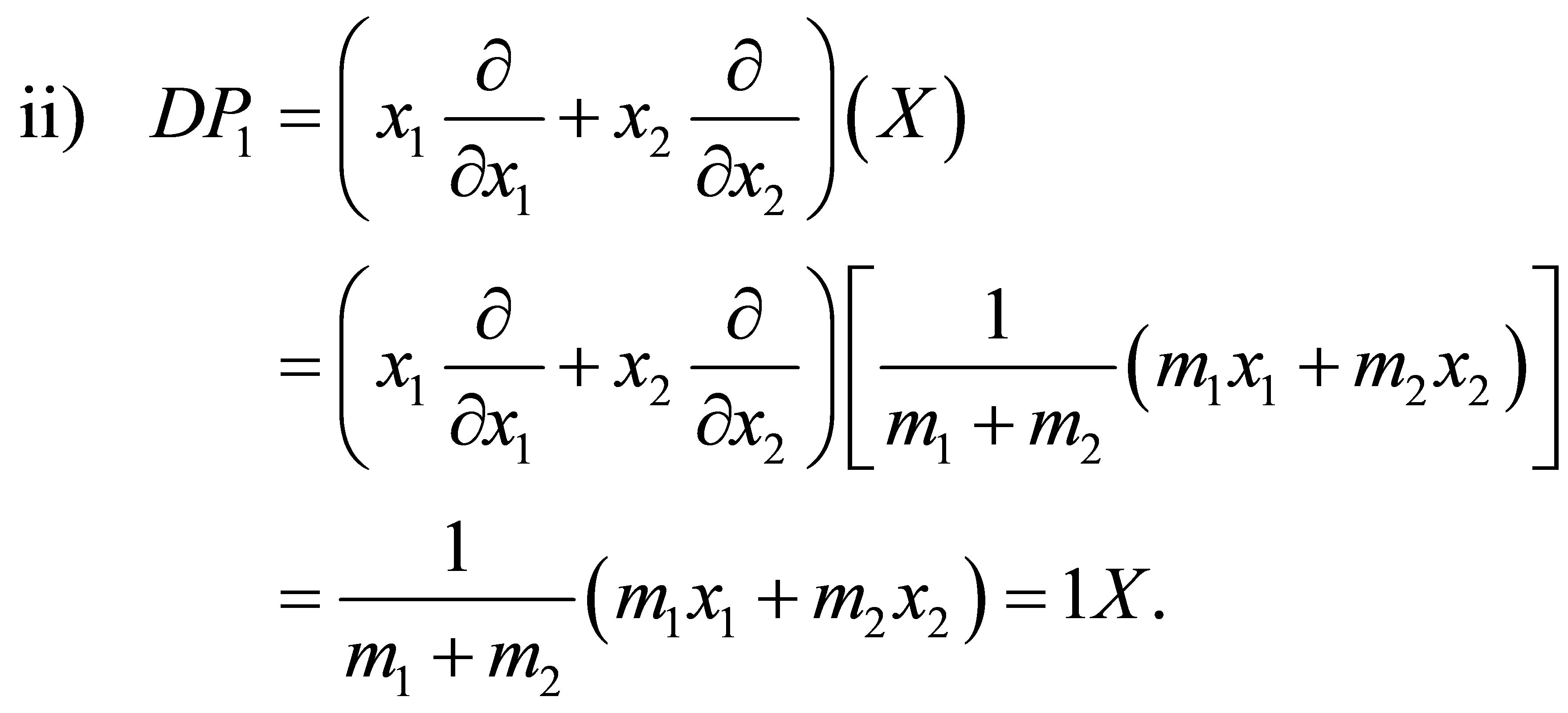 (22)
(22)
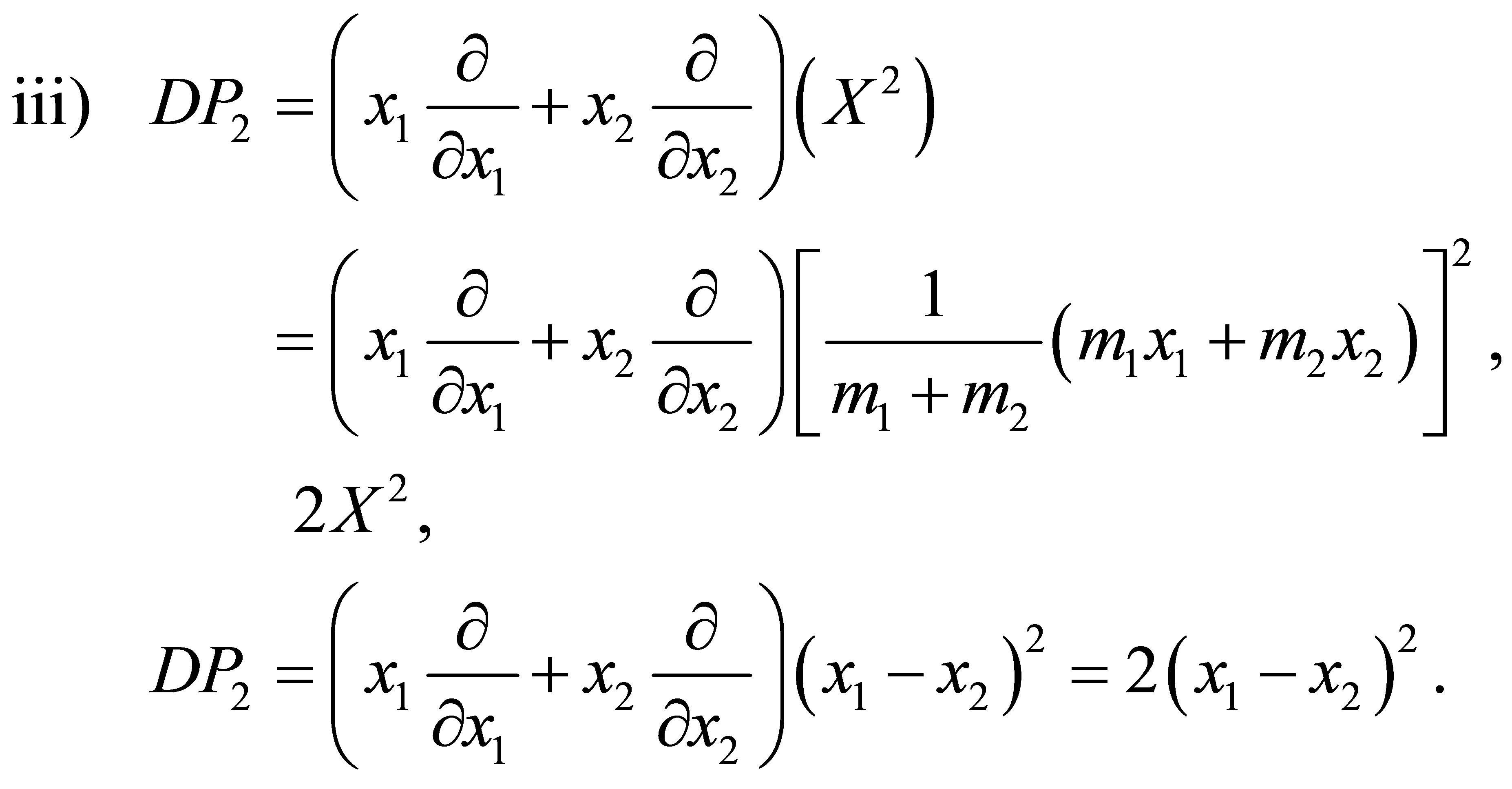 (23)
(23)
The spectrum for the above case is 0;
1;
2, 2.
More generally, the vector spaces preserved by  are of the form
are of the form
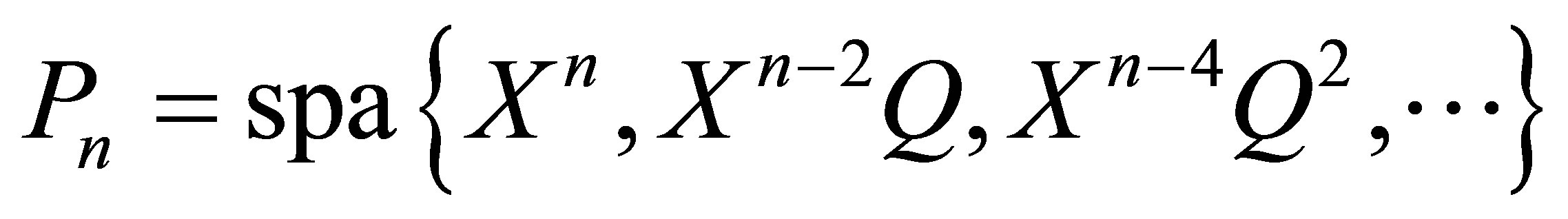 (24)
(24)
and any eigenvector of  can be written according to
can be written according to
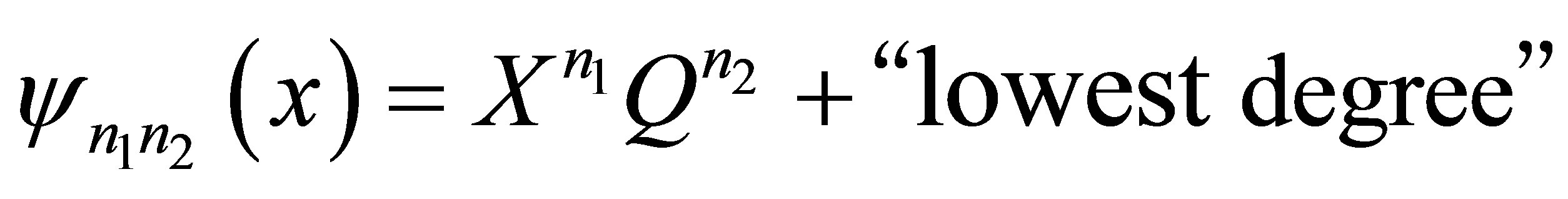 (25)
(25)
while the corresponding eigenvalues are given by
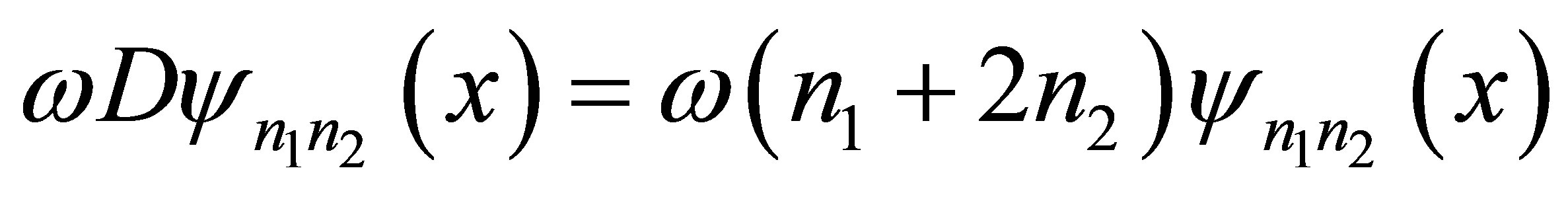 (26)
(26)
so that the spectrum of  consists of integers of the form
consists of integers of the form
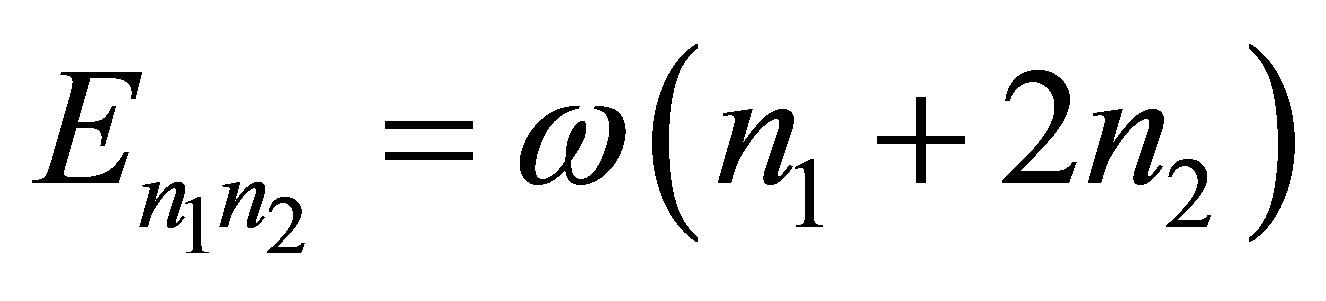 (27)
(27)
as generic in [5]. In this way, we have redemonstrated the result of these authors by following the algebraic technique of operators preserving spaces of monomials as suggested by [6].
We have attempted to construct invariant spaces of polynomials involving the monomials
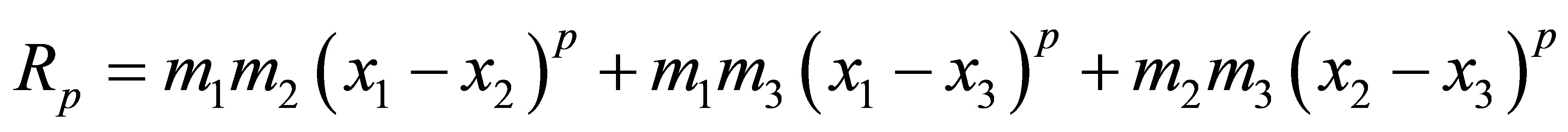 (28)
(28)
with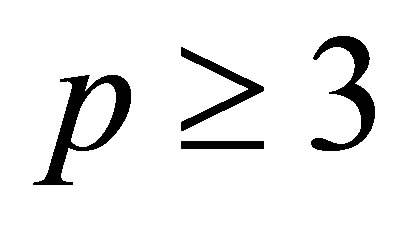 . These polynomials are indeed such that
. These polynomials are indeed such that 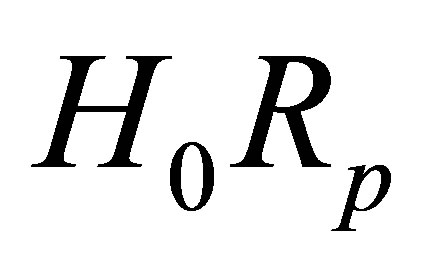 is a polynomial but the new polynomials
is a polynomial but the new polynomials 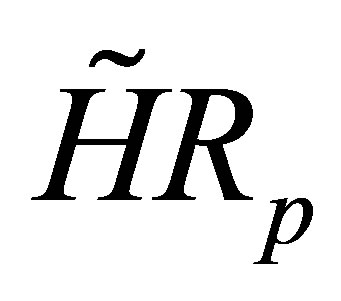 are not in general expressible as polynomials of the two variables X and
are not in general expressible as polynomials of the two variables X and 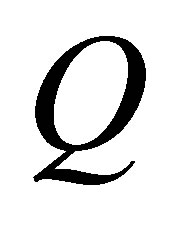 (i.e.
(i.e.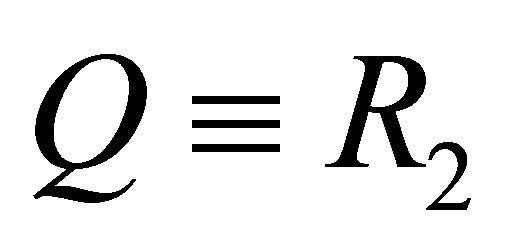 ). More generally, the polynomials for
). More generally, the polynomials for 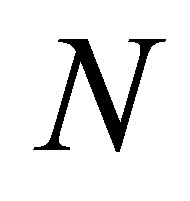 body can be written as follows
body can be written as follows
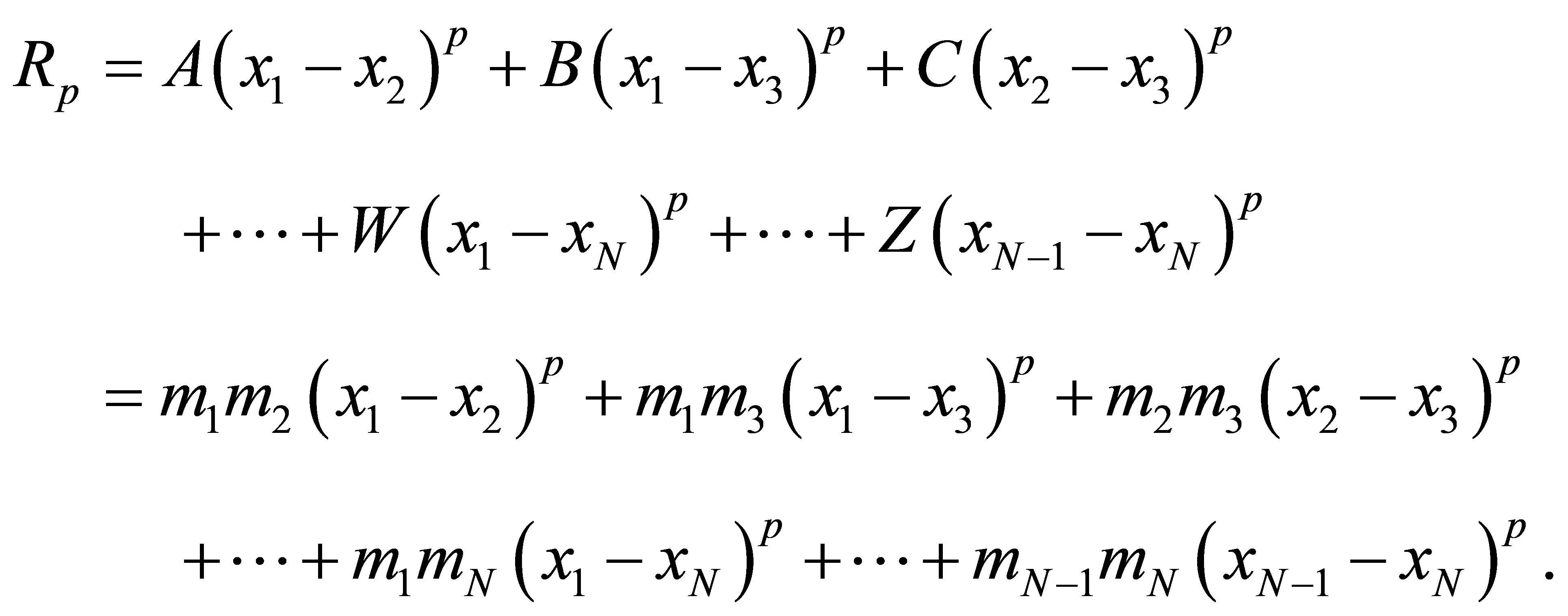 (29)
(29)
3. Conclusion
Here we have constructed the operator  which preserves some spaces of polynomials and compared with the invariant spaces available in the usual Calogero model (
which preserves some spaces of polynomials and compared with the invariant spaces available in the usual Calogero model (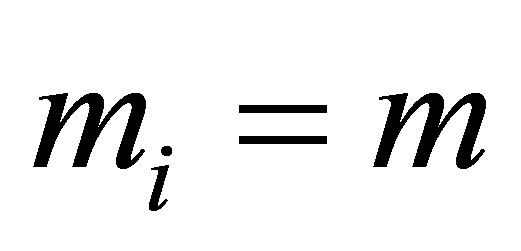 i.e. the masses are equal). We have determined the real spectrum for the case with different masses and for the case for equal masses where
i.e. the masses are equal). We have determined the real spectrum for the case with different masses and for the case for equal masses where 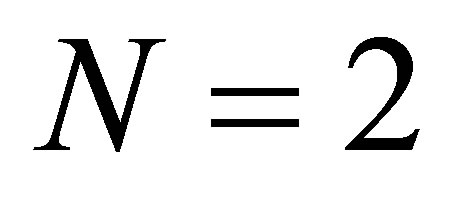 i.e. two body case. This extended Calogero model exhibits some remarkable properties which are absent in the case of usual Calogero model. For example, the number of eigenvalues in the case with different masses is lower than one of eigenvalues of the usual Calogero model.
i.e. two body case. This extended Calogero model exhibits some remarkable properties which are absent in the case of usual Calogero model. For example, the number of eigenvalues in the case with different masses is lower than one of eigenvalues of the usual Calogero model.
4. Acknowledgements
I thank Pr. Yves Brihaye for useful discussions
REFERENCES
- F. Calogero, “Solution of a Three-Body Problem in One Dimension,” Journal of Mathematical Physics, Vol. 10, No. 12, 1969, pp. 2191-2197. doi:10.1063/1.1664820
- F. Calogero, “Solution of the One-Dimensional N-Body Problems with Quadratic and/or Inversely Quadratic Pair Potentials,” Journal of Mathematical Physics, Vol. 12, No. 3, 1971, p. 419. doi:10.1063/1.1665604
- M. A. Olshanetsky and A. M. Perelomov, “Classical Integrable Finite-Dimensional Systems Related to Lie Algebras,” Physics Reports, Vol. 71, No. 5, 1981, pp. 313-400. doi:10.1016/0370-1573(81)90023-5
- V. Bardek, L. Jonke, S. Meljanac and M. Milchovic, “Calogero Model, Deformed Oscillators and the Collapse,” Physical Letters, Vol. B531, No. 3-4, 2002, pp. 311-315. doi:10.1016/S0370-2693(02)01481-8
- S. Meljanac, M. Milekovic and A. Samsarov, “A Multispecies Calogero Model,” Physical Letters, Vol. B573, 2003, pp. 202-208. doi:10.1016/j.physletb.2003.08.029
- W. Ruhl and A. Turbiner, “Exact Solvability of the Calogero and Sutherland Models,” Modern Physics Letters A, Vol. 10, No. 29, 1995, p. 2213. doi:10.1142/S0217732395002374
- A. Turbiner, “Quasi-Exactly-Solvable Problems and sl(2) Algebra,” Communications in Mathematical Physics, Vol. 118, No. 3, 1988, pp. 467-474. doi:10.1007/BF01466727
- Y. Brihaye and A. Nininahazwe, “On Pt-Symmetric Extensions of the Calogero and Sutherland Models,” International Journal of Modern Physics A, Vol. 19, No. 26, 2004, pp. 4391-4400. doi:10.1142/S0217751X04019858

