Open Journal of Discrete Mathematics
Vol.3 No.1(2013), Article ID:27363,9 pages DOI:10.4236/ojdm.2013.31002
Counting Types of Runs in Classes of Arborescent Words
1Mathematics Institute, Free University of Berlin, Berlin, Germany
2University of Quebec at Montreal, Montreal City, Canada
Email: labbe@math.fu-berlin.de, labelle.gilbert@uqam.ca
Received November 16, 2012; revised December 16, 2012; accepted December 25, 2012
Keywords: Runs; Structured Words; Treelike Structures; Combinatorial Species; Generating Series; Cycle Index Series
ABSTRACT
An arborescence is a directed rooted tree in which all edges point away from the root. An arborescent word is obtained by replacing each element of the underlying set of an arborescence by an arbitrary letter of a given alphabet (with possible repetitions). We define a run in an arborescent word as a maximal sub-arborescent word whose letters are all identical. Various types of runs (e.g., runs of size ≤ k, linear runs, etc.) are studied in the context of R-enriched arborescent words, where R is a given species of structures.
1. Introduction
Classically, a word over an alphabet Ω is a finite sequence  of letters taken from Ω and a run in a word
of letters taken from Ω and a run in a word 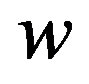 is a maximal subsequence of consecutive identical letters in
is a maximal subsequence of consecutive identical letters in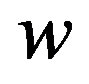 , see, for example, Feller [1], or Mood [2]. A slightly different point of view consists of interpreting a word over Ω as the result of the replacement of each element of a linear digraph by an arbitrary letter of Ω. A run in a word is then interpreted as a maximal linear sub-digraph whose letters are all identical. Here are four runs,
, see, for example, Feller [1], or Mood [2]. A slightly different point of view consists of interpreting a word over Ω as the result of the replacement of each element of a linear digraph by an arbitrary letter of Ω. A run in a word is then interpreted as a maximal linear sub-digraph whose letters are all identical. Here are four runs,  , in a 9-letter word over the alphabet
, in a 9-letter word over the alphabet ) obtained under this interpretation (see below).
) obtained under this interpretation (see below).
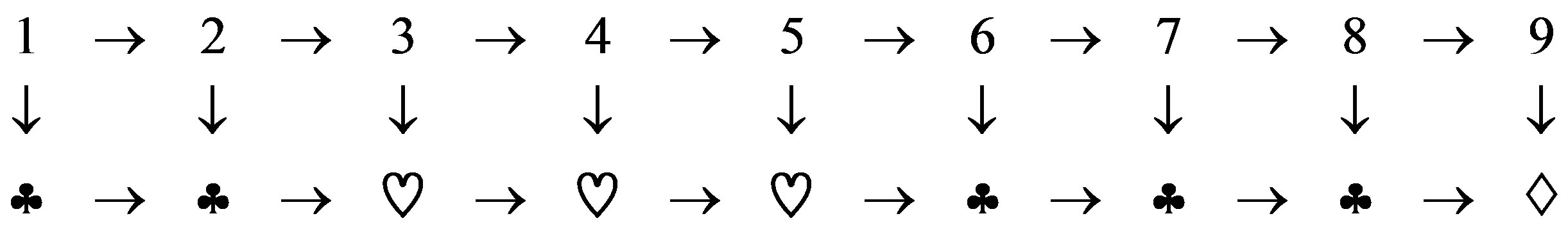 (1)
(1)
Using this point of view, words can now be easily “structured” in various ways. For example, starting from the digraph of a circular permutation (instead of a linear digraph, as above) gives rize to the concept of a circular word studied probabilistically in the basic paper of Koutras et al. [3]. In a similar way, any combinatorial species F, in the sense of Joyal [4], leads to a corresponding notion of F-structured word (or F-word, for short). Technically speaking, a F-word of length n over an alphabet Ω is an orbit of the following natural action of the symmetric group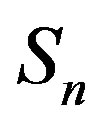 ,
,
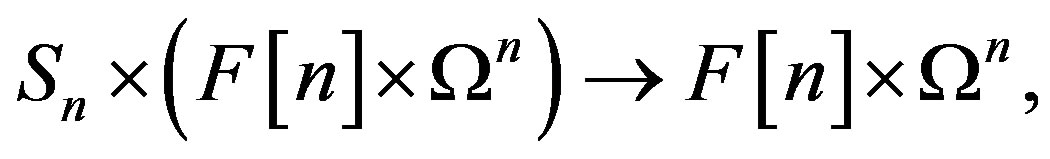 (2)
(2)
defined by
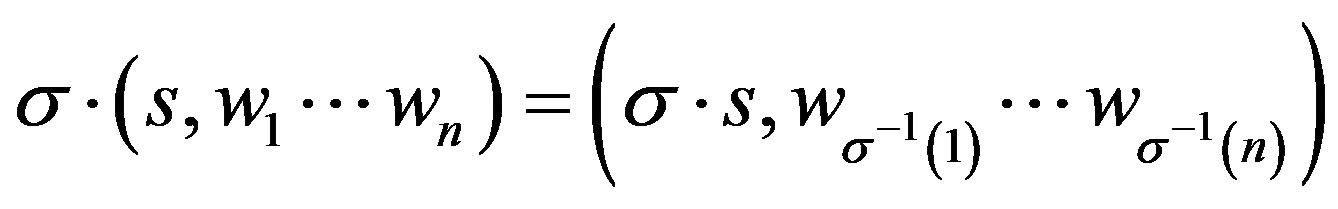 where
where 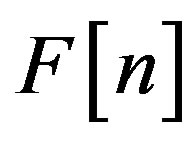 is the set of F-structures on
is the set of F-structures on
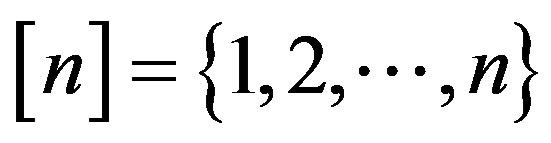 ,
,
 is the result of relabelling the
is the result of relabelling the  -structure
-structure 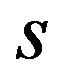 along the permutation
along the permutation , and
, and 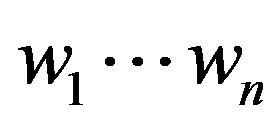 is an ordinary word of length
is an ordinary word of length 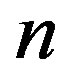 over the alphabet Ω. Figure 1 illustrates this action for
over the alphabet Ω. Figure 1 illustrates this action for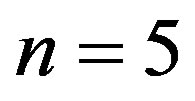 ,
, 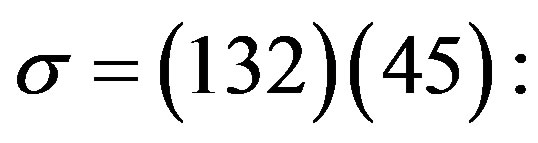 the left drawing shows the ordered pair
the left drawing shows the ordered pair 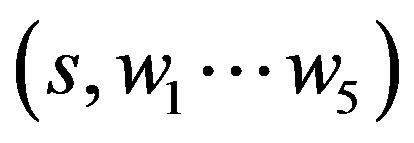 where
where  is a graph on [5] and
is a graph on [5] and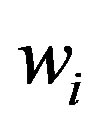 , the
, the 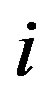 -th letter of the word
-th letter of the word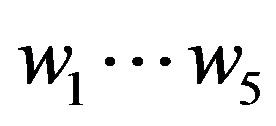 , is written just under its associated vertex
, is written just under its associated vertex 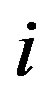 in the graph, for
in the graph, for ; while the right drawing shows the resulting
; while the right drawing shows the resulting 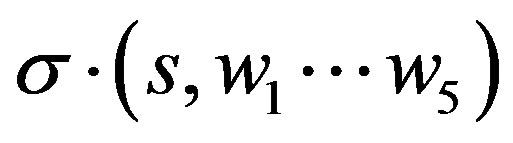 under the action of
under the action of .
.
It is important to note that under this action, the labels 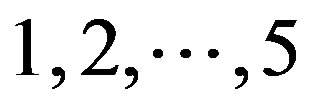 of the underlying set of the structure
of the underlying set of the structure 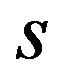 are permuted while each letter is kept in a fixed position. This is a general fact and it constitutes the reason why the orbits of action (2) can be though of as unlabelled F-structures whose nodes are replaced (or filled) by letters of the alphabet, that is, F-words. Summing the orbits of (2) over
are permuted while each letter is kept in a fixed position. This is a general fact and it constitutes the reason why the orbits of action (2) can be though of as unlabelled F-structures whose nodes are replaced (or filled) by letters of the alphabet, that is, F-words. Summing the orbits of (2) over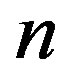 , gives rize to the concept of an analytic functor in the sense of Joyal [5],
, gives rize to the concept of an analytic functor in the sense of Joyal [5],
| |
 of the category of sets into itself, sending each set Ω (= alphabet) to the set of all F-words over Ω.
of the category of sets into itself, sending each set Ω (= alphabet) to the set of all F-words over Ω.
There exist special classes of F-words, for which a natural notion of “run” can be defined. This is the case when F is a graphical species, whose structures are of the form of a simple graph possibly equipped with extra structures. Standard examples of graphical species include: the species of digraphs, of circular permutations, of tree-like structures (e.g., when F is the species of 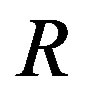 -enriched rooted trees or the species of R-enriched trees in the sense of Labelle [6]), etc.
-enriched rooted trees or the species of R-enriched trees in the sense of Labelle [6]), etc.
Definition 1 Let F be a graphical species and 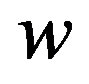 be a F-word over an alphabet Ω. A run in
be a F-word over an alphabet Ω. A run in 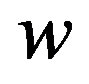 is a maximal connected subgraph of
is a maximal connected subgraph of 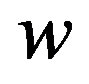 having identical letters.
having identical letters.
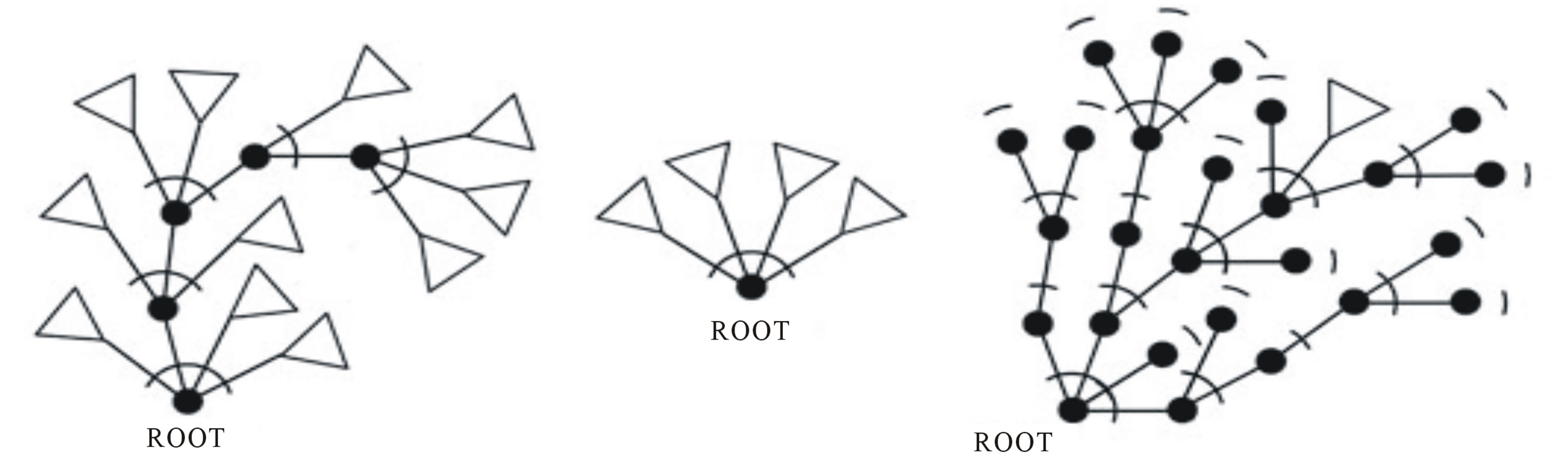
This definition makes sense since the relabellings of F-structures under the action (2) is letter-invariant. Figure 2 shows the runs in a circular word and in a tree-like word over the alphabet .
.
The study of runs in graphical words appears far from being trivial, under such a general setting. For example, a simple graph word whose runs are all singletons is equivalent to a proper coloring of the graph. So that, even in this special case, the whole theory of chromatic polynomials must be used. In the present text, our run analysis will be concentrated on classes of arborescent words.
In Section 2, we study various types of runs in Fwords over an alphabet of k letters, where F is the graphical species of R-enriched rooted trees, called here R-arborescences, for short. To do so, we make use of functional equations on multisort species and introduce a special 2-sort species, that we call a runs selector species, to classify the types of runs under study. Various enu-

Figure 1. The pairs 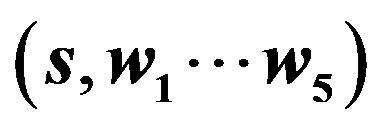 and
and 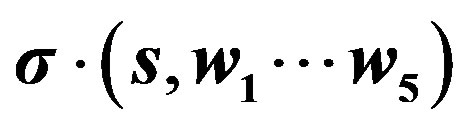 under the action of
under the action of .
.
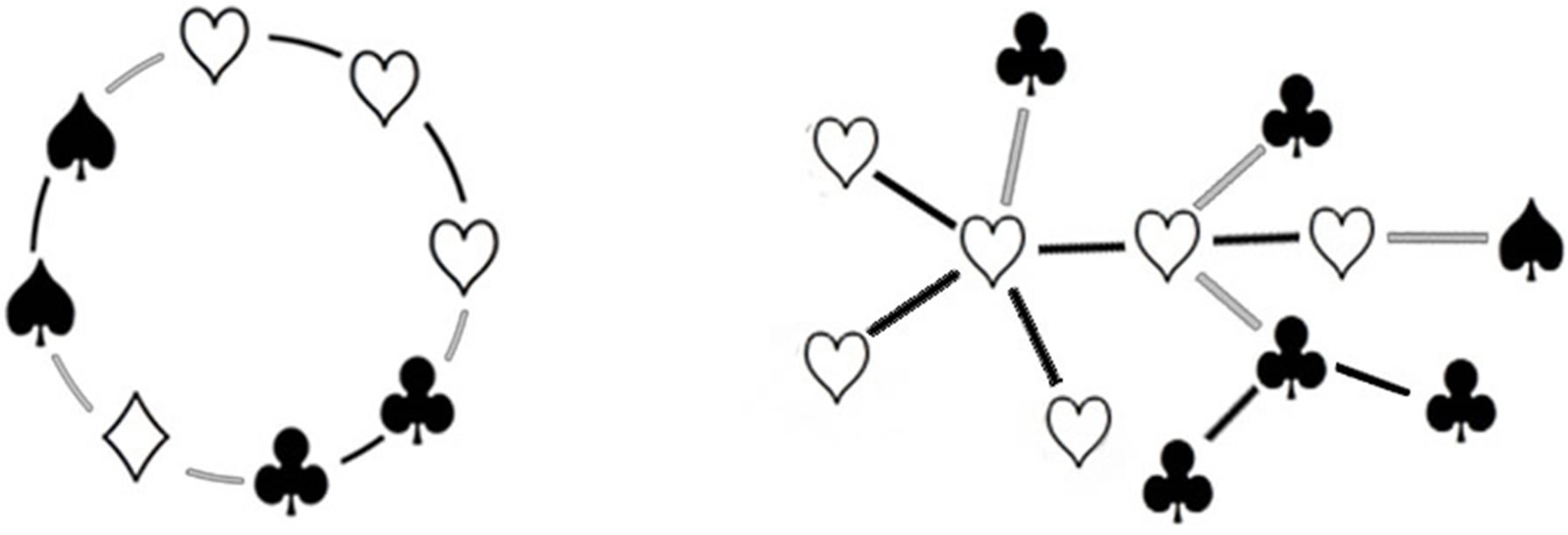
Figure 2. Four runs in a circular word and five runs in a tree-like word.
merative results about runs (e.g., number of F-words whose runs are all of a given type, distribution of the biggest run, etc) then follow by making use of appropriate underlying cycle index series. Section 3 illustrates the theory of R-arborescent words by analysing specific examples and their associated series. Section 4 indicates some extensions to be developed in the future. For an introduction to species, the reader can consult the basic paper of Joyal [4] or the book by Bergeron, Labelle, and Leroux [7].
2. Runs in Enriched Arborescent Words
An arborescence is a directed rooted tree in which all edges point away from the root. Let 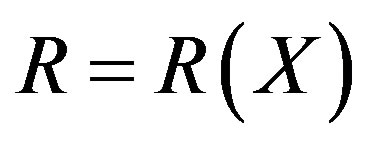 be a given species of structures having at least one structure on the empty set. Recall that an
be a given species of structures having at least one structure on the empty set. Recall that an  -arborescence is an arborescence in which the (possibly empty) set of direct successors of each vertex is equipped with an
-arborescence is an arborescence in which the (possibly empty) set of direct successors of each vertex is equipped with an  - structure. Figure 3(a) shows an
- structure. Figure 3(a) shows an 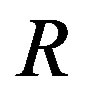 -arborescence, where the black dots represent the elements of its underlying set. The small arc at each vertex represents the
-arborescence, where the black dots represent the elements of its underlying set. The small arc at each vertex represents the 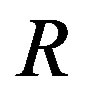 -structure placed on the set of successors of the vertex. The species
-structure placed on the set of successors of the vertex. The species 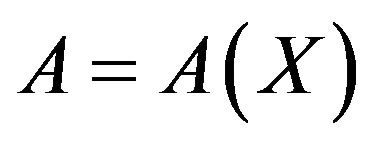 of
of 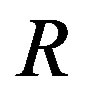 -arborescences is characterized by the combinatorial equation,
-arborescences is characterized by the combinatorial equation,
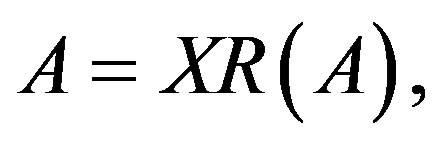 (3)
(3)
and, depending on the choice of the “enriching” species, 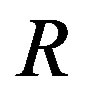 , various species of tree-like structures are obtained. Now, take a
, various species of tree-like structures are obtained. Now, take a  -letter alphabet
-letter alphabet 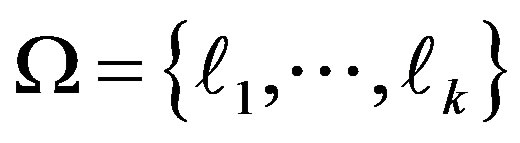 and associate a sort
and associate a sort 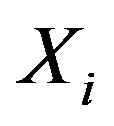 of singletons to letter
of singletons to letter 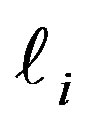 , for
, for 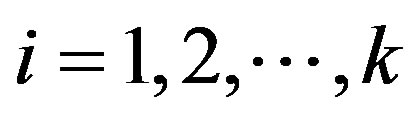 . The structures belonging to the
. The structures belonging to the  -sort species
-sort species 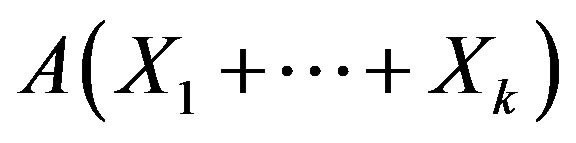 are
are 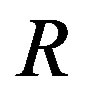 -arborescences whose vertices are of arbitrary sorts taken among the
-arborescences whose vertices are of arbitrary sorts taken among the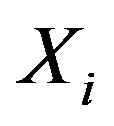 ’s. The first step in our run analysis is to classify these multisort structures according the runs of sorts they contain. From (3), we obviously have,
’s. The first step in our run analysis is to classify these multisort structures according the runs of sorts they contain. From (3), we obviously have,
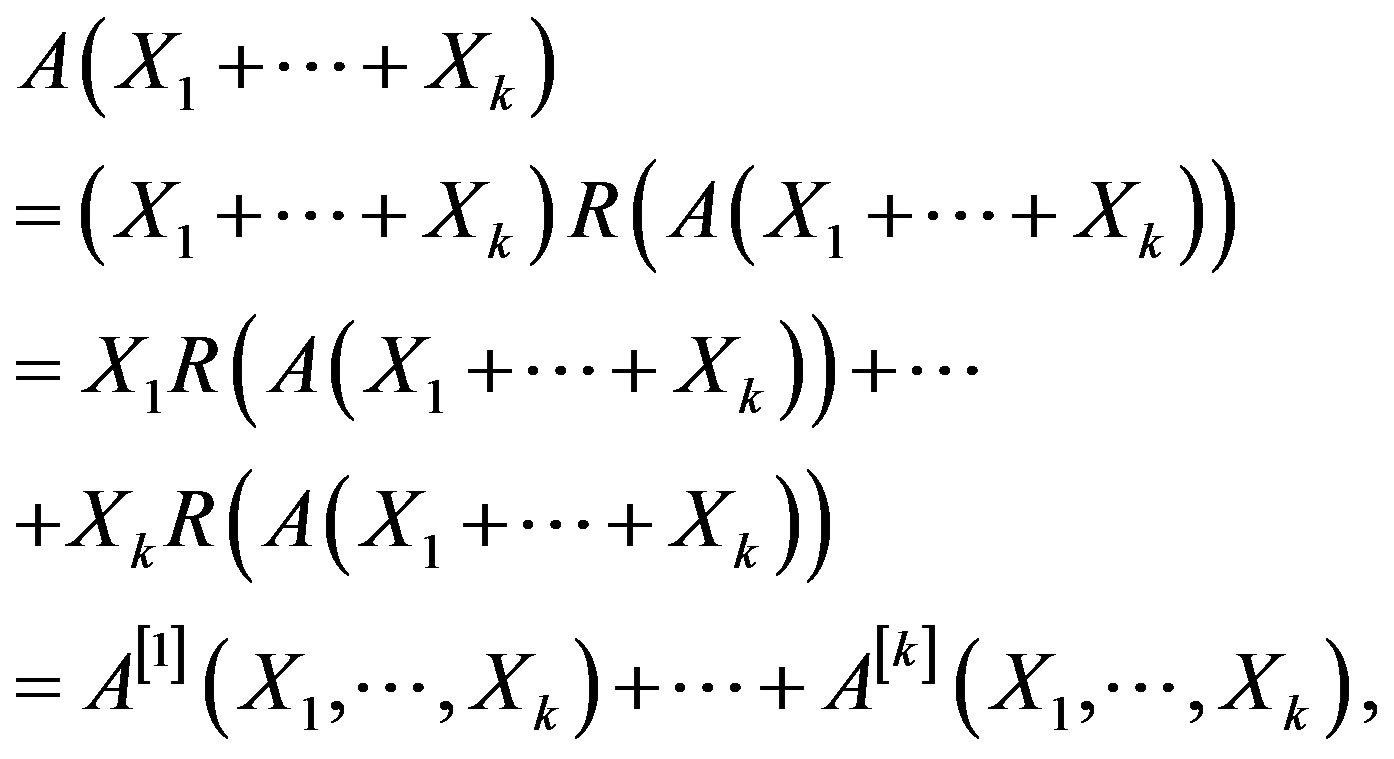 (4)
(4)
where 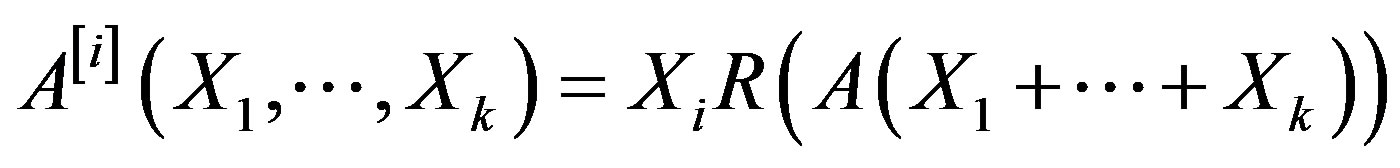 is the species of the
is the species of the  -structures having a root of sort
-structures having a root of sort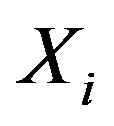 . Note that this root of sort
. Note that this root of sort  is part of a run of vertices of sort
is part of a run of vertices of sort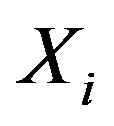 . To reflect this fact, we now give an alternate expression for
. To reflect this fact, we now give an alternate expression for 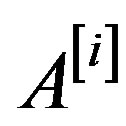 in terms of an auxiliary two-sort species,
in terms of an auxiliary two-sort species, 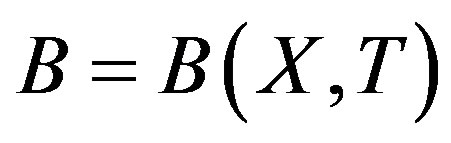 , where
, where 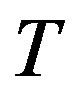 is an extra sort of singletons.
is an extra sort of singletons.
Lemma 2 Let 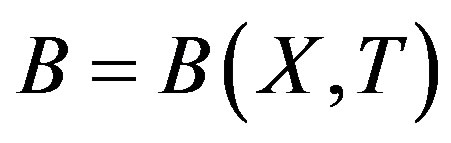 be the two-sort species defined by
be the two-sort species defined by
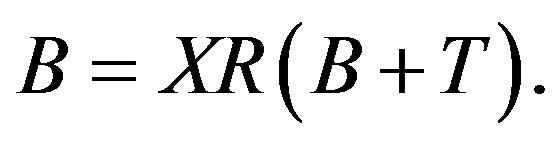 (5)
(5)
Then, the species 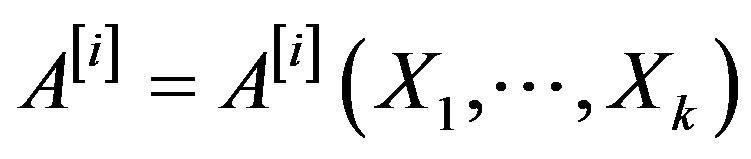 satisfy the combinatorial equations,
satisfy the combinatorial equations,
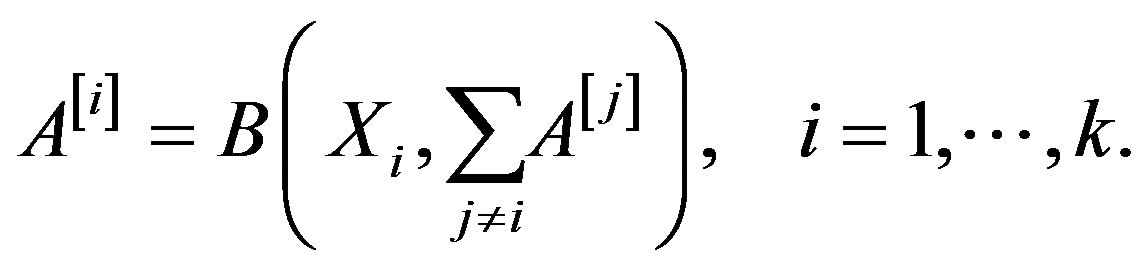 (6)
(6)
Proof. Figure 3(b) shows a 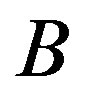 -structure, where singletons of sort
-structure, where singletons of sort 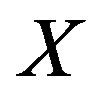 (resp.,
(resp.,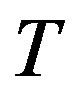 ) are drawn as black dots (resp., white triangles). The result follows from the fact that an
) are drawn as black dots (resp., white triangles). The result follows from the fact that an 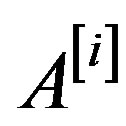 -structure is a
-structure is a 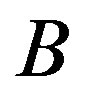 -structure in which the blackdots are replaced by singletons of sort
-structure in which the blackdots are replaced by singletons of sort 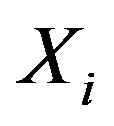 and the white triangles are replaced by arbitrary
and the white triangles are replaced by arbitrary 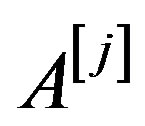 -structures,
-structures,  , (see Figure 4(a), where
, (see Figure 4(a), where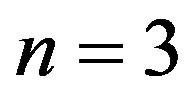 ,
, 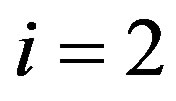 ,
, 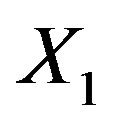 -singletons are white dots,
-singletons are white dots, 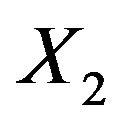 -singletons are black dots,
-singletons are black dots, 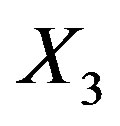 -singletons are gray dots).
-singletons are gray dots).
Definition 3 Any subspecies 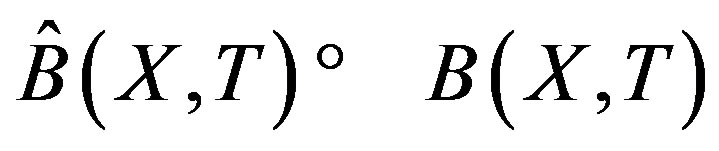 is called a runs selector species. The species
is called a runs selector species. The species
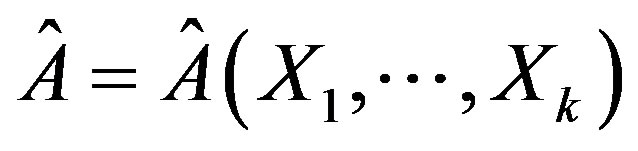
of  -arborescences having only runs of sorts of type
-arborescences having only runs of sorts of type 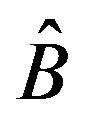 is defined by
is defined by
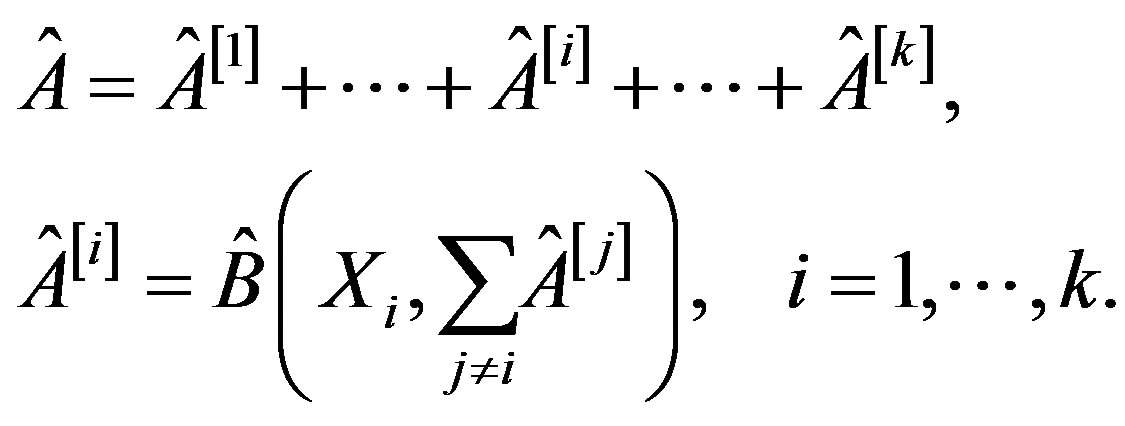 (7)
(7)
An 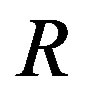 -arborescent word having only runs of type
-arborescent word having only runs of type 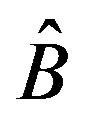 over the alphabet
over the alphabet 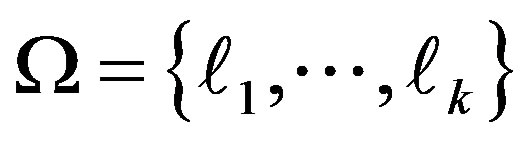 is the result of the replacement of each vertex of sort
is the result of the replacement of each vertex of sort 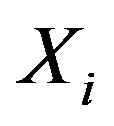 in a
in a  -structure, by the letter
-structure, by the letter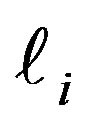 , for
, for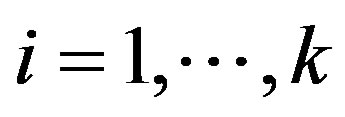 .
.
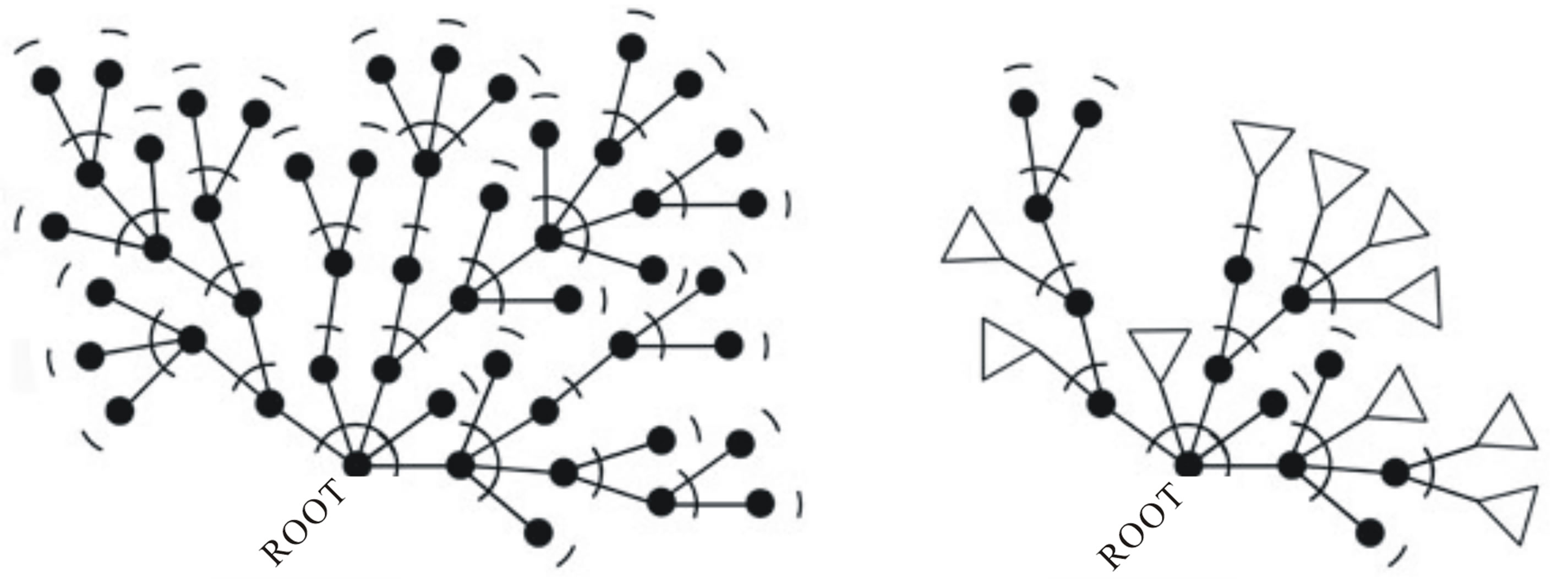
It is worthwhile to note that a runs selector species 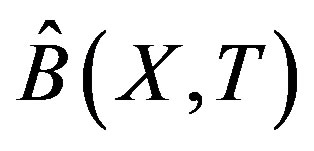 does not only select the shape of the runs under interest but also specifies how these runs should be interconnected. For example•
does not only select the shape of the runs under interest but also specifies how these runs should be interconnected. For example• 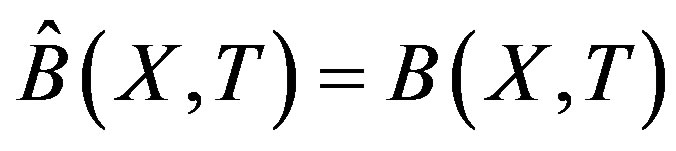 selects unrestricted runs (see Figure 3(b))•
selects unrestricted runs (see Figure 3(b))•  selects linear runs (see Figure 5(a))•
selects linear runs (see Figure 5(a))• 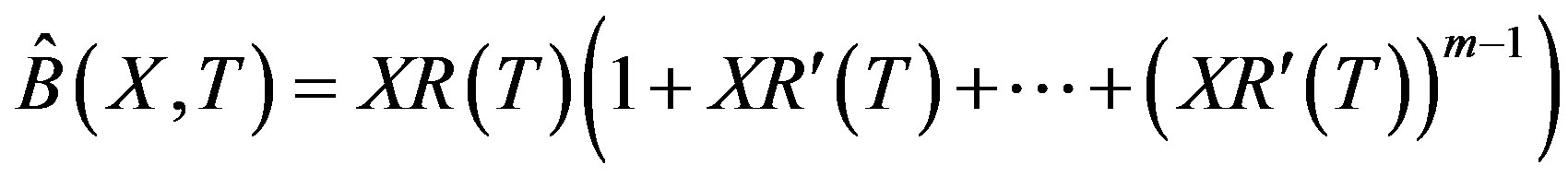 selects linear runs of length
selects linear runs of length •
•  selects singleton runs (see Figure 5(b))•
selects singleton runs (see Figure 5(b))• 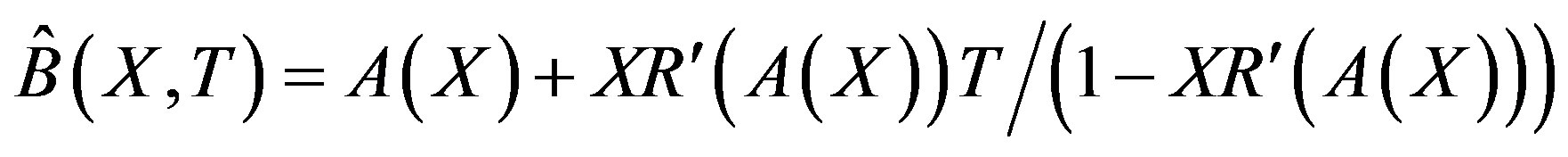 selects maximal runs (see Figure 5(c)), etc.
selects maximal runs (see Figure 5(c)), etc.
The main interest in Lemma 2 and Definition 3 is that they give rise to a variety of enumerative results about runs of various types in 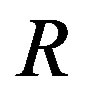 -arborescent words by the introduction of special weight counters in (5)-(7). For example, taking
-arborescent words by the introduction of special weight counters in (5)-(7). For example, taking , where
, where 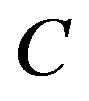 is the species of cyclic permutations, Equation (3) takes the form
is the species of cyclic permutations, Equation (3) takes the form
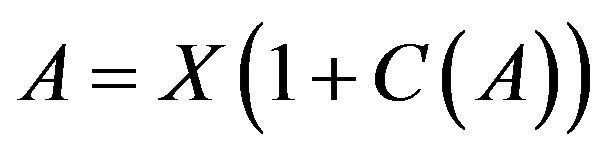 whose solution is the species
whose solution is the species  of
of
 (a) (b)
(a) (b)
Figure 3. (a)  -arborescence; (b)
-arborescence; (b)  -structure.
-structure.
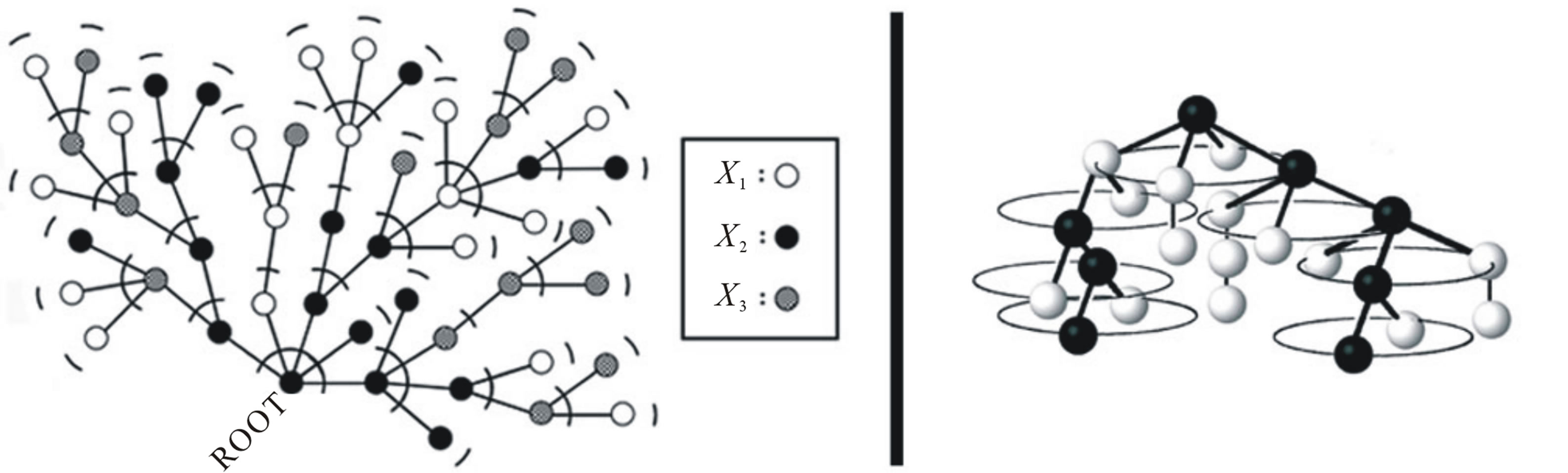 (a) (b)
(a) (b)
Figure 4. (a) 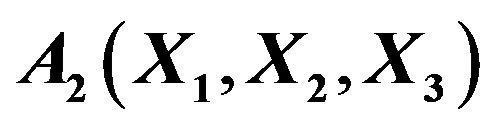 -structure; (b) Mobile word with linear runs.
-structure; (b) Mobile word with linear runs.
 (a) (b) (c)
(a) (b) (c)
Figure 5. Selectors for (a) Linear runs; (b) Singleton runs; and (c) Maximal runs.
mobiles in the terminology of Bergeron, Labelle, and Leroux [7]. The reason is the following. Putting the root on top, the descendents of each node can be thought as turning around along horizontal circles. In this case, 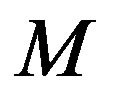 -words are called mobile words. Figure 4(b) illustrates a mobile word on a 2-letter alphabet
-words are called mobile words. Figure 4(b) illustrates a mobile word on a 2-letter alphabet
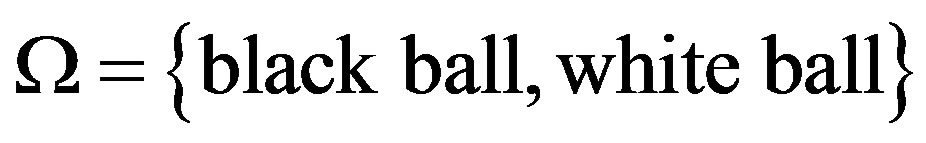 having only linear (type (ii)) runs.
having only linear (type (ii)) runs.
The introduction of a run counter 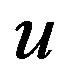 and the simultaneous substitutions
and the simultaneous substitutions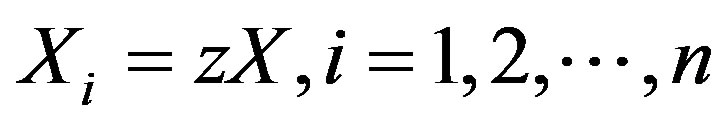 , in (5)-(7), where
, in (5)-(7), where 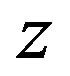 is a letter counter lead to the following general proposition.
is a letter counter lead to the following general proposition.
Proposition 4
Let 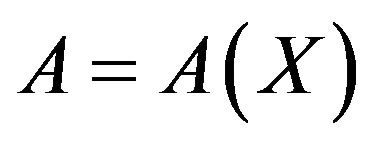 be the species of R-arborescences and
be the species of R-arborescences and 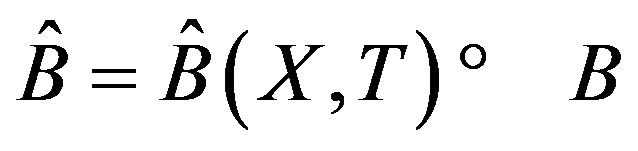 be a runs selector species. Give a weight
be a runs selector species. Give a weight 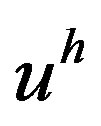 to each
to each  -word with
-word with 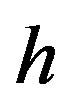 runs and let
runs and let 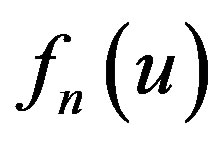 be the total weight of
be the total weight of  -words of length
-words of length 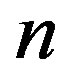 having only runs of type
having only runs of type 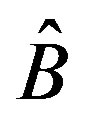 over the
over the 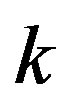 -letter alphabet
-letter alphabet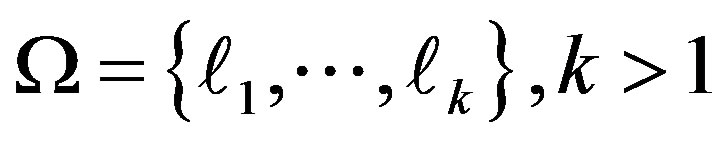 . Then the generating series
. Then the generating series
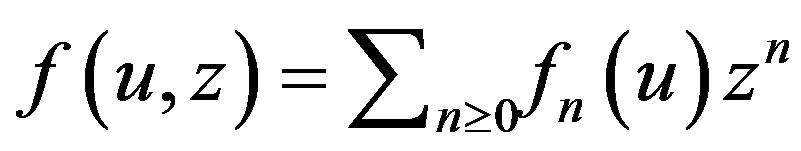
is given by,
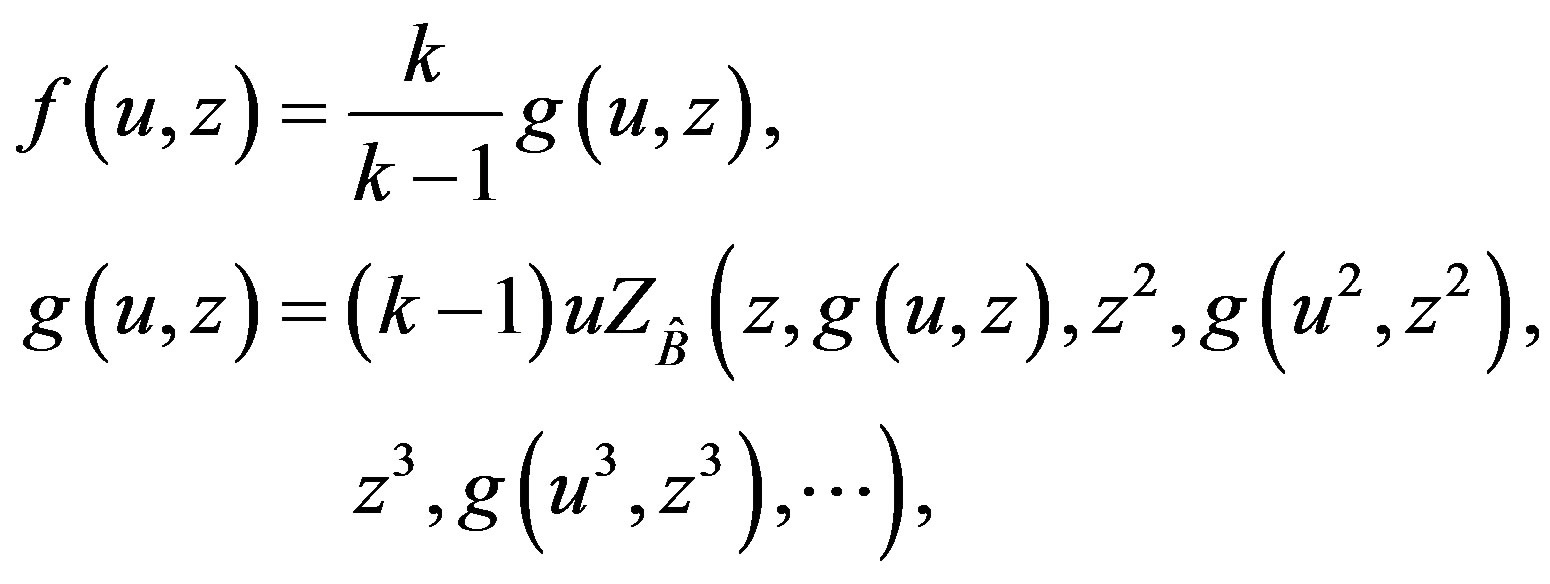 (8)
(8)
where 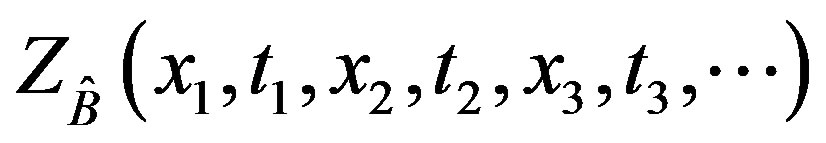 is the cycle index series of
is the cycle index series of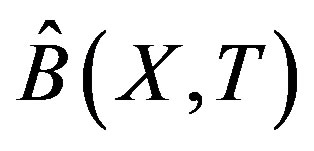 . In the special case,
. In the special case, 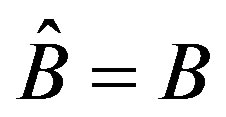 , corresponding to unrestricted runs, the generating series
, corresponding to unrestricted runs, the generating series 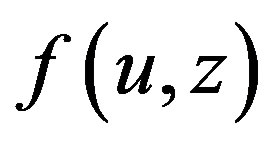 is explicitely given by,
is explicitely given by,
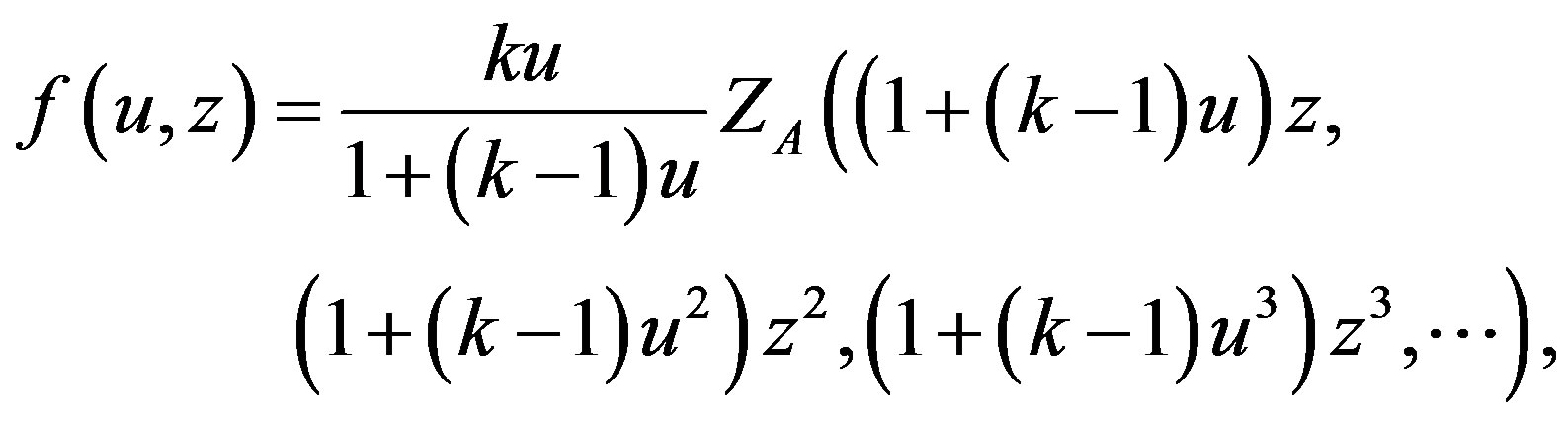 (9)
(9)
where 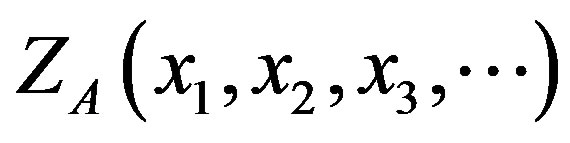 is the cycle index series of
is the cycle index series of .
.
Proof. The series 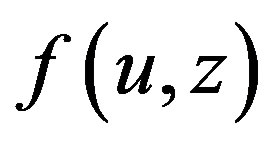 is the total weight of all unlabelled
is the total weight of all unlabelled  -structures in which each node is replaced by the variable counter
-structures in which each node is replaced by the variable counter 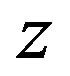 and each run is given a weight
and each run is given a weight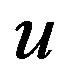 . Now, consider the species
. Now, consider the species
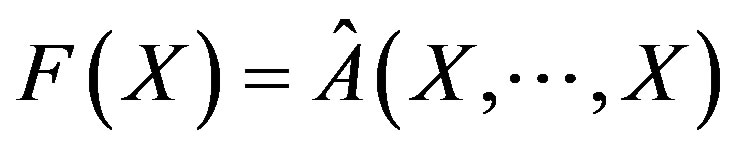
obtained by the substitutions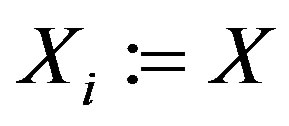 ,
, 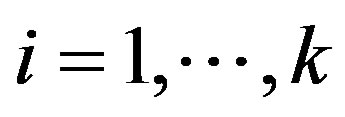 , in the species
, in the species 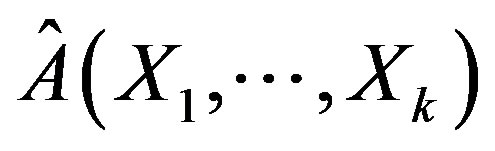 weighted as above. Then, from Pólya Theory in the context of species,
weighted as above. Then, from Pólya Theory in the context of species, 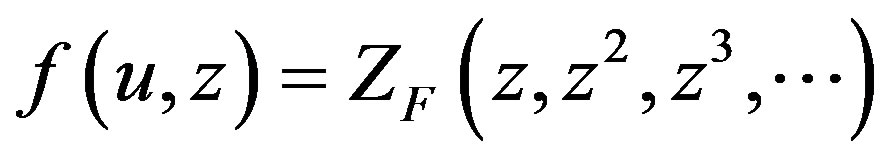 , where
, where 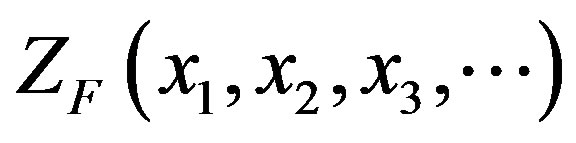 is the cycle index series of
is the cycle index series of 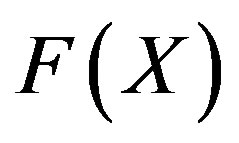 . By symmetry, all the species
. By symmetry, all the species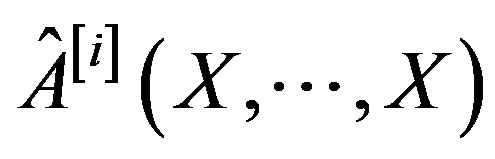 ,
,  , are equal. So that they can all be identified with a single species,
, are equal. So that they can all be identified with a single species, 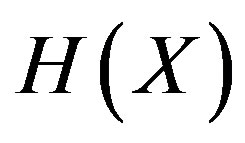 , say. By (4), taking into account the run counter,
, say. By (4), taking into account the run counter, 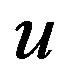 ,
,
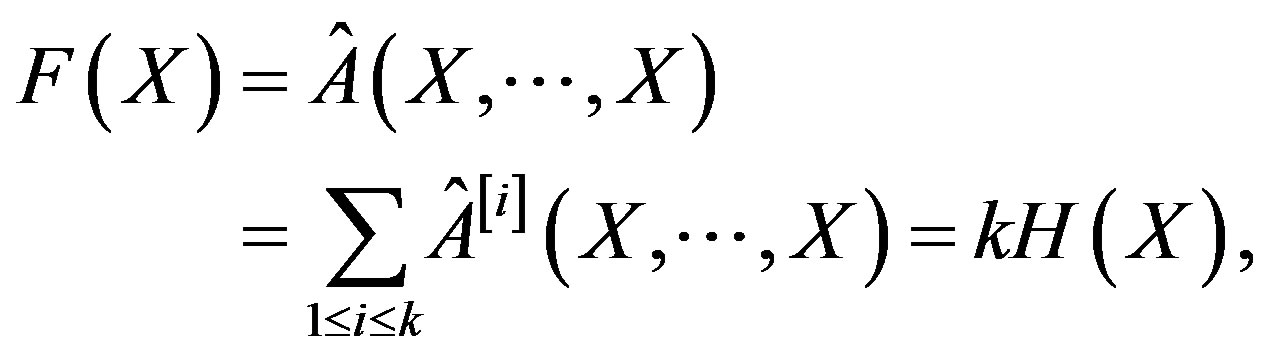 (10)
(10)
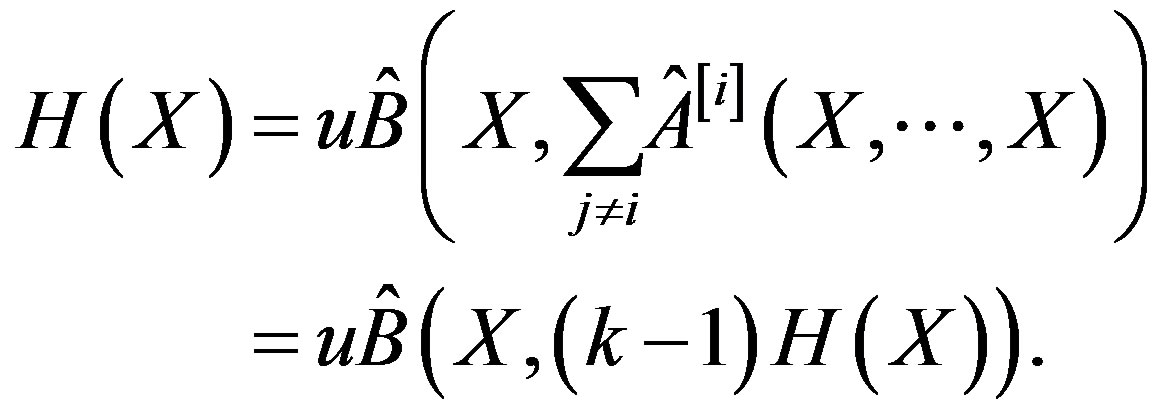 (11)
(11)
Define the species 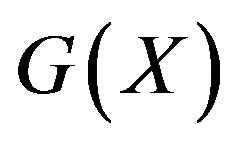 by
by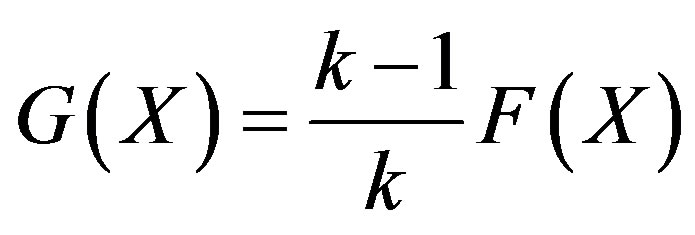 .
.
Then, 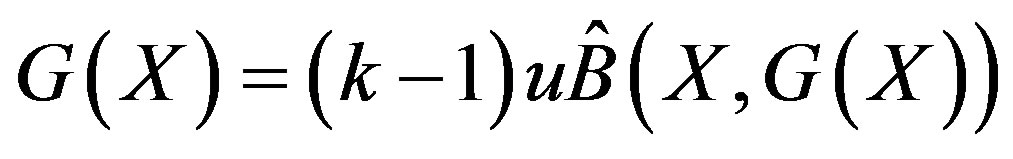 , and the recursive scheme (8) follows by taking the cycle index series of both sides of this last equation. Now, consider the special choice,
, and the recursive scheme (8) follows by taking the cycle index series of both sides of this last equation. Now, consider the special choice, . Then, using the fact that
. Then, using the fact that
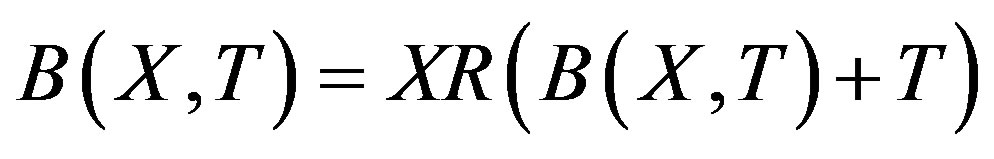 we have,
we have,
 (12)
(12)
Hence,
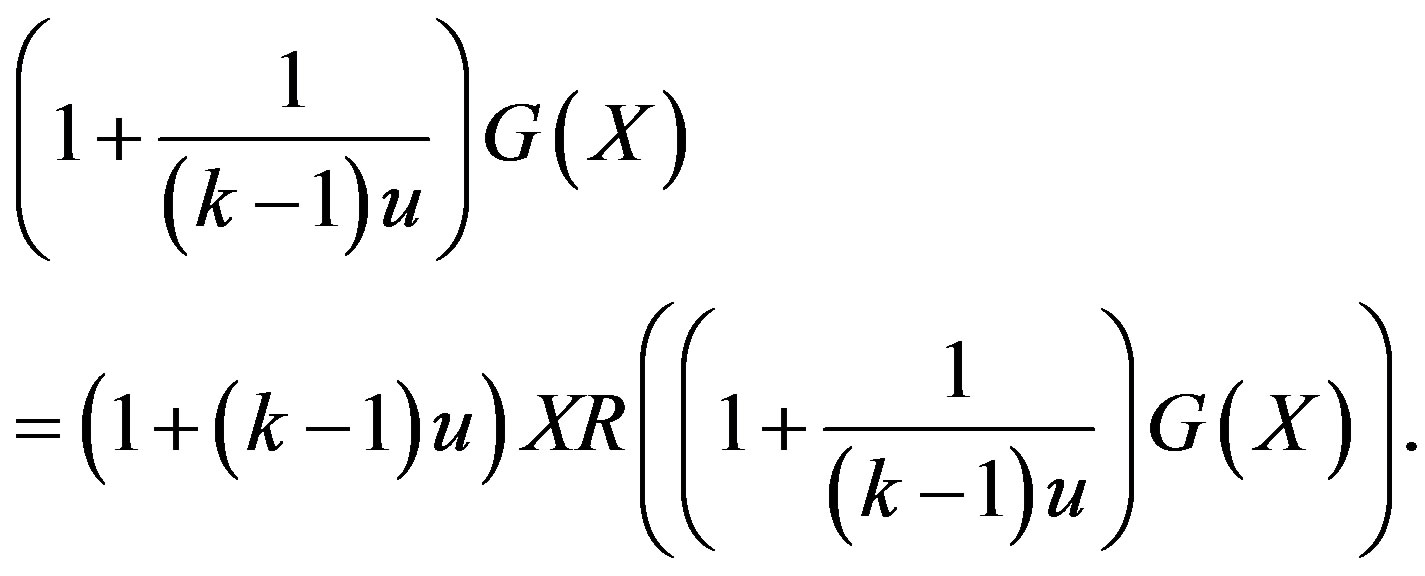
This implies that the species
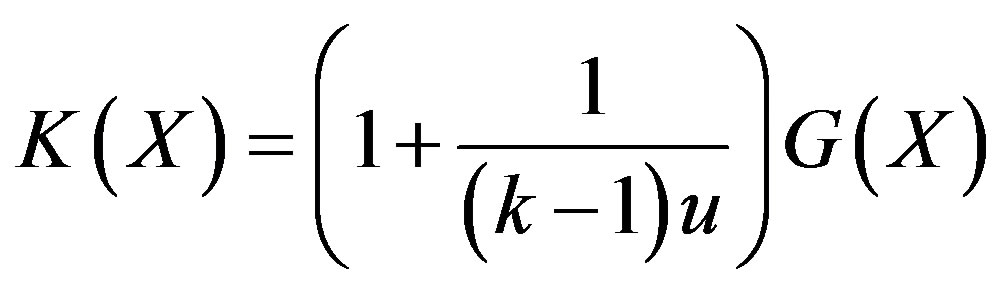
satisfies the equation
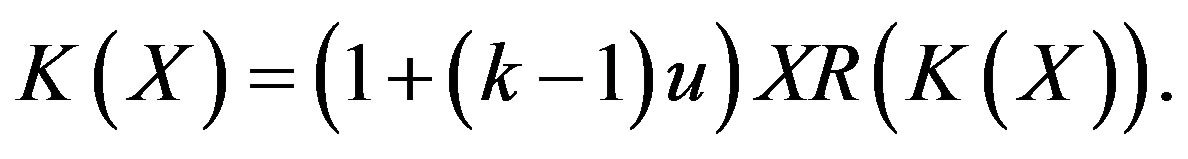
Since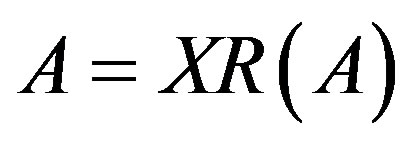 , we must have
, we must have

by the implicit species theorem [4]. So that,
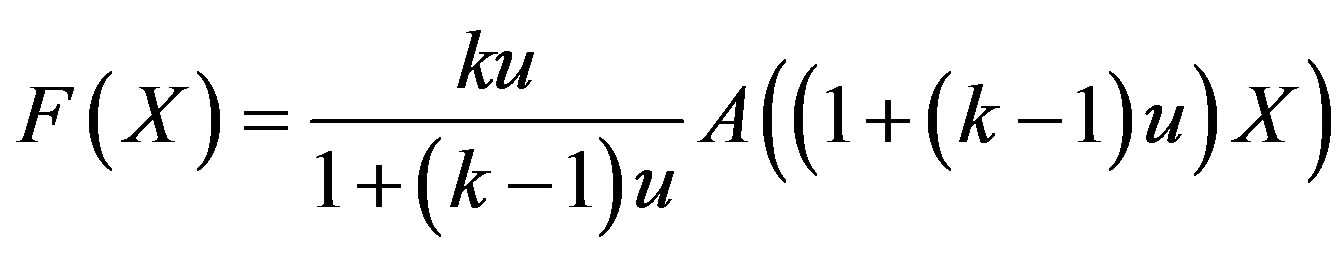 and (9) follows by taking the cycle index series on both sides of this combinatorial equation.
and (9) follows by taking the cycle index series on both sides of this combinatorial equation.
The following corollary is immediate from Proposition 4, via the substitution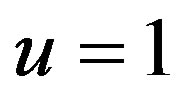 .
.
Corollary 5 Let 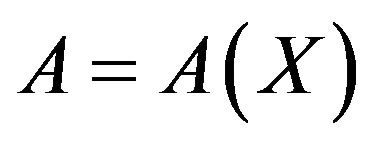 be the species of
be the species of 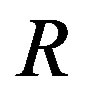 arborescences and
arborescences and 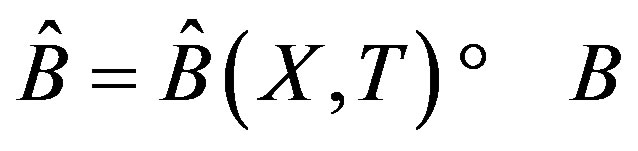 be a runs selector species. Let
be a runs selector species. Let 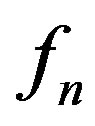 be the number of
be the number of  -words of length
-words of length 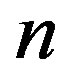 having only runs of type
having only runs of type 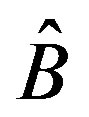 over the
over the 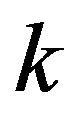 - letter alphabet
- letter alphabet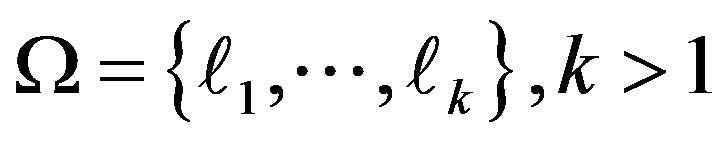 . Then the generating series,
. Then the generating series, 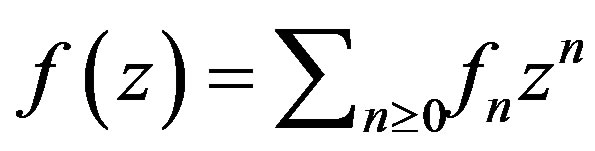 , is given by,
, is given by,
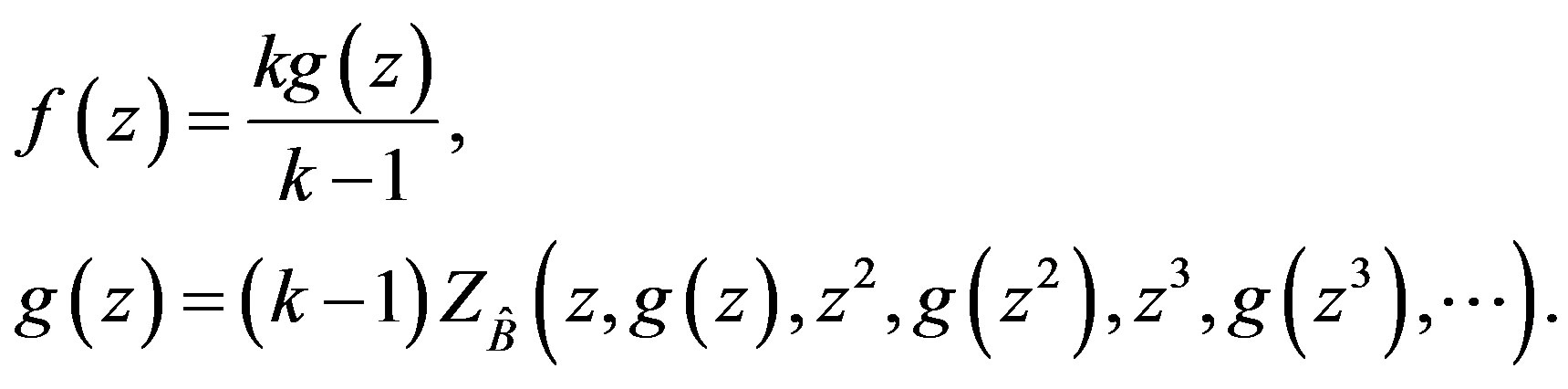 (13)
(13)
In the special case, 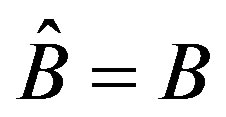 , corresponding to unrestricted runs, the series
, corresponding to unrestricted runs, the series 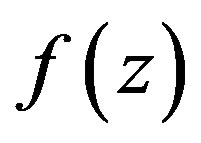 is explicitely given by,
is explicitely given by,
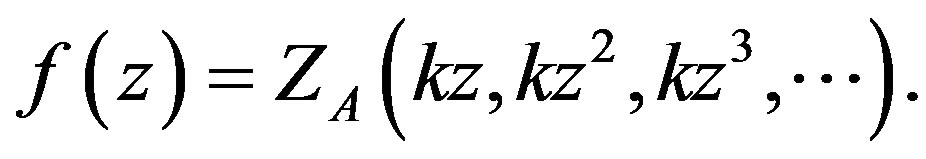 (14)
(14)
The use of cycle index series above comes from the fact that R-enriched arborescences have non-trivial automorphisms, in general. This occurs when R-structures have non-trivial automorphisms. In the asymmetric case, however, that is when the automorphism group of each 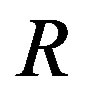 -structure is trivial, it is well-known that cycle index series reduce to exponential generating series.
-structure is trivial, it is well-known that cycle index series reduce to exponential generating series.
Corollary 6 If the species, 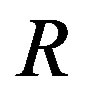 , is asymmetric, then
, is asymmetric, then
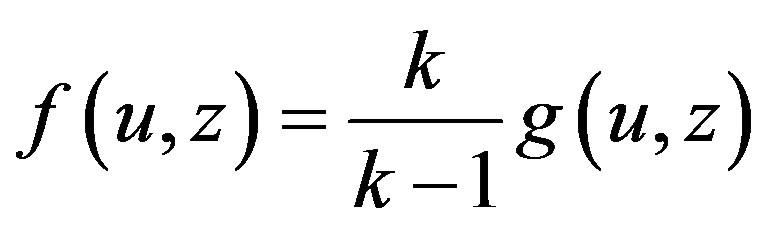 and
and 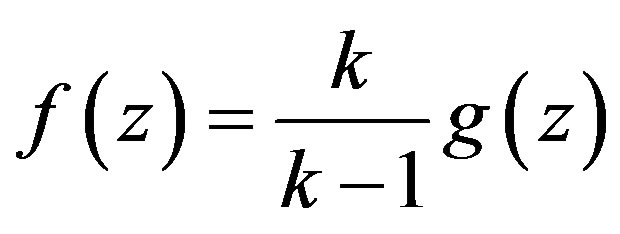 with
with
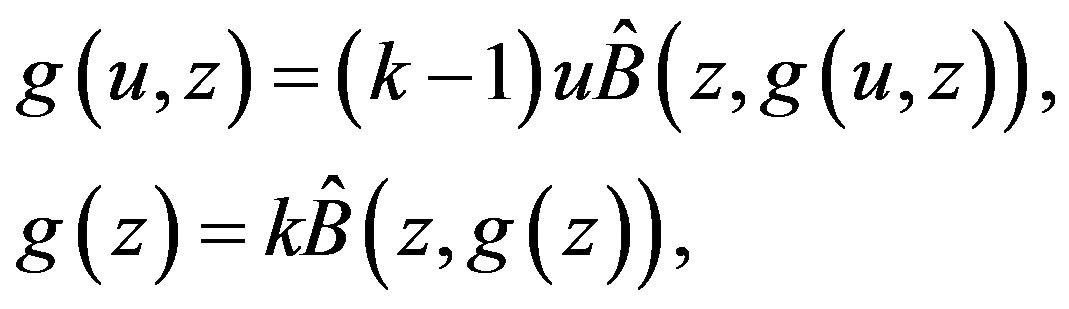 (15)
(15)
where 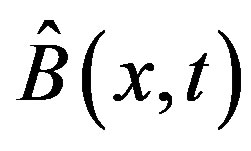 is the exponential generating series of
is the exponential generating series of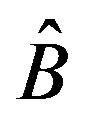 . Moreover, if
. Moreover, if , then
, then
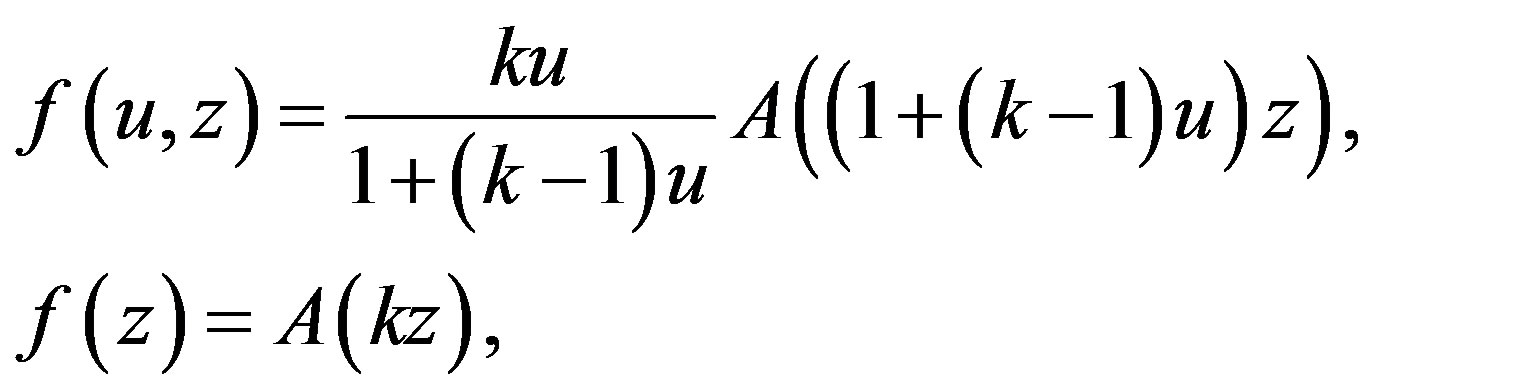 (16)
(16)
where 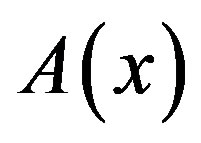 is the exponential generating series of
is the exponential generating series of .
.
Various computational general methods exist to obtain successive approximations (or explicit expressions, sometimes) for 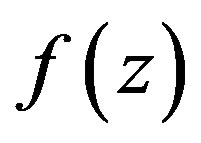 and
and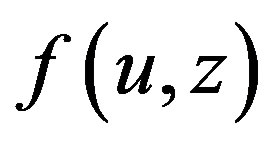 . Simple iteration is the most obvious one, but more sophisticated methods include Good-Lagrange inversion, the use of eclosion operators and Newton-Raphson iteration adapted to cycle index series (see Labelle [8,9]). There is not enough space here to describe these methods in detail in the present context. Instead, we have chosen to illustrate our analysis of runs by focusing on specific examples.
. Simple iteration is the most obvious one, but more sophisticated methods include Good-Lagrange inversion, the use of eclosion operators and Newton-Raphson iteration adapted to cycle index series (see Labelle [8,9]). There is not enough space here to describe these methods in detail in the present context. Instead, we have chosen to illustrate our analysis of runs by focusing on specific examples.
3. Specific Examples
3.1. Runs in Ordinary Words
With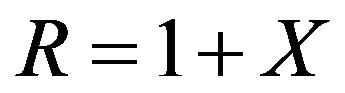 , Equation (3) takes the form
, Equation (3) takes the form 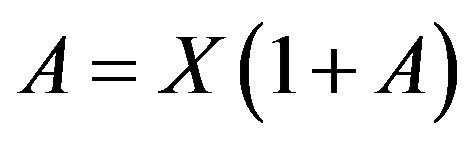 whose solution is the asymmetric species
whose solution is the asymmetric species 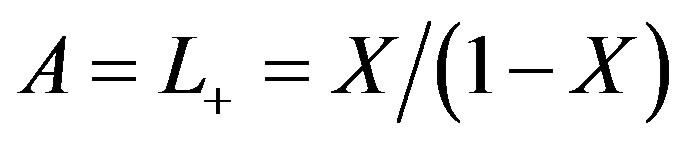 of non empty linear orders. In this case,
of non empty linear orders. In this case,  -words are simply (ordinary non empty) words and Equation (5) takes the form
-words are simply (ordinary non empty) words and Equation (5) takes the form 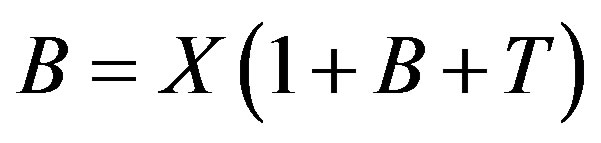 whose solution is
whose solution is
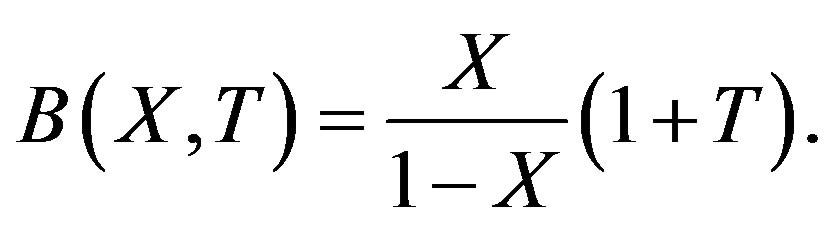 (17)
(17)
• In the case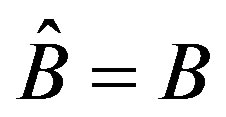 , of unrestricted runs, (16) immediately gives,
, of unrestricted runs, (16) immediately gives,
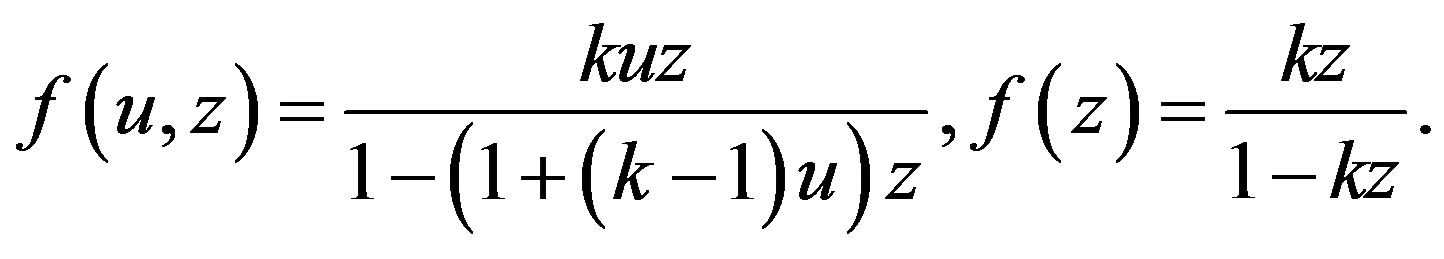 (18)
(18)
• Take the runs selector
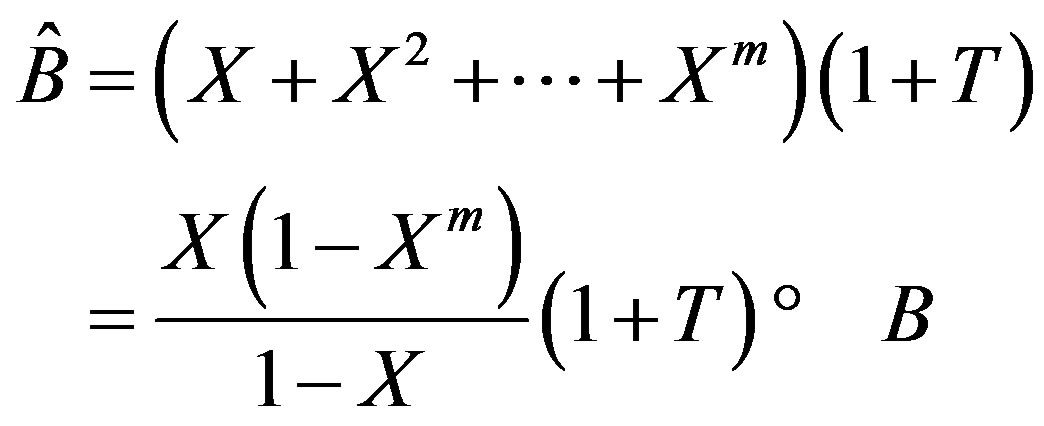
of linear runs of length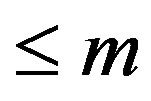 . Then recurrences (15) take the form,
. Then recurrences (15) take the form,
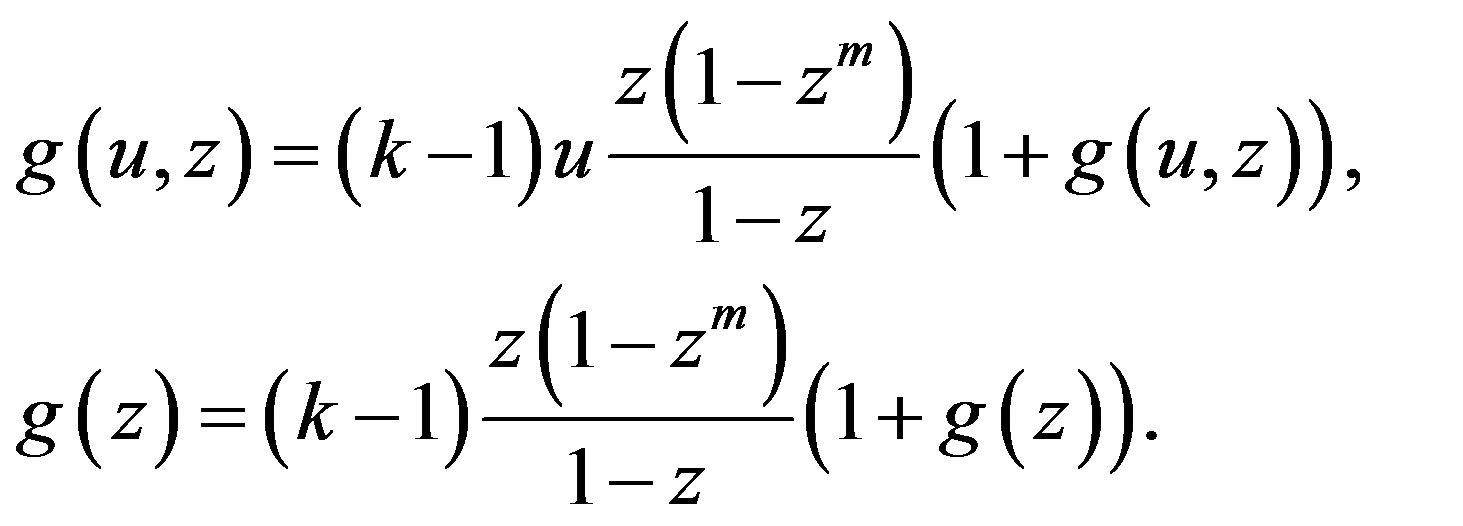 (19)
(19)
Solving for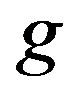 , we get the well known g.f.’s of words whose runs are of size
, we get the well known g.f.’s of words whose runs are of size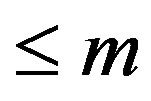 ,
,
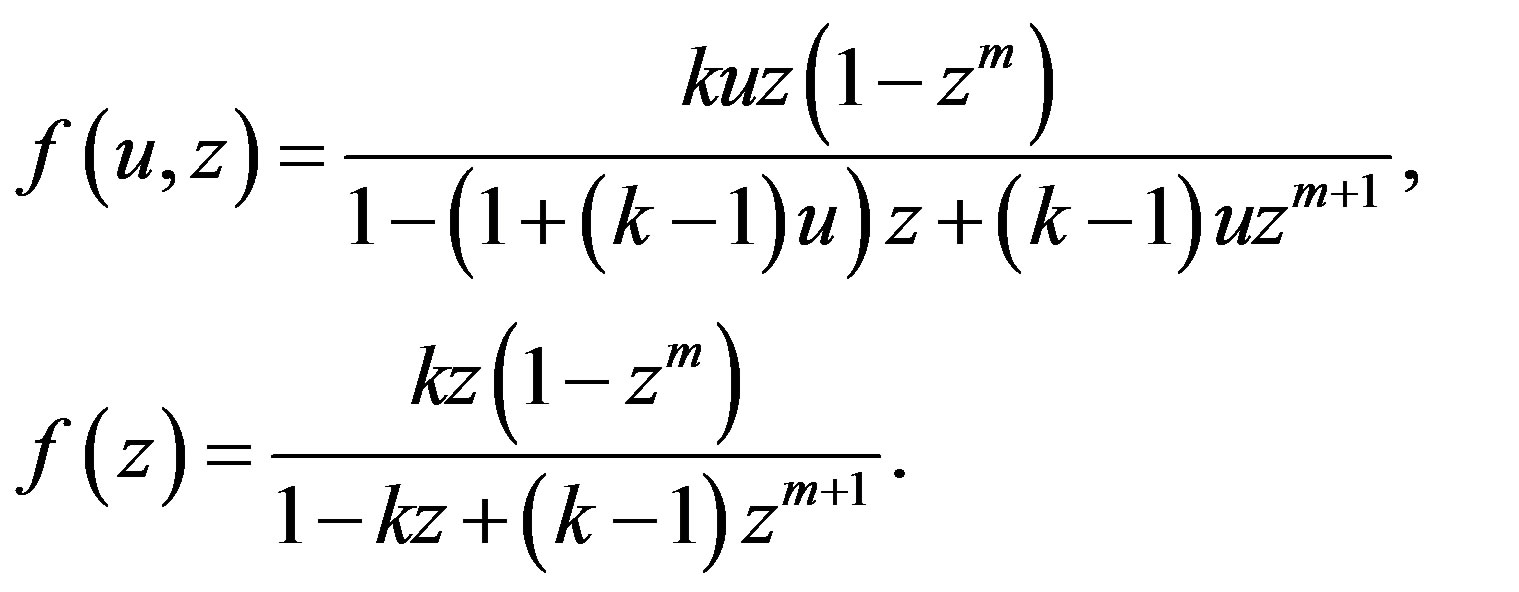 (20)
(20)
Note that taking  in (20) can be called the Fibonacci case since gives,
in (20) can be called the Fibonacci case since gives,
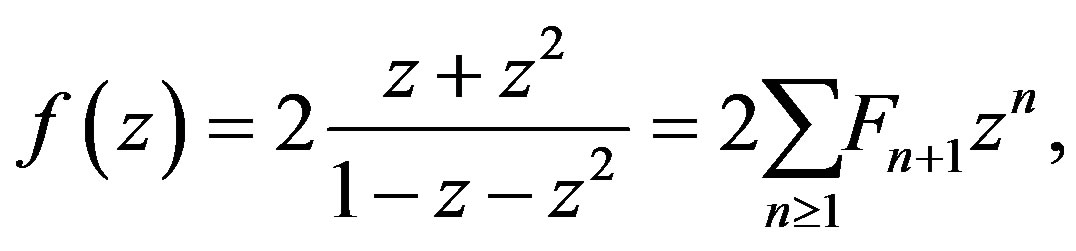 (21)
(21)
where 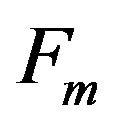 is the
is the 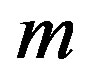 -th Fibonacci number. This corresponds to twice the well known fact that a sequence of
-th Fibonacci number. This corresponds to twice the well known fact that a sequence of 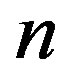 adjacent squares can be tiled by monominos or dominos in
adjacent squares can be tiled by monominos or dominos in 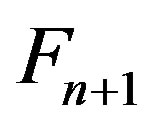 ways. Taking
ways. Taking 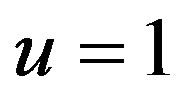 in (20) and substracting gives the g.f. of words whose maximal runs are of size
in (20) and substracting gives the g.f. of words whose maximal runs are of size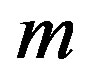 ,
,
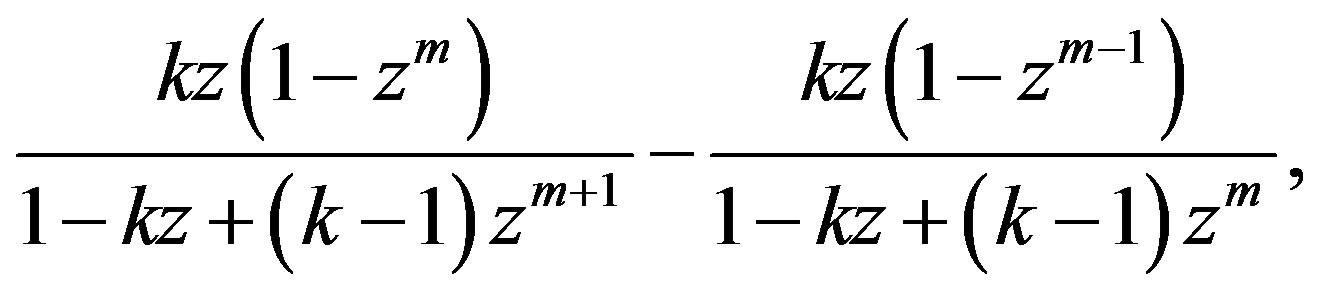 (22)
(22)
from which one can compute or estimate the expected size of the largest run (see, for example, Erdos and Revesz [10], Guibas and Odlyzko [11], Kong [12]).
• Take any subset 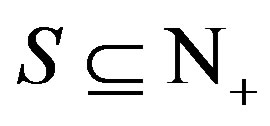 and the selector
and the selector
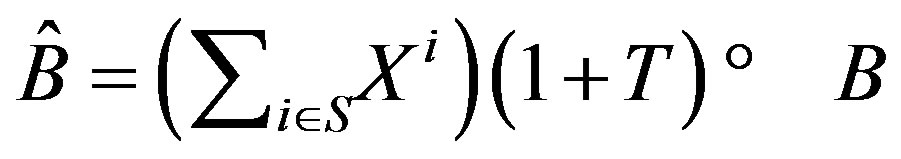 of runs of length in
of runs of length in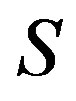 . Then,
. Then,
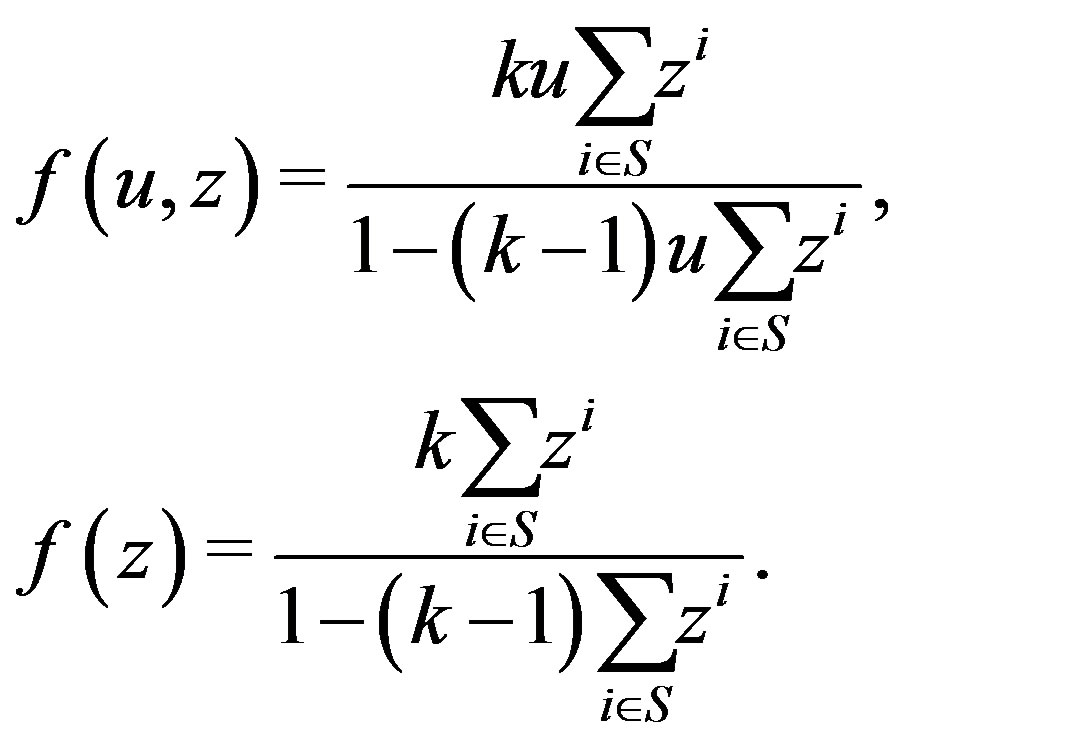 (23)
(23)
3.2. Runs in Binary Arborescent Words
Planar case. With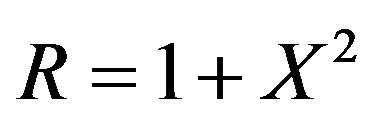 , where
, where 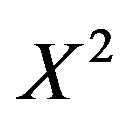 is the species of linearly ordered 2-point sets, Equation (3) takes the form
is the species of linearly ordered 2-point sets, Equation (3) takes the form 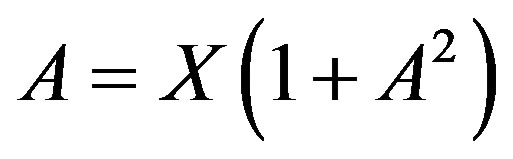 whose solution is the asymmetric species
whose solution is the asymmetric species  of binary plane arborescences. Accordingly,
of binary plane arborescences. Accordingly,  -words are binary plane arborescent words and (5) takes the form
-words are binary plane arborescent words and (5) takes the form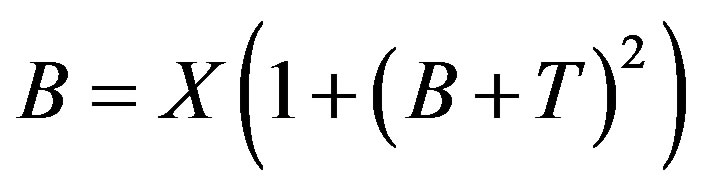 .
.
• Take the linear runs selector
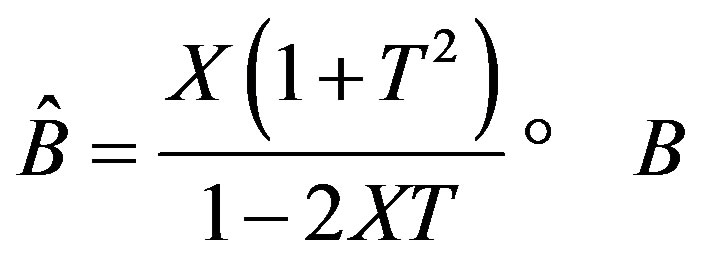
corresponding to (ii) above. Then by (16), the g.f.’s of plane binary arborescent words having only linear runs are given by,
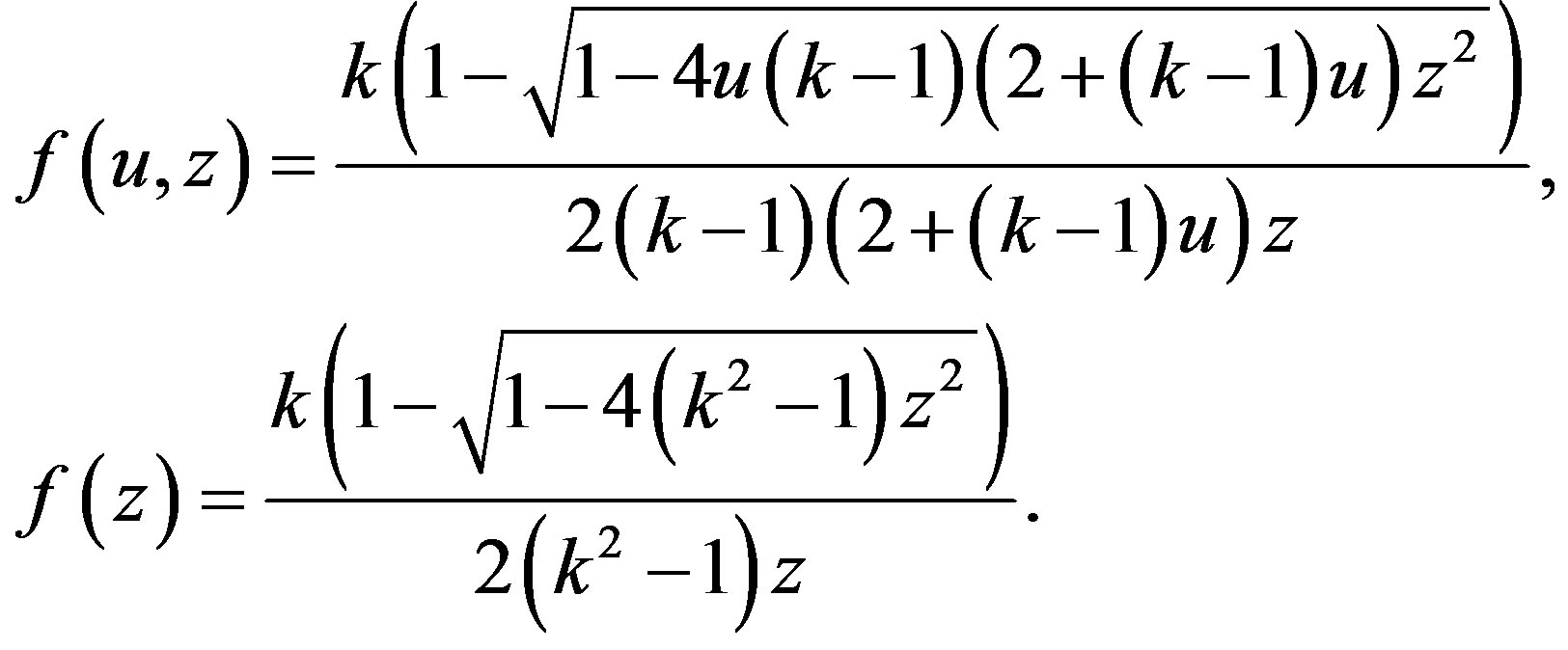 (24)
(24)
Coefficient extractions give, for 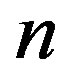 odd,
odd,
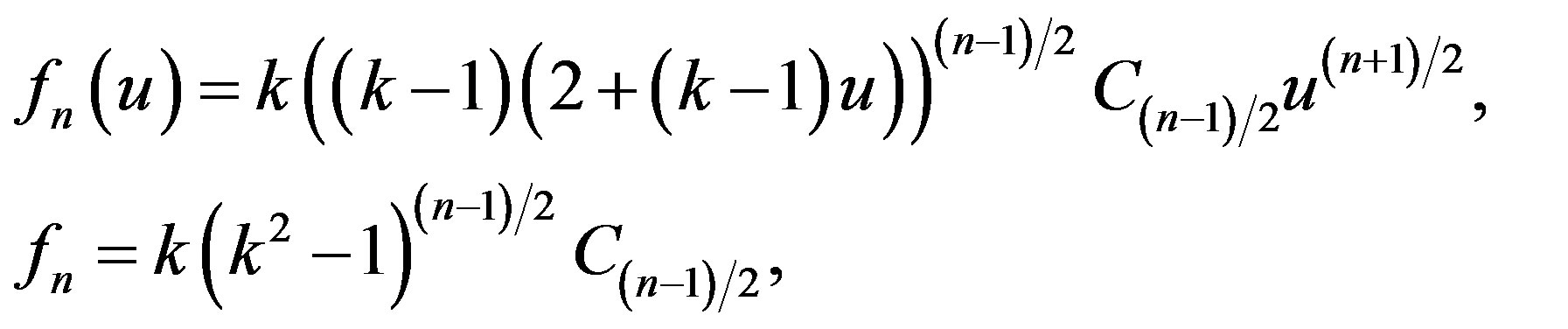
where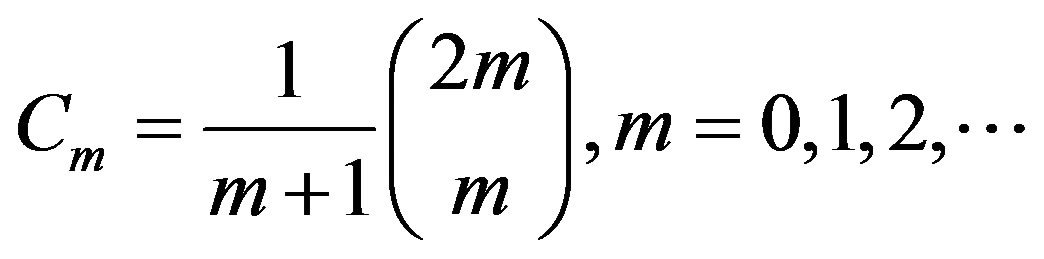 , are the Catalan numbers.
, are the Catalan numbers.
• The Fibonacci case, k = 2, m = 2, corresponds, in the present context, to case (iii) above with a 2-letter alphabet. Here,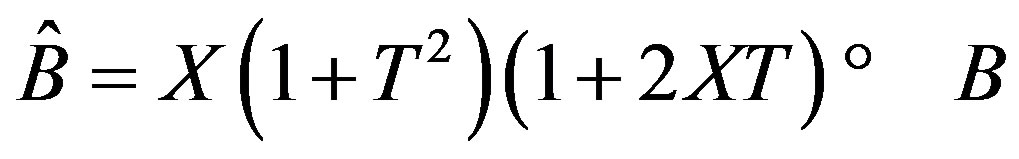 . So that,
. So that,
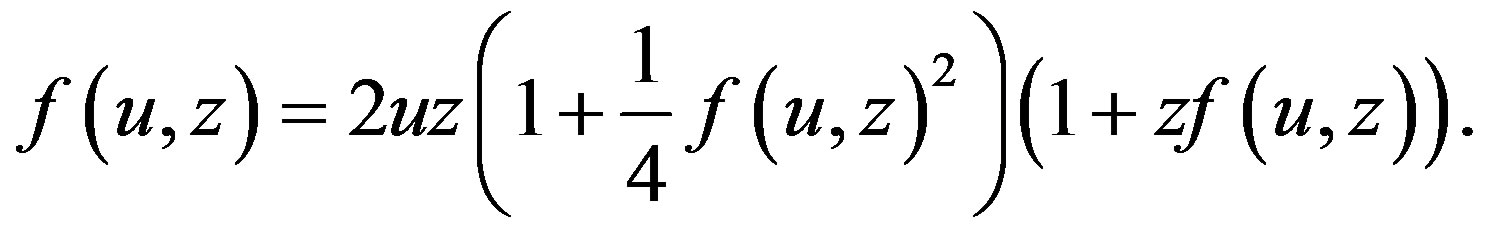 (25)
(25)
The first terms of 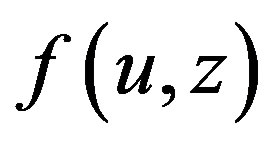 and of
and of 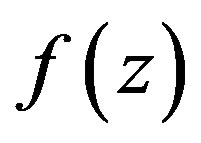 are given by,
are given by,
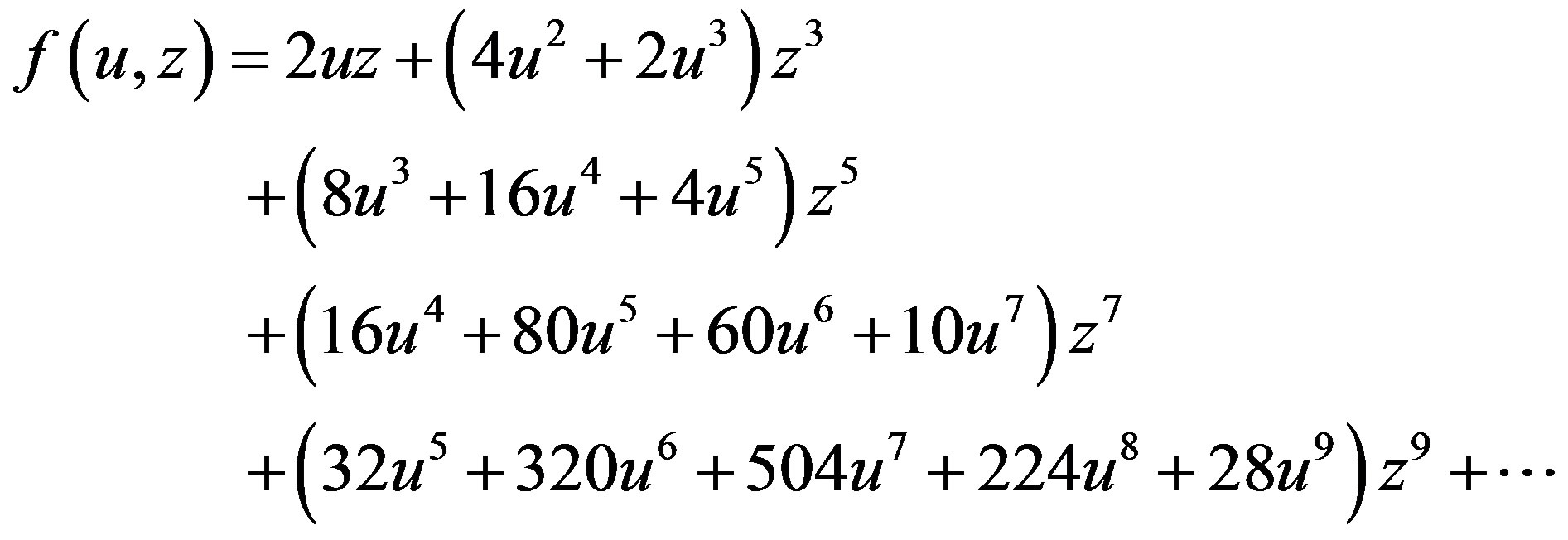
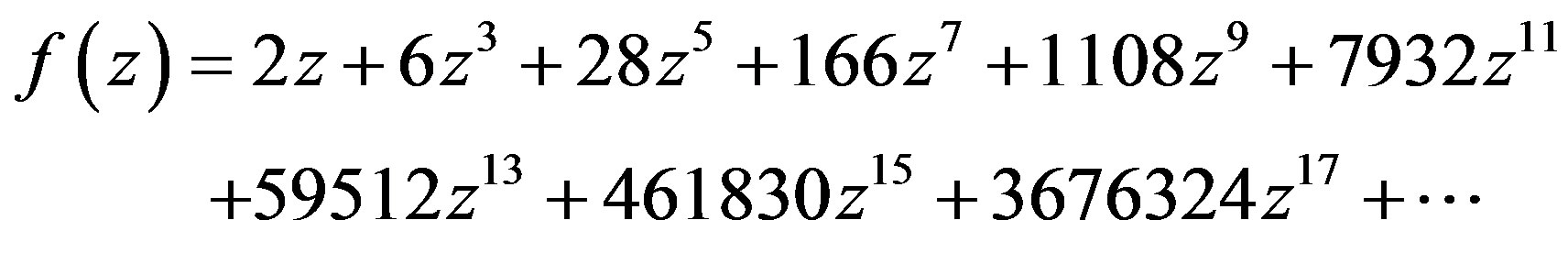
Non-planar case. With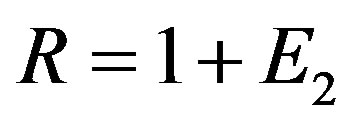 , where
, where 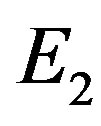 is the species of 2-element sets, Equation (3) takes the form
is the species of 2-element sets, Equation (3) takes the form 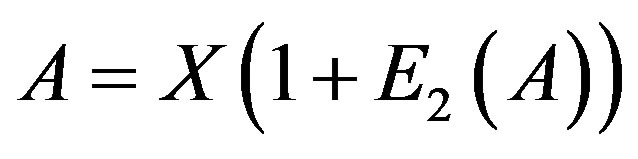 whose solution is the species
whose solution is the species 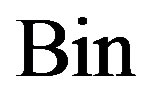 of (free) binary arborescences. Accordingly,
of (free) binary arborescences. Accordingly, 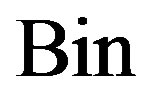 -words are binary arborescent words and (5) takes the form
-words are binary arborescent words and (5) takes the form . Note that in this case, Binstructures have non trivial symmetries in general, so that cycle-index series must be used instead of exponential generating series.
. Note that in this case, Binstructures have non trivial symmetries in general, so that cycle-index series must be used instead of exponential generating series.
• Take the linear runs selector
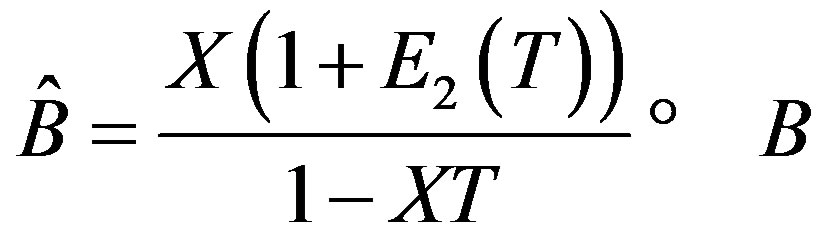
corresponding to (ii) above. Then
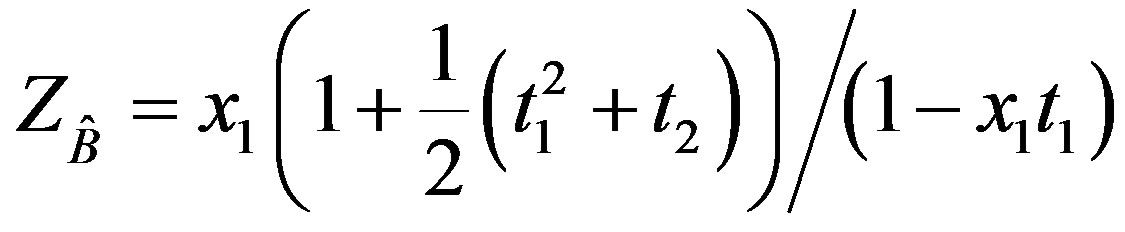
and by (8),  where,
where,
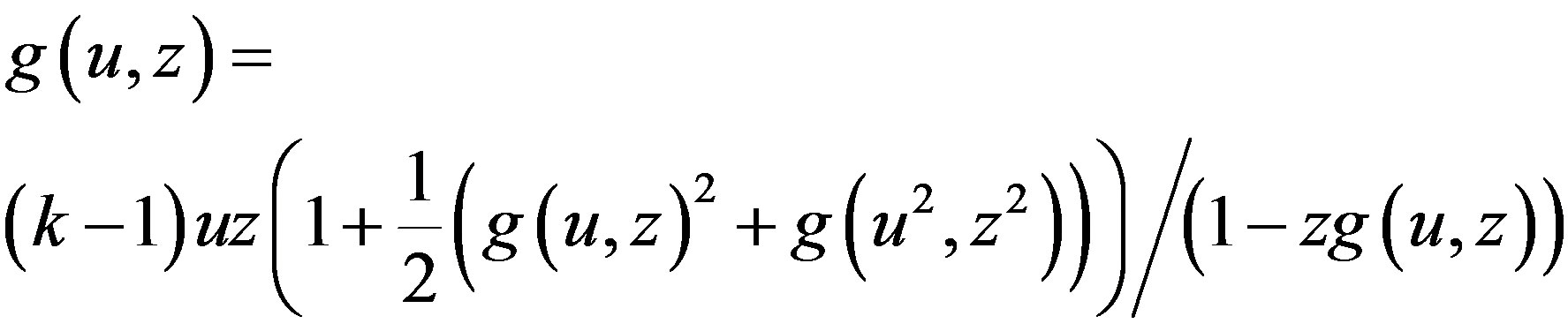 (26)
(26)
For example, the first terms of 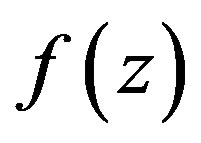 are given by•
are given by• 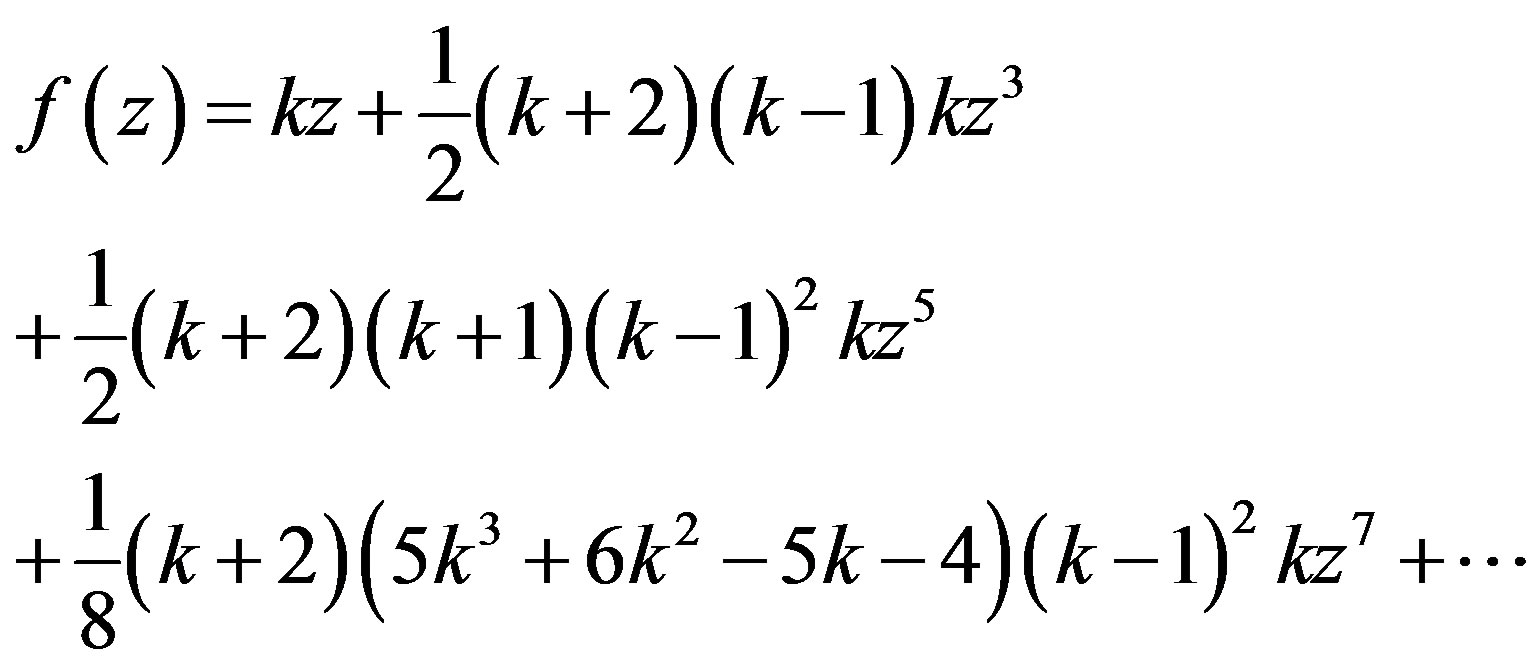
• In the Fibonacci case, k = 2, m = 2, the runs selector (iii) above is given by
 .
.
So that,
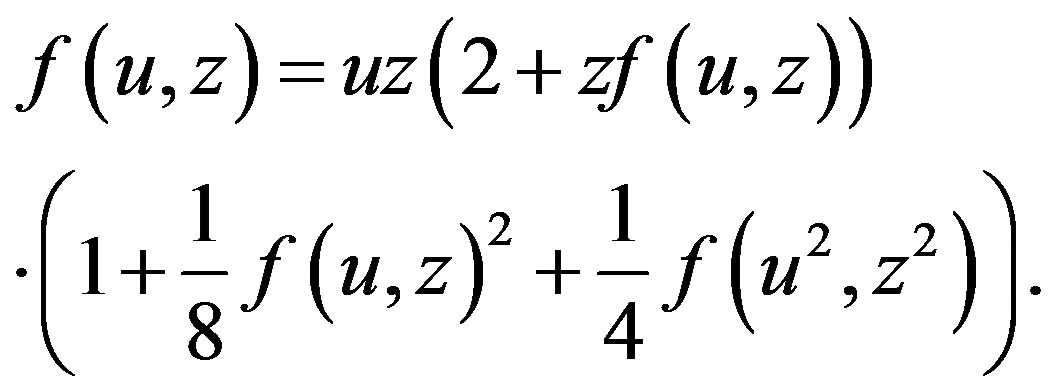 (27)
(27)
The first terms of 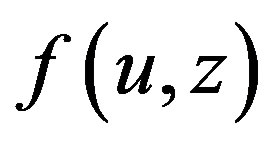 and of
and of 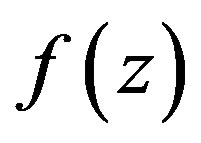 are given by,
are given by,
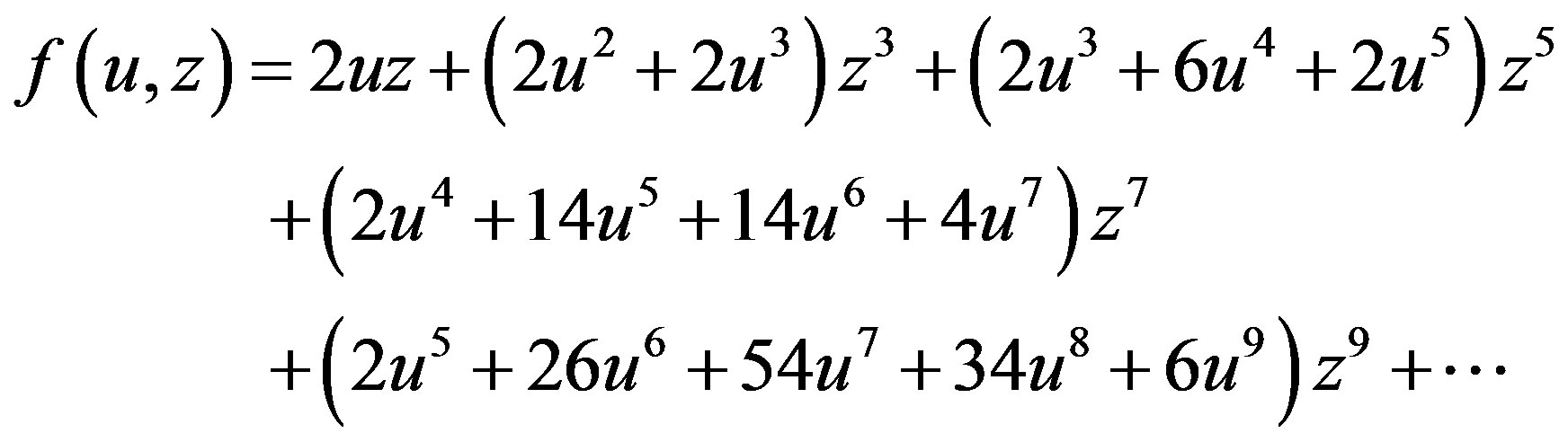

3.3. Runs in Cayley Arborescent Words
With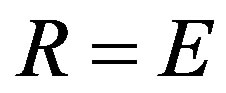 , where
, where 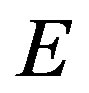 is the species of finite sets, Equation (3) takes the form
is the species of finite sets, Equation (3) takes the form 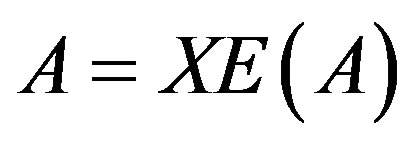 whose solution is the species
whose solution is the species  of standard Cayley arborescences. In this case,
of standard Cayley arborescences. In this case,  -words are Cayley arborescent words and equation (5) takes the form
-words are Cayley arborescent words and equation (5) takes the form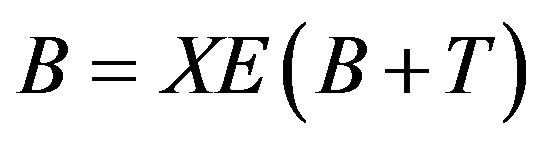 . Since
. Since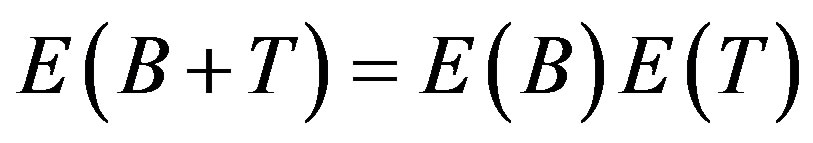 , this equation can be rewritten as
, this equation can be rewritten as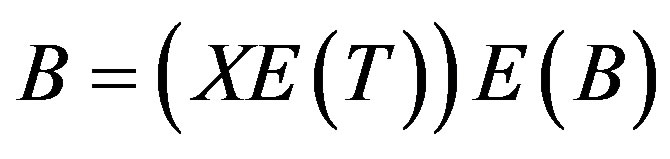 . Hence, from the unicity of solution in the implicit species theorem, we have,
. Hence, from the unicity of solution in the implicit species theorem, we have,
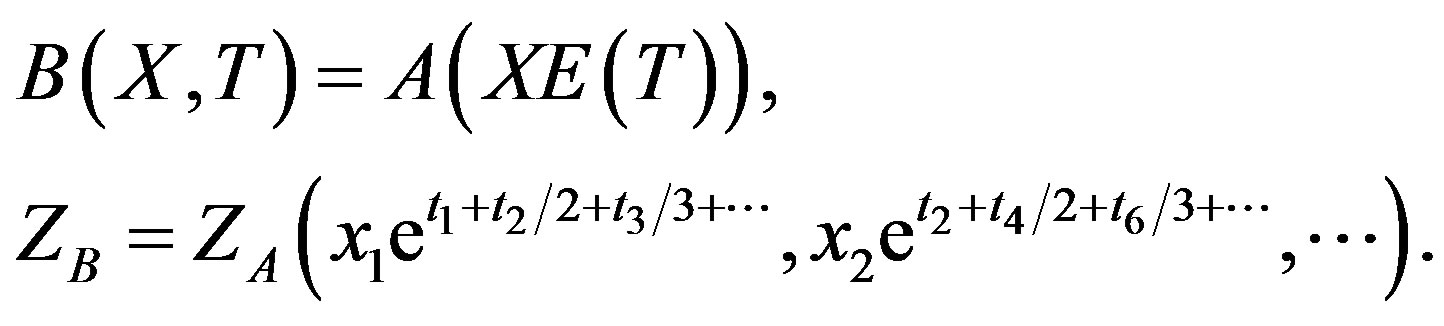 (28)
(28)
• Take the runs selector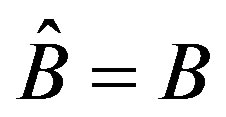 . Then since
. Then since 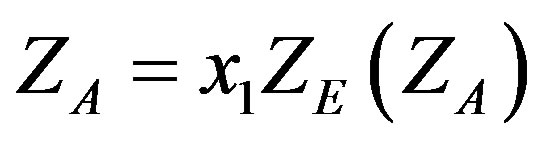 , (9) and (14) can be rewritten as,
, (9) and (14) can be rewritten as,
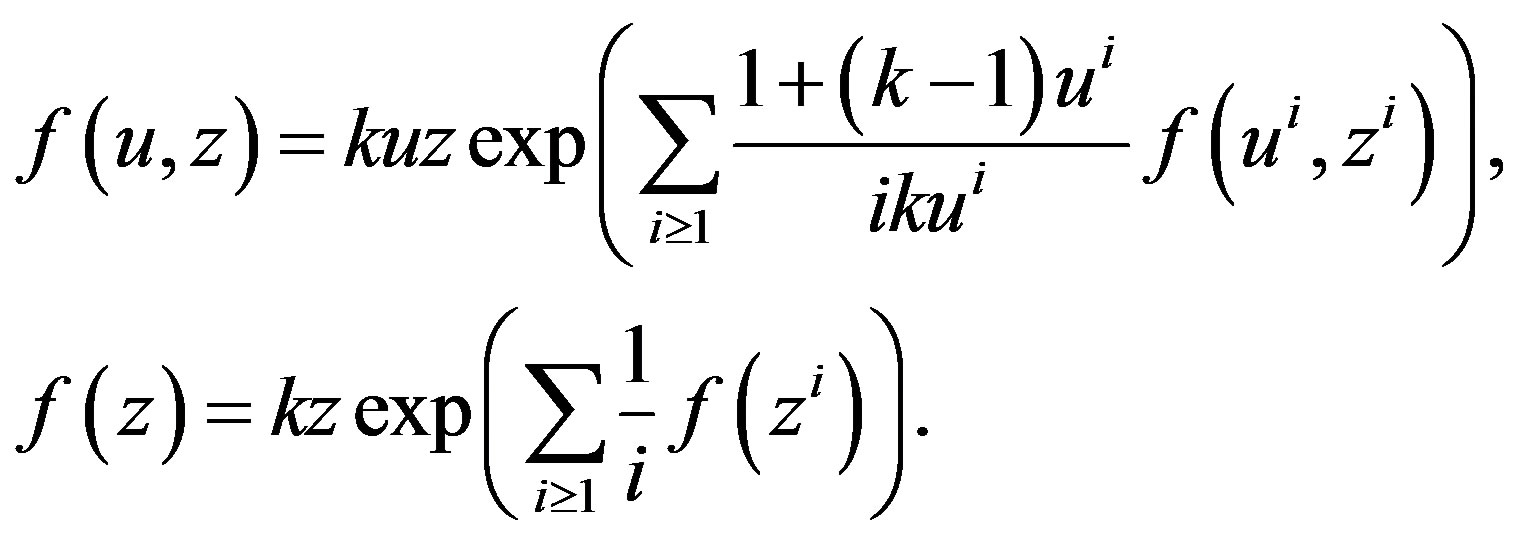 (29)
(29)
For example, the first terms of 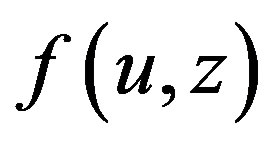 and
and 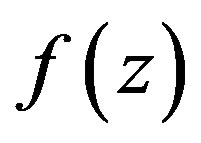 are given by•
are given by• 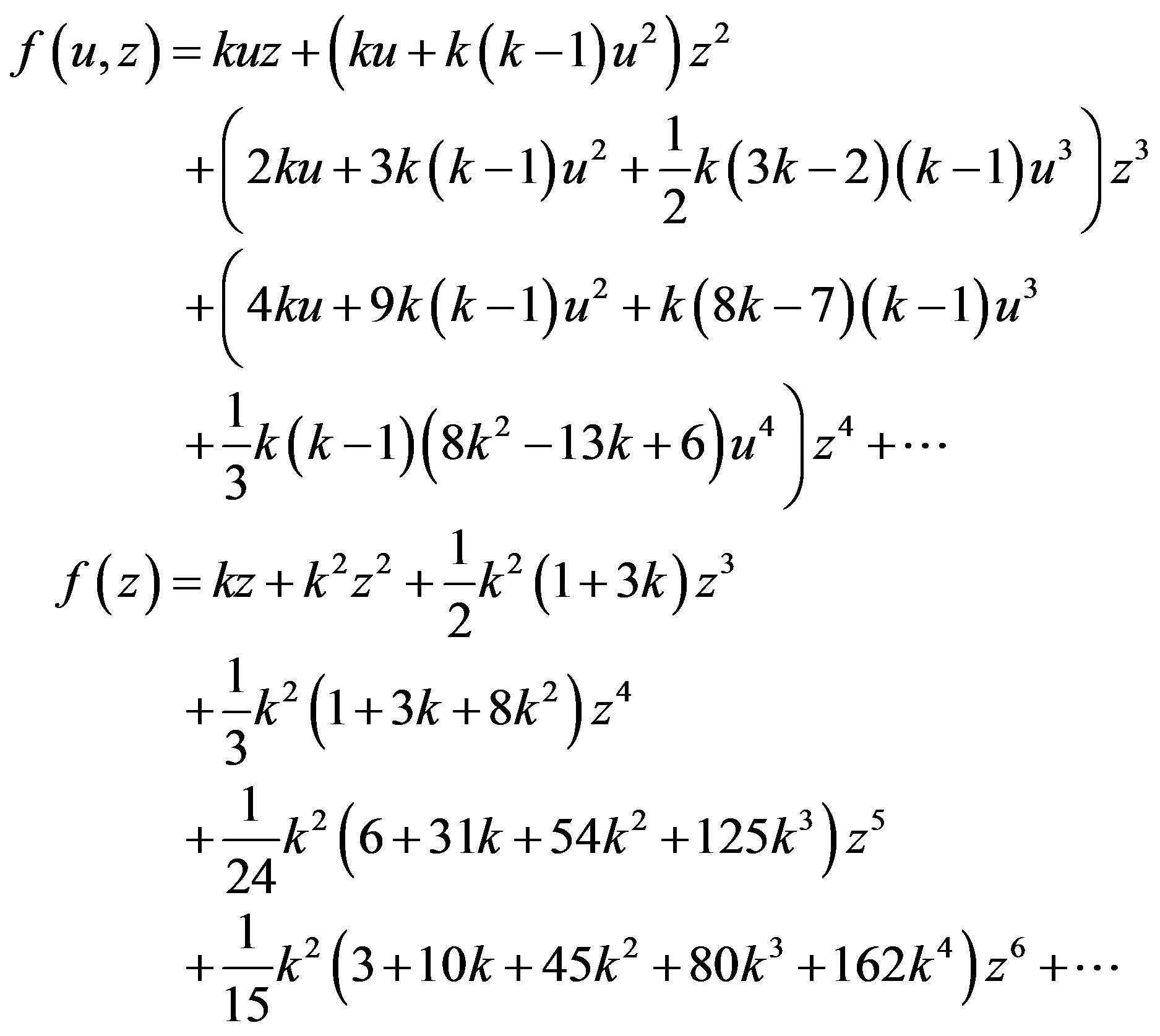
• Take the linear runs selector 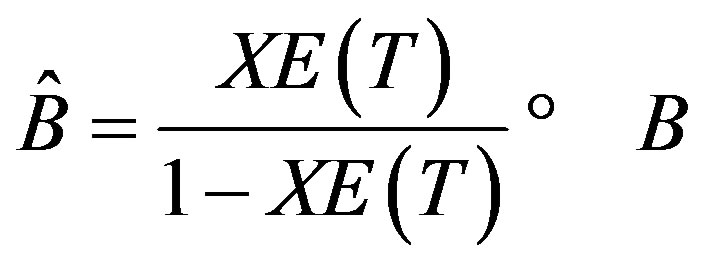 corresponding to (ii) above. Then,
corresponding to (ii) above. Then,
 (30)
(30)
More generally, the runs selector
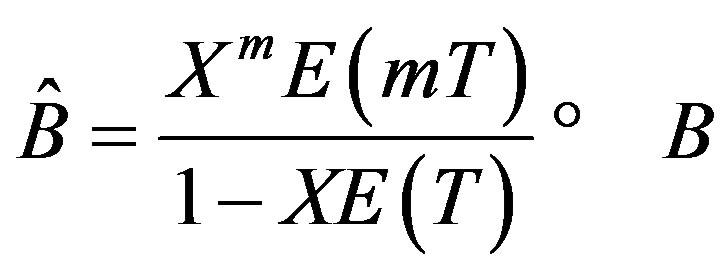
corresponds to linear runs of size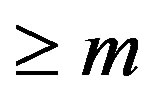 . Here,
. Here,
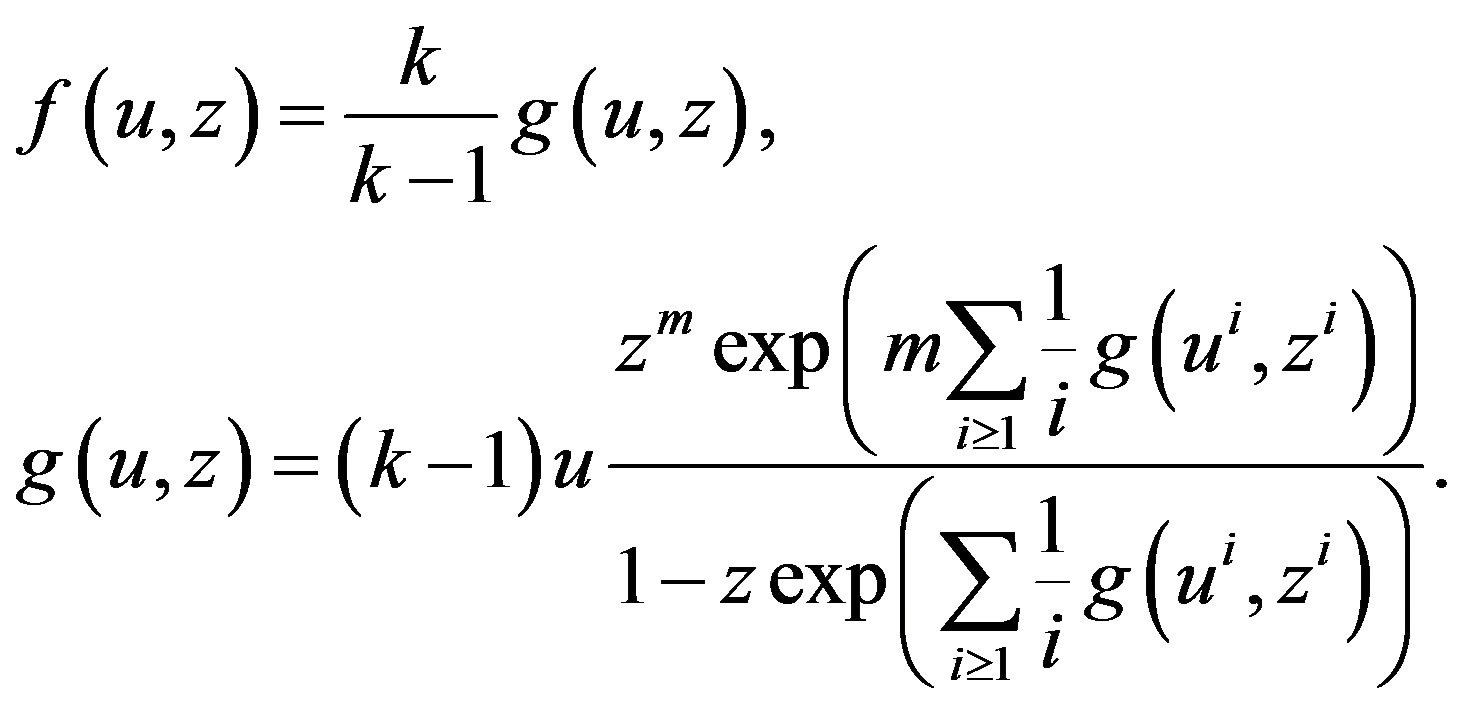 (31)
(31)
• For Cayley arborescences, the Fibonacci case, k = 2, m = 2, corresponds to the runs selector 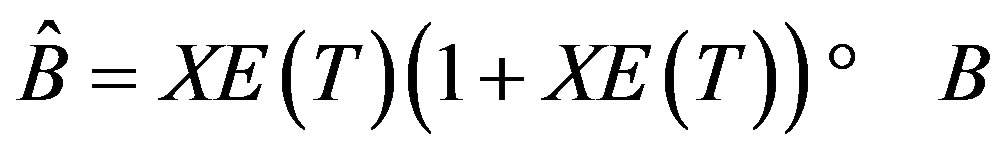 . So that,
. So that,
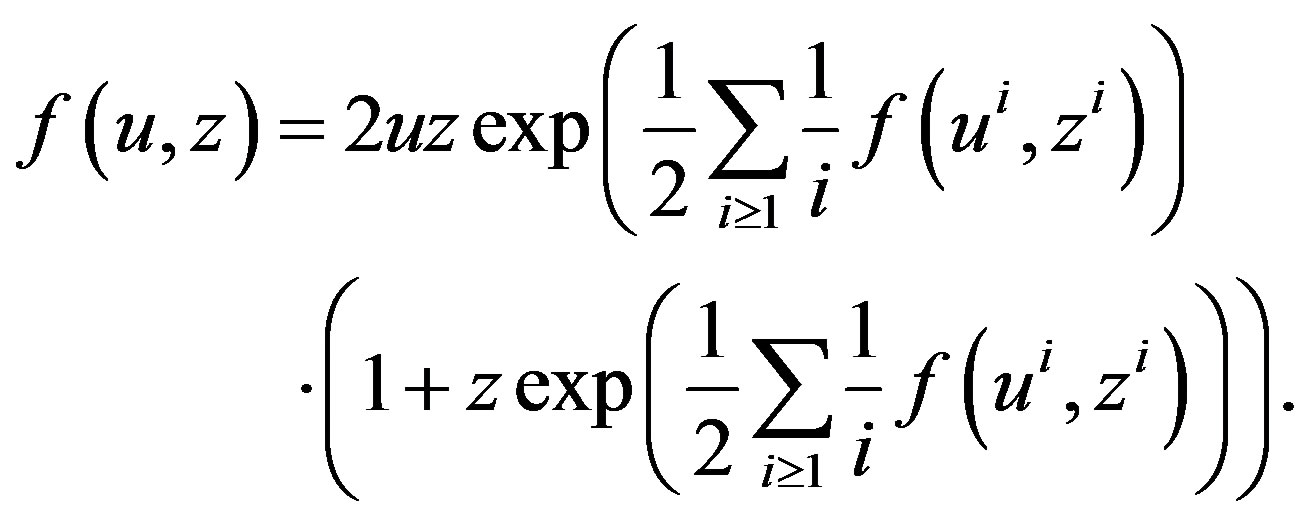 (32)
(32)
• For singleton runs (case (iv) above), 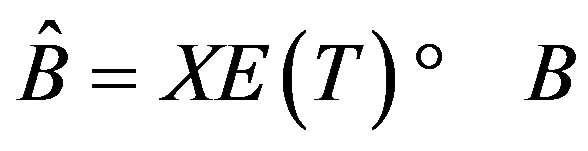 . So that,
. So that,
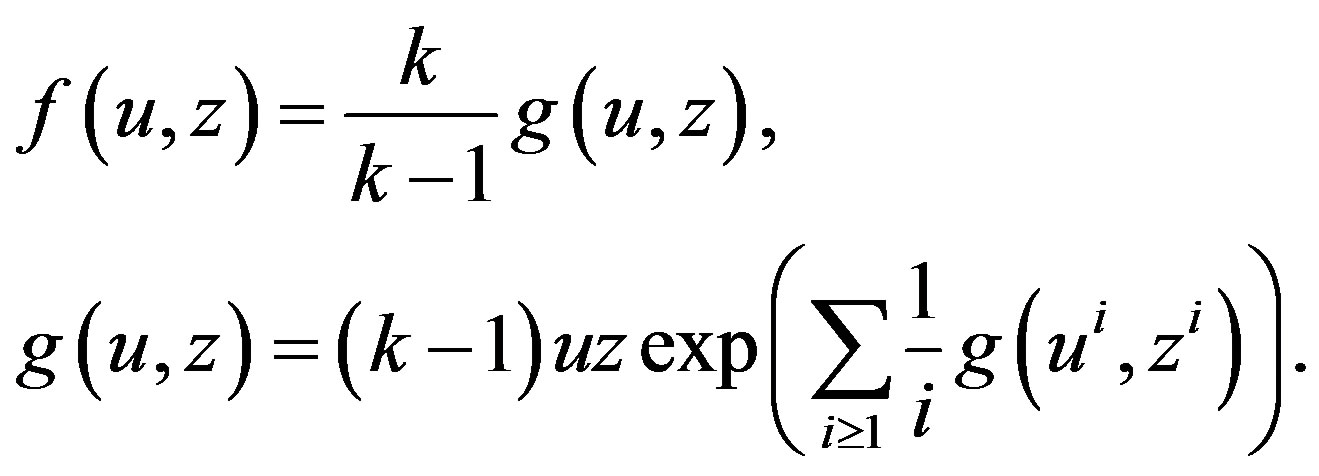 (33)
(33)
• For maximal runs (case (v) above), 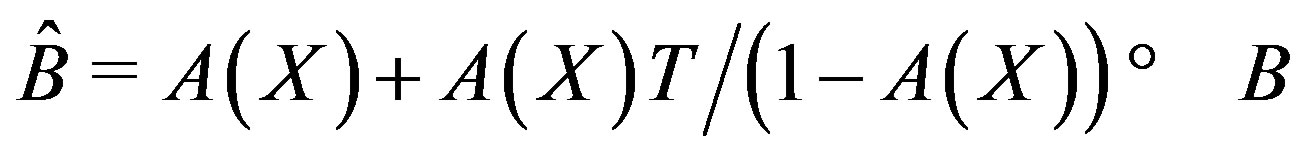 , since
, since 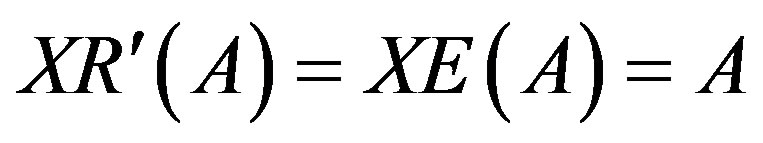 . So that,
. So that,
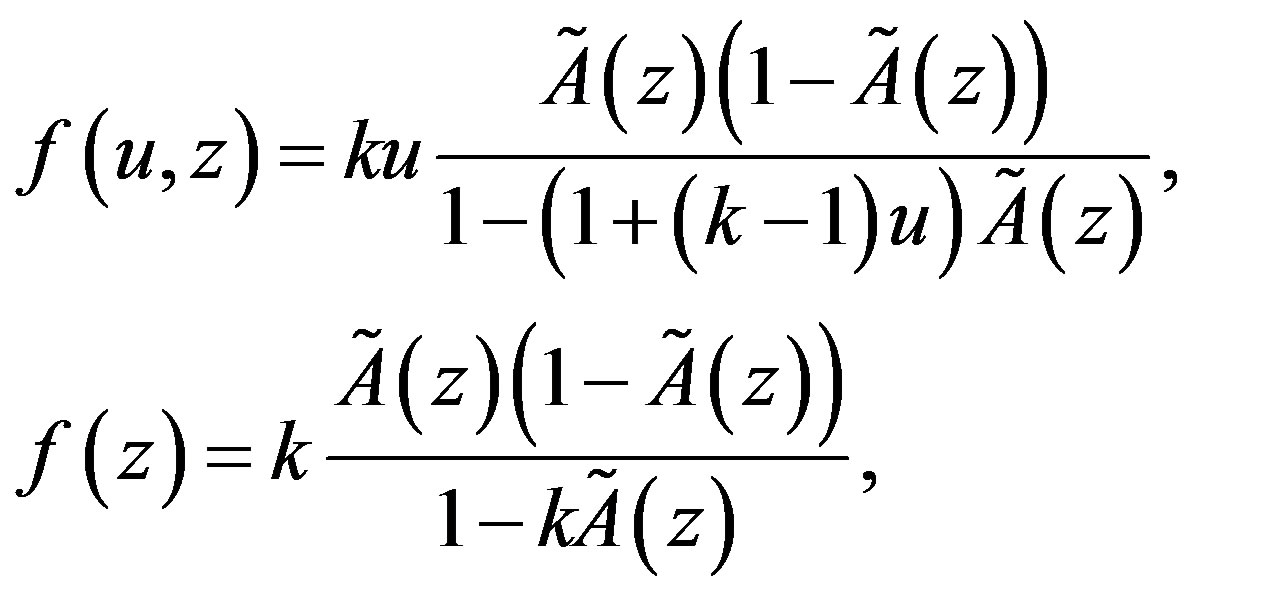 (34)
(34)
where 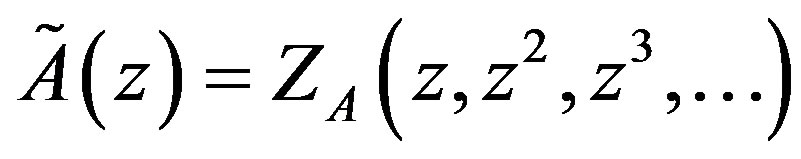 is the ordinary generating series of unlabelled Cayley arborescences.
is the ordinary generating series of unlabelled Cayley arborescences.
3.4. Runs in Mobile Words
Recall that with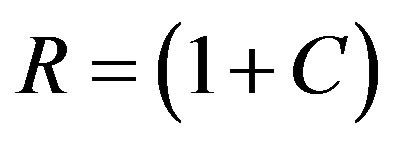 , where
, where 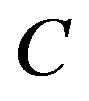 is the species of cyclic permutations, Equation (3) takes the form
is the species of cyclic permutations, Equation (3) takes the form 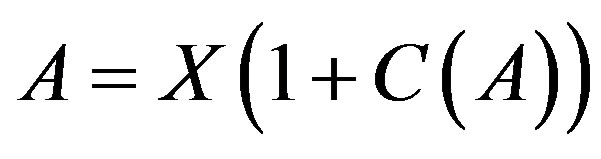 whose solution is the species
whose solution is the species 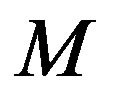 of mobiles. In this case,
of mobiles. In this case, 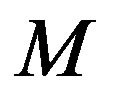 -words are called mobile words (see Figure 4(b)). Since
-words are called mobile words (see Figure 4(b)). Since
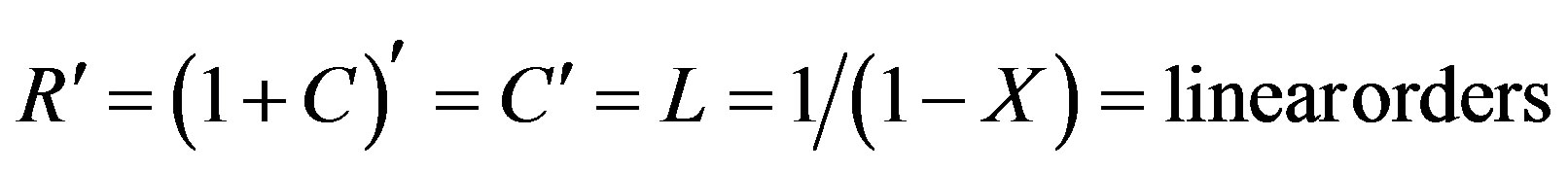 the above case (ii) of linear runs, for example, corresponds to the runs selector,
the above case (ii) of linear runs, for example, corresponds to the runs selector,
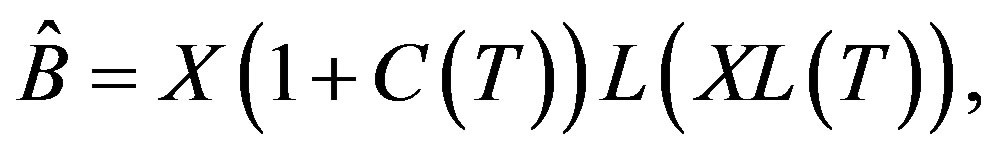 (35)
(35)
and the generating series, 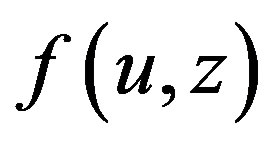 , of mobile words having only linear runs, weighted by the run counter
, of mobile words having only linear runs, weighted by the run counter 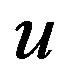 is given by,
is given by,
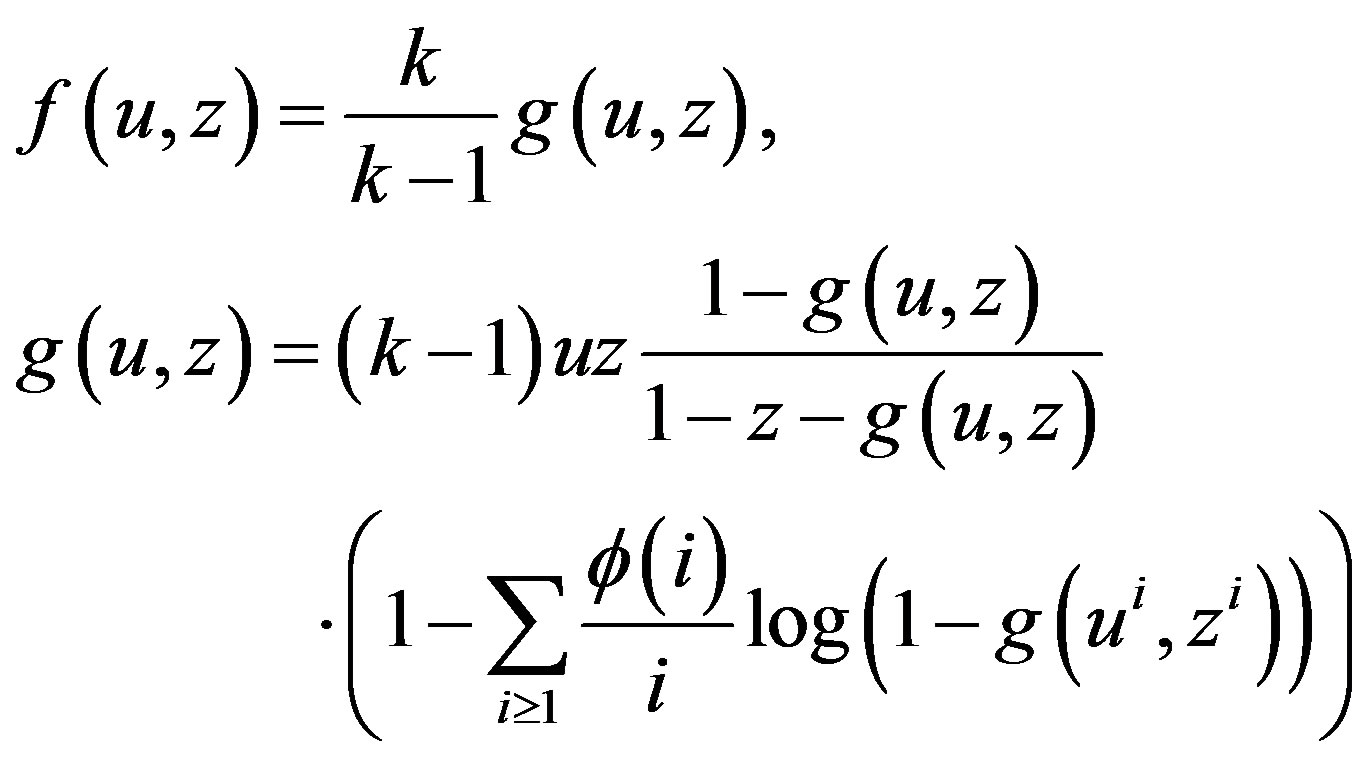 (36)
(36)
since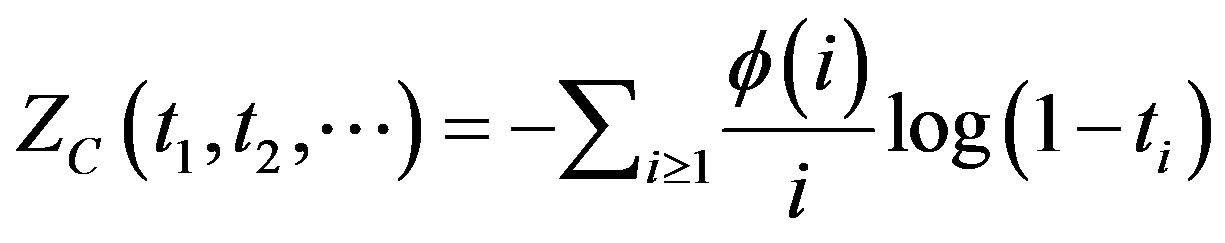 , where
, where 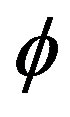 is the Euler function. For example, the first terms of
is the Euler function. For example, the first terms of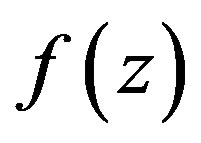 , for a 2-letter alphabet
, for a 2-letter alphabet 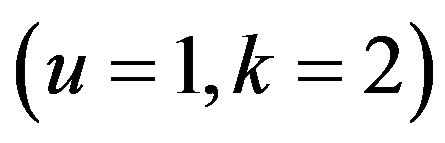 are given by,
are given by,

4. Some Extensions
The present approach to runs in structured words can be extended in various directions. For example, a probabilistic analysis of runs can be made by adding a probability of occurence  to letter
to letter  in the alphabet
in the alphabet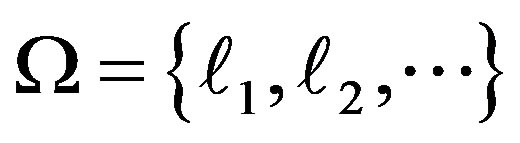 . Runs in other classes of graphical words can also be considered. For example, the class of
. Runs in other classes of graphical words can also be considered. For example, the class of  - enriched tree words is a tractable one. Here, the species
- enriched tree words is a tractable one. Here, the species 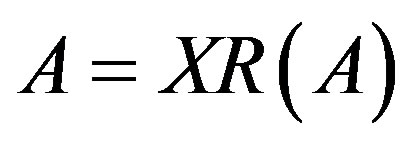 of
of 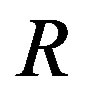 -enriched arborescences is replaced by the species
-enriched arborescences is replaced by the species  of
of 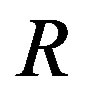 -enriched trees. This case needs a more complex analysis since extra symmetries of the structures have to be taken into account (the structures being unrooted). The (enriched) dissymmetry formula,
-enriched trees. This case needs a more complex analysis since extra symmetries of the structures have to be taken into account (the structures being unrooted). The (enriched) dissymmetry formula,
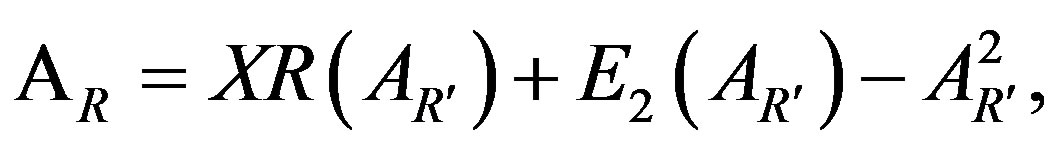 (37)
(37)
which expresses the species 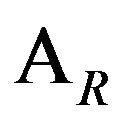 of
of 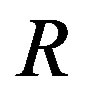 -enriched trees in terms of the species
-enriched trees in terms of the species  of
of 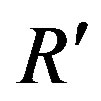 -enriched arborescences (see Bergeron, Labelle, and Leroux [7]), is the tool to be used for the analysis of the multisort species
-enriched arborescences (see Bergeron, Labelle, and Leroux [7]), is the tool to be used for the analysis of the multisort species 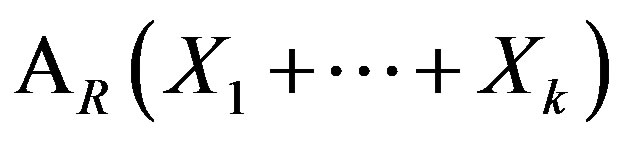 leading to an analogue of Proposition 4 for
leading to an analogue of Proposition 4 for 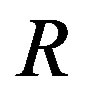 -enriched tree words. These extensions will be developed elsewere.
-enriched tree words. These extensions will be developed elsewere.
5. Acknowledgements
The authors would like to thank Jérôme Tremblay for his LaTeX and Maple help.
REFERENCES
- W. Feller, “An Introduction to Probability Theory and Its Applications: Vol. I,” 3rd Edition, John Wiley & Sons Inc., New York, 1968.
- A. M. Mood, “The Distribution Theory of Runs,” Annals of Mathematical Statistics, Vol. 11, No. 4, 1940, pp. 367- 392. doi:10.1214/aoms/1177731825
- M. V. Koutras, G. K. Papadopoulos and S. G. Papastavridis, “Runs on a Circle,” Journal of Applied Probability, Vol. 32, No. 2, 1995, pp. 396-404. doi:10.2307/3215295
- A. Joyal, “Une Théorie Combinatoire des Séries Formelles,” Advances in Mathematics, Vol. 42, No. 1, 1981, pp. 1-82. doi:10.1016/0001-8708(81)90052-9
- A. Joyal, “Foncteurs Analytiques et Espèces de Structures,” In: Combinatoire Énumérative (Montreal, Quebec, 1985), Vol. 1234 of Lecture Notes in Mathematics, Springer, Berlin, 1986, pp. 126-159.
- G. Labelle, “Une Nouvelle Démonstration Combinatoire des Formules D’inversion de Lagrange,” Advances in Mathematics, Vol. 42, No. 3, 1981, pp. 217-247. doi:10.1016/0001-8708(81)90041-4
- F. Bergeron, G. Labelle and P. Leroux, “Combinatorial species and tree-like structures, Vol. 67 of Encyclopedia of Mathematics and its Applications,” Cambridge University Press, Cambridge, 1998.
- G. Labelle, “Éclosions Combinatoires Appliquées à L'inversion Multidimensionnelle des Séries Formelles,” Journal of Combinatorial Theory, Series A, Vol. 39, No. 1, 1985, pp. 52-82. doi:10.1016/0097-3165(85)90083-4
- G. Labelle, “Some New Computational Methods in the Theory of Species,” In Combinatoire Énumérative Lecture Notes in Mathematics Vol. 1234, Springer, Berlin, 1986, pp. 192-209.
- P. Erdös and P. Révész, “On the Length of the Longest Head-Run,” In Topics in Information Theory (Second Colloq., Keszthely, 1975), Colloquia Mathematica Societatis János Bolyai, Vol. 16, North-Holland, Amsterdam, 1977, pp. 219-228.
- L. J. Guibas and A. M. Odlyzko, “Long Repetitive Patterns in Random Sequences,” Zeitschrift für Wahrscheinlichkeitstheorie und Verwandte Gebiete, Vol. 53, No. 3, 1980, pp. 241-262. doi:10.1007/BF00531434
- Y. Kong, “Distribution of Runs and Longest Runs: A New Generating Function Approach,” Journal of the American Statistical Association, Vol. 101, No. 475, 2006, pp. 1253-1263. doi:10.1198/016214505000001401

