Z. YIN
39
1
1
shock point and the spin axis of the tangent plane (Equa-
torial vertical plane), shown as EVP.
Longitudinal ecliptic plane: after a shock point and
the yellow surface of the Earth axis tangent plane (Eclip-
tic plane vertical), shown as EPV.
Circular symmetry point: Circular , shown as CSP.
Similar point: Similar Point, shown as SP.
Earthquake effects: measure the Earth’s surface; the
intensity level of shock is unstable, the stability of the
boundary tangent plane level. Plane is the ball away from
the more deviation, the more poor results, and the more
unstable and more likely point of this earthquake.
3. Basic Assumptions and the Co-Planarity
and Symmetry Principle of Earthquake
3.1. Basic Assumptions
1) The Earth became positive sphere with earthquakes
which rise or low the ground. That is, because of the ecli-
ptic (23.26 degrees), the Ecliptic Plane TPPTE line tan-
gent planes ELTP have tended to be round;
2) The Earth has tended to balance, and because the
earthquake, to re-balance;
3) Crustal thickness tends to vary. Earth’s core and
mantle movement space tends to a ball space;
4) With the earthquake, the earth rotation axis offset.
When there is a earthquake which makes the low rise, the
earth rotation axis moves to the seismic vertical, those
makes the high low is opposite.
3.2. Co-planarity and Symmetry Principle
After the observation of earthquakes on Earth N (154
earthquakes over 6 scales of magnitude in this article)
times, it is occur time of adjacent earth-
quakes measure with UTC.
KK
H,H
KK
M,M
A) The previous earthquake is a cause of the after. The
tangent plane balances was destroyed, then tend to a new
balance, which Ecliptic line tangent plane generate new
state plane all the times.
B) Seismic vertical and seismic vertical
are on the same plane at the time or . That is
parallel to the cut-plane in the equatorial plane or Eclip-
tic line cut the ecliptic plane, or longitudinal vertical
plane or equatorial plane.
K
MK+1
M
K
HK+1
H
C) If the tangent of the plane where seismic vertical
and seismic vertical are tends to round, or
fall-rise movement in the earthquake tends to stable,
and tends to symmetry in the circle; other-
wise, coplanar circle from the difference of the most un-
stable point will have an earthquake.
K
M
K
M
K+1
M
K+1
M
D) After earthquake on occurs, if the most unsta-
ble point (poor seismic effect) is still, earthquake
will take place near the same point.
K
M
K
M
K+1
M
4. Research Basis and Calculation Method
Assumptions (1), (2), (3) are prerequisite of conclusion
(A), (B), (C) and (D); symmetry points including the spin
axis symmetry, yellow axis symmetry and geocentric
axial symmetry. With the existence of the ecliptic obliq-
uity (ecliptic plane and the equatorial plane angle 23.26
degrees) and the Ecliptic plane tangent line, parallel to
the equatorial plane tangent tends to a circle, the shape of
the earth tends to a sphere becomes possible. This can be
concluded with (C) and (D). Assumptions (4) decided the
longitudinal movement of the after . As-
sumptions (1) and (3) show the possibility that the
earthquake and the earthquake potential basis for the
order. Be noted that symmetrical points at any time
change the fact that, in the premise revolution, a startled
different points of the Ecliptic OK UTC timing of your
cutting surface is subject to change, and cut parallel to
the equatorial plane remain unchanged.
K+1
MK
M
Earthquake time: the time the earthquake is very
important, when the earthquake occurred; it generates
and identifies Ecliptic line tangent plane, tangent plane
parallel to the equator, the ecliptic plane and the equato-
rial vertical longitudinal cut tangent plane, as well as the
corresponding point of the plane of symmetry at the
same time.
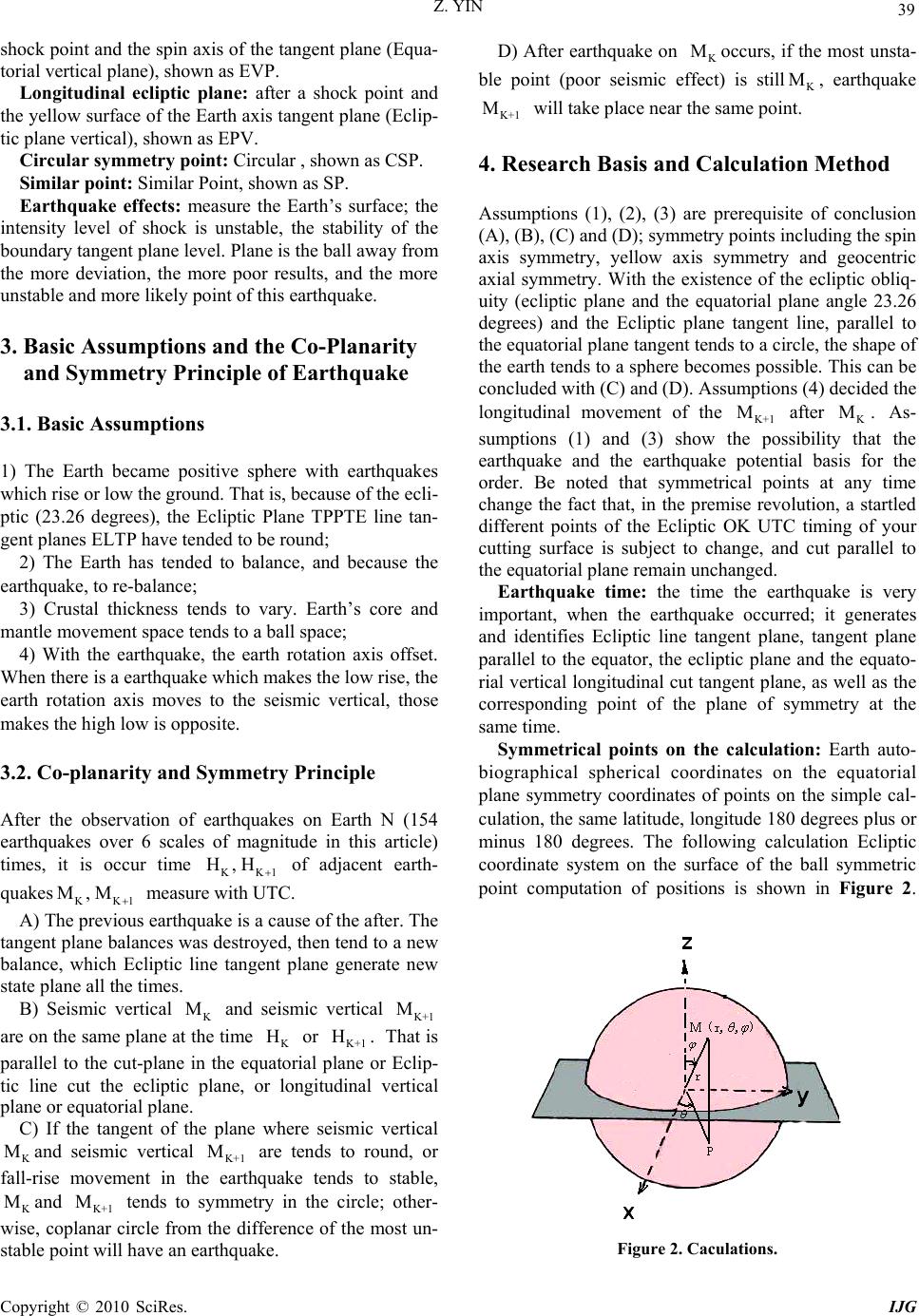
Symmetrical points on the calculation: Earth auto-
biographical spherical coordinates on the equatorial
plane symmetry coordinates of points on the simple cal-
culation, the same latitude, longitude 180 degrees plus or
minus 180 degrees. The following calculation Ecliptic
coordinate system on the surface of the ball symmetric
point computation of positions is shown in Figure 2.
Figure 2. Caculations.
Copyright © 2010 SciRes. IJG