Gravitational Model of the Three Elements Theory

doi:10.4236/jmp.2012.35054

Paper Menu >>

Journal Menu >>

Journal of Modern Physics, 2012, 3, 388-397

http://dx.doi.org/10.4236/jmp.2012.35054 Published Online May 2012 (http://www.SciRP.org/journal/jmp)

Gravitational Model of the Three Elements Theory

Frederic Lassiaille

University of Nice Sophia-Antipolis, Valbonne, France

Email: lumimi2003@hotmail.com

Received March 8, 2012; revised March 25, 2012; accepted April 15, 2012

ABSTRACT

The gravitational model of the three elements theory is an alternative theory to dark matter. It uses a modification of

Newton’s law in order to explain gravitational mysteries. The results of this model are ex planations for the dark matter

mysteries, and the Pioneer anomaly. The disparity of the gravitational constant measurements might also be explained.

Concerning the Earth flyby anomalies, the theoretical order of magnitude is the same as the experimental one. A very

small change of the perihelion advance of the planet orbits is calculated by this model. Meanwhile, this gravitational

model is perfectly compatible with restricted relativity and general relativity, and is part of the three element theory, a

unifying theory.

Keywords: Relativity; Gravitation; Newton

1. Introduction

This article addresses an issue yielded by general relati-

vity, which is a determination of a global space’s shape.

This absolute space-time is the general relativity space-

time, and this space deformation in space-time must be in

conformity with Newton’s law at least for long distances.

The adopted point of view is a Euclidean relativity. A

Euclidean mathematical context is used, with 4 dimen-

sions (three of space, x, y, z, and one of time: ct). This is

used for restricted relativity. For general relativity, of

course we apply the same and we extend it with a tensor,

except that here locally it is a Euclidean metric used to

represent space-time. In one word, a Riemannian tensor

is used, in place of the usual pseudo-Riemannian Min-

kowskian tensor.

The physics principles within this mathematical frame-

work will be exactly the principles of relativity. There

exists, however, a difference between relativity and the

approach of this document. Indeed, constancy of space-

time distance, in a global representation of space-time.

Minkowski’s representation is not used, and the Lor-

entz’s invariant length is left off.

At first, the method is postulating th at Lorentz’s equa-

tions are simply a consequence of space-time deforma-

tions by energy. In other words we try to express the

general relativity “deformation” principle, in the context

of restricted relativity. Once this is done, physics incon-

sistencies are revealed. Of course a solution is searched.

This will finally lead to postulate the existence of indi-

visible particles, from which matter is made of. Thus, the

space-time determination is calculated, by means of rela-

tivity energy equation. By construction, this determina-

tion is coherent with Lorentz’ s equations. This is the fi-

nal determination of the shape of global space inside

space-time. After this theoretical construction, this arti-

cle will describe some results of this gravitational

model:

 Galaxy speed profiles mystery,

 Galaxy velocit y my st ery ,

 Pioneer anomaly,

 Saturn flyby by Pioneer 11,

 Earth flyby anom alies,

 Perihelion advance or precession of Mercury and

Saturn,

 Disparity of gravitational constant measurements.

The detailed about this article can be found in [1-3].

Reference [1] was the first article about the gravitational

model of the three elements theory. It describes the theo-

retical basis of the model. It has been published in the

17th NPA proceedings, vixra database, and on the site of

the three elements theory, since 2010. It has also been

published on Amazon site. Reference [2] was only pub-

lished on the site of the three elements theory. But fig-

ures where extracted from it for publishing in the pro-

ceedings below. Reference [3] was published on the site

of the three elements theo ry, vixra database, and leads to

a short version of it, which is in progress in the fo llowing

proceedings: 2011 Dark matter symposium (poster), 18th

NPA proceedings, PIRT 2011, ECLA 2011, FFP12, and

may be SMFNS2011, STARS2011, and the 8th Interna-

tional Conference on Progress in Theoretical Physics.

These “proceeding versions” are also reminding briefly

the content of [1], and using some figures of [1].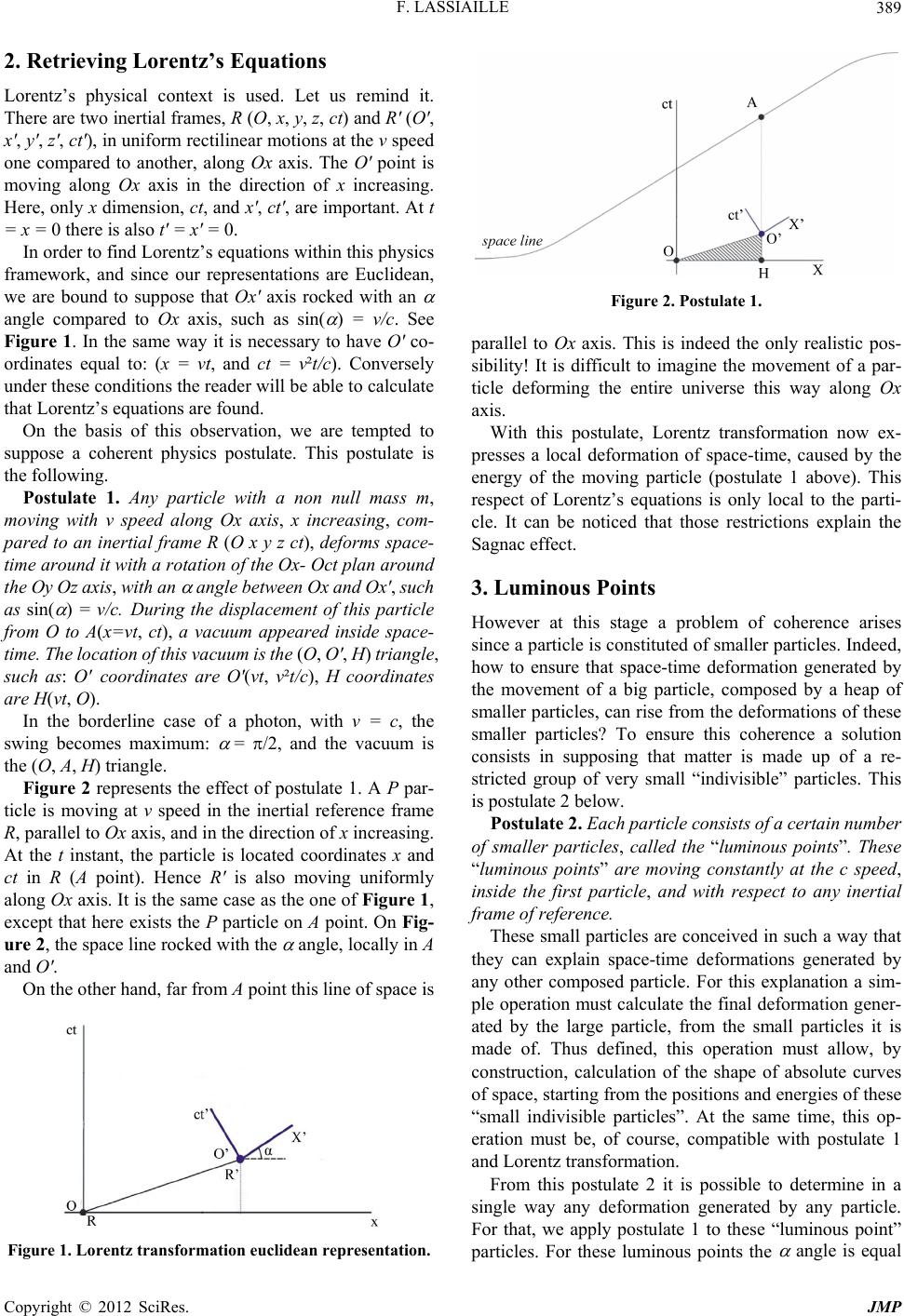

F. LASSIAILLE 389

2. Retrieving Lorentz’s Equations

Lorentz’s physical context is used. Let us remind it.

There are two inertial frames, R (O, x, y, z, ct) and R' (O',

x', y', z', ct'), in uniform rectilinear motions at the v speed

one compared to another, along Ox axis. The O' point is

moving along Ox axis in the direction of x increasing.

Here, only x dimension, ct, and x', ct', are important. At t

= x = 0 there is also t' = x' = 0.

In order to find Lorentz’s equations within this ph ysics

framework, and since our representations are Euclidean,

we are bound to suppose that Ox' axis rocked with an



angle compared to Ox axis, such as sin(



) = v/c. See

Figure 1. In the same way it is necessary to have O' co-

ordinates equal to: (x = vt, and ct = v²t/c). Conversely

under these conditions the reader will be able to calcu late

that Lorentz’s equations are found.

On the basis of this observation, we are tempted to

suppose a coherent physics postulate. This postulate is

the following.

Postulate 1. Any particle with a non null mass m,

moving with v speed along Ox axis, x increasing, com-

pared to an inertial frame R (O x y z ct), deforms space-

time around it with a rotation of the Ox- Oct plan around

the Oy Oz axis, with an



angle between Ox and Ox', such

as sin(



) = v/c. During the displacement of this particle

from O to A(x=vt, ct), a vacuum appeared inside space-

time. The location of this vacuum is the (O, O', H) triangle,

such as: O' coordinates are O'(vt, v²t/c), H coordinates

are H(vt, O).

In the borderline case of a photon, with v = c, the

swing becomes maximum:



= /2, and the vacuum is

the (O, A, H) triangle.

Figure 2 represents the effect of postulate 1. A P par-

ticle is moving at v speed in the inertial reference frame

R, parallel to Ox axis, and in the direction of x increasing.

At the t instant, the particle is located coordinates x and

ct in R (A point). Hence R' is also moving uniformly

along Ox axis. It is the same case as the one of Figure 1,

except that here exists the P particle on A point. On Fig-

ure 2, the space line rocked with the



angle, locally in A

and O'.

On the other h a nd, far from A point this line of space is

Figure 1. Lorentz transformation euclidean representation.

Figure 2. Postulate 1.

parallel to Ox axis. This is indeed the only realistic pos-

sibility! It is difficult to imagine the movement of a par-

ticle deforming the entire universe this way along Ox

axis.

With this postulate, Lorentz transformation now ex-

presses a local deformation of space-time, caused by the

energy of the moving particle (postulate 1 above). This

respect of Lorentz’s equations is only local to the parti-

cle. It can be noticed that those restrictions explain the

Sagnac effect.

3. Luminous Points

However at this stage a problem of coherence arises

since a particle is constituted of smaller particles. Indeed,

how to ensure that space-time deformation generated by

the movement of a big particle, composed by a heap of

smaller particles, can rise from the deformations of th ese

smaller particles? To ensure this coherence a solution

consists in supposing that matter is made up of a re-

stricted group of very small “indivisible” particles. This

is postulate 2 below.

Postulate 2. Each particle consists of a certain number

of smaller particles, called the “luminous points”. These

“luminous points” are moving constantly at the c speed,

inside the first particle, and with respect to any inertial

frame of reference.

These small particles are conceived in such a way that

they can explain space-time deformations generated by

any other composed particle. For this explanation a sim-

ple operation must calculate the final deformation gener-

ated by the large particle, from the small particles it is

made of. Thus defined, this operation must allow, by

construction, calculation of the shape of absolute curves

of space, starting from the positions and energies of these

“small indivisible particles”. At the same time, this op-

eration must be, of course, compatible with postulate 1

and Lorentz transformation.

From this postulate 2 it is possible to determine in a

single way any deformation generated by any particle.

For that, we apply postulate 1 to these “luminous point”

particles. For these luminous points the



angle is equal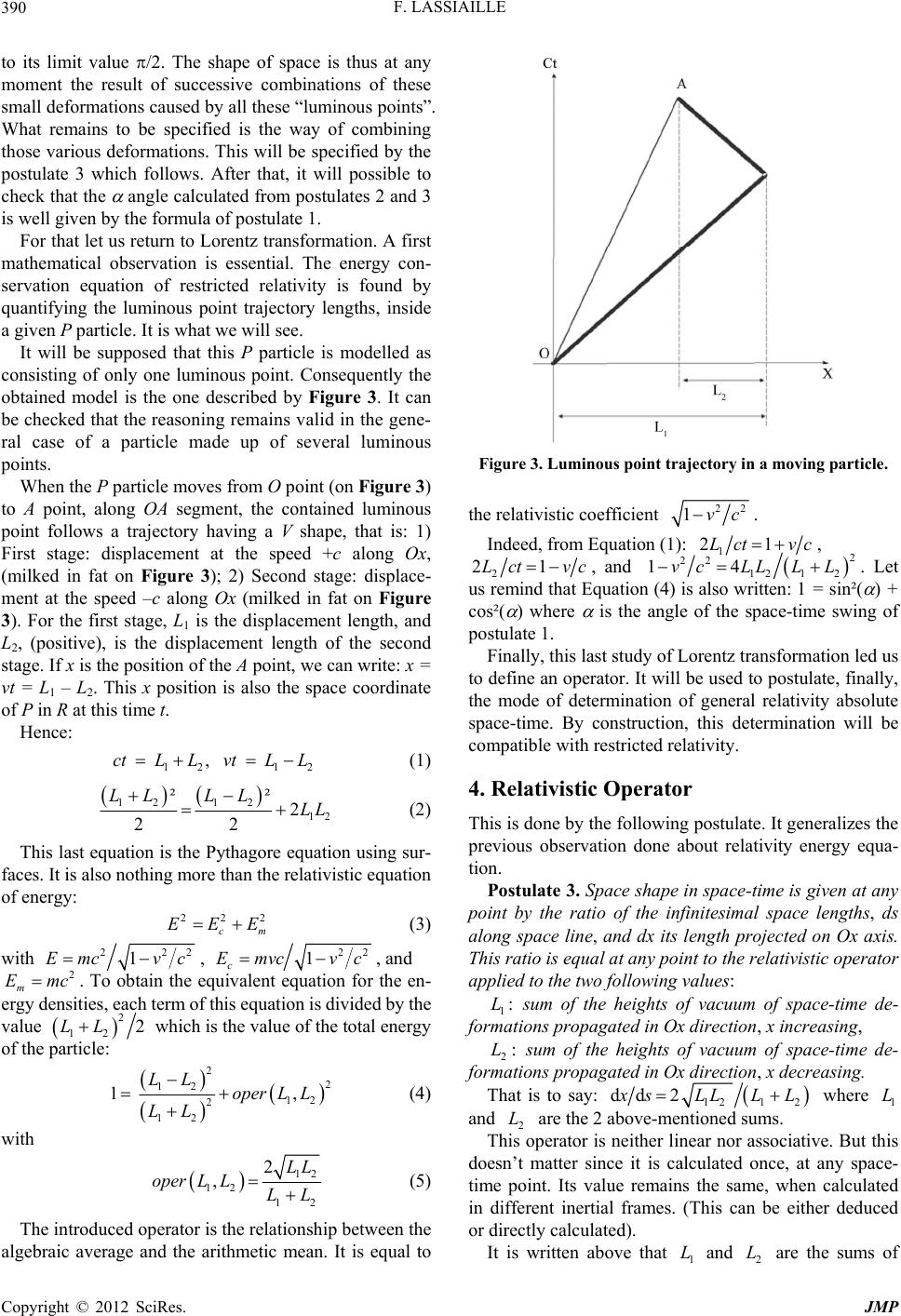

F. LASSIAILLE

390

to its limit value /2. The shape of space is thus at any

moment the result of successive combinations of these

small deformations caused by all these “luminou s poin ts”.

What remains to be specified is the way of combining

those various deformations. This will be specified by th e

postulate 3 which follows. After that, it will possible to

check that the



angle calculated from postulates 2 and 3

is well given by the formula of postulate 1.

For that let us return to Lorentz transformation. A first

mathematical observation is essential. The energy con-

servation equation of restricted relativity is found by

quantifying the luminous point trajectory lengths, inside

a given P parti cle. It is what we will see.

It will be supposed that this P particle is modelled as

consisting of only one luminous point. Consequently the

obtained model is the one described by Figure 3. It can

be che cked that the r easo nin g r ema in s v al id in the ge ne-

ral case of a particle made up of several luminous

points.

When the P particle moves from O point (on Figure 3)

to A point, along OA segment, the contained luminous

point follows a trajectory having a V shape, that is: 1)

First stage: displacement at the speed +c along Ox,

(milked in fat on Figure 3); 2) Second stage: displace-

ment at the speed –c along Ox (milked in fat on Figure

3). For the first stage, L1 is the displacement length, and

L2, (positive), is the displacement length of the second

stage. If x is the position of the A point, we can write: x =

vt = L1 – L2. This x position is also the space coordinate

of P in R at this time t.

Hence:

12 1

,ct LLvt LL  2

(1)



12 1212

²²

LL LLLL

 

 (2)

This last equation is the Pythag ore equation using sur-

faces. It is also nothing more than the relativistic equation

of energy:

EEE 2

(3)

with 222

1Emc vc, 22

Emvc vc, and

. To obtain the equivalent equation for the en-

ergy densities, each term of th is equation is divided by t he

value

Emc



122LL which is the valu e of the to tal energ y

of the particle:



 

12 12

LL oper LL



 





(4)

with



12 12

,LL

oper LL LL

  (5)

The introduced o perator is the relationship between the

algebraic average and the arithmetic mean. It is equal to

Figure 3. Luminous point trajectory in a moving particle.

the relativistic coefficient 22

1vc.

Indeed, from Equati on (1): 1

21Lct vc ,

21Lctvc



 , and



22 12 12

14vcLLL L . Let

us remind that Equation (4) is also written: 1 = sin²(



) +

cos²(



) where



is the angle of the space-time swing of

postulate 1.

Finally, this last study of Lorentz transformatio n led us

to define an o perator. It will be used to postulate, finally,

the mode of determination of general relativity absolute

space-time. By construction, this determination will be

compatible with restricted relativity.

4. Relativistic Operator

This is done by the following postulate. It generalizes the

previous observation done about relativity energy equa-

tion.

Postulate 3. Space shape in space-time is given at any

point by the ratio of the infinitesimal space lengths, ds

along space line, and dx its length projected on Ox axis.

This ratio is equal at any point to the relativistic operator

applied to the two following values:

1: sum of the heights of vacuum of space-time de-

formations propagated in Ox direction, x increasing

2: sum of the heights of vacuum of space-time de-

formations propagated in Ox direction, x decreasing.

That is to say:



121 2

dd 2

sLLLL



 where

and are the 2 above-ment i oned sums. 1

This operator is neither linear n or associative. But this

doesn’t matter since it is calculated once, at any space-

time point. Its value remains the same, when calculated

in different inertial frames. (This can be either deduced

or directly calculated).

It is written above that and are the sums of

F. LASSIAILLE 391

the heights of vacuum of the “propagated deformations”.

It is necessary to describe how these “space-time defor-

mations” are propagated. The mechanism is very similar

to waves propagated by the movement of a boat over a

water surface. Let us consider Figure 1. The initial de-

formation relates to the Ox-Oct plan. The propagations of

this deformations in space-time are carried out on re-

maining space dimensions, i.e. Oy and Oz, more gener-

ally on any Or direction, half-line based on O and con-

tained in the Oy-Oz plan. The form of these propagated

deformations is each time exactly the same as the one of

the initial deformation. The initial deformation was done

on Ox-Oct plan (space-time swing represented Figure 1).

Now the propagated deformation is the same but it re-

lates to the (Ox+Or)-Oct plan in place of Ox-Oct plan.

The height of this propagated deformation attenuates

progressively as r increases. The attenuation law, g, will

be given further. At each moment, the “luminous point”

thus emits this deformation. Therefore, like in the case of

the boat, the finally overall propagated deformation is th e

envelope of all these propagated deformations. (In the

case of a boat this envelope has a V shape which is the

final shape of these waves over the water surface). Here

this envelope is a cone whose axis is Ox axis.

Let us study the overall result of all the propagated

deformations which are received in the same M point at

the same moment. The postulate 3 above expresses the

length ratio along Ox axis only. But at one M point there

are numerous such directions coming to. Hence it is ne-

cessary to calculate the relativistic operator of postulate 3

for each space direction. The final deformation is then

obtained. The only qu estion is: “What is the combination

rule for the deformations of all these directions in order

to obtain the result?” This result will be the resulting

space-time deformation at M point. It will be thus neces-

sary to generalize the above operator with a second more

generic operator, which will take in to account each sp ace

directions. The result given by this second generalized

operator must be the famous final space-time deforma-

tion at M point. It will be probably useful to use a mathe-

matical base like the quaternions for that. In this article

this complexity will not be seen because fortunately not

necessary.

We thus found relativity starting from postulates 1, 2,

and 3. The



angle of the postulate 1 rotation is calcu-

lated, by applying postulates 2 and 3. Overall, we ob-

tained a way for calculating space shape inside space-

time. We can now study Newton’s law.

5. Modification of Newton’s Law

The studied case is a particle of mass M isolated in a

space filled uniformly with a constant energy density.

The particle coordinates are x = y = z = 0, which are

those of the O point in our usual inertial frame R of re-

ference. The studied case being invariant by any rotation

of center O, only the Ox axis with x > 0, and the axis of

times Oct, are important.

How does evolve the local slope tan(



 of space, along

Ox axis? The postulate 3 above is applied. Let us con-

sider a space-time P point to which comes at least one

deformation from a luminous point pertaining to the M

mass. We suppose P x-coordinate positive strict that is x

> 0. The M mass particle propagates on P point the fol-

lowing defo rmations, with pr opagation directions give n:





Lgx x increasing (6)



x decreasing (7)

For Equation (6), g is the attenuation function given

further. There is no deformation propagated in the direc-

tion of x decreasing, coming from M, because x > 0. The

surrounding universe with constant energy density propa-

gates on P point the following deformations, with pro-

pagation directions given:



x increasing (8)



x decreasing (9)

Therefore, the and sums are the following.





11 1umu

LL LLg



 (10)

22 2um

LL LL



 (11)



d(),

xoperLg xL





 (12)

The last equation is the application of postulate 3. Af-

ter calculations:



cos( )1

d8u



  (13)

In addition let’s ap ply the formula of the expr ession of

a force, to an m mass moving. The traditional relativistic

equation is the following one:









(14)

This is a very classical relativity result. Now let us

take the case of a particle with a negligible mass at rest.

It is with this particular case that is applied the principle

of general relativity: the trajectory of this particle will

follow a space-time geodesic. Moreover, if the particle is

located at rest infinitively far at t = 0, then we have, for

any x, v = ctan (



), where



is the slope angle of the re-

quired curve ct = h(x). This curve is the searched space

curve. From where:



232

dtan( )tan( )

d1tan()

Fmc x





 (15)

F. LASSIAILLE

392

For x large, this must be equal to 2

mMG x ,

which is Newton’s equation. Hence, after calculation, we

have, for x large:

() u

gx L

 (16)

With 2

RMGc . Now it will be postulated that this

Equation (16) is correct not only for long distances but

for any values of x. It will be supposed also that space is

no longer filled uniformly with a constant energy density.

Let us write f as being the normalized space-time height

of the propagated deformations which are coming from

the closed surrounding matter. For example, in the case

of a galaxy, this f contribution will comes from the gal-

axy’s stars. It will be us ed also s = 1 + f, where 1 stands

here for the normalized contribution coming from the

universe. With this notation, the and sums be-

comes the following. 1

LL f



 









(17)







 (18)

After calculations, Equation (15) becomes the fol-

lowing.

=²882

ssx

mMG

FxRRR

sss s

xxx





 







 













(19)

In this equation, G' stands for the value of G valid for

long distances and in extra-galactic locations, and R' is

equal to MG'/c2. It must be noticed that, if f = 0, it still

remains a correction of Newton’s law expressed by

Equation (19). But this modification is only noticed for x

close to the Schwarzschild radius (2R').

In this document, if f = 0 then this Newton’s law

modification will be called the “first modification of

Newton’s law”. If f



0, it will be called the “second

modification of Newton’s law”.

6. The Pioneer Anomaly

This modification of Newton’s law has been using a

fitting of Newton’s law for long distances. But for

studying the Pioneer anomaly, this fitting must not be

done for long distances. It must be done for a distance

from the sun where the heliocentric gravitational constant

is known to be perfect. This value is around the sun to

Saturn distance. This can be seen on the measured curve

of the Pioneer anomaly, in [4]. With this supposition the

calculations yields a theoretical anomaly value equal to

10 2

7.2510 ms

A

 , in place of the measured value

10 2

8.7410 ms

A

 . But the shape of the theoretical

curve is not perfect. Figure 4 shows this theoretical

curve. It has been plotted using Equation (19) and the

“first mo dification of Newton ’s law”.

There is also a negative predicted anomaly for the probe’s

trajectory between the sun and Saturn (x-coordinate

lesser than 10 AU). This work is in progress, and notice-

ably exact comparison with ephemerides must be done.

In order to retrieve the perfect curve, it must be

supposed that f is not null nor constant but varies (“sec-

ond modification of Newton’s law”). The Kuiper belt

will be taken into account. The Kuiper belt is a belt of

asteroids located beyond the location of Saturn , along the

ecliptic plane. Now the result, on Figure 5, is very

encouraging. On this theoretical curve, the maximum

value is exactly 10 2

8.7410ms

A

 , the measured

value. But this theoretical curve has been obtained with

fitted values for the Kuiper belt space-time deformations

contributions. On the contrary, the curve of Figure 4 was

calculated without any fitting.

Figure 4. Theoretical curve of the Pioneer anomaly, using

“first modification of Newton’s law”. X-coordinate is the

distance from the sun, in AU, y-coordinate is the added ac-

celeration toward the sun, in 10–10 m/s2. This theoretical

curve must be compared with the experimental one located

in [4].

Figure 5. Theoretical curve of the Pioneer anomaly, using

the Kuiper belt, and the “second modification of Newton’s

law” for calculation. X-coordinate is the distance from the

sun, in AU, y-coordinate is the added acceleration toward

the sun, in 10–10 m/s2.

F. LASSIAILLE 393

7. The Pioneer 11 Flyby of Saturn

When applying this gravitational model to the case of

Pioneer 11 trajectory, an added anomaly is found. It is an

acceleration anomaly during the flyby of Saturn. The

model is calculating an anomalous decrease before the

Saturn encounter, and an anomalous increase after the

encounter. This is shown on Figure 6. An important pa-

rameter has been fitted. It is 0

, the distance at which the

Saturn gravitational constant,

GM , is perfect (

stands for Saturn mass). Indeed, in this gravitational

model, this distance is always of great importance: the

distance between the attracted masses, where Newton’s

law is perfect.

The values of Figure 6 are very strong, as compared to

the Pioneer an omaly values, but the distanc es range of this

anomaly is very short. This range is roughly [9.550 AU,

9.555 AU]. It has been checked that the global Pioneer

anomaly curve is still correct for this Pioneer 11 travel,

after taking into account this Saturn flyby anomaly. In fact,

this global curve shape depends strongly on the 0

value.

8. Speed Profile Mystery

In order to obtain the explanation of the stars speed in a

galaxy it is necessary to take into account these star

masses. For this, a P point is supposed located in the

middle of these stars i.e. inside the studied galaxy. It will

be supposed that the deformation propagated by these

stars and received in P is only coming from the sur-

rounding stars closed to P. This supposition is conf irmed

later on, outside of this article.

It will be supposed th at this matter density in a galaxy

evolves following a 1/x2 law. (x is the distance from the

galactic center). As a consequence, the f contribution of

Equation (19) is equal to r/x, where r is the ray from

which the gravitational effect of the surrounding stars is

noticed. With those suppositions and notations, Equation

(19) becomes:

()

FmMG



 

 (20)

Now, of course M stands for the mass of the galactic

center. It is noticed that the gravitational force can be

null, and even negative for x < r. As usual the tangential

speed of the stars is calculated using the classical equa-

tion vFxm .

This Equation (20) yields the curve located on the

bottom of Figure 7. It has been used: r = 1 kpc. This

value has been adjusted in order to obtain the best possi-

ble curve.

The variation of the speed of the stars in a galaxy is

theoretically explained. Hence we retrieve the global

Figure 6. Theoretical curve of the Pioneer 11 anomaly, in

the vicinity of Saturn. X-coordinate is the distance from the

sun, in AU, and y-coordinate is the anomalous acceleration

toward the sun, in m/s2. The represented anomaly is the

sum of the Pioneer anomaly and the Saturn flyby anomaly.

Figure 7. Theoretical speed profile for the stars in a galaxy,

calculated using a 1/x2 matter density curve. X-coordinate

represents, in kpc, the distance between the star and the

galactic center. The unit of the ordinate, y, is km/s. The

curve located on the bottom represents the speed resulting

from the new model. The curve located on the top repre-

sents the speed resulting from traditional Newton’s law.

shape of a typical galaxy speed profile.

More practically, a program calculating the NGC 7541

speed profile yields the curve of Figure 8. This curve has

been computed using the matter density profile available

in [5]. The corresponding measured speed profile of

NGC 7541 is also found in [5]. The shape of this mea-

sured curve is the same as the theoretical curve of Figure

8. But there is an issue of sign for the theoretical speed

profiles of those real galaxies. Each time, locally, the

F. LASSIAILLE

394

Figure 8. Theoretical speed profile of the NGC 7541 galaxy.

X-coordinate is in kpc, and y ordinate is a relative speed.

The sign of the gravitational force has been changed, and

the speed values has been raise d with a fitted constant value,

before normalization.

shape of the curve is not retrieved, but another one,

which is exactly the same as the measured one, except

that it is reversed along the y-coordinate. Hence, using an

opposite sign for the gravitational force equation, the

measured curve shape is retrieved. This is true for each

of the five real galaxies which has been calculated: NGC

3310, NGC 106 8, NGC 157, NG C 7541, and N GC 7331.

This is calculated in [2]. A plausible explanation of this

error is an occultation mechanism during the propagation

of the space-time deformations coming from the galaxy

luminous points. In this mechanism, the galaxy dust is

acting like a fog, occulting the space-time deformation

propagations coming from the matter located beyond it.

This mechanism has been confirmed by calculations, but

still remains to be explained fully.

For some galaxies, it is possible to calculate the exact

values of the theoretical speed, for the maximums of the

speed profiles. For NGC 3310, the measured value in [6]

for the first maximum is around 175 km/s. The corre-

sponding theo retical value is in the rang e [20, 700] km/s.

For NGC 1068, the measured value in [6] is 210 km/s,

the theoretical value is 260 km/s. The precision of those

theoretical calculations has not been evaluated. But those

calculations are not fitted.

9. Galaxy Speed Mystery

Let us study the mystery of the velocities of the galaxies.

From the above equations we have, after calculations:

















(21)

This sum is done for each luminous point, p, along a

half-cone. This half-cone is centered on the location in

which we want to calculate G.

e is the energy of each

luminous point.

is the distance of each luminous

point from the location in which we calculate G. Let us

write:

inside outside



 



(22)

is for the p luminous points inside the Milky Way.

o is for luminous points outside the Milky Way. There-

fore:



2



 



 (23)

It has been written G as the gravitational constant valid

inside the Milky Way, and G' when located outside any

galaxy. Therefore this modeling of relativity explains

qualitatively the “dark matter” mystery for the velocities

of the galaxies. Also, this exp lanation is the same for the

mystery of light beam deviation in the vicinity of a gal-

axy.

10. PPN Parameters

The PPN parameters (Parameterized Post-Newtonian for-

malism) are exactly the same as for relativity. Indeed, the

only differences between relativity and the gravitational

model of the three elements theory are the following.

 Lorentz equations are true only between inertial ref-

erence frames which get “energy attached locally”.

“Ener gy attached locally” to a reference fra me ( O, ct, x,

y, z) means that there is a particle or a group of parti-

cles whom inertial poi nt i s const antly equal to O.

 Newton’s law is not used, like in relativity, but re-

trieved. There is a slight difference between Newton’s

law and this corrected Newton’s law.

 There exists nonlinearity in the superposition law for

gravity. This work is in progress.

Each relativistic equation remains also the same, ex-

cept Einstein’s equation because it is calculated using

Newton’s law. In the gravitational model of the three

elements theory, Einstein’s equation is only an approxi-

mation.

11. Earth Flyby Anomalies

The issue of the flyby anomalies is explained in [7]. When

applying the “first modification of Newton’s law”, the

order of magnitude is well retrieved for these anomalies.

Table 1 shows the theoretical added ve locities, which are

roughly of the same order of magnitude as the measured

ones.

However, those calculations are much too simpler. The

F. LASSIAILLE 395

Table 1. Comparison between the real and the theoretical

Earth flyby anomalies for 6 probes. The values represents

added perigee velocitie s. Units in mm/s.

Probe Measured anomaly Theoretical Anomaly

Galilleo I 2.56 2.53

Galilleo II –2 –11

NEAR 7.21 –6.6

Cassini –1.7 4.5

Rosetta I 0.67 22

Messenger 0.008 29

calculations must be done at least in the Schwarzsch ild’s

metric of the sun, using the motion of the Earth, the exact

trajectory of the probes, and the contributions of the sur-

rounding matter (asteroids, planets, etc.). Moreover, the

“second modification of Newton’s law must be used also,

as the Pioneer anomaly analysis shows. Noticeably, the

location of the ecliptic plane as compared to the exact

probes trajectories, has an important impact on the final

result. Indeed, the Kuiper belt is located on this plane, and

the Kuiper belt influence has been proven in the Pioneer

anomaly analysis above. This remark might explain the

experim ental verification of the importance of the location

of the equator plane as c ompared to the probes trajectories.

Indeed, the equator and the ecliptic planes are not far away

from each other.

12. Perihelion Advance

When applying the “first modification of Newton’s law”

to the perihelion advance of Mercury and Saturn, the

results of Table 2 are retrieved. The theoretical values are

very close from the general relativity values. They do not

explain the anomaly of the precession of the Saturn peri-

helion explained in [8], which is of –0.006 arc-second by

century.

But, since the calculated values are very close to t he GR

values, it results that the three elements theory model

seems to be compatible here with gravitation experimental

measurements. Moreover, as well as for Earth flyby ano-

malies, the “second modification of Newton’s law” must

be conducted, i n order to check if t he Saturn anomaly of [8]

is theoretically explained.

13. Measurements of G

The issue is well defined. For nearly three centuries, G has

been measured, without getting roughly a better precision

than 0.7%. The reason of this poor precision is the fact that

many measurements values are contradicting each others,

taking into account their con fid ent interval. The details of

this issue is, for example, well described in [9]. The “first

modification of Newton’s law” is not able to exp lain this

issue. Indeed, the order of magnitude of the theoretical

Table 2. Theoretical modification of the perihelion advance

or precession of Mercury and Saturn. On the left are the

general relativity results calculated with a computer pro-

gram. On the right are the added values from these general

relativity values. Units are arc-second by century.

Planet GR value 3elt value

Mercury 42.7848 GR value – 0.0014

Saturn 1.66291 GR value + 0.00056

error is far below the experimental one.

But the “second modification of Newton’s law” re-

trieves the same order of magnitude as the measured one.

Let’s compare first with data coming from [10]. A pre-

diction of the model of this document is that the G value is

depending of the surrounding matter distribution. This is

not a ne w i dea , it has bee n s u gge ste d i n [ 1 1]. M ore ov e r, i t

has been proven by experimental data in [10], that the

value of G depends of the orientation. This behavior is

also a consequence of this theoretical idea. In [10], the

amplitude of the variation of the G value is more than

0.054% as compared to its absolute value. The prediction

of the gravitational model of the three elements theory for

this value is be tween 1% and 0.01 3% depending o f galaxy

matter distribution. Therefore, the order of magnitude of

this model prediction is compatible with experimental

data.

Now let’s try another estimation, not tak ing in account

the presence of the stars, like in [10], but the presence of

mountains around the apparatus during the measurement

of G. The ratio below is the relative difference between

two values of G, 1m, and 2m. 1m is the measured

value of G in [12], and is the measured value in

[13].

G G

6.5 10



 (24)

Those two official measurements of G have been cho-

sen in order to get the greatest possible ratio above.

Let us assume that those two experiments have been

done in completely different places. And the important

difference between them is the distribution of matter in the

surrounding neighbourhood.

 Experiment {1}, for example, is done at the very top

of a hill on the floor of a desert, and this floor is com-

pletely plane outside of the hill on which we are lo-

cated.

 Experiment {2}, is done in the middle of a valley,

which is surrounded by mountains.

The interesting thing is that the measured value of G

will be completely different between those two cases even

if exactly the same experiment apparatus is used and the

same measurement procedure is applied. Indeed, the pre-

sence of the surrounding mountains in the second mea-

surement has an important effect on the final measured

F. LASSIAILLE

396

value. After calculations, the corresponding theoretical

ratio of G is estimated by the following formula.



 (25)

r stands for the maximum distance between the sur-

rounding mountains, and the location of experiment {2}.

It will be used .

4 km

r

r stands for the greatest distance between a galactic

object and the location of the experiments. It will be used

the same value as for the Pioneer anomaly calculation:

. This value is based on the occultation me-

chanism solving the sign issue which has been noticed

above for the speed profiles. In other words, galactic ob-

jects located beyond this distance will not be noticed.

Their space-time deformation will vanish, during propa-

gation, before arrival, because of the occultation mecha-

nism. This

140 pc

r

r value has been fitted in a coherent man-

ner by the model when comparing its predictions to ex-

perimental data.



is the mean matter density of the surrounding

mountains of experime nt {2}. It will be used ρs = 1.7 g/cm 3,

which is weaker than granite density .

granite 2.7 g/cm







is the matter density of the galaxy. We wil l use the

value , which is the matter den-

sity in the galaxy, near the solar system.

20 3

0.70910 kg/m







With those numerical values, the final result is the fol-

lowing.

0.910





 (26)

This theoretical value is not far from the meas ure d o ne,

of Equation (24). This proves that the order of magnitude

of the measured difference can be explained by our cor-

rection of Newton’s Law.

As an intermediate conclusion, the gravitational model

of this study might explain the disparity between the

measurements of G. This theoretical value of G is de-

pending on the distribution of matter in the surrounding

neighborhood (buildings, hills, mountains, and sea) of the

place where the measurement of G is done.

A linearity violation of gravitational forces might be

predicted by the m odel. If predicted, it could explain, also,

this historical disparity in the measurements of G. This

work is in progress.

14. Conclusions

As a conclusion, this new modeling of space-time retri-

eves general and restricted relativity. Nevertheless, it is

more than a simple Euclidean representation of relativity,

as shown by postulate 1 and 2.

A direct explanation of the Sagnac effect is given by

this modeling. Moreover, it is enough to add a third pos-

tulate in order to explain those “dark matter mysteries”: 1)

galaxy speed profiles, 2) speed of the galaxies them-

selves, inside their group, and deformation of light tra-

jectories in the vicinity of a galaxy, 3) Pionee r anomaly.

In more details, this third postulate conducts a modify-

cation of Newton’s law. This modification is conceived

in order to find exactly Newton’s law in the specific case

of pin pointed masses inside an homogeneous universe,

and long distances. Using the model’s equation, immedi-

ately a correction of Newton’s law is noticed in the case

of short distances. This first correction occurs in fact for

relativistic speed. Now, adjusting this correction in order

to fit Newton’s law with this new law for some particular

distance, yields immediately a theoretical explanation of

Pioneer anomaly. This model is also predicting an anom-

aly for Saturn flyby b y Pioneer 11.

Another result of this correction of Newton’s law oc-

curs when the galaxy’ s stars are introduced in the model.

After this, a strong difference appears between the cal-

culated force and Newton’s law. The predicted galaxy

speed profiles are close to measured speed profiles.

For the mystery of galaxy velocities, the explanation is

more direct. This mystery is explained by a different

value of G, between our case inside the Milky Way, and

the case outside any galaxy. Here again, the “third spea-

ker”, which decreases G constant, is the stars of our gal-

axy.

This model m ight ex plain als o, a fter s ome calculations,

the following anomalies:

 Earth flyby anomalies,

 perihelion precession of Saturn,

 disparity of the gravitational constant measurements.

Moreover, and from a theoretical point of view, this

study finds a way for space-time determination. At any

space-time point, this determination is based upon the

matter density distribution throughout the whole un iverse.

This model is compatible by construction with restricted

and general relativity.The PPN parameters are exactly

the same as for relativity.It seems to be compatible also

with gravitation experim e ntal m easurements.

As a conclusion, the gravitational model of the three

elements theory seems to be validated. As such, this is a

validation of the three elements theory itself. This theory

is described in [14]. A next step will be to solve the sign

issue for the dark matter mystery (speed profiles). The

exact comparison with ephemerides must be done also.

Another interesting work will be to calculate the linearity

violation of gravitational forces predicted by the model,

and to compare it to experimental data. It remains also to

check if the model described in this article is coherent

with other actual physics theories (electromagnetism,

quantum mechanics, etc). As an answer to this last ques-

tion one will notice that this m odel is in conformity with a

F. LASSIAILLE

397

unifying theory called three elements theory.

REFERENCES

[1] F. Lassiaille, “A Solution for the Dark Matter Mystery

Based on Euclidean Relativity,” 2010.

http://lumi.chez-alice.fr/anglais/mystmass.pdf

[2] F. Lassiaille, “Validation of Dark Matter Explanation,”

2010.

http://lumi.chez-alice.fr/anglais/Calcul_NGC3310.pdf

[3] F. Lassiaille, “Gravitational Model of the Three Elements

Theory: Explanation of the Pioneer Anomaly,” 2012.

http://lumi.chez-alice.fr/anglais/PioneerExpl.pdf

[4] S. T. Turyshev and V. T. Toth, “The Pioneer Anomaly,”

Living Reviews in Relativity, Vol. 13, 2010, p. 4.

[5] G. A. Kyazumov, “The Velocity Field of the Galaxy

NGC 7541,” Pis’ma v Astronomicheskii Zhurnal, Vol. 6,

1980, pp. 398-401.

[6] G. Galletta, and E. Recillas-Cruz, “The Large Scale Trend

of Rotation Curves in the Spiral Galaxies NGC 1068 and

NGC 3310,” Astronomy and Astrophysics, Vol. 112, 1982,

pp. 361-365.

[7] J. D. Anderson, J. K. Campbell, J. E. Ekelund, J. Ellis,

and J. F. Jordan, “Anomalous Orbital-Energy Changes

Observed during Spacecraft Flybys of Earth,” Physical Re-

view Letters, Vol. 100, No. 9, 2008, pp. 091102-091105.

doi:10.1103/PhysRevLett.100.091102

[8] I. Lorenzo, “The Recently Determined Anomalous Peri-

helion Precession of Saturn,” Astronomical Journal, Vol.

137, N o . 3, 2009, p. 3615.

doi:10.1088/0004-6256/137/3/3615

[9] G. T. Gillies, “The Newtonian Gravitational Constant:

Recent Measurements and Related Studies,” Physical Re-

view Letters, Vol. 60, No. 2 , 1 997 , p . 151.

doi:10.1088/0034-4885/60/2/001

[10] M. L. Gershteyn, L. I. Gershteyn, A. Gershtey n and O. V.

Karagioz, “Experimental Evidence That the Gravitational

Constant Varies with Orientation,” Classical Physics,

Gravitation & Cosmology, Vol. 8, No. 3, 2002, pp. 1-6.

[11] M. L. Gershteyn and L. I. Gershteyn, “The Attractive

Universe Theory,” Australian National University, Re-

daktsiya Izvestiya Vuzov Radiophysica, 2004.

http://hdl.handle.net/1885/41360

[12] W. Michaelis, H. Haars and R. Augustin, “A New Precise

Determination of Newton’s Gravitational Constant,”

Metrologia, Vol. 32, No. 4, 1995, pp. 267-270.

doi:10.1088/0026-1394/32/4/4

[13] C. H. Bagley and G. G. Luther, “Preliminary Results of a

Determination of the Newtonian Constant of Gravitation:

A Test of the Kuroda Hypothesis,” Physical Review Let-

ters, Vol. 78, 1997, pp. 3047-3050.

doi:10.1103/PhysRevLett.78.3047

[14] F. Lassiaille, “Three Elements Theory,” 1999.

http://lumi.chez-alice.fr/3elt.pdf