Journal of Modern Physics
Vol.06 No.09(2015), Article ID:59195,9 pages
10.4236/jmp.2015.69140
Low Density Instability in Asymmetric Nuclear Matter Using Pion Dressing
S. K. Sahu
Department of Physics, Banki College, Banki, Cuttack, India
Email: saroj9a@yahoo.co.in
Copyright © 2015 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 7 July 2015; accepted 24 August 2015; published 27 August 2015
ABSTRACT
We study the asymmetric nuclear matter in a nonperturbative manner. The bulk nuclear matter studied by the consistent exchange of σ, ω and π mesons is used to investigate its stability. The equation of state (EOS) at zero temperature is considered to study the symmetry energy, curvature parameter of symmetry energy and asymmetry energy. The effect of the density dependence of the symmetry energy on instability property is investigated and analyzed using proton fraction in the nuclear matter. Here a microscopic density-dependent model of the nucleon-meson coupling is used to reexamine the instability of asymmetric nuclear matter.
Keywords:
Symmetry Energy, Equation of State, Nucleon-Pion Interaction

1. Introduction
The characteristics of dense nuclear matter are very much interesting for particle physics, astrophysics, as well as nuclear physics [1] -[7] . The nuclear equation of state, especially its stability, is an important item for the study of dynamic evolution of the early Universe, the stability of the neutron stars and the pattern of change of nuclear matter properties below the saturation density. Nuclear matter is predicted to exhibit phase transition between Fermi liquid and nucleonic gas at saturation density and elevated temperature. Analysis of nuclear multifragmentation [8] supports the idea that the mixed phase of neutron and proton matter may be formed [9] -[11] . Since these nuclei are made of neutrons and protons, the nuclear liquid-gas phase transition is a binary system where one has to deal with two independent proton and neutron chemical potentials for baryon number and charge conservation. There are experiments showing that the formation of highly excited compound nuclei in equilibrium is interpreted as the two coexisting phases of liquid and gas in the frame work of hydrodynamics. The liquid-gas phase transition is also useful for the study of core of compact stars in the range of the densities from ρ = 0.03 fm−3 to saturation density ρ0 = 0.15 fm−3. It is observed that the phase transition leads to an isospin distillation phenomenon [8] (isospin content of each phase is different). In fact, the information coming from the experiments with heavy ions in intermediate and high energy collisions [8] shows that the equation of state (EOS) depends not only on the energy beam but also sensibly on the proton fraction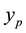 . The symmetry-energy, which describes the single nucleonic energy or nuclear matter changes as one, replaces protons with neutrons in the system. It determines the birth of neutron stars and supernova neutrinos and also plays the crucial role in the evolution of core collapse of supernovae.
. The symmetry-energy, which describes the single nucleonic energy or nuclear matter changes as one, replaces protons with neutrons in the system. It determines the birth of neutron stars and supernova neutrinos and also plays the crucial role in the evolution of core collapse of supernovae.
Understanding of the properties of the hot nuclear matter at normal and low density regions is of crucial importance for explaining the stability and structure of nutron stars after the supernova explosion. The experiments with unstable nuclear beams and relativistic heavy ions are the potential tools in determining the best equation of state (EOS) which may be derived from either relativistic or potential model. The problem of dense nuclear matter therefore has been a hot bed of investigations for the past few years and was looked by Walecka [12] , and others [13] [14] known as the Non Linear Walecka Model (NLWM). They consider the nucleon-neutral scalar field interactions with σ and ω in a nonperturbative method, treating these scalar fields as elementary. They reproduce correct bulk modulus of dense nuclear matter [15] . However, several open questions still remain unanswered. One of the basic questions is the microscopic nature of σ-field which is unclear in Walecka model since σ cannot be interpreted as the physical particle as it has not been discovered. Secondly, one cannot have the nonrelativistic limit of the model in a straight forward way. Since the points are playing a crucial role in neutron matter in terms of pion-nucleon interaction, it is the essential ingredient for studying such neutron stars. It is worth mentioning here that pion-nucleon interaction was a basic ingredient to study the nuclear processes [16] in the past with pion as elementary particle, and might play a crucial role in nucleon matter in terms of pion-nucleon interaction and enhance the nuclear matter properties. The
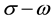 model with further inclusion of mesons like π, ρ etc. [14] [17] was taken to study its properties. Being motivated, we try to study and understand the stability and the symmetry energy of nuclear matter with pion dressing [18] in the present work. In fact it is known that the introduction of σ and ρ mesons in the constant coupling model leads to stability of the nuclear matter which is not oblivious for density-dependent models.
model with further inclusion of mesons like π, ρ etc. [14] [17] was taken to study its properties. Being motivated, we try to study and understand the stability and the symmetry energy of nuclear matter with pion dressing [18] in the present work. In fact it is known that the introduction of σ and ρ mesons in the constant coupling model leads to stability of the nuclear matter which is not oblivious for density-dependent models.
The article is organized as follows. In Section 2, we review the formalism of asymmetric dense nuclear matter taking nonrelativistic pion nucleon interaction and determine the symmetry energy Esym, symmetry energy slope L and curvature parameter of symmetry energy Ksym and their effects on stability of nuclear matter. In Section 3, we study the stability condition using stability matrix eigen vectors dependence on densities. In Section 4, we analyze our results which agree with other works [19] in this field.
2. Formalism
We consider the effective Hamiltonian for pion nucleon interaction [18] and [20] [21] as
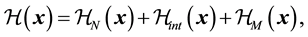 (1)
(1)
where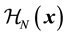 ,
,
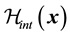 and
and
 are the Hamiltonians for the free nucleon part, the pion-interaction part and the free meson part respectively. Here
are the Hamiltonians for the free nucleon part, the pion-interaction part and the free meson part respectively. Here
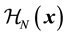 is given by
is given by
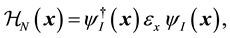 (2)
(2)
and the effective Hamiltonian
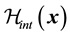 for pion nucleon
for pion nucleon
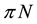 interaction part [22] is given by
interaction part [22] is given by
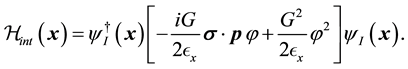 (3)
(3)
We have taken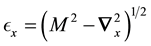 , with M as the mass of the nucleon. The free meson part of the Hamiltonian is given by
, with M as the mass of the nucleon. The free meson part of the Hamiltonian is given by
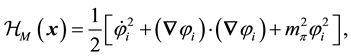 (4)
(4)
where
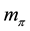 denotes the mass of the meson and
denotes the mass of the meson and . The pion field expansion in Equation (4) in terms of annihilation and creation operators is given by
. The pion field expansion in Equation (4) in terms of annihilation and creation operators is given by
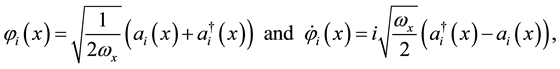 (5)
(5)
with
The two pions constitute a scalar-isoscalar interaction of nucleons and thus could simulate the effects of σ- mesons. The bare nucleon states however can get dressed with pions. These states are necessary for the correct description of nuclear matter. We now proceed to introduce a “meson” dressing of nuclear matter through the state [18]

where the two pion creation operator


and the free nucleon energy density becomes

where the spin degeneracy factor



With the meson field operator expansion as in Equation (5) we may write Equation (4) as

Using Equation (10), we now obtain kinetic energy density due to the mesons as

where


In the non-relativistic limit and using Equation (7), the kinatic energy density (12) becomes

The meson energy density thus is given as

Now extremising Equation (14) with respect to

where


where a and


In place of Equation (14) we now obtain the expression for


where the terms




where

where the parameter



Similarly, we have to include the repulsion energy from the ρ-mesons [20] [21]

where



where the total energy density


The total density




respectively. Now the expression for pressure is given by the equation

The symmetry energy parameter


The symmetry energy slope is defined by

The curvature parameter of symmetry energy slope is

and the curvature parameter of the asymmetry energy is

In the next section we study the stability condition considering the above properties of nuclear matter.
3. Stability Condition
Now the proton fraction is introduced to study the stability conditation as


where

and

In the above,


The stability conditation for asymmetric nuclear matter at constant temperature and constant volume are obtained from the free energy density




is positive-definite with i and j run for proton and neutron respectively. We note here that the equivalent condition for convex function [26]

where we use
The two eigenvalues of the


and the eigenvectors


Both the eigenvalues of stability matrix as above should be positive to guarantee the stability of the system. Since the symmetry energy is dependent on the quadratic function of the asymmetry parameter (27) and a positive increasing symmetry energy with the total density (28), it ensure that only one eigenvalue can become negative. In principle



4. Results and Discussions
The model presented here contains four parameters, namely the phenomenological strength and length scale parameters of meson repulsion a and









We now discuss the results obtained in the calculations. Firstly the parameters are fixed using the saturation properties of the nuclear matter i.e. EB = −16 MeV and ρ0 = 0.15 fm−3 MeV. The pion-nucleon coupling constant is as

In Figure 1, the symmetry energy variation is studied as the function of density. The variation of symmetry energy with density agrees with results of Non Linear Walecka Model (NL3) [28] , Quark Meson Coupling model (QMC) [29] and density-dependant relativistic Hadron model (TW) [30] , while our symmetry energy is relatively
Figure 1. The symmetry energy Esym as a function of nucleon density ρ.
less above density 0.2 fm−3. It is due to the effect of pion dressing as it is negative relative to



In Figure 2, variation of the curvature parameter of the symmetry energy with density in the present study for both the compressibilities K = 270 and 290 coincides. Again our results agree almost with results of QMC [29] and agree nearly with that of NL3 [28] . It appears that the pion dressing of the nuclear matter in the present case hopefully gives better results.
The variation of slope of symmetry energy with density in Figure 3 shows that it starts decreasing in the very low densities i.e. up to

In Figure 4, we show how the ratio of the proton and neutron density fluctuation changes with densities corresponding to the unstable mode. This also shows that the instability exits for the different proton fractions, although small, in the asymmetric nuclear matter (ANM). In Figure 4(a), for the proton fraction



We show in Figure 5 that the variation of ratio of proton and neutron density fluctuation with proton fraction yp for a given density ρ = 0.06 fm−3 has an upward trend and reaches the value 1 at
Figure 2. The curvature parameter of symmetry energy ksym as a function of nucleon density ρ.
Figure 3. The slope parameter L as a function of nucleon density ρ.
Figure 4. The instability (eigenvector of negative eigenvalue) as a function of nucleon density ρ calculated for different values of the proton fraction yp = 0.05, 0.1 and 0.3.
Figure 5. The variation of negative eigenvector with the proton fraction yp for the value of ρ = 0.06 fm−3.
in all models ((NL3) [28] , (QMC) [29] and (TW) [30] ). Baring some differences, we note that the pion dressing of ANM is a need to understand to some extent its stability at zero temperature.
Cite this paper
S. K.Sahu, (2015) Low Density Instability in Asymmetric Nuclear Matter Using Pion Dressing. Journal of Modern Physics,06,1350-1359. doi: 10.4236/jmp.2015.69140
References
- 1. Margueron, J. and Chomaz, P. (2003) Physical Review C, 67, Article ID: 041602.
http://dx.doi.org/10.1103/PhysRevC.67.041602 - 2. Chomaz, P., Colonna, M. and Randrup, J. (2004) Physics Reports, 389, 263.
http://dx.doi.org/10.1016/j.physrep.2003.09.006 - 3. Avancini, S.S., Brito, L., Menezes, D.P. and Providência, C. (2004) Physical Review C, 70, Article ID: 015203.
http://dx.doi.org/10.1103/PhysRevC.70.015203 - 4. Avancini, S.S., Brito, L., Chomaz, Ph., Menezes, D.P. and Providência, C. (2006) Physical Review C, 74, Article ID: 024317.
- 5. Margueron, J., van Dalen, E. and Fuchs, C. (2007) Physical Review C, 76, Article ID: 034309.
- 6. Vidana, I. and Polls, A. (2008) Physics Letters B, 666, 232.
http://dx.doi.org/10.1016/j.physletb.2008.07.066 - 7. Pais, H., Santos, A. and Providência, C. (2009) Physical Review C, 80, Article ID: 045808.
http://dx.doi.org/10.1103/PhysRevC.80.045808 - 8. Xu, H.S., et al. (2000) Physical Review Letters, 85, 716.
http://dx.doi.org/10.1103/PhysRevLett.85.716 - 9. Botvina, A.S., Mishustin, L.N. and Begenann-Blaich, M. (1995) Nuclear Physics A, 584, 737.
http://dx.doi.org/10.1016/0375-9474(94)00621-S - 10. Li, B.A., De Angelis, A.R. and Gross, D.H.E. (1993) Physics Letters B, 303, 225.
http://dx.doi.org/10.1016/0370-2693(93)91424-L - 11. Agostino, M.D., Botvina, A.S., Milazzo, P.M., Bruno, M., Kunde, G.J., Bowman, D.R., et al. (1996) Physics Letters B, 371, 175-180.
http://dx.doi.org/10.1016/0370-2693(96)00008-1 - 12. Walecka, J.D. (1974) Annals of Physics, 83, 491-529.
http://dx.doi.org/10.1016/0003-4916(74)90208-5 - 13. Gmuca, S. (1991) Journal of Physics G, 17, 1115-1126.
http://dx.doi.org/10.1088/0954-3899/17/7/010 - 14. Serot, B.D. and Walecka, J.D. (1986) Advances in Nuclear Physics, 16.
- 15. Gambhir, Y.K. and Ring, P. (1988) Physics Letters B, 202, 5-9.
http://dx.doi.org/10.1016/0370-2693(88)90843-X - 16. Friar, J.L. and Coon, S.A. (1994) Physical Review C, 49, 1272-1280.
http://dx.doi.org/10.1103/PhysRevC.49.1272 - 17. Matsui, T. and Serot, B.D. (1982) Annals of Physics, 144, 107-167.
http://dx.doi.org/10.1016/0003-4916(82)90106-3 - 18. Mishra, H., Misra, S.P., Panda, P.K. and Parida, B.K. (1992) International Journal of Modern Physics E, 1, 405-419.
http://dx.doi.org/10.1142/S0218301392000229 - 19. Santos, A.M., Providencia, C. and Panda, P.K. (2009) Physical Review C, 79, Article ID: 045805.
http://dx.doi.org/10.1103/PhysRevC.79.045805 - 20. Sarangi, S., Panda, P.K., Sahu, S.K. and Maharana, L. (2008) International Journal of Modern Physics B, 22, 4524-4537.
http://dx.doi.org/10.1142/S0217979208050279 - 21. Sarangi, S., Panda, P.K., Sahu, S.K. and Maharana, L. (2010) Indian Journal of Physics, 84, 431-447.
http://dx.doi.org/10.1007/s12648-010-0028-9 - 22. Mishra, A., Mishra, H. and Mishra, S.P. (1990) International Journal of Modern Physics A, 5, 3391-3399.
http://dx.doi.org/10.1142/S0217751X90001483 - 23. Baran, V., Colonna, M., Di Toro, M. and Larionov, A.B. (1998) Nuclear Physics A, 632, 287-303.
http://dx.doi.org/10.1016/S0375-9474(98)00006-2 - 24. Margueron, J. and Chomaz, P. (2003) Physical Review C, 67, Article ID: 041602.
Comaz, P., Colonna, M. and Randrup, J. (2004) Physics Reports, 389, 262. - 25. Mueller, H. and Serot, B.D. (1995) Physical Review C, 52, 2072-2091.
http://dx.doi.org/10.1103/PhysRevC.52.2072 - 26. van Dalen, E.N.E. and Muether, H. (2013) Physical Review C, 87, Article ID: 024317.
http://dx.doi.org/10.1103/PhysRevC.87.024317 - 27. Steiner, A.W., Prakash, M., Lattimer, J.M. and Ellis, P.J. (2005) Physics Reports, 441, 325-375.
http://dx.doi.org/10.1016/j.physrep.2005.02.004 - 28. Fuchs, C., Holter, H.H. (2006) European Physical Journal A, 30.
Lalazissis, G.A., Koeningf, J. and Ring, P. (1997) Physical Review C, 55, 540-543. - 29. Guichon, P.A.M. (1988) Physics Letters B, 200, 235-240.
http://dx.doi.org/10.1016/0370-2693(88)90762-9
Saito, K. and Thomas, A.W. (1994) Physics Letters B, 327, 9.
http://dx.doi.org/10.1016/0370-2693(94)91520-2
Saito, K. and Thomas, A.W. (1994) Physics Letters B, 335, 17.
http://dx.doi.org/10.1016/0370-2693(94)91551-2
Saito, K. and Thomas, A.W. (1995) Physics Letters B, 363, 157.
http://dx.doi.org/10.1016/0370-2693(95)01204-4
Guichon, P.A.M., Saito, K., Rodionov, E. and Thomas, A.W. (1996) Nuclear Physics A, 601, 349-379.
Saito, K., Tsushima, K. and Thomas, A.W. (1996) Ibid, A609, 339.
Saito, K., Tsushima, K. and Thomas, A.W. (1997) Physical Review C, 55, 2637.
http://dx.doi.org/10.1016/0375-9474(96)00033-4
Saito, K., Tsushima, K. and Thomas, A.W. (1996) Ibid, A609, 339.
Saito, K., Tsushima, K. and Thomas, A.W. (1997) Physical Review C, 55, 2637.
http://dx.doi.org/10.1103/PhysRevC.55.2637
Saito, K., Tsushima, K. and Thomas, A.W. (1997) Physics Letters B, 406, 287.
http://dx.doi.org/10.1016/S0370-2693(97)00693-X
Panda, P.K., Mishra, A., Eisenberg, J.M. and Greiner, W. (1997) Physical Review C, 56, 3134-3139.
http://dx.doi.org/10.1103/PhysRevC.56.3134
Panda, P.K., Menezes, D.P. and Providencia, C. (2004) Physical Review C, 69, Article ID: 025207. - 30. Typel, S. and Holter, H.H. (1999) Nuclear Physics A, 656, 331-364.
http://dx.doi.org/10.1016/S0375-9474(99)00310-3







