ABSTRACT

We considered a kind of singular integral operator with Weierstrass function kernel on a simple closed smooth curve in a fundamental period parallelogram. Using the method of complex functions, we established the Bertrand Poincaré formula for changing order of the corresponding integration, and some important properties for this kind of singular integral operator.

1. Introduction

The properties of singular integral operator with Cauchy or Hilbert kernel on simple closed smooth curve or open arc have been elaborately discussed in [1-3]. Based on these, for the boundary curve is a closed curve or an open arc, the authors discussed the singular integral operators and corresponding equation with Cauchy kernel or Hilbert kernel in [1-3]. In recent years, many authors discussed the numerical solution of a class of systems of Cauchy singular integral equations with constant coefficients, Numerical methods for nonlinear singular Volterra integral equations in [4-6].

In this paper, we consider a kind of singular integral operator with Weierstrass function kernel on a simple closed smooth curve in a fundamental period parallelogram. Our goal is to develop the Bertrand poincaré formula for changing order of the corresponding integration, and some important properties of the above singular integral operator.

2. Preliminaries

Definition 1 Suppose that 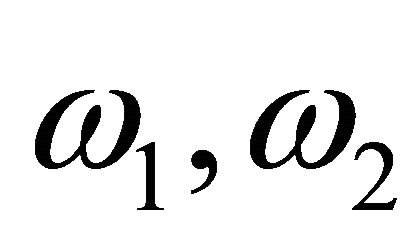are complex constants with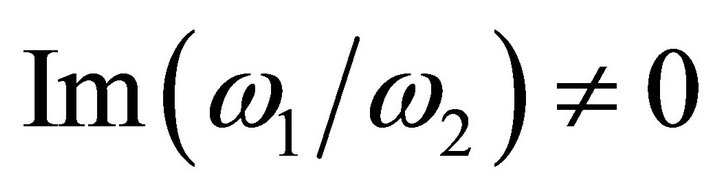, and P denotes the fundamental period parallelogram with vertices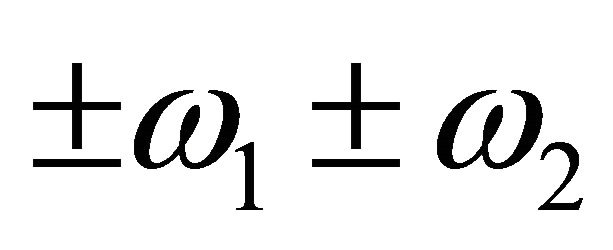. Then the function

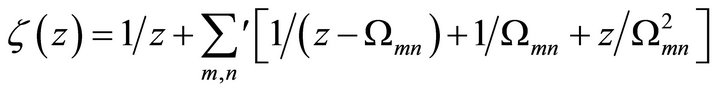
is called the Weierstrass -function, where

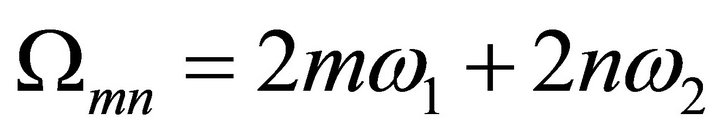
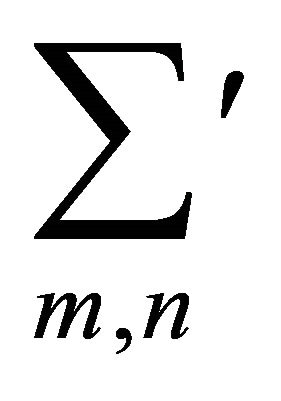denotes the sum of all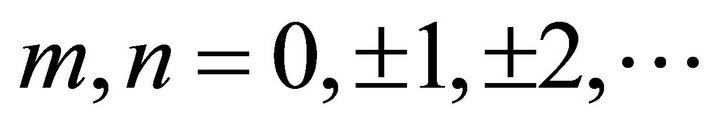, except for.

Definition 2 Suppose that 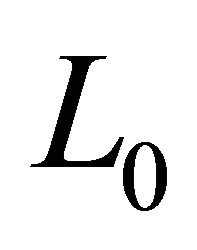is a smooth closed curve in the counterclockwise direction, lying entirely in the fundamental period parallelogram P, with and the origin lying in the domain 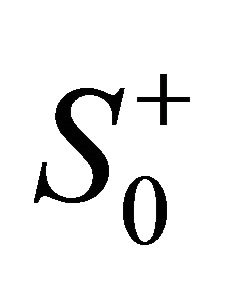enclosed by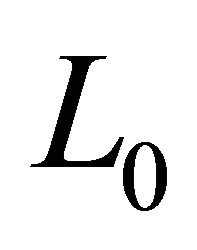. The following operator

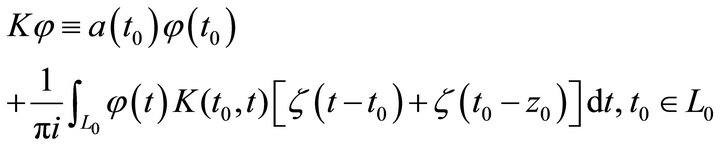(1)

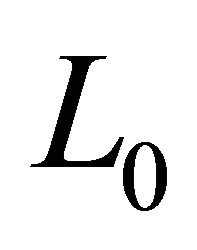is called the singular integral operator with -function kernel on, where 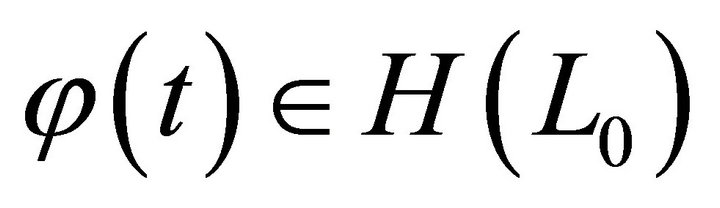is the unknown function, and

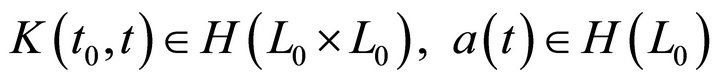
are the given functions.

Letting, then (1) becomes

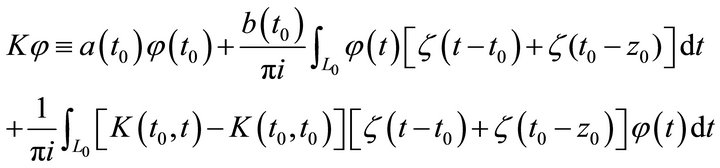(2)

Since 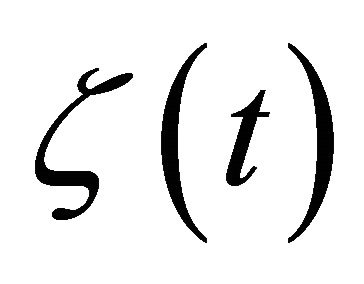is uniformly convergent in any closed bounded region lying entirely in P,

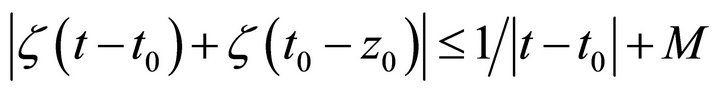
for any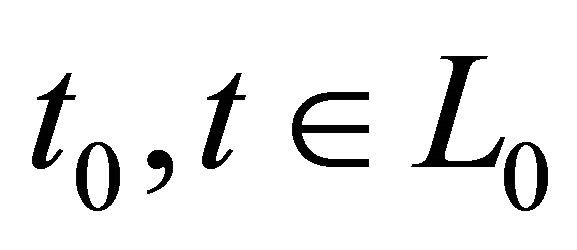, where 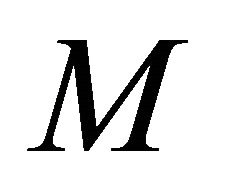is some positive finite constant. By noting that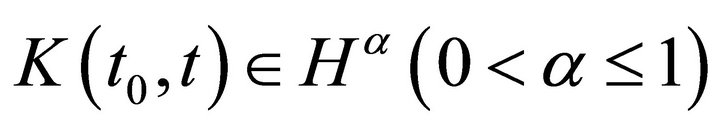, we obtain

, where is some positive finite constant. Write

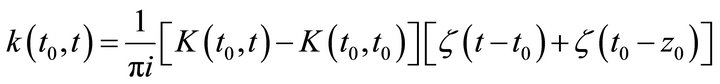
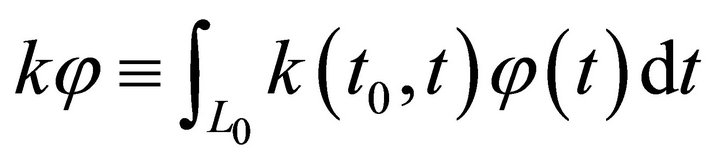
then (1) can be rewritten in the form

, (3)

where 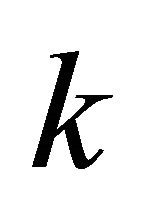is a Fredholm operator and is called the characteristic operator of. Now the index of is defined as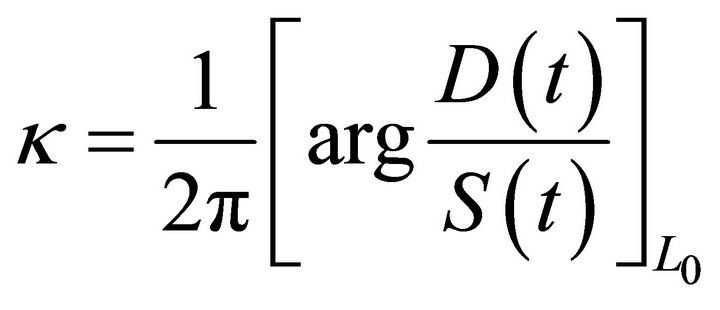, where

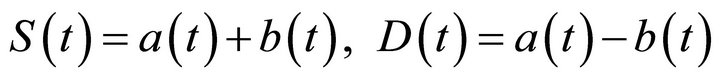
and for definiteness we assume that, namely we assume that is an operator of normal type.

Now the associated operator of (1) takes the form

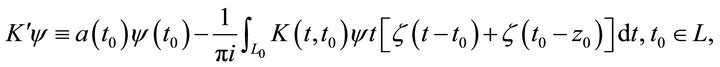(4)

or

          (4)′

and so that the associated operator of 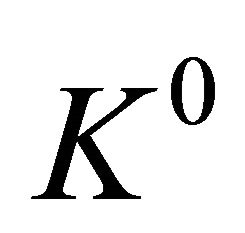becomes

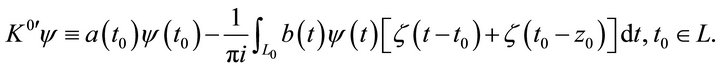
In addition, if we write

then (4) can be rewritten as

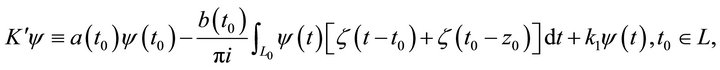(5)

where

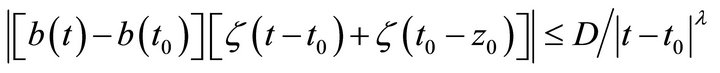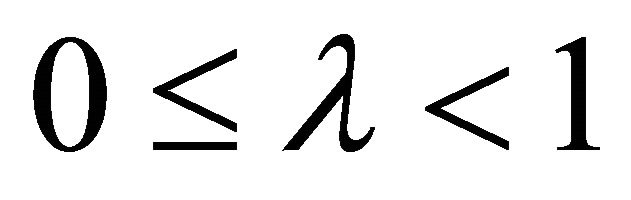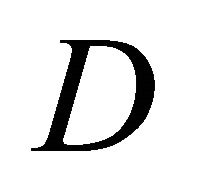(,is some finite constant).

So 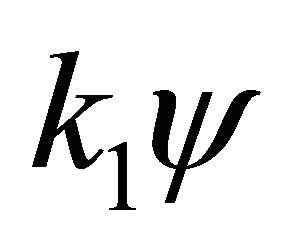is a Fredholm operator, and then the characteristic operator of 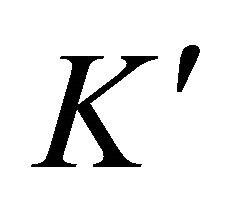operator becomes

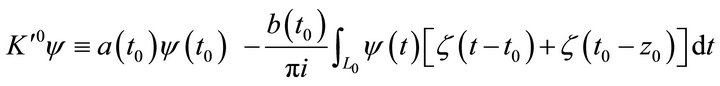(6)

Therefore, we concluded that 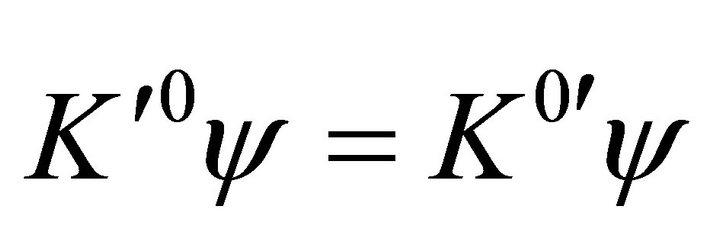usually can not be established, that is.

For convenience, we write

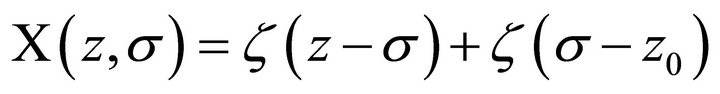
where the fixed nonzero point 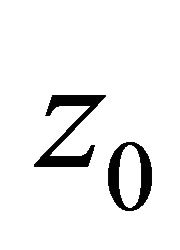and the origin lie in. It is not difficult to get the following results.

Lemma 1 Suppose that, and with the same as mentioned before, then a)

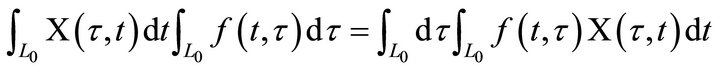
b) (Poincare-Bertrand formula)

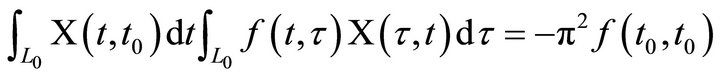
3. Some Properties of Operator K

1) If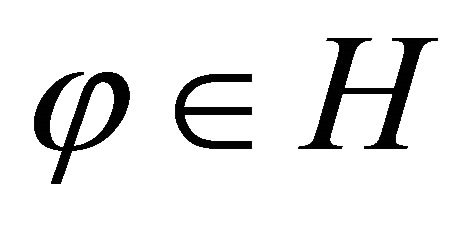, then.

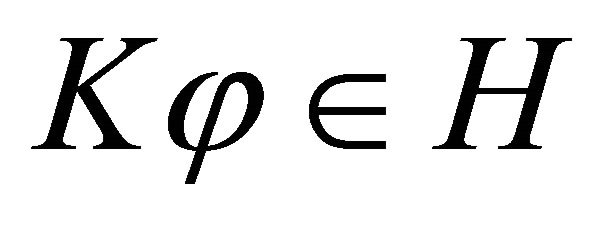Proof Through calculation and estimation, we have

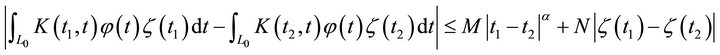(7)

for any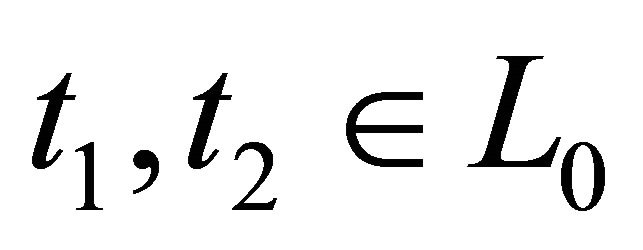, where and are all finite constant. While for any, we have

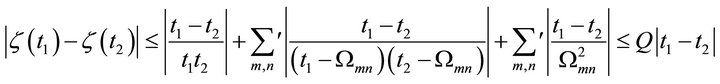(8)

where is some finite constant. Substituting (8) into (7), we obtain

(9)

Similarly we know that

Consequently, we have.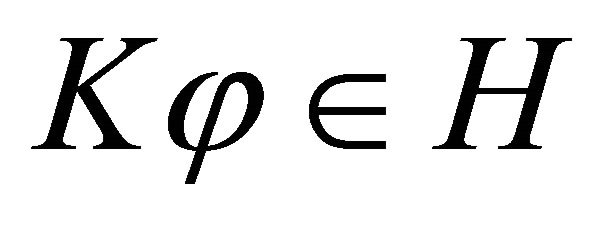

2) If are singular integral operator, then is also a singular integral operator. That is, if

then

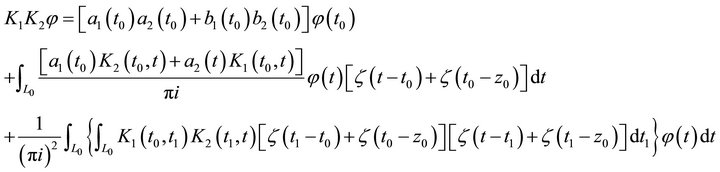, (10)

where the sum of the former two terms in the right hand of Equation (10) are the characteristic operator, and the remainder in that is a Fredholm operator.

Proof By definition, we deduce that

where

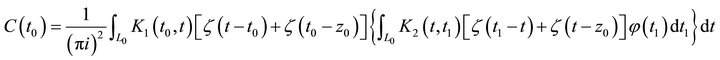
By virtue of Lemma 1 (b), can be rewritten in the form

Consequently, (10) is established.

Now we write

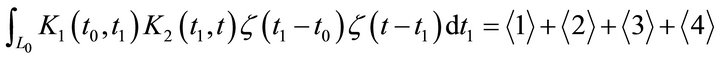
where

, ,

,

.

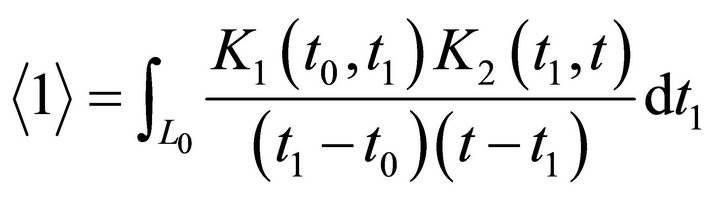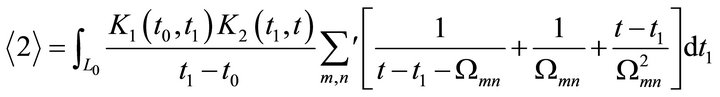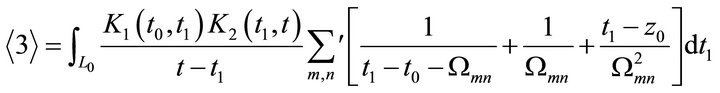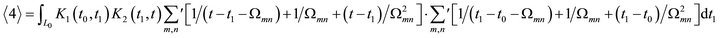By [1], we know that is a Fredholm integral. For, we know from

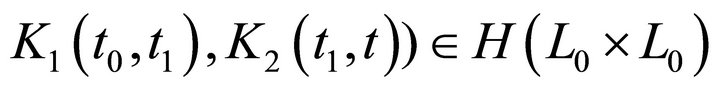
that is continuous about the variable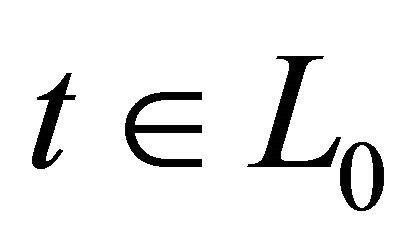, and so that 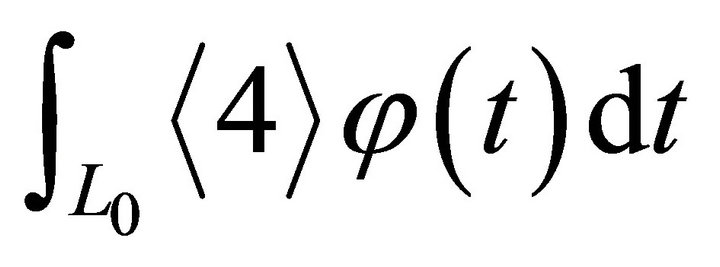is also a Fredholm integral. By nothing that 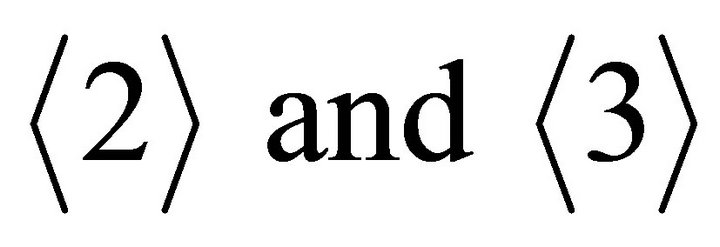have the same form, we only need to discuss either one of them. Here we consider the integral. Write

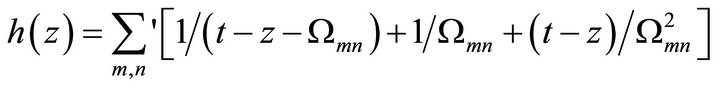
then 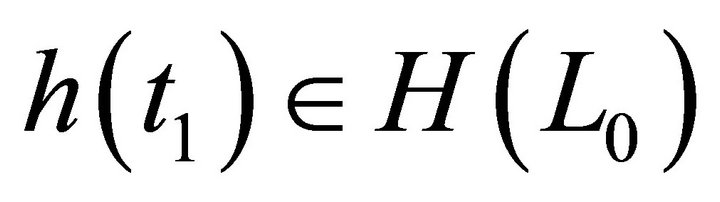is analytic in P and so that. Consequently, we read from

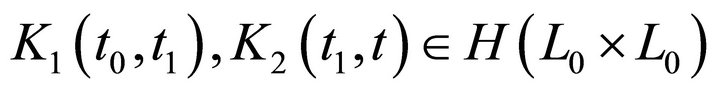
that 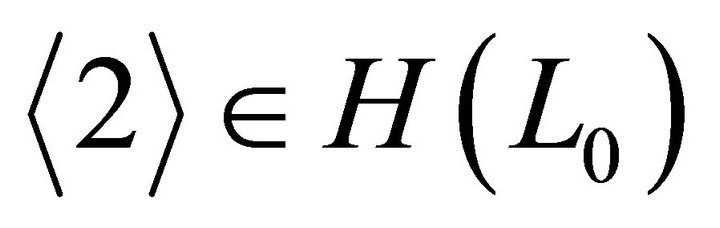and so that is continuous on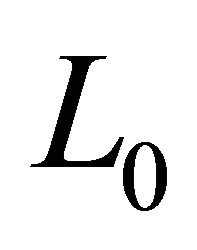, therefore 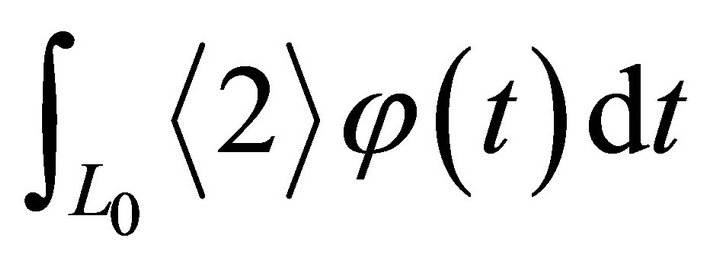is also a Fredholm integral.

So far, we conclude that 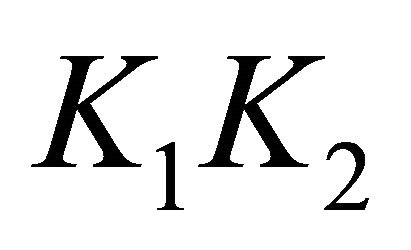is a singular integral operator.

3) Let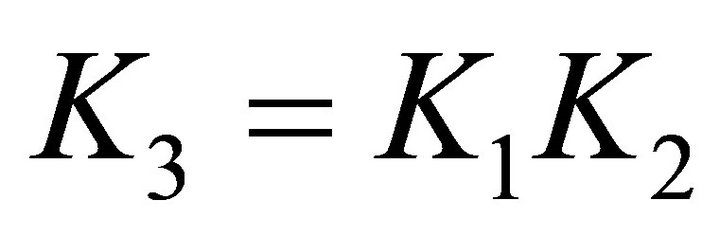, where denotes the indices of, then.

Proof From 2), we know

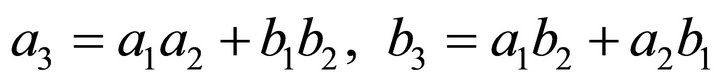
and

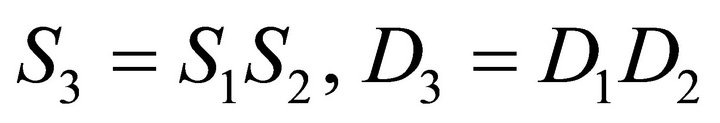
so.

In addition, we can see from

and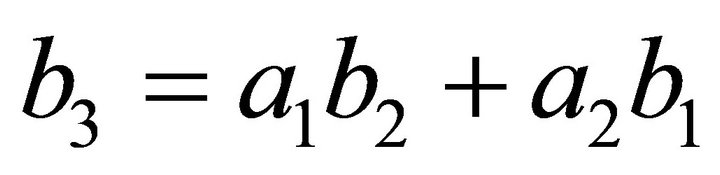that when 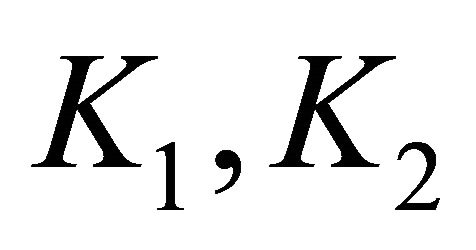are normal, 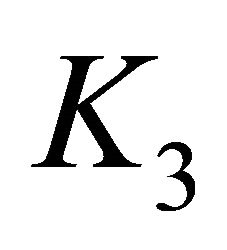is also normal.

4).

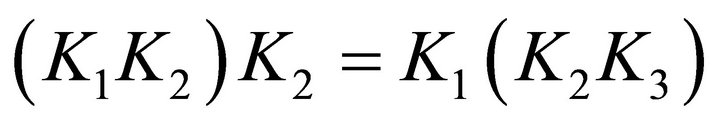5) If is a singular integral operator, and is a Fredholm integral operator of the first kind, then and are also Fredholm integral operators of the first kind.

6) If the indies of 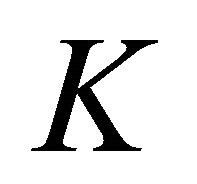and are and 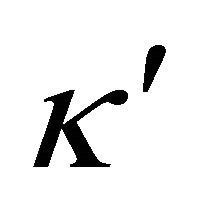respectively , then.

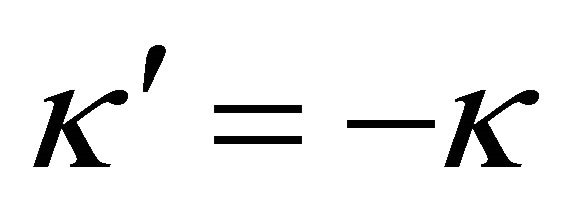7).

Through careful calculation, we may obtain 4) - 7).

8) Generally speaking,

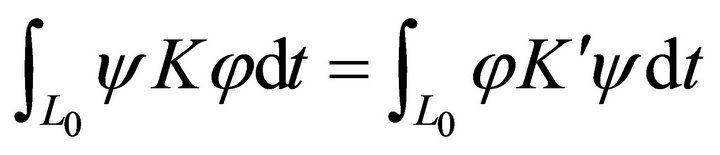
can not be established for.

Proof By definition and calculation, we have

. (11)

Whereas

. (12)

Let

then by Lemma 1(a), we have

, (13)

Substituting (13) into (12), we see that

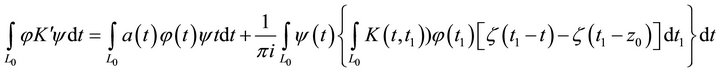. (14)

Therefore, 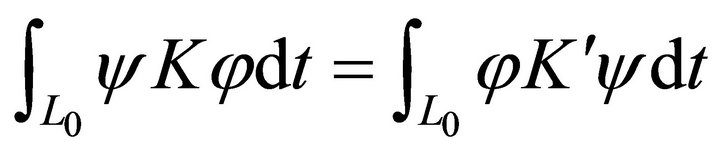cannot be established.

REFERENCES