Í. L. DE OLIVEIRA ET AL. 223
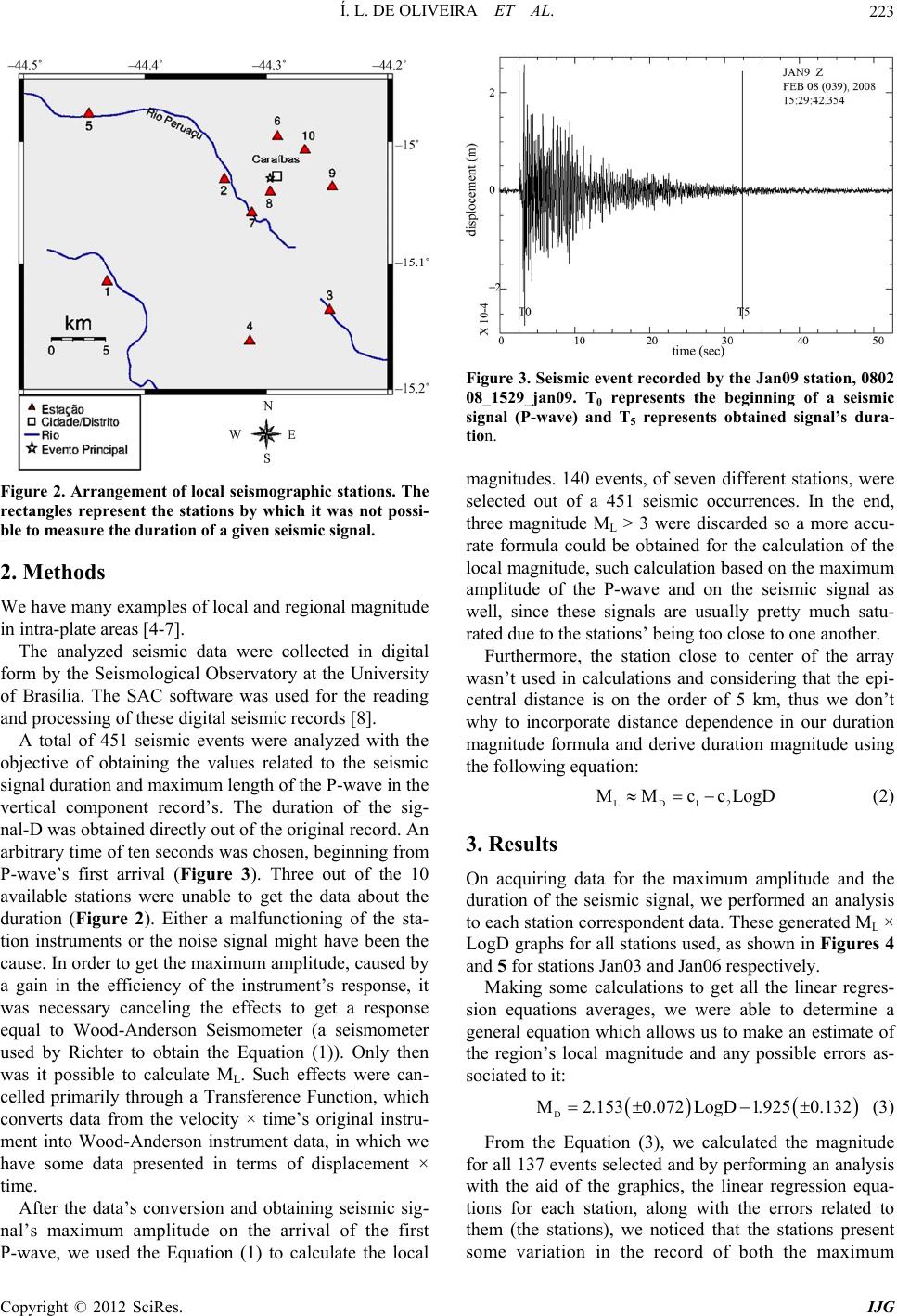
Figure 2. Arrangement of local seismographic stations. The
rectangles represent the stations by which it was not possi-
ble to measure the duration of a given seismic signal.
2. Methods
We have many examples of local and regional magnitude
in intra-plate areas [4-7].
The analyzed seismic data were collected in digital
form by the Seismological Observatory at the University
of Brasília. The SAC software was used for the reading
and processing of these digital seismic records [8].
A total of 451 seismic events were analyzed with the
objective of obtaining the values related to the seismic
signal duration and maximum length of the P-wave in the
vertical component record’s. The duration of the sig-
nal-D was obtained directly out of the original record. An
arbitrary time of ten seconds was chosen, beginning from
P-wave’s first arrival (Figure 3). Three out of the 10
available stations were unable to get the data about the
duration (Figure 2). Either a malfunctioning of the sta-
tion instruments or the noise signal might have been the
cause. In order to get the maximum amplitude, caused by
a gain in the efficiency of the instrument’s response, it
was necessary canceling the effects to get a response
equal to Wood-Anderson Seismometer (a seismometer
used by Richter to obtain the Equation (1)). Only then
was it possible to calculate ML. Such effects were can-
celled primarily through a Transference Function, which
converts data from the velocity × time’s original instru-
ment into Wood-Anderson instrument data, in which we
have some data presented in terms of displacement ×
time.
After the data’s conversion and obtaining seismic sig-
nal’s maximum amplitude on the arrival of the first
P-wave, we used the Equation (1) to calculate the local
Figure 3. Seismic event recorded by the Jan09 station, 0802
08_1529_jan09. T0 represents the beginning of a seismic
signal (P-wave) and T5 represents obtained signal’s dura-
tion.
magnitudes. 140 events, of seven different stations, were
selected out of a 451 seismic occurrences. In the end,
three magnitude ML > 3 were discarded so a more accu-
rate formula could be obtained for the calculation of the
local magnitude, such calculation based on the maximum
amplitude of the P-wave and on the seismic signal as
well, since these signals are usually pretty much satu-
rated due to the stations’ being too close to one another.
Furthermore, the station close to center of the array
wasn’t used in calculations and considering that the epi-
central distance is on the order of 5 km, thus we don’t
why to incorporate distance dependence in our duration
magnitude formula and derive duration magnitude using
the following equation:
LD12
MMccLogD (2)
3. Results
On acquiring data for the maximum amplitude and the
duration of the seismic signal, we performed an analysis
to each station correspondent data. These generated ML ×
LogD graphs for all stations used, as shown in Figures 4
and 5 for stations Jan03 and Jan06 respectively.
Making some calculations to get all the linear regres-
sion equations averages, we were able to determine a
general equation which allows us to make an estimate of
the region’s local magnitude and any possible errors as-
sociated to it:
D
M2.1530.072 LogD1.9250.132 (3)
From the Equation (3), we calculated the magnitude
for all 137 events selected and by performing an analysis
with the aid of the graphics, the linear regression equa-
tions for each station, along with the errors related to
them (the stations), we noticed that the stations present
some variation in the record of both the maximum
Copyright © 2012 SciRes. IJG