Engineering, 2010, 2, 228-236
doi:10.4236/eng.2010.24033 Published Online April 2010 (http://www.SciRP.org/journal/eng)
The Stability of Cylindrical Shells Containing an FGM
Layer Subjected to Axial Load on the Pasternak
Foundation
1Abdullah Heydaroglu Sofiyev, 2Mehmet Avcar
1,2Department of Civil Engineering of Suleyman Demirel University, Isparta, Turkey
Received July 7, 2009; revised November 4, 2009; accepted November 12, 2009
Abstract
In this study, the stability of cylindrical shells that composed of ceramic, FGM, and metal layers subjected to
axial load and resting on Winkler-Pasternak foundations is investigated. Material properties of FGM layer
are varied continuously in thickness direction according to a simple power distribution in terms of the ce-
ramic and metal volume fractions. The modified Donnell type stability and compatibility equations on the
Pasternak foundation are obtained. Applying Galerkin’s method analytic solutions are obtained for the criti-
cal axial load of three-layered cylindrical shells containing an FGM layer with and without elastic foundation.
The detailed parametric studies are carried out to study the influences of thickness variations of the FGM
layer, radius-to-thickness ratio, material composition and material profile index, Winkler and Pasternak
foundations on the critical axial load of three-layered cylindrical shells. Comparing results with those in the
literature validates the present analysis.
Keywords: FGM Layer, Stability, Cylindrical Shell, Critical Axial Load, Winkler and Pasternak Foundations
1. Introduction
Functionally graded materials (FGM) are characterized
by a gradual change in properties within the specimen as
a function of the position coordinates. The property gra-
dient in the material is typically caused by a posi-
tion-dependent chemical composition, micro structure or
atomic order. There are several studies about the proc-
essing of FGM, and an overview of the different manu-
facturing methods can be found in Koizumi [1] and Kie-
back et al. [2]. The mechanical behavior of FGMs shells,
such as bending due to mechanical loads, free vibration,
stability and buckling, etc., have been studied by many
scientists [3-8]. However, the literature on the investiga-
tion of vibration and stability problems of composite
structures that composed of ceramic, FGM, and metal
layers is scarce. A self-consistent constitutive framework
is proposed to describe the behaviour of a generic three-
layered system containing an FGM layer subjected to
thermal loading given by Pitakthapanaphong and Busso
[9]. Na and Kim [10] investigated three dimensional
thermo-mechanical buckling analysis for FG composite
plates that composed of ceramic, FGM, and metal layers
by using the finite element methods. Liew et al. [11]
presented the non-linear vibration analysis for layered
cylindrical panels containing FGMs and subjected to a
temperature gradient arising from steady heat conduction
through the panel thickness. Lia and Batra [12] investi-
gated buckling of axially compressed thin cylindrical
shells with the functionally graded middle layer. Sofiyev
et al. [13] and Sofiyev [14] studied vibration and stabil-
ity of three-layered truncated conical and cylindrical
shells containing an FGM layer subjected to an axial
compressive load, respectively.
For some cases, these shells are embedded in an elas-
tic medium. There are different approaches to analyze
the interaction between a structure and an ambient me-
dium. Most earthen soils can be appropriately repre-
sented by a mathematical model from Pasternak, whereas
sandy soils and liquids can be represented by Winkler’s
model [15].
Despite the evident importance of practical applica-
tions cylindrical shells, the investigations on vibration
and stability of homogeneous and FGM shells on elastic
foundations are limited in the literature [16-23]. The sta-
bility problem of composite cylindrical shells that com-
posed of ceramic-FGM-metal layers embedded in an
elastic medium, subjected to an axial compressive load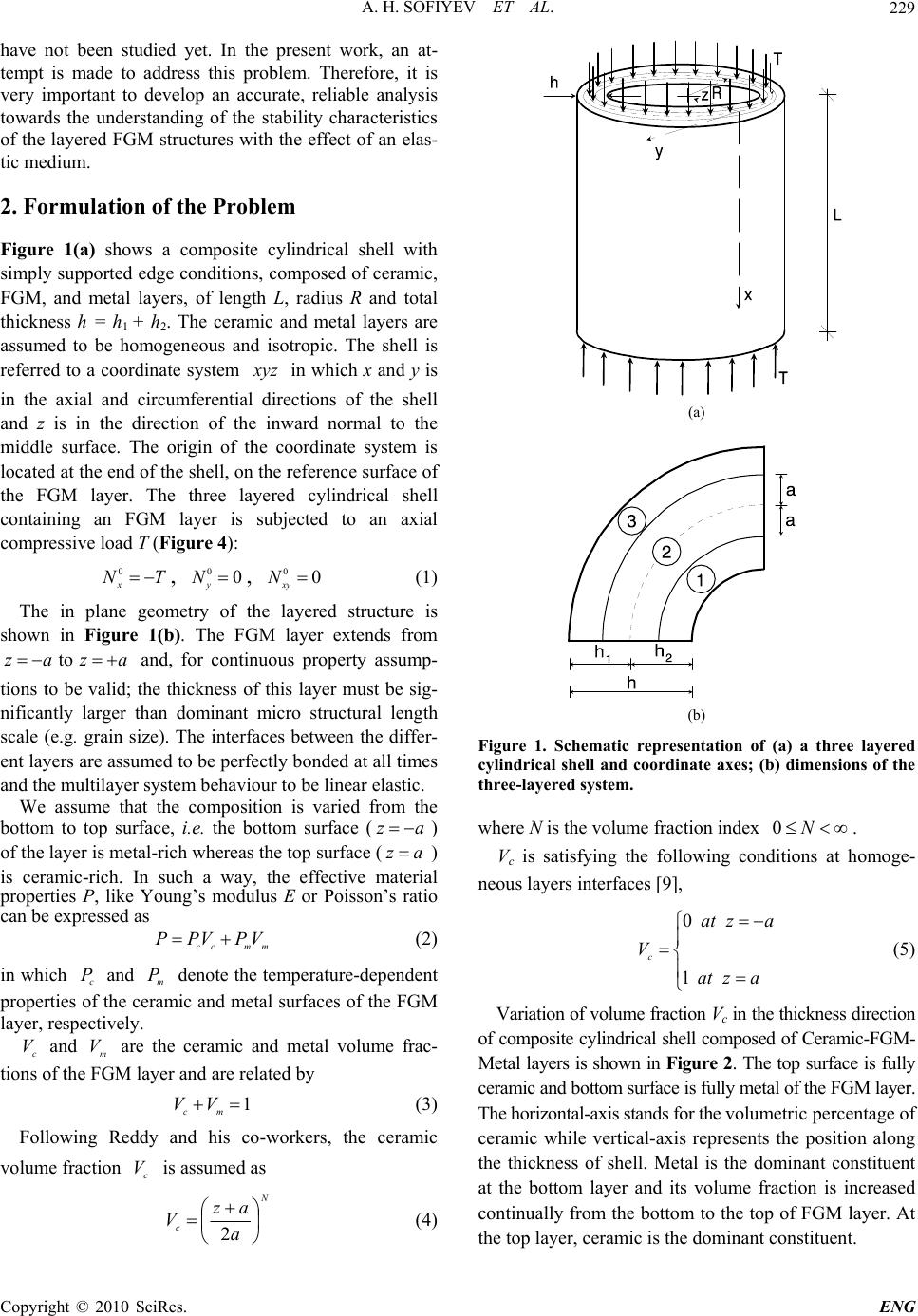

A. H. SOFIYEV ET AL. 229
have not been studied yet. In the present work, an at-
tempt is made to address this problem. Therefore, it is
very important to develop an accurate, reliable analysis
towards the understanding of the stability characteristics
of the layered FGM structures with the effect of an elas-
tic medium.
2. Formulation of the Problem
Figure 1(a) shows a composite cylindrical shell with
simply supported edge conditions, composed of ceramic,
FGM, and metal layers, of length L, radius R and total
thickness h = h1 + h2. The ceramic and metal layers are
assumed to be homogeneous and isotropic. The shell is
referred to a coordinate system
x
y
z
in which x and y is
in the axial and circumferential directions of the shell
and z is in the direction of the inward normal to the
middle surface. The origin of the coordinate system is
located at the end of the shell, on the reference surface of
the FGM layer. The three layered cylindrical shell
containing an FGM layer is subjected to an axial
compressive load T (Figure 4):
TNx
0, , (1) 0 0
y
N0
0
xy
N
The in plane geometry of the layered structure is
shown in Figure 1(b). The FGM layer extends from
and, for continuous property assump-
tions to be valid; the thickness of this layer must be sig-
nificantly larger than dominant micro structural length
scale (e.g. grain size). The interfaces between the differ-
ent layers are assumed to be perfectly bonded at all times
and the multilayer system behaviour to be linear elastic.
azaz  to
We assume that the composition is varied from the
bottom to top surface, i.e. the bottom surfacea
(
z


)
of the layer is metal-rich whereas the top surfacea (
z

)
is ceramic-rich. In such a way, the effective material
properties P, like Young’s modulus E or Poisson’s ratio
can be expressed as
mmcc VPVPP  (2)
in which and denote the temperature-dependent
properties of the ceramic and metal surfaces of the FGM
layer, respectively.
c
Pm P
c
V and are the ceramic and metal volume frac-
tions of the FGM layer and are related by
m
V
1

mc VV (3)
Following Reddy and his co-workers, the ceramic
volume fraction is assumed as
c
V
N
ca
az
V





2 (4)
(a)
(b)
Figure 1. Schematic representation of (a) a three layered
cylindrical shell and coordinate axes; (b) dimensions of the
three-layered system.
where N is the volume fraction index

 N0.
Vc is satisfying the following conditions at homoge-
neous layers interfaces [9],








azat
azat
Vc
1
0
(5)
Variation of volume fraction Vc in the thickness direction
of composite cylindrical shell composed of Ceramic-FGM-
Metal layers is shown in Figure 2. The top surface is fully
ceramic and bottom surface is fully metal of the FGM layer.
The horizontal-axis stands for the volumetric percentage of
ceramic while vertical-axis represents the position along
the thickness of shell. Metal is the dominant constituent
at the bottom layer and its volume fraction is increased
continually from the bottom to the top of FGM layer. At
the top layer, ceramic is the dominant constituent.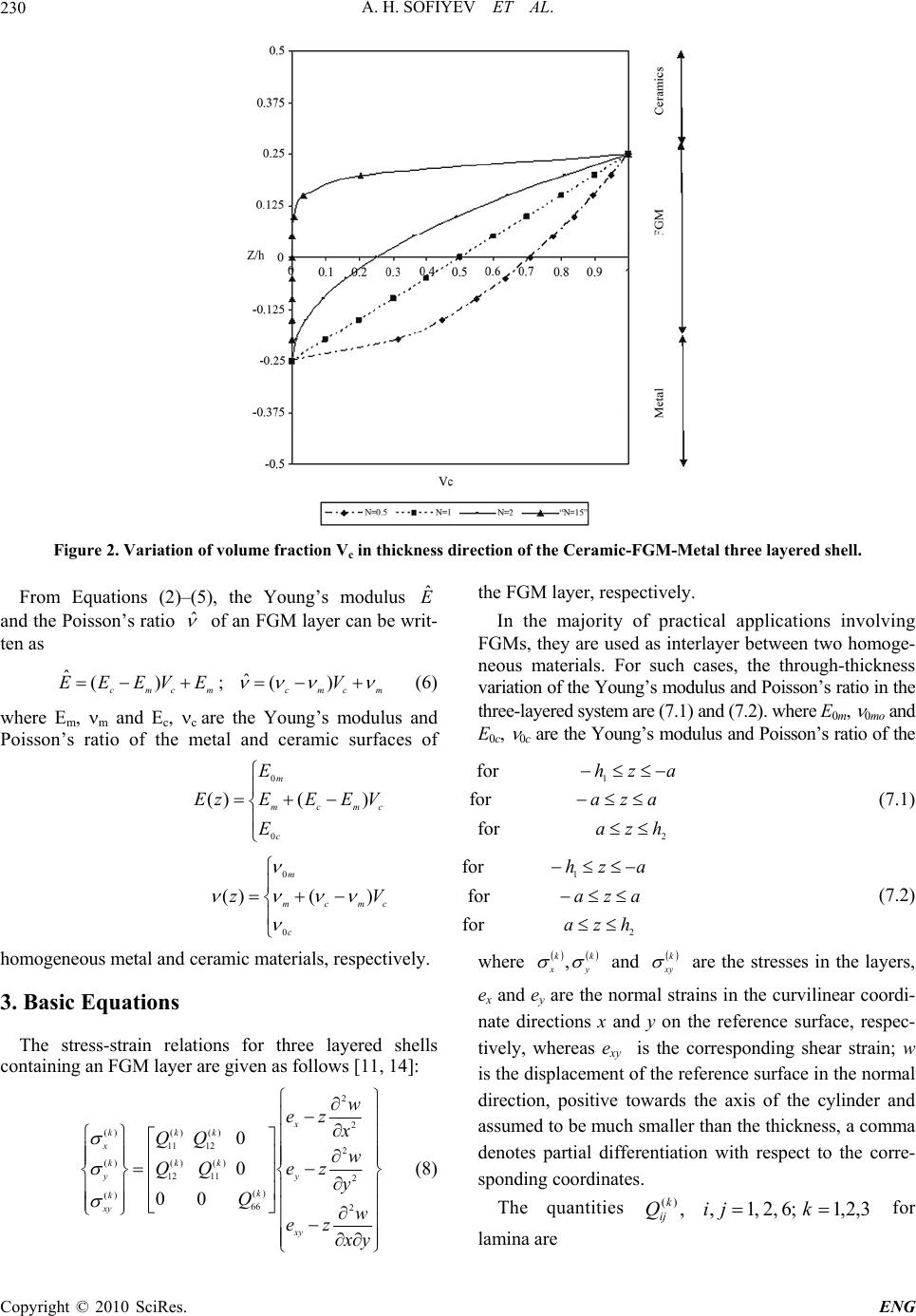

A. H. SOFIYEV ET AL. 230
Figure 2. Variation of volume fraction Vc in thickness direction of the Ceramic-FGM-Metal three layered shell.
From Equations (2)–(5), the Young’s modulus
and the Poisson’s ratio
E
ˆ

ˆ of an FGM layer can be writ-
ten as
mcmc EVEEE  )(
ˆ; mcmc V




 )(
ˆ (6)
where Em, m and Ec, c are the Young’s modulus and
Poisson’s ratio of the metal and ceramic surfaces of
the FGM layer, respectively.
In the majority of practical applications involving
FGMs, they are used as interlayer between two homoge-
neous materials. For such cases, the through-thickness
variation of the Young’s modulus and Poisson’s ratio in the
three-layered system are (7.1) and (7.2). where E0m, 
0mo and
E0c, 
0c are the Young’s modulus and Poisson’s ratio of the









20
10
for
for)(
for
)(
hzaE
azaVEEE
azhE
zE
c
cmcm
m
(7.1)









20
10
for
for)(
for
)(
hza
azaV
azh
z
c
cmcm
m




(7.2)
homogeneous metal and ceramic materials, respectively.
3. Basic Equations
The stress-strain relations for three layered shells
containing an FGM layer are given as follows [11, 14]:
























































yx
w
ze
y
w
ze
x
w
ze
Q
QQ
QQ
xy
y
x
k
kk
kk
k
xy
k
y
k
x
2
2
2
2
2
)(
66
)(
11
)(
12
)(
12
)(
11
)(
)(
)(
0 0
0
0



(8)
where



 k
y
k
x
 , and

 k
xy
 are the stresses in the layers,
ex and ey are the normal strains in the curvilinear coordi-
nate directions x and y on the reference surface, respec-
tively, whereas exy is the corresponding shear strain; w
is the displacement of the reference surface in the normal
direction, positive towards the axis of the cylinder and
assumed to be much smaller than the thickness, a comma
denotes partial differentiation with respect to the corre-
sponding coordinates.
The quantities for
lamina are
3,2,1;6 ,2 ,1, ,
)( kjiQ k
ij

A. H. SOFIYEV ET AL. 231
m
m
m
mm
m
mE
Q
E
Q
E
QQ
0
0
)1(
66
2
0
00
)1(
12
2
0
0
)1(
22
)1(
11 1
,
1
,
1 

 






2
)2(
22
)2(
11 )(1
)(
mcmc
mcmc
V
EVEE
QQ
 






2
)2(
12 )(1
)()(
mcmc
mcmcmcmc
V
VEVEE
Q







mcmc
mcmc
V
EVEE
Q
 

)(1
)(
)2(
66
c
c
c
cc
c
cE
Q
E
Q
E
QQ
0
0
)3(
66
2
0
00
)3(
12
2
0
0
)3(
22
)3(
11 1
,
1
,
1 

 




 (9)
The well-known force and moment resultants are ex-
pressed by [14, 25]
  
  



 3
1
1d ,,z 1, ,,(),,,
k
z
z
k
xy
k
y
k
xxyyxxyyx
k
k
zMMMNNN 
(10)
The relations between the forces and the
Airy stress function
yxNN ,, xy
N
h/ are given by














yxxy Nxyyx
2
2
2
2
2
,,N ,N , (11)
For the elastic foundation, one assumes the
two-parameter elastic foundation model proposed by
Pasternak [15]. The foundation medium is assumed to be
linear, homogenous and isotropic. The bonding between
the truncated conical shell and the foundation is perfect
and frictionless. If the effects of damping and inertia
force in the foundation are neglected, the foundation in-
terface pressure p may be expressed as













 2
2
2
2
y
w
x
w
KwKp pw (12)
where is the modulus of subgrade reaction for the
foundation and the shear modulus of the subgrade.
Note that by setting , the Pasternak model be-
comes that of the Winkler foundation model.
w
K
p
K
0
p
K
When the above assumptions are taken into considera-
tion, the modified Donnell type stability and compatibil-
ity equations of composite cylindrical shells that com-
posed of ceramic, FGM, and metal layers, subjected axial
compressive load and resting on the Pasternak founda-
tion are given, respectively by
2
2
2
22
2
2
2x
w
T
R
N
y
M
yx
M
x
Myyxy
x









0
2
2
2
2








 y
w
x
w
KwK pw




(13)
2
2
2
2
2
2
21
2x
w
Ryx
e
x
e
y
exyy
x








 (14)
where are moment resultants.
yxyx MMM ,,
Substituting expressions (8)-(11) and (12) in Equa-
tions. (13) and (14) a system of differential equations for
the stress function and the normal displacement can be
obtained in form as
0
L
L
2221
1211





















wL
L
(15)
where the following definitions apply:

2
2
22
4
51
4
4
4
4
211
1
2 xRyx AA
yx
AL 



















(16.1)

22
4
64
4
4
4
4
312 2 yx
AA
yx
AL 


























2
2
2
2
2
2
yx KK
x
Tpw




(16.2)

22
4
52
4
4
4
4
121 2 BLyx
BB
yx 















 (16.3)

22
4
63
4
4
4
4
4
2
2
22 2
R
1
Lyx BB
yx
B
x



















(16.4)
in which expressions are defined as
follows:
)61(, iBA ii
,
2211111 BCBCA 

,
1212112 BCBCA 

,
124213113 CBCBCA 


,
223214114 CBCBCA 


,
5615BCA

,
626616 CBCA 
,
101 DCB

,
202 DCB



 ,
101121203DCCCCB


 ,
102111204 DCCCCB

,/1 605CB

,/ 60616 CCB







2
20
2
10
/1 CCD  (17)

A. H. SOFIYEV ET AL. 232
in which expressions are
defined as follows:
)2,1,0(and,1621 111 kCCC kkk
d
11
1
12
0
0
1




a
h
k
m
m
kzz
E
C








211 d
1
d
)(1
)(
2
0
0
2
h
a
k
c
c
a
amcmc
mcmc
kzz
E
z
V
EVEE
z
 
(18.1)

















2
1
1
1
1
d
1
d
1
d
)(1
)()(
2
0
0
2
0
0
2
1
2
h
a
k
c
c
a
h
k
m
m
a
amcmc
mcmcmcmc
k
k
zz
E
zz
E
z
V
VEVEE
zC



(18.2)









a
amcmc
mcmc
k
a
h
k
m
m
kz
V
EVEE
zzz
E
Cd
)(1
)(
d
1
1
1
1
1
0
0
6



21d
10
0
h
a
k
c
czz
E

(18.3)
Equation (15) is the basic differential equations for the
vibration and stability of generic composite cylindrical
shells containing an FGM layer resting on a Pasternak
foundation.
4. Solution of Basic Equations
Consider a cylindrical shell with simply supported edge
conditions. The solution of the system of equation (15) is
sought as follows [24]:
R
ny
sin
m
sin ,
R
ny
sin
m
sin w11
R
x
R
x
  (19)
where LRmm/
1


y
, m is the half wave length in the di-
rection of the x-axis, is the wave number in the direc-
tion of the -axis,
n

and

are the amplitudes.
Substituting expression (19) in the equation set (15), ap-
plying Galerkin’s method in the ranges Lx

0 and
Ry

20 and eliminating ζ from the equations, the fol-
lowing equation for the critical axial load is obtained:
 
  
 

 
The minimum values of the critical axial load ()
are obtained by minimizing Equation (20) with respect to
m and n, the number of longitudinal and circumferential
buckling waves.
cr
wp
T
As 0

p
K, from Equation (20), in special case for
the critical axial load () of three layered cylindrical
shells containing an FGM layer on the Winkler founda-
tion is obtained.
cr
w
T
As 0


pw KK , from Equation (20), in special case
for the critical axial load (cr
T
) of three layered cylindri-
cal shells containing an FGM layer without an elastic
foundation is obtained.
5. Numerical Results and Discussions
The buckling loads of single-layer orthotropic and
(0o/90o/0o) cross-ply laminated graphite/epoxy circular
cylindrical shells under pure axial load are compared in
Table 1 with results of the Jones and Morgan [25], using
their material properties, i.e., E1 = 30  106 psi; E2 = 0.75 
106 psi; G12 = 0.375  106 psi; 
1 = 0.25;

2 = 0.0625, and
cylindrical shell parameters L = 34.64 in; R = 10.0 in; h =
0.12 in. It can be seen that the present results are in very
good agreement with results of Jones and Morgan [25].
As there are presently no results in the open literature
for the buckling of cylindrical shells under an axial load
and resting on elastic foundations, comparison of results
in this study is made with those of Paliwal et al. [17] for
the free vibration analysis of homogenous isotropic cy-
lindrical shells resting on a Winkler foundation. The
comparison is shown in Table 2.
When the inertial term is added into the left side of Equa-
tion (13), after integrating and after some mathematical
operations for the dimensionless frequency parame-
Table 1. Comparisons of dimensionless critical axial loads
for single-layer orthotropic and (0o/90o/0o) cross-ply lami-
nated cylindrical shells.
Jones and Morgan [25]Present study
Lay-up TcrL2/E2h3 TcrL2/E2h3
(0o) 1482 1482.0 (3,7)
(0o/90o/0o) 1859.8 1859.8 (3,6)


2
1
222
1
2
22
152
44
11
22
163
44
14
2
1
22
151
44
12
2
1
22
164
44
13
22
1
)(
2
2
2
2
1
m
RnmKKR
nmBBnmB
nmBBnmBRm
nmAAnmARm
nmAAnmA
Rm
T
pw
cr
wp





















(20)
Table 2. Comparison of the dimensionless frequency pa-
rameter for a cylindrical shell resting on the Winkler
foundation ().
1w
ω
R/h 34
wN/m10K2;L/R100;

Paliwal et al. [17] Present study
0.6788(1) 0.6792(1)
0.3639(2) 0.3646 (2)
0.2053(3) 0.2080(3)
0.1275(4) 0.1382(4)

A. H. SOFIYEV ET AL. 233
Based on the above comparisons of Tables 1 and 2, the ter w1w of the free vibration of FGM cylindrical shells
resting on the Winkler-Pasternak foundations, the fol-
lowing expression is obtained in Equation (21):
accuracy of the present study is validated.
5.1. Numerical Results
where the mass density per unit length defined as
 )(d )()(20
a
a-
10 ahzVahcmcmcmt 

 (22) The buckling analysis of three layered cylindrical shells
containing an FGM layer was conducted for ceramic and
metal combinations. The FGM material considered was
Zirconium oxide and Titanium alloy, referred to as
ZrO2/Ti–6Al–4V or FGM. The temperature dependence
This comparison is to ensure that the elastic foundation
effects have been correctly integrated into the present
formulation.
 



 

 









































222
1
22
152
44
11
22
163
44
14
2
1
22
151
44
12
2
1
22
164
44
13
4
1
/)(
2
2
2
2
1
1
RnmKK
nmBBnmB
nmBBnmBRm
nmAA
nmARm
nmAAnmA
R
pw
t
w


(21)
that the critical axial load of three layered cylindrical
shells containing an FGM layer increases gradually with
increasing or separately or together for all
compositional profiles. The effect of the composi ional
profiles on the critical axial loads reduce, as the consid-
ering affect of elastic foundations. Further more, for the
small values of and the variations of volume
fractions of the FGM has a considerable influence on the
values of the critical axial load. When the volume frac-
tion index N increases, the values of the critical axial
loads with and without elastic foundation decrease.
When the volume fraction index N increases, the effect
to the critical axial loads with and without elastic foun-
dation increases.
w
Kp K
w
Kp K
of FGM material properties can be expressed as
)1( 3
3
2
21
1
10 TPTPTPTPPP    (23)
in which (room temperature), P0, P-1, P1, P2 ,
P3 are the coefficients of temperature T (K) expressed
in Kelvin and are unique to the constituent materials
Reddy and Chin [4].
K300T
Typical values for ZrO2 and Ti–6Al–4V listed in Table
3. The materials are assumed to be perfectly elastic
throughout the deformation. E0c, E0m, 
0c,

0m, are the
Young’s modulus and Poisson’s ratio of the metal and
ceramic materials of the cylindrical shell, respectively.
Numerical computations, for pure metal, pure ceramic
and three layered cylindrical shells containing an FGM
layer with or without the Pasternak-Winkler foundations
have been carried out using expression (20). The results are
presented in Table 4 and Figures 4-7. The results given in
all tables below for the values of the critical axial load, cor-
responding to the numbers of longitudinal and circumferen-
tial waves (m,n) are presented in parentheses.
When the shear subgrade modulus is zero and the
Winkler foundation stiffness is changed, the effect on
the critical axial load () are 5.95%, 11.62%; 49.08% for
, respectively, for N =
0.5 of the FGM layer of three layered cylindrical shells.
p
K
w
K
/( mN
cr
w
T
10)5;10;105 3887 Kw
In Table 4 variations of the critical axial load and cor-
responding wave numbers for pure metal, pure ceramic
and three layered cylindrical shells containing an FGM
layer with different compositional profiles (N=0.5; 1; 2;
15), versus the Winkler foundation stiffness and the
shear subgrade modulus are presented. It is observed
w
K
p
K
When is keep constant () and the
Winkler foundation stiffness is changed the effect on
the critical axial load () are 25.82%, 31.48%; 69% for
, respectively, for N =
.5 of the FGM layer of three layered cylindrical shells.
p
K
;107
N/m10 6 
p
K
)
3
w
K
(N/m
cr
wp
T
10 8 5;105 8 
w
K
0
Table 3. Temperature-dependent coefficients of Young’s modulus E (MPa) and Poisson’s ratio  for ceramics and metals
(Reddy and Chin [4]).
Coefficients Zirconia (ZrO2) Titanium alloy (Ti-6Al-4V)
E
c (Pa) c

Em (Pa) m

P0 2.4427×1011 0.2882 1.2256×1011 0.2884
P-1 0 0 0 0
P1 -1.371×10-3 1.133×10-4 -4.586×10-4 -1.121×10-4
P2 1.214×10-6 0 0 0
P3 -3.681×10-10 0 0 0
P 1.68063×1011 0.2980 1.05698×1011 0.2981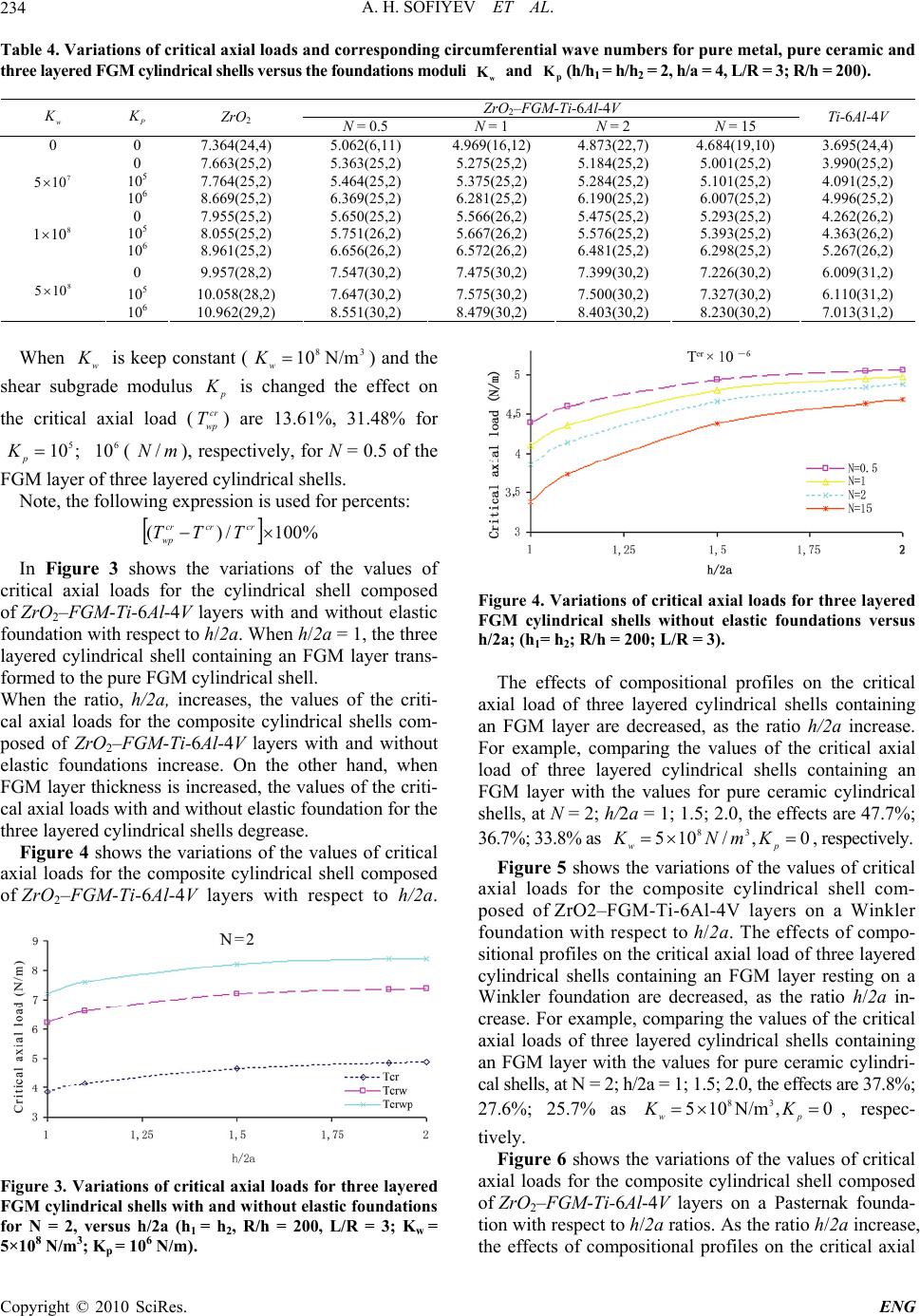

A. H. SOFIYEV ET AL. 234
Table 4. Variations of critical axial loads and corresponding circumferential wave numbers for pure metal, pure ceramic and
three layered FGM cylindrical shells versus the foundations moduli and (h/h1 = h/h2 = 2, h/a = 4, L/R = 3; R/h = 200).
w
Kp
K
ZrO2–FGM-Ti-6Al-4V
w
K P
K ZrO2 N = 0.5 N = 1 N = 2 N = 15 Ti-6Al-4V
0 0 7.364(24,4) 5.062(6,11) 4.969(16,12) 4.873(22,7) 4.684(19,10) 3.695(24,4)
0 7.663(25,2) 5.363(25,2) 5.275(25,2) 5.184(25,2) 5.001(25,2) 3.990(25,2)
105 7.764(25,2) 5.464(25,2) 5.375(25,2) 5.284(25,2) 5.101(25,2) 4.091(25,2)
7
105
106 8.669(25,2) 6.369(25,2) 6.281(25,2) 6.190(25,2) 6.007(25,2) 4.996(25,2)
0 7.955(25,2) 5.650(25,2) 5.566(26,2) 5.475(25,2) 5.293(25,2) 4.262(26,2)
105 8.055(25,2) 5.751(26,2) 5.667(26,2) 5.576(25,2) 5.393(25,2) 4.363(26,2)
8
101
106 8.961(25,2) 6.656(26,2) 6.572(26,2) 6.481(25,2) 6.298(25,2) 5.267(26,2)
0 9.957(28,2) 7.547(30,2) 7.475(30,2) 7.399(30,2) 7.226(30,2) 6.009(31,2)
105 10.058(28,2) 7.647(30,2) 7.575(30,2) 7.500(30,2) 7.327(30,2) 6.110(31,2)
8
105 
106 10.962(29,2) 8.551(30,2) 8.479(30,2) 8.403(30,2) 8.230(30,2) 7.013(31,2)
When is keep constant () and the
shear subgrade modulus is changed the effect on
the critical axial load () are 13.61%, 31.48% for
(), respectively, for N = 0.5 of the
FGM layer of three layered cylindrical shells.
w
K
510;
38 N/m10
w
K
p
K
cr
wp
T
6
10
p
KmN /
Note, the following expression is used for percents:


%100/)(  crcrcr
wp TTT
In Figure 3 shows the variations of the values of
critical axial loads for the cylindrical shell composed
of ZrO2–FGM-Ti-6Al-4V layers with and without elastic
foundation with respect to h/2a. When h/2a = 1, the three
layered cylindrical shell containing an FGM layer trans-
formed to the pure FGM cylindrical shell.
When the ratio, h/2a, increases, the values of the criti-
cal axial loads for the composite cylindrical shells com-
posed of ZrO2–FGM-Ti-6Al-4V layers with and without
elastic foundations increase. On the other hand, when
FGM layer thickness is increased, the values of the criti-
cal axial loads with and without elastic foundation for the
three layered cylindrical shells degrease.
Figure 4 shows the variations of the values of critical
axial loads for the composite cylindrical shell composed
of ZrO2–FGM-Ti-6Al-4V layers with respect to h/2a.
Figure 3. Variations of critical axial loads for three layered
FGM cylindrical shells with and without elastic foundations
for N = 2, versus h/2a (h1 = h2, R/h = 200, L/R = 3; Kw =
5×108 N/m3; Kp = 106 N/m).
Figure 4. Variations of critical axial loads for three layered
FGM cylindrical shells without elastic foundations versus
h/2a; (h1= h2; R/h = 200; L/R = 3).
The effects of compositional profiles on the critical
axial load of three layered cylindrical shells containing
an FGM layer are decreased, as the ratio h/2a increase.
For example, comparing the values of the critical axial
load of three layered cylindrical shells containing an
FGM layer with the values for pure ceramic cylindrical
shells, at N = 2; h/2a = 1; 1.5; 2.0, the effects are 47.7%;
36.7%; 33.8% as , respectively. 0,/105 38  pw KmNK
Figure 5 shows the variations of the values of critical
axial loads for the composite cylindrical shell com-
posed of ZrO2–FGM-Ti-6Al-4V layers on a Winkler
foundation with respect to h/2a. The effects of compo-
sitional profiles on the critical axial load of three layered
cylindrical shells containing an FGM layer resting on a
Winkler foundation are decreased, as the ratio h/2a in-
crease. For example, comparing the values of the critical
axial loads of three layered cylindrical shells containing
an FGM layer with the values for pure ceramic cylindri-
cal shells, at N = 2; h/2a = 1; 1.5; 2.0, the effects are 37.8%;
27.6%; 25.7% as , respec-
tively.
0,N/m105 38  pw KK
Figure 6 shows the variations of the values of critical
axial loads for the composite cylindrical shell composed
of ZrO2–FGM-Ti-6Al-4V layers on a Pasternak founda-
tion with respect to h/2a ratios. As the ratio h/2a increase,
the effects of compositional profiles on the critical axial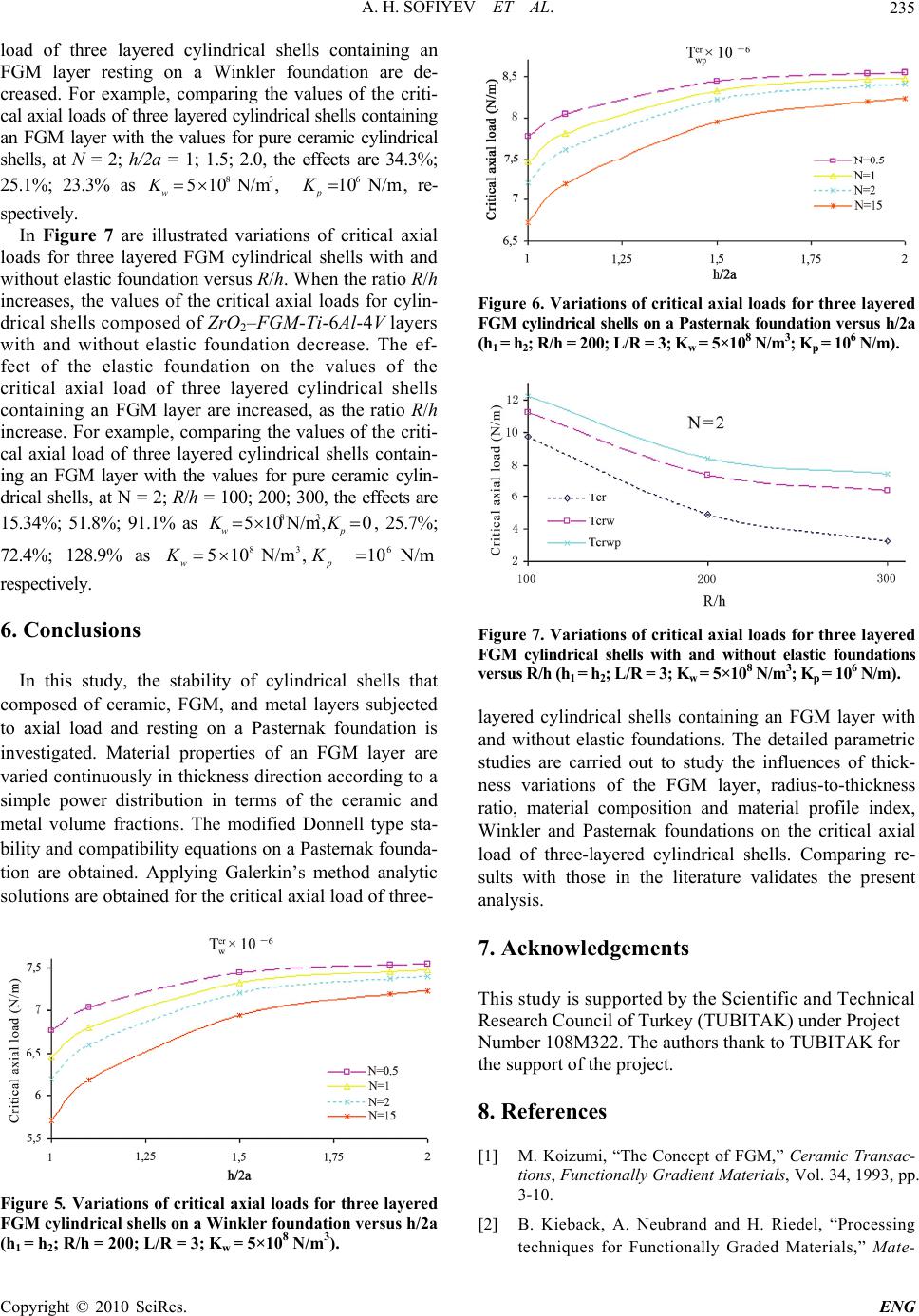

A. H. SOFIYEV ET AL. 235
load of three layered cylindrical shells containing an
FGM layer resting on a Winkler foundation are de-
creased. For example, comparing the values of the criti-
cal axial loads of three layered cylindrical shells containing
an FGM layer with the values for pure ceramic cylindrical
shells, at N = 2; h/2a = 1; 1.5; 2.0, the effects are 34.3%;
25.1%; 23.3% as , re-
spectively.
,N/m105 38 
w
KN/m106 
p
K
In Figure 7 are illustrated variations of critical axial
loads for three layered FGM cylindrical shells with and
without elastic foundation versus R/h. When the ratio R/h
increases, the values of the critical axial loads for cylin-
drical shells composed of ZrO2–FGM-Ti-6Al-4V layers
with and without elastic foundation decrease. The ef-
fect of the elastic foundation on the values of the
critical axial load of three layered cylindrical shells
containing an FGM layer are increased, as the ratio R/h
increase. For example, comparing the values of the criti-
cal axial load of three layered cylindrical shells contain-
ing an FGM layer with the values for pure ceramic cylin-
drical shells, at N = 2; R/h = 100; 200; 300, the effects are
15.34%; 51.8%; 91.1% as , 25.7%;
72.4%; 128.9% as
respectively.
0,N/m105 38  pw KK
p
K,N/m105 38 10
w
KN/m
6
6. Conclusions
In this study, the stability of cylindrical shells that
composed of ceramic, FGM, and metal layers subjected
to axial load and resting on a Pasternak foundation is
investigated. Material properties of an FGM layer are
varied continuously in thickness direction according to a
simple power distribution in terms of the ceramic and
metal volume fractions. The modified Donnell type sta-
bility and compatibility equations on a Pasternak founda-
tion are obtained. Applying Galerkin’s method analytic
solutions are obtained for the critical axial load of three-
Figure 5. Variations of critical axial loads for three layered
FGM cylindrical shells on a Winkler foundation versus h/2a
(h1 = h2; R/h = 200; L/R = 3; Kw = 5×108 N/m3).
Figure 6. Variations of critical axial loads for three layered
FGM cylindrical shells on a Pasternak foundation versus h/2a
(h1 = h2; R/h = 200; L/R = 3; Kw = 5×108 N/m3; Kp = 106 N/m).
Figure 7. Variations of critical axial loads for three layered
FGM cylindrical shells with and without elastic foundations
versus R/h (h1 = h2; L/R = 3; Kw = 5×108 N/m3; Kp = 106 N/m).
layered cylindrical shells containing an FGM layer with
and without elastic foundations. The detailed parametric
studies are carried out to study the influences of thick-
ness variations of the FGM layer, radius-to-thickness
ratio, material composition and material profile index,
Winkler and Pasternak foundations on the critical axial
load of three-layered cylindrical shells. Comparing re-
sults with those in the literature validates the present
analysis.
7. Acknowledgements
This study is supported by the Scientific and Technical
Research Council of Turkey (TUBITAK) under Project
Number 108M322. The authors thank to TUBITAK for
the support of the project.
8
. References
[1] M. Koizumi, “The Concept of FGM,” Ceramic Transac-
tions, Functionally Gradient Materials, Vol. 34, 1993, pp.
3-10.
[2] B. Kieback, A. Neubrand and H. Riedel, “Processing
techniques for Functionally Graded Materials,” Mate-

A. H. SOFIYEV ET AL. 236
rials Science and Engineering A, Structural Materials:
Properties, Microstructure and Processing, Vol. 362, No.
1-2, 2003, pp. 81-105.
[3] V. Birman, “Buckling of Functionally Graded Hybrid
Composite Plates,” Proceedings of the 10th Conference
on Engineering Mechanics, Boulder, USA, 1995.
[4] J. N. Reddy and C. D. Chin, “Thermomechanical Analy-
sis of Functionally Graded Cylinders and Plates,” Journal
of Thermal Stresses, Vol. 21, 1998, pp. 593-602.
[5] T. Y. Ng, K. Y. Lam, K. M. Liew and J. N. Reddy, “Dy-
namic Stability Analysis of Functionally Graded Cylin-
drical Shells under Periodic Axial Loading,” Interna-
tional Journal of Solids Structures, Vol. 38, No. 8, 2001,
pp. 1295-1309.
[6] A. H. Sofiyev, “Dynamic Buckling of Functionally
Graded Cylindrical Shells under Non-periodic Impulsive
Loading,” Acta Mechanica, Vol. 165, No. 3-4, 2003, pp.
153-162.
[7] H. S. Shen and N. Noda, “Post-buckling of FGM Cylin-
drical Shell under Combined Axial and Radial Mechani-
cal Loads in Thermal Environments,” International
Journal of Solids Structures, Vol. 42, No. 16, 2005, pp.
4641- 4662.
[8] H. S. Shen, “Functionally Graded Materials: Nonlinear
Analysis of Plates and Shells,” CRC Press, Boca Raton,
2009.
[9] S. Pitakthapanaphong and E. P. Busso, “Self-consistent
elasto-plastic Stress Solutions for Functionally Graded
Material Systems Subjected to Thermal Transients,”
Journal of Mechanics and Physics of Solids, Vol. 50, No.
4, 2002, pp. 695-716.
[10] K. S. Na and J. H. Kim, “Three-dimensional Thermo-
mechanical Buckling Analysis for Functionally Graded
Composite Plates,” Composite Structures, Vol. 73, No. 4,
2006, pp. 413-422.
[11] K. M. Liew, J. Yang and Y. F. Wu, “Nonlinear Vibration
of a Coating-FGM-substrate Cylindrical Panel Subjected
to a Temperature Gradient,” Computer Methods in Ap-
plied Mechanics and Engineering, Vol. 195, No. 9-12,
2006, pp. 1007-1026.
[12] S. R. Lia and R. C. Batra, “Buckling of Axially Com-
pressed Thin Cylindrical Shells with Functionally Graded
Middle Layer,” Thin Walled Structures, Vol. 44, No. 10,
2006, pp. 1039-1047.
[13] A. H. Sofiyev, A. Deniz, I. H. Akcay and E. Yusufoglu,
“The Vibration and Stability of a Three-layered Conical
Shell Containing a FGM Layer Subjected to Axial Com-
pressive Load,” Acta Mechanica, Vol. 183, No. 3-4, 2006,
pp. 129-144.
[14] A. H. Sofiyev, “Vibration and Stability of Composite
Cylindrical Shells Containing a FG Layer Subjected to
Various Loads,” Structural Engineering and Mechanics
an International Journal, Vol. 27, No. 3, 2007, pp. 365-
391.
[15] P. L. Pasternak, “On a New Method of Analysis of an
Elastic Foundation by Means of Two Foundation Con-
stants,” Gosudarstvennoe Izdatelstvo Literaturi po Stroitel-
stvu I Arkhitekture, Moscow, 1954.
[16] V. A. Bajenov, “The Bending of the Cylindrical Shells in
an Elastic Medium,” Visha Shkola, Kiev, 1975.
[17] D. N. Paliwal, R. K. Pandey and T. Nath, “Free Vibration
of Circular Cylindrical Shell on Winkler and Pasternak
Foundation,” International Journal of Pressure Vessels
and Piping, Vol. 69, 1996, pp. 79-89.
[18] T. Y. Ng and K. Y. Lam, “Free Vibrations Analysis of
Rotating Circular Cylindrical Shells on an Elastic Foun-
dation,” Journal of Vibration and Acoustics, Vol. 122, No.
1, 2000, pp. 85-89.
[19] H. G. Tj, T. Mikami, S. Kanie and M. Sato, “Free Vibra-
tion Characteristics of Cylindrical Shells Partially Buried
in Elastic Foundations,” Journal of Sound and Vibration,
Vol. 290, No. 3, 2006, pp. 785-793.
[20] A. H. Sofiyev, S. N. Keskin and Al. H. Sofiyev, “Effects
of Elastic Foundation on the Vibration of Laminated Non-
homogeneous Orthotropic Circular Cylindrical Shells,”
Journal of Shock and Vibration, Vol. 11, 2004, pp. 89-101.
[21] Z. Q. Cheng and S. Kitipornchai, “Membrane Analogy of
Buckling and Vibration of Inhomogeneous Plates,”
Journal of Engineering Mechanics–ASCE, Vol. 125, No.
11, 1999, pp. 1293-1297.
[22] Z. Y. Huang, C. F. Lu and W. Q. Chen, “Benchmark
Solutions for Functionally Graded Thick Plates Resting
on Winkler–Pasternak Elastic Foundations,” Composite
Structures, Vol. 85, No. 2, 2008, pp. 95-104.
[23] P. Malekzadeh, “Three-dimensional Free Vibration Analysis
of Thick Functionally Graded Plates on Elastic Founda-
tions,” Composite Structures, Vol. 89, No. 3, 2009, pp.
367-373.
[24] V. L. Agamirov, “Dynamic problems of nonlinear shells
theory”, Nauka, Moscow, 1990.
[25] R. M. Jones and H. S. Morgan, “Buckling and Vibration
of Cross-ply Laminated Circular Cylindrical Shells,”
American Institute of Aeronautics and Astronautics
Journal, Vol. 13, No. 5, 1975, pp. 664-671.