 Applied Mathematics, 2011, 2, 1453-1461 doi:10.4236/am.2011.212207 Published Online December 2011 (http://www.SciRP.org/journal/am) Copyright © 2011 SciRes. AM Propagation of Torsional Surface Waves under the Effect of Irregularity and Initial Stress Shishir Gupta, Dinesh K. Majhi, Sumit K. Vishwakarma, Santimoy Kundu Department of Ap pl i e d M athematics, Indi an Sch o ol of Mi nes, Dhanbad, India E-mail: shishir_ism@yahoo.com Received August 24, 2011; revised October 14, 2011; accepted October 23, 2011 Abstract The present paper has been framed to study the influence of irregularity, initial stress and porosity on the propagation of torsional surface waves in an initially stressed anisotropic poro-elastic layer over a semi-infi- nite heterogeneous half space with linearly varying rigidity and density due to irregularity at the interface. The irregularity has been taken in the half-space in the form of a parabola. It is observed that torsional sur- face waves propagate in this assumed medium. In the absence of irregularity the velocity of torsional surface wave has been obtained. Further, it has been seen that for a layer over a homogeneous half space, the velo- city of torsional surface waves coincides with that of Love waves. Keywords: Irregularity, Torsional Surface Waves, Anisotropic, Initial Stress 1. Introduction The study of surface waves in a half-space is important to seismologists due to its possible applications in Geo- physical prospecting and in understanding the cause and estimation of damage due to earthquakes. Surface waves carry the greatest amount of energy from shallow shocks and are of primary cause of destruction that can result from earthquakes. The propagation of surface waves in detail are well documented in the text book literature (Achenbach, J. D. [1], Ewing, W. M., Jardetzky, W. S., Press, F. [2], Bath, M. [3]). One type of surface wave may be available in non-homogeneous earth known as torsional surface waves. These waves are horizontally polarized but give a twist to the medium when it propa- gates. Although much information is available on the propagation of surface waves such as Rayleigh waves, Love waves and Stonely waves etc., the torsional wave has not drawn much attention and very little literature is available on the propagation of this wave. Some papers have been published on the propagation of torsional waves in elastic medium with different types of inho- mogeneity. Lord Rayleigh [4] in his remarkable paper showed that the isotropic homogeneous elastic half-space does not allow a torsional surface wave to propagate, in this connection Georgiadis et al. [5] have examined the torsional surface wave in a linear gradient-elastic half- space. Meissner [6] pointed out that in an inhomogene- ous elastic half-space with quadratic variation of shear modulous and density varying linearly with depth, tor- sional surface waves do exist. Vardoulakis, I. [7] has stu- died the problem on torsional surface waves in inhomo- geneous elastic media. Also S. Dey et al. [8] studied the propagation of torsional waves in a homogeneous sub- stratum over a heterogeneous half-space. The study of porous medium in recent time has ac- quired prime interest. The layer of the earth usually of such materials and the medium is generally dealt under the name of poro-elastic medium. Investigation on pro- pagation of waves in liquid saturated porous solids are relevant to geophysical prospecting methods, survey techniques are very useful in oil industry. While deriving the mechanics of such medium it is assumed that the pore sizes are small and macroscopially speaking their average distribution is uniform. The role of pore water in seismology has been emphasized in many studies. Biot [9] has established the theory of the propagation of elas- tic waves in a porous elastic solid saturated by a viscous fluid. Under the assumption of dynamic coupling be- tween solid and fluid mass, Biot [10] has developed the mathematical theory for th e propagation of elastic waves in a fluid saturated porous medium. Based on this theory, many problems of surface waves in poro-elastic materi- als have been studied in the past years by Buckingham, M. J. [11], Sharma, M. D. and Gogna, M. L. [12], Shar- ma, M. D., Kumar, R. and Gogna, M. L. [13], Sharma, M. D., Kumar, R. and Gogna, M. L. [14]. The development of initial stresses in the medium is  S. GUPTA ET AL. 1454 due to many reasons, for example resulting from the dif- ference of temperature, process of quenching, shot peen- ing and cold working, slow process of creep, differential external forces, gravity variations etc. These stresses have a pronounced influence on the propagation of waves as shown by Biot [15]. The earth is also an initially stressed medium. It is therefore of much interest to study the in- fluence of these stresses on the propagation of torsional surface wave. The study of surface waves in an initially stressed medium is of interest not for theoretical taste only but for practical purposes too. Based on the pio- neering work of Biot [15] on pre-stressed solids, various studies of body and surface wave propagation in the pre- stressed solids have been carried out by many research- ers such as Chattopadhyay et al. [16], Kar, B. K. and Kalyani, V. K. [17] and Dey, S. and Addy, S. K. [18] . The study of wave propagation in elastic medium with different irregularities is of great importance to seismo- logists as well as to geophysicists to understand and pre- dict the seismic behavior at the different margins of earth. This fact leads us towards this study. The present paper discusses the possibility of existence of torsional surface wave in an initially stressed anisotropic poro-elastic layer over a semi-infinite heterogeneous half space with line- arly varying rigidity and density du e to irregularity at the interface. The irregularity has been taken in the half- space in the form of a parabola. It is observed that tor- sional surface waves propagate in this assumed medium. Irregularity and initial stress play an importan t role in th e seismic wave propagation. This paper has been framed out to show the effect of irregularity at the interface in the parabolic form and initial stress on the propag ation of torsional surface wave. As the porosity parameter de- creases, the half-space will become an elastic solid with less pores and the velocity of torsional surface waves decreases and ultimately vanishes when the medium is elastic solid. The effect of irregularity and the initial stresses are very prominent on the propagation of tor- sional surface waves. The presence of initial compressive stress reduces the velocity of torsional surface waves. As the initial compressive stress increases, the velocity of propagation decreases. It is also observed that in the li- miting case if the porous medium changes to a liquid layer then a torsional surface wave don’t exist. It is interesting to note that in a poro-elastic medium over a homogene- ous half-space the torsional wave mode changes to Love wave mode. Parabolic irregularity is mostly found in the earth so the present study can help the seismologists to understand and predict the seismic behaviour at different margins of the earth. 2. Formulation of the Problem Let us Consider a model which consists of a water satu- rated porous layer M1 of thickness H with anisotropy of Weiskopf type under compressive initial stresses rr Ps along the radial direction and with one para- bolic irregularity on the interface between the layer and a semi-infinite non-homogeneous elastic half-space M2 as shown in Figure 1. The heterogeneity has been consid- ered both in density and rigidity. We assume that the irregularity is of the form of a parabola with span of length 2 m and depth h. Assuming the origin of the cylindrical co-ordinate system at the middle point of the interface irregularity and the z-axis downward positive, the following varia- tion in rigidity and density has been taken 1) for the layer, 0 , o 2) for the half-space, 11 1, 1az bz where and are rigidity and density of the media respectively and are constants having dimensions that are inverse of length. , ab The equation of irregularity has b e en taken as 2 2 1 for ,where 0 for r hr m zFr Fr rm m and 2 h m and 1 . 3. Solution of the Problem 3.1. Solution for Porous Layer The dynamical equations of initially stressed poroelastic medium is obtained by suitably coupling Biot’s [19] dy- namical equations of an initially stressed medium with the equations of poro-elasticity given by Weiskopf [20] and Biot [9,10]. Those are 2 2 1rrr rr rz rr rrr sss ss P rrz rz uU t (1) 2 2 12 rz r rr r sss sP rr zrr vV t (2) 2 2 11 z rzzz rz rr zrz s ss sP rr zrr wW t (3) and 2 2rr r suU rt (4) Copyright © 2011 SciRes. AM  S. GUPTA ET AL.1455 Figure 1. Geometry of the problem. 2 2r svV t (5) 2 2rz z swW zt (6) where ij ,,uv =incremental stress components; rz = components of the displacement vector of the solid; w ,, r UVW z = components of the displacement vector of the liquid; = stress in the liquid, and 11 , 22 () 1 2 rz r z v wu r rz z rv v rr w r (7) are the components of the rotational vector . The stress-strain relations for the water saturated ani- sotropic porous layer under normal initial stress P are 2 2 2,2, 2 rr rrzz rr zz zz rrzz rrzz rzzr APeA NPeFPeQ sAN eAeFeQ sFeFeCeQ sNesGesGe (8) where ,,, FCN N and are elastic constants of the medium. and G are, in particular, shear moduli of the anisotropic layer in the radial and the z-direction re- spectively. G zz 1 , , 111 1 , 22 1 2 rrz rr r rz zr zr v uuw ee e rrr z vv v u ee rrr zr wu erz z w (9) Further, Q being the measure of coupling between the volume change of the solid and the liquid is a posi- tive quantity. s is the stress vector due to the liquid. The stress vector sis related to the fluid pressure by the relation P sP (10) where f is porosity of the layer. The mass coefficients , rr r and are related to the densities ,, w of the layer, the solid and the water respectively by 1, rrs rwr f (11) so that the mass density of the aggregate is ws f (12) The above relation shows that in case the fluid of lighter density w is filled up in the solid matrix of density then the density of the aggregate will be less than the density of the solid , there may be the case of heavier fluid such as mercury, molten metal etc. filled in the solid matrix, when the density of the aggregate will be more than that of solid. Further this relation shows that as the porosity factor f decreases from 1 to 0 i.e. as the volume of pores decreases, the density of the aggregate tends to the density of the solid. It has been shown by Biot that the mass coefficients obey the following inequalities also 2 0, 0 , 0 rrrrrr 0, (13) For torsional surface waves propagating along the ra- dial direction having displacement of the particles along direction we have, 0,, , 0,, , rz vvrzt VVrzt 0, 0, rz uw UW (14) The above displacements will produce e and r e strain components and the other strain components will be zero. Hence the stress-strain relations are 2,2 zr r Ge sNe (15) Using (15) in (1) to (6) the equations of motion, which are not automatically satisfied, are 2 2 rz z rrr ss 2r Pv rz r t V r (16) 0 rvV 2 2 t (17) Using stress-strain relations (8), (16) may be written as 22 22 2 2 2 1 2 rr r vv vv P NG rr rr z vV t (18) Copyright © 2011 SciRes. AM  S. GUPTA ET AL. 1456 y From (17), we have rvVdsa r dv V Now, 2 2 22 rr r v vVd tt , (19) where 2 r rr d Using (19) in (18), we get 22 222 2 1 2 vv vvv P NG rr rrz t 2 d (20) From Equation (20) it is clear that the velocity of the shear wave along radial(r) direction is 1 2 2 P N d and that along z-direction is 1 2. G d Now the shear wave velocity in the porous medium along radial direc- tion may be expressed as, 1 2 2 1,c d where N c is the velocity of the shear wave in the corresponding initial stress free non-porous anisotropic elastic medium along the radial direction, 2 P N is the non-dim ensional param eter due to the initial stress and the density ratio, P 22 12 11 22 1r rr d d where 11 1222 ,, r rr are the non-di- mensional parameters for the material of the porous layer as obtained by Biot. Introducing the non-dimensional radial and depth co- ordinates we have 0 ,, rz RLL 0 being a constant and 1 L being wave number where 1 k 22 2 22 2 1 22 vvv v GdL PP RR RR NN 2 2 v t (21) We assume a solution of Equation (21) of the form 21 eit vv JR , where 1 R is the Bessel func- tion of the first kind and 2 v is the solution of the following equation 22 22 2 d0 d vqv (22) where 22 2 1 2 2 Ld P N qGP N The solution of Equation (22) may be taken as 2iq iq vAeBe Therefore, for the torsional surface wave propagating in the radial direction, the so lution of Equation (21) may be taken as 1iqiqi t vJRAeBe e (23) where 2 22 1, c qd d c where , Nc GL is the phase velocity of the torsional surface wave. 3.2. Solution for Non-Homogeneous Elastic Half Space The lower medium is considered as non-homogeneous elastic half space. The Equaiton of motion may be writ- ten as 2 2 2 rz rv rrzt (24) where r and are stress components in the half space, ,,z vr is the displacement and is the density of the material of the half space. The non-homogeneity in the medium are taken as 11 1, 1az bz (25) where, 1 and 1 are the values of and re- spectively at 0z , and are constants having di- mensions that are inverse of length. ,ab Using the stress-strain relations , rz vv v rr z (26) L, the Equation (20) may be written as Copyright © 2011 SciRes. AM  S. GUPTA ET AL.1457 and the relations (25), the Equation of motion (24) may be written as 221 222 1 1 1 11 bz vvvvav rrazz az rrz 2 2 v t (27) Introducing the non-dimensional co-ordinates 0 and , rz RLL Equation (27) takes the form as 22 2220 22 0 12 10 1 1 1 1 vvvvLa RR RR aL bL Lv aL t v (28) We assume a solution of Equation (28) of the form 21 , it vv JRe where 2 is the solution of the following equation 222 20 202 20 1 dd 1d d 110 1 vv aL aL bL cv aL c L (29) In the above, c is the phase velocity of the to r- sional surface wave and 1 11 ,c velocity of shear wave in an initially stress-free elastic medium and 1 R is the Bessel function of the first kind. Substituting 1 2 21 2 0 1 L v a L in the Equa- tion (29) to eliminate the term 2 d d v , we obtain 220 22 10 0 1 1 1 1 4 0 b ac L ca aL L (30) Using in (30), where 0 21pa aL 2 11 0 4 4 s (31) where 2 222 1 2 cabL scpaL ,2 22 1 1bc pac Equation (31) is Whittaker’s equ a tion. We are interested in the solution of Equation (31) which is bounded and vanishes as vz , therefore we search for the solution which gives as 0 z. This condition is equivalent to 0 lim . Therefore the solution of Equation (31) satisfying the above condition may be written as 1,0 1 1 12 2 () 2 sit DW vJ a Lp Re (32) 4. Boundary Conditions and Dispersion Equation The boundary conditions are as follows 1) 00 at vzH z 2) at vvz Fr 3) 01 at rr zFr where 00 0 r vv ln z , 11 1 rvv ln z and ,0,ln are components of unit normal (to the in- terface at z = 0). 0,1 at ln zH 22 22 1 , 11 at F ln F zFr ; where d d Fr The boundary condition (3) may be written as 00 22 22 11 22 22 1 1'1 1 11 vv v F rr z FF vv Fv rr z FF , at zFr we get Now, using boundary conditions (1), (2) and (3) re- spectively we get Copyright © 2011 SciRes. AM 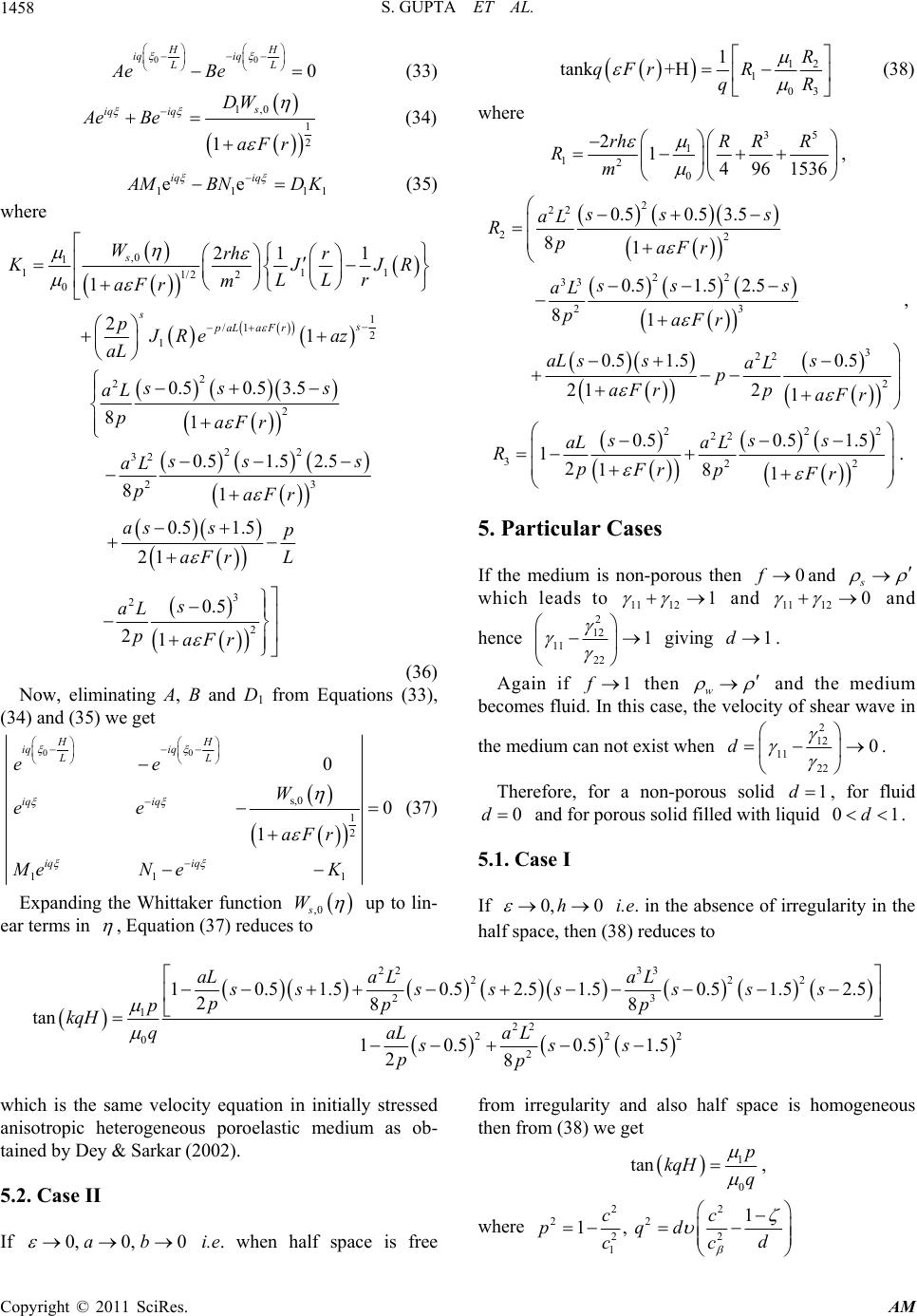 S. GUPTA ET AL. Copyright © 2011 SciRes. AM 1458 00 0 HH iq iq LL Ae Be 12 103 1 tank +HR qFr R qR (38) (33) 1,0 1 2 1 s iqiq DW Ae Be aFr (34) where 35 1 120 21496 1536 rhR RR Rm , 11 ee iqiq11 MBND K (35) where ,0 1 111 1/22 0 1 /1 2 1 2 2 2 22 32 23 3 2 21 1 1 21 0.50.5 3.5 81 0.51.5 2.5 81 0.5 1.5 21 0.5 21 s ss paL aFr Wrh r JJR LL r m aF r pJRe az aL sss aL paFr ss s aL paF r as sp L aF r s aL pa 2 22 22 22 33 23 3 22 2 0.50.53.5 81 0.51.5 2.5 81 0.5 1.50.5 2 21 1 ss s aL RpaFr ss s aL paFr aL sss aL pp aFr aFr , 22 22 322 0.50.5 1.5 128 11 sss aLa L Rpp Fr Fr 2 . 5. Particular Cases If the medium is non-porous then and 0fs which leads to 11 121 and 11 120 and 2 Fr hence 2 12 11 22 1 giving d. 1 (36) Again if then w 1f and the medium becomes fluid. In this case, the velocity of shear wave in Now, eliminating A, B and D1 from Equations (33), (34) and (35 ) we g et the medium can not exist when 2 12 11 22 0d . 00 s,0 1 2 11 0 0 1 HH iq iq LL iq iq iq iq ee W ee aFr MeN eK Therefore, for a non-porous solid , for fluid 1d 0d and for porous solid filled with liquid 01d . 1 (37) 5.1. Case I Expanding the Whittaker function ,0s W up to lin- ear terms in , Equation (37) reduce s to If 0, 0h i.e. in the absence of irregularity in the half space, then (38) reduces to 22 33 22 23 122 222 02 10.5 1.50.52.5 1.50.51.52.5 288 tan 10.50.51.5 28 aLaLa L ssssss s s ppp p kqH qaLa L sss pp 2 which is the same velocity equation in initially stressed anisotropic heterogeneous poroelastic medium as ob- tained by Dey & Sarkar (2002). 5.2. Case II If 0, 0, 0ab i.e. when half space is free from irregularity and also half space is homogeneous then from (3 8) we g et 1 0 tan p kqH q , where 22 22 22 1 1 1, cc pqd d cc 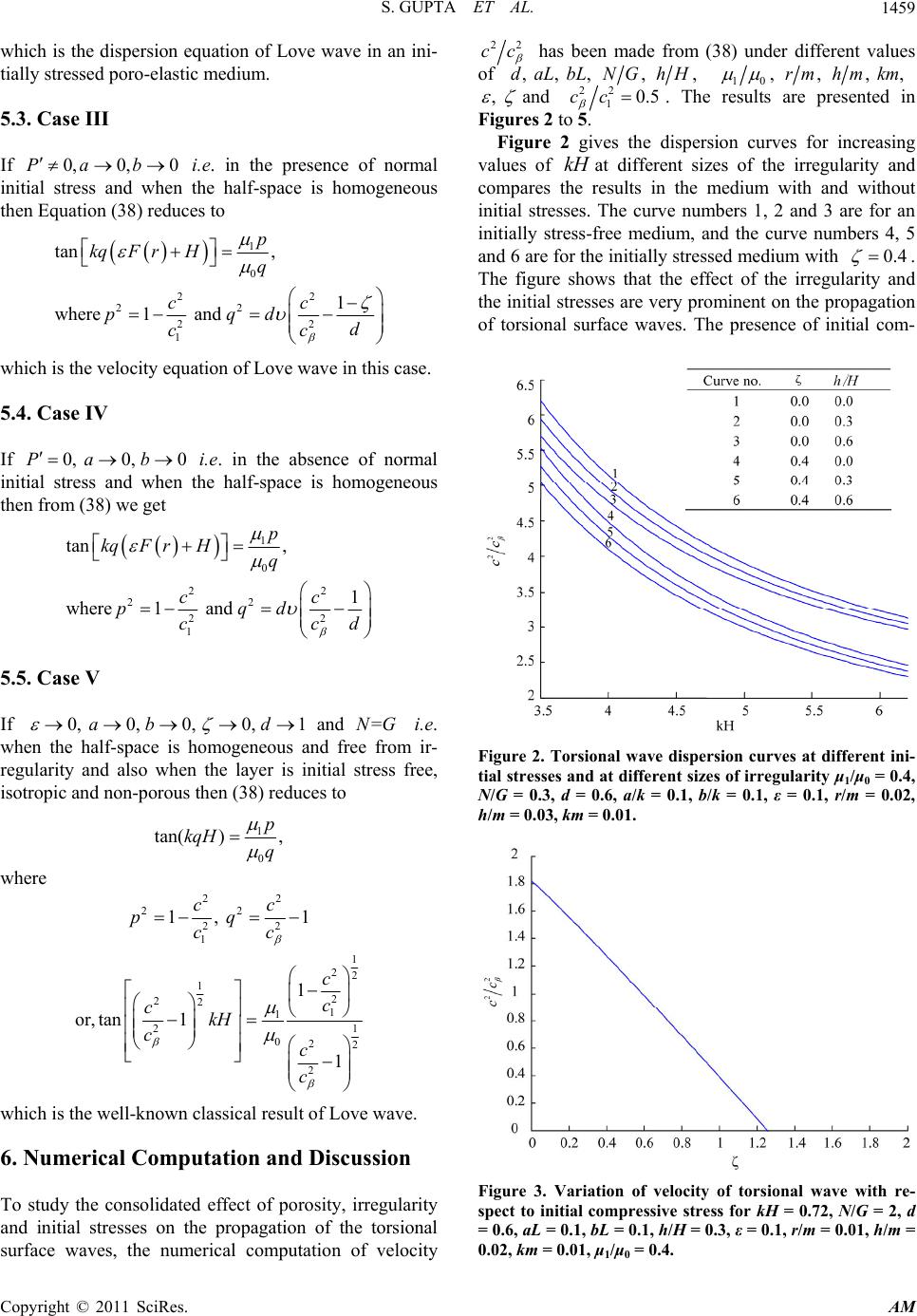 S. GUPTA ET AL.1459 which is the dispersion equation of Love wave in an ini- tially stressed poro-elastic medium. 5.3. Case III If i.e. in the presence of normal initial stress and when the half-space is homogeneous then Equation (38) reduces to 0,0, 0Pab 1 0 22 22 22 1 tan, 1 where 1 and p kqF rHq cc pqd d cc which is the velocity equation of Love wave in this case. 5.4. Case IV If i.e. in the absence of normal initial stress and when the half-space is homogeneous then from (3 8) we g et 0, 0, 0Pab 1 0 22 22 22 1 tan, 1 where 1 and p kqF rHq cc pqd d cc 5.5. Case V If 0, 0, 0, 0, 1 and =abd NG i.e. when the half-space is homogeneous and free from ir- regularity and also when the layer is initial stress free, isotropic and non-porous then (38) re duces to 1 0 tan(), p kqH q where 22 22 22 1 1, 1 cc pq cc 1 22 12 221 1 21 022 2 1 or,tan 1 1 c c ckH cc c which is the well-known classical result of Love wave. 6. Numerical Computation and Discussion To study the consolidated effect of porosity, irregularity and initial stresses on the propagation of the torsional surface waves, the numerical computation of velocity 22 cc has been made from (38) under different values of , , , , ,daLbLNG hH 10 , , , rmhmkm, , and 22 10.5cc . The results are presented in Figures 2 to 5. Figure 2 gives the dispersion curves for increasing values of at different sizes of the irregularity and compares the results in the medium with and without initial stresses. The curve numbers 1, 2 and 3 are for an initially stress-free medium, and the curve numbers 4, 5 and 6 are for the initially stressed medium with kH 0.4 . The figure shows that the effect of the irregularity and the initial stresses are very prominent on the propagation of torsional surface waves. The presence of initial com- Figure 2. Torsional wave dispersion curves at different ini- tial stresses and at different size s of irregularity μ1/μ0 = 0.4, N/G = 0.3, d = 0.6, a/k = 0.1, b/k = 0.1, ε = 0.1, r/m = 0.02, h/m = 0.03, km = 0.01. Figure 3. Variation of velocity of torsional wave with re- spect to initial compressive stress for kH = 0.72, N/G = 2, d = 0.6, aL = 0.1, bL = 0.1, h/H = 0.3, ε = 0.1, r/m = 0.01, h/m = 0.02, km = 0.01, μ1/μ0 = 0.4. Copyright © 2011 SciRes. AM 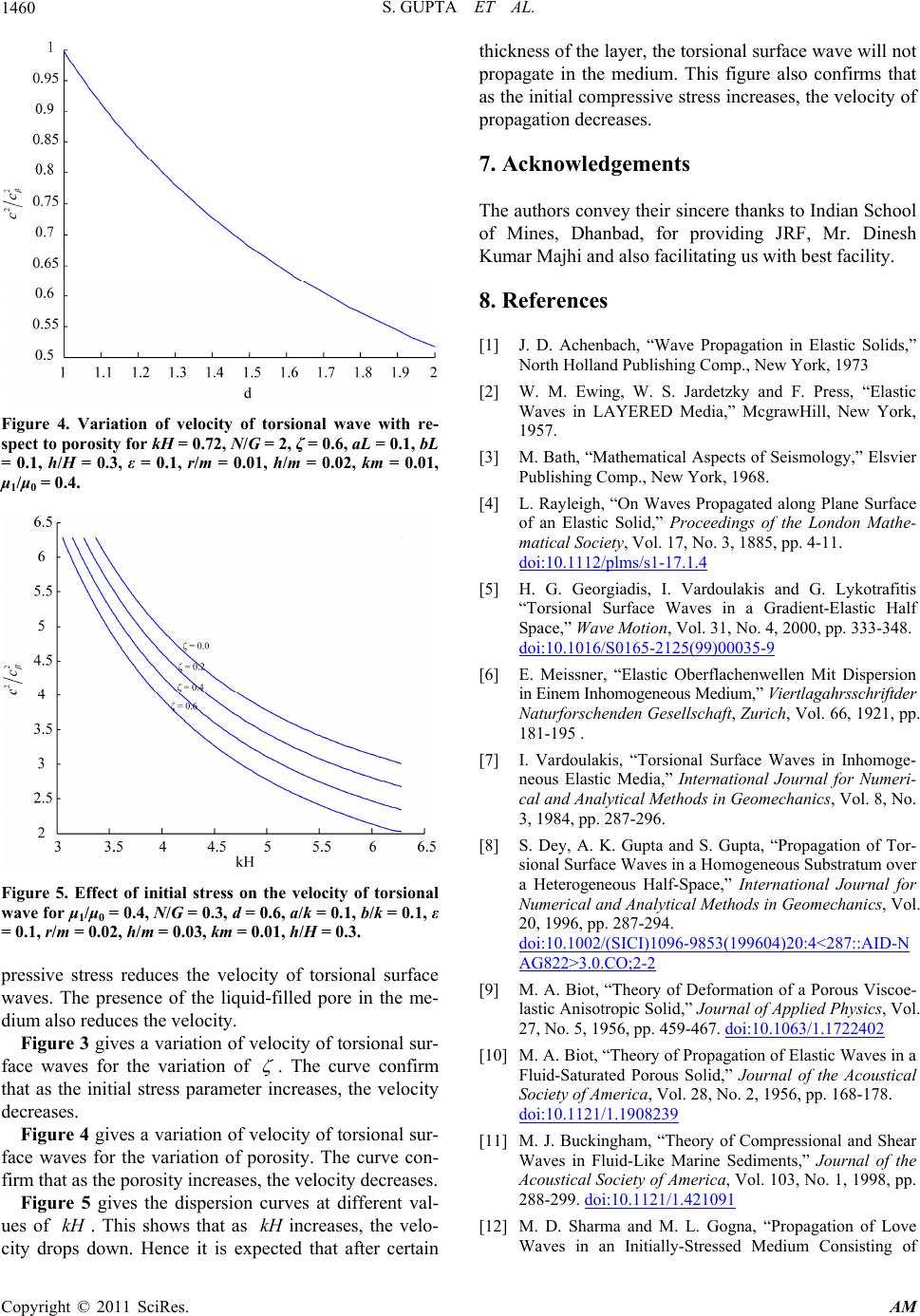 S. GUPTA ET AL. 1460 Figure 4. Variation of velocity of torsional wave with re- spect to porosity for kH = 0.72, N/G = 2, ζ = 0.6, aL = 0.1, bL = 0.1, h/H = 0.3, ε = 0.1, r/m = 0.01, h/m = 0.02, km = 0.01, μ1/μ0 = 0.4. Figure 5. Effect of initial stress on the velocity of torsional wave for μ1/μ0 = 0.4, N/G = 0.3, d = 0.6, a/k = 0.1, b/k = 0.1, ε = 0.1, r/m = 0.02, h/m = 0.03, km = 0.01, h/H = 0.3. pressive stress reduces the velocity of torsional surface waves. The presence of the liquid-filled pore in the me- dium also reduces the velocity. Figure 3 gives a variation of velocity of torsional sur- face waves for the variation of . The curve confirm that as the initial stress parameter increases, the velocity decreases. Figure 4 gives a variation of velocity of torsional sur- face waves for the variation of porosity. The curve con- firm that as the porosity increases, the velocity decreases. Figure 5 gives the dispersion curves at different val- ues of . This shows that as increases, the velo- city drops down. Hence it is expected that after certain thickness of the layer, the torsional surface wave will not propagate in the medium. This figure also confirms that as the initial compressive stress increases, the velocity of propagation decreases. kH kH 7. Acknowledgements The authors convey their sincere thanks to Indian School of Mines, Dhanbad, for providing JRF, Mr. Dinesh Kumar Majhi and also facilitating us with best facility. 8. References [1] J. D. Achenbach, “Wave Propagation in Elastic Solids,” North Holland Publishing Comp., New York, 1973 [2] W. M. Ewing, W. S. Jardetzky and F. Press, “Elastic Waves in LAYERED Media,” McgrawHill, New York, 1957. [3] M. Bath, “Mathematical Aspects of Seismology,” Elsvier Publishing Comp., New York, 1968. [4] L. Rayleigh, “On Waves Propagated along Plane Surface of an Elastic Solid,” Proceedings of the London Mathe- matical Society, Vol. 17, No. 3, 1885, pp. 4-11. doi:10.1112/plms/s1-17.1.4 [5] H. G. Georgiadis, I. Vardoulakis and G. Lykotrafitis “Torsional Surface Waves in a Gradient-Elastic Half Space,” Wave Motion, Vol. 31, No. 4, 2000, pp. 333-348. doi:10.1016/S0165-2125(99)00035-9 [6] E. Meissner, “Elastic Oberflachenwellen Mit Dispersion in Einem Inhomogeneous Medium,” Viertlagahrsschriftder Naturforschenden Gesellschaft, Zurich, Vol. 66, 1921, pp. 181-195 . [7] I. Vardoulakis, “Torsional Surface Waves in Inhomoge- neous Elastic Media,” International Journal for Numeri- cal and Analytical Methods in Geomechanics, Vol. 8, No. 3, 1984, pp. 287-296. [8] S. Dey, A. K. Gupta and S. Gupta, “Propagation of Tor- sional Surface Waves in a Homogeneous Substratum over a Heterogeneous Half-Space,” International Journal for Numerical and Analytical Methods in Geomechanics, Vol. 20, 1996, pp. 287-294. doi:10.1002/(SICI)1096-9853(199604)20:4<287::AID-N AG822>3.0.CO;2-2 [9] M. A. Biot, “Theory of Deformation of a Porous Viscoe- lastic Anisotropic Solid,” Journal of Applied Physics, Vol. 27, No. 5, 1956, pp. 459-467. doi:10.1063/1.1722402 [10] M. A. Biot, “Theory of Propagation of Elastic Waves in a Fluid-Saturated Porous Solid,” Journal of the Acoustical Society of America, Vol. 28, No. 2, 1956, pp. 168-178. doi:10.1121/1.1908239 [11] M. J. Buckingham, “Theory of Compressional and Shear Waves in Fluid-Like Marine Sediments,” Journal of the Acoustical Society of America, Vol. 103, No. 1, 1998, pp. 288-299. doi:10.1121/1.421091 [12] M. D. Sharma and M. L. Gogna, “Propagation of Love Waves in an Initially-Stressed Medium Consisting of Copyright © 2011 SciRes. AM  S. GUPTA ET AL. Copyright © 2011 SciRes. AM 1461 sandy Layer Lying over a Liquid Saturated Porous Solid Half-Space,” Journal of the Acoustical Society of Amer- ica, Vol. 89, No. 6, 1991, pp. 2584-2588. doi:10.1121/1.400697 [16] A. Chattopadhyay, S. Bose and M. Chakraborty, “Reflec- tion of Elastic Waves under Initial Stress at a Free Sur- face,” Journal of the Acoustical Society of America, Vol. 72, No. 1, 1982, pp. 255-263. doi:10.1121/1.387987 [13] M. D. Sharma, R. Kumar and M. L. Gogna, “Surface Wave Propagation in a Transversely Isotropic Elastic Layer Overlying a Liquid-Saturated Porous Solid Half- Space and Lying under a Uniform Layer of Liquid,” Pure and Applied Geophysics, Vol. 133, No. 3, 1990, pp. 523- 539. doi:10.1007/BF00878003 [17] B. K. Kar and V. K. Kalyani, “Reflection and Refraction of SH-Waves Due to the Presence of a Sandwiched Ini- tially Stressed Sandy Layer,” Geophysical research bul- letin, Vol. 25, No. 3, 1987, pp. 117-124. [18] S. Dey and S. K. Addy, “Reflection of Plane Waves un- der Initial Stress at a Free Surface,” International Journal of Non-Linear Mechanics, Vol. 12, No. 6, 1977, pp. 371- 381. doi:10.1016/0020-7462(77)90038-5 [14] M. D. Sharma, R. Kumar and M. L. Gogna, “Surface Wave Propagation in a Liquid-Saturated Porous Layer Over-Lying a Homogeneous Transversely Isotropic Half- Space and Lying under a Uniform Layer of Liquid,” In- ternational Journal of Solids and Structures, Vol. 27, 1991, pp. 1255-1267. doi:10.1016/0020-7683(91)90161-8 [19] M. A. Biot, “Mechanics of Incremental Deformation,” Wiley, New York, 1965. [20] W. H. Weiskopf, “Stresses in Soils under a Foundation,” Journal of the Franklin Institute, Vol. 239, No. 6, 1945, pp. 445-465. doi:10.1016/0016-0032(45)90189-X [15] M. A. Biot, “Influence of Initial Stress on Elastic Waves,” Journal of Applied Physics, Vol. 11, No. 8, 1940, pp. 522-530. doi:10.1063/1.1712807 Appendix : Stress in the liquid ,, r z : Components of the rotational vector in radial, circumferential and axial directions respectively. : Thickness of the layer : Rigidity of the medium : Circular frequency : Density of the medium k: Wave number , ab: Constants having dimensions that are inverse of length : Porosity of the layer c: Phase velocity of the torsional surface wave ij : Incremental stress components 1 c: Velocity of shear wave in an initially stress-free elas- tic medium. ij e: Components of strains R: Dimensionless quantity. ,, r uvw z z : Displacement components of the solid in the radial, circumferential and axial directions respectively. A, B and D1: Arbitrary constant ,, r UVW : Displacement components of the liquid in the radial, circumferential and axial directions respec- tively.
|