Journal of Modern Physics
Vol.07 No.12(2016), Article ID:70232,12 pages
10.4236/jmp.2016.712141
Quantum Statistical Theory of Superconductivity in MgB2
S. Fujita1, A. Suzuki2, Y. Takato3
1Department of Physics, University at Buffalo, State University of New York, Buffalo, NY, USA
2Department of Physics, Faculty of Science, Tokyo University of Science, Tokyo, Japan
3Okinawa Institute of Science and Technology Graduate University, Okinawa, Japan

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 30 June 2016; accepted 28 August 2016; published 31 August 2016
ABSTRACT
A quantum statistical theory of the superconductivity in MgB2 is developed regarding it as a member of the graphite intercalation compound. The superconducting temperature Tc for MgB2, C8K º KC8, CaC6, are 39 K, 0.6 K, 11.5 K, respectively. The differences arise from the lattice structures. In the plane perpendicular to the c-axis, B’s form a honeycomb lattice with the nearest neighbour distance 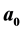 while Mg’s form a base-hexagonal lattice with the nearest neighbour distance
while Mg’s form a base-hexagonal lattice with the nearest neighbour distance 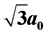 above and below the B-plane distanced by
above and below the B-plane distanced by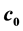 . The more compact B-plane becomes superconducting due to the electron-phonon attraction. Starting with the generalized Bardeen- Cooper-Schrieffer (BCS) Hamiltonian and solving the generalized Cooper equation, we obtain a linear dispersion relation
. The more compact B-plane becomes superconducting due to the electron-phonon attraction. Starting with the generalized Bardeen- Cooper-Schrieffer (BCS) Hamiltonian and solving the generalized Cooper equation, we obtain a linear dispersion relation  for moving Cooper pairs. The superconducting temperature Tc identified as the Bose-Einstein condensation temperature of the Cooper pairs in two dimensions is given by
for moving Cooper pairs. The superconducting temperature Tc identified as the Bose-Einstein condensation temperature of the Cooper pairs in two dimensions is given by , where
, where 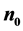 is the Cooper pair density,
is the Cooper pair density, 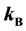 the Boltzmann constant. The lattices of KC8 and CaC6 are clearly specified.
the Boltzmann constant. The lattices of KC8 and CaC6 are clearly specified.
Keywords:
Crystal Structure, BCS Hamiltonian, Electron-Phonon Interaction, Cooper Pairs, Bose-Einstein Condensation, Superconductivity

1. Introduction
Nagamatsu et al. [1] reported in 2001 superconductivity at 39 K in magnesium diboride MgB2. MgB2 forms a lattice closely related to that of a graphite intercalation compound (GIC). It is similar to NaC2 composition- wise, but the lattice structures are distinct as shown below. The superconducting temperatures 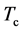 of MgB2 and NaC2 are 39 K and 5 K, respectively. This difference arises from the lattice structures. Canfield and Crabtree [2] wrote a comprehensive review in Physics Today (2003). From the isotope effect study [2] [3] they concluded that the B-plane contains a honeycomb lattice which becomes superconducting at 0 K, while the Mg-plane is base-hexagonal and is metallic. Their conjectured lattice structure of MgB2 is shown in Ref. 2, Figure 3. The superconducting state occurs at 0 K, where the entropy of an electron-phonon system vanishes. The third law of the thermodynamics applies. The crystal must be specified with the location of all atoms. Ref. 2, Figure 3 contains only the B-lattice and Mg-lattice with unspecifyed lattice constants. We must specify lattice systems with basic lattice units with the lattice constants. A currently presumed lattice for MgB2 [4] is a fully intercalated graphite compound similar to that in NaC2. We propose a different lattice. The two lattices have the same first neighbour configurations but different second nearest neighbours. Our proposed lattice has a lower Coulomb energy and should be realized in practice.
of MgB2 and NaC2 are 39 K and 5 K, respectively. This difference arises from the lattice structures. Canfield and Crabtree [2] wrote a comprehensive review in Physics Today (2003). From the isotope effect study [2] [3] they concluded that the B-plane contains a honeycomb lattice which becomes superconducting at 0 K, while the Mg-plane is base-hexagonal and is metallic. Their conjectured lattice structure of MgB2 is shown in Ref. 2, Figure 3. The superconducting state occurs at 0 K, where the entropy of an electron-phonon system vanishes. The third law of the thermodynamics applies. The crystal must be specified with the location of all atoms. Ref. 2, Figure 3 contains only the B-lattice and Mg-lattice with unspecifyed lattice constants. We must specify lattice systems with basic lattice units with the lattice constants. A currently presumed lattice for MgB2 [4] is a fully intercalated graphite compound similar to that in NaC2. We propose a different lattice. The two lattices have the same first neighbour configurations but different second nearest neighbours. Our proposed lattice has a lower Coulomb energy and should be realized in practice.
We shall develop a quantum statistical theory of the superconductivity in MgB2, starting with a generalized Bardeen-Cooper-Schrieffer (BCS) Hamiltonian [5] and calculateing everything using the standard quantum statistical methods. Canfield-Crabtree’s and our lattices have nearly the same energies if the first neighbour configurations are examined. The second neighbour confi- gurations are different. Each B+ in our lattice is surrounded by six Mg+’s while each B+ in Canfield-Crabtree’s lattice is surrounded by three Mg+. Hence our lattice is more stable. In the course of the development, we clearly specify the lattices of C8K º KC8 and CaC6.
2. Electron Dynamics
Following Ashcroft and Mermin (AM) [6] , we assume that “electrons” and “holes” in solids run as wave packets (not point-particles). We adopt the semiclassical model of electron dynamics in solids [6] . It is necessary to introduce a k-vector:
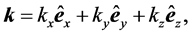 (1)
(1)
where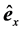 ,
, 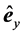 ,
, 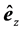 are the orthonormal vectors, since the k-vectors are involved in the semiclassical equation of motion:
are the orthonormal vectors, since the k-vectors are involved in the semiclassical equation of motion:
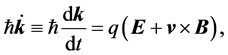 (2)
(2)
where 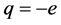 is the electron charge, and
is the electron charge, and  and
and 

is the electron velocity, where 
If the electron is in a continuous energy range (energy band), then it will be accelerated by the electric force



where n is the electron density and 
We consider a graphene which forms a 2D honeycomb lattice. The Wigner-Seitz (WS) unit cell, a rhombus, contains two C’s. We showed in our earlier work [7] [8] that graphene has “electrons” and “holes” based on the rectangular unit cell. We briefly review our calculations below. We assume that the “electron” (“hole”) wave packet has the charge 






Hence, “electrons” are the majority carriers in graphene. The thermally activated electron densities are given by

where 
3. Lattice Structures in C8K = KC8, CaC6 and MgB2
Graphite is composed of graphene layers stacked in the manner ABAB∙∙∙ along the c-axis. We may choose an orthogonal unit cell shown in Figure 1.
The carbons (circles) in the A (B) planes are shown in dark (light) gray circles.
The unit cell contains 16 C’s. The two rectangles (white solid lines) are stacked vertically with the interlayer separation, 


The unit cell has three side-lengths:

Clearly, the system is periodic along the orthogonal directions with the three periods 




The negatively charged “electron” (with the charge


We now consider GIC. Let us first take C8K. The K+ ions should enter as interstitials and occupy the sites away from the positive ions C+. We see in Figure 1 that the center of the unit cell is empty. Each K+ should occupy the midpoint between two graphene layers. The 3D unit cell contains 16 C’s and 2 K’s. Alkali metal GIC, including C8Li, C8Rb, should form similar lattices. Next we condier C6Ca. Carbons (C) in graphite form a honeycomb lattice in the A plane as shown in Figure 1. There are eight (8) C’s and four (4) hexagon centers, (two full circles, two half-circles and four quarter-circles). If we fill the hexagon centers with C’s, then we obtain twelve (12) C’s in the 2D unit cell. Similarly the configuration of the B-plane and that one below is prescribed. There are 2 × 12 C’s in the planes A and B, and 2 × 2 Ca’s between the planes for C6Ca. The composition ratio 6:1 is correct. After the C-filling, the C-plane becomes primitive (base)-hexagonal and has a 60˚ rotation symmetry. The primitive unit cell contains six (6) C’s. Two Ca’s are likely to occupy below the centers of the primitive cells located at the two-light gray circles in Figure 1. A real 3D C6Ca is obtained by stacking the C6Ca sheets in the manner ABAB… We note that the structure of C6Ca is significantly more compact than that of C8K. C6Yb should have a similar lattice structure. GIC C4Na (C3K, C2Na) should have the same 12 C-sheets and 3 Na (4 K, 6 Na) intersheets.
Figure 1. An orthogonal unit cell (white solid lines) viewed from the top for graphite. The carbons (circles) in the A (B) planes are shown in dark (light) gray circles.
Consider now MgB2. It is only natural to start with the B-plane since this plane becomes superconducting at 0 K. Let us look at the top sheet in Figure 1. Within the white rectangle, there are eight (8) balls and four (4) vacant hexagons. If B’s occupy the ball sites and Mg’s occupy the hexagon-centers sites in the neighbour sheet above (or below), then we obtain the most likely lattice. The composition ratio 2:1 is correct. The B-plane contains a honeycomb lattice with a nearest neighbour distance 

Our lattice and Canfield-Crabtree’s are different in the second nearest neighbour configuration. Each B+ in our lattice is surrounded by six Mg+ while each B+ in Canfield-Crabtree’s lattice is surrounded by three Mg+. Hence our lattice is more stable. The B-plane contains a honeycomb lattice of B's for both. Our Mg-plane contains a base-hexagonal lattice of the nearest neighbour distance



4. The Hamiltonian
The countability and statistics of the fluxons (magnetic flux quanta) are the fundamental particle properties. We postulate that the fluxon is a half-spin fermion with zero mass and zero charge.
We assume that the magnetic field 

with the states




A longitudinal phonon, acoustic or optical, generates a charge density wave, which affects the electron (fluxon) motion through the charge displacement (current). Let us first consider the case of superconductivity. The phonon exchange between two electrons shown in Figure 2 generates a transition in the electron states with the effective interaction

where 


An electric current 






Figure 2. Electron’s (phonon’s) motions are represented by solid (dotted) lines, and the time is measured upwards. Two phonon exchange processes (a) and (b) generate the momenta change from the initial pair state 

where 







interaction with the energy denominators 

(11). The interaction is attractive (negative) and most effective when the states before and after the exchange have the same energy 
BCS [5] assumed the existence of Cooper pairs [12] in a superconductor, and wrote down a Hamiltonian containing the “electron” and “hole” kinetic energies and the pairing interaction Hamiltonian with the phonon variables eliminated. We start with a BCS-like Hamiltonian 

where 




The fluxon number operator 



The phonon exchange can create electron-fluxon composites, bosonic or fermionic, depending on the number of fluxons. The CM of any composite moves as a fermion (boson) if it contains an odd (even) numbers of elementary fermions. The electron (hole)-type c-particles carry negative (positive) charge. Electron (hole)-type Cooper-pair-like c-bosons are generated by the phonon-exchange attraction from a pair of electron (hole)-type c-fermions. The pair operators B are defined by

The prime on the summation in Equation (12) means the restriction:

Figure 3. Fluxon’s motion is represented by dot-dashed lines. The phonon exchange processes (a) and (b) generate the momenta change from the initial (electron, fluxon) state 

The pairing interaction terms in Equation (12) conserve the charge. The term

pairing strength, generates a transition in electron-type c-particle states. Similarly, the exchange of a phonon
generates a transition between hole-type c-particle states, represented by
can also pair-create (pair-annihilate) electron (hole)-type c-boson pairs, and the effects of these processes are
represented by 

The Cooper pair, also called the pairon, is formed from two “electrons” (or “holes”). The pairons move as bosons, which are shown in Appendix. Likewise the c-bosons may be formed by the phonon-exchange attraction from two like-charge c-fermions. If the density of the c-bosons is high enough, then the c-bosons will be Bose- condensed and exhibit a superconductivity.
The pairing interaction terms in Equation (12) are formally identical with those in the generalized BCS Hamiltonian [13] . Only we deal here with c-fermions instead of conduction electrons.
The c-bosons, having the linear dispersion relation, can move in all directions in the plane with the constant speed 


is given by

where 



where 

Hence we obtain the Hall resistivity as

For the integer QHE at



The supercurrent generated by equal numbers of ± c-bosons condensed monochromatically is neutral. This is reflected in our calculations in Equation (18). The supercondensate whose motion generates a supercurrent must be neutral. If it has a charge, it would then be accelerated indefinitely by any external electric field because the impurities and phonons cannot stop the supercurrent to grow. That is, the circuit containing a superconducting sample and a battery must be burnt out if the supercondensate is not neutral. In the calculation of 
Equation (21), we used the unaveraged drift velocity
averaged drift velocity cancels out 
We now extend our theory to include elementary fermions (electron, fluxon) as members of the c-fermion set. We can then treat the 2D superconductivity and the QHE in a unified manner. The c-boson containing one electron and one fluxon can be used to describe the principal QHE. Important pairings and effects are listed below: a) a pair of conduction electrons, superconductivity; b) c-fermions and fluxon, QHE; c) a pair of like- charge conduction electrons with two fluxons, QHE in graphene.
5. Superconductivity in C8K, CaC6 and MgB2
5.1. Preliminaries
5.1.1. “Electrons”, “Holes” and “Phonons”
The conduction electrons (“electrons”, “holes”) are excited based on the orthogonal unit cells. As mentioned earlier the “electrons” are the majority carriers in both graphene and graphite. The excitation energy for the “electrons” is smaller than for the “holes”. Phonons are generated based on the same orthogonal unit cells. Phonons are bosons, and hence can be generated with no activation energies. The phonons are distributed, following the Planck distribution function:

which is a sole function of the Kelvin temperature T.
As an example consider acoustic phonons with a linear dispersion relation:

where s is the sound speed. The phonon size may be characterized by the average wave length:

The average size of phonons at the room temperature is greater by a few orders of magnitudes than the electron size.
5.1.2. The Ground-State Cooper Pair (Pairon) Energy
Cooper solved the Cooper equation [Ref. 12, Equation (1)] with a negative interaction energy constant, 

where 






5.2. The Superconductivity in GIC and MgB2
The superconductivity occurs only in regular crystals. That is, it occurs only in crystals and not in liquids. C8K and graphene have a 120˚ rotation symmetry. C6Ca has a base-hexagonal (60˚ rotation) symmetry.
C8K has graphene sheets, and each sheet is likely to become superconducting below the critical temperatures
BCS [5] used the fermionic pair operator equation.

constructed a ground-state vector and obtained a ground-state energy of an electron-phonon system:

where N is the pairon number per spin and 


which is shown below.
The number operator in the k-q representation

has eigenvalues 0 or 1: [13]

The total number of a system of pairons, N, is represented by

where

represents the number of pairons having net momentum

and obtain, after simple calculations,

Although the occupation number 








The present author’s group [7] [8] [13] regards the superconductivity as a result of the BEC of the c-bosons. The free c-bosons moving in 2D with the linear dispersion relation 

where 


is greater several times than the BCS coherence length (pairon size):

where 

Thus 2D pairons do not overlap in space. Hence the 
Formula (25) is distinct from the BCS formula in the weak coupling limit:

Our Formula (35) obtained after identifying superconducting temperature as the BEC condensation temperature contains familiar quantities, the Fermi speed 

For illustration let us take GaAs/AlGaAs. We assume 




The neutral supercondensate is generated from the two ranges of energies of “electrons” and “holes”. Hence it is difficult to precisely determine the critical temperature from the theoretical consideration alone. The com- parison between theory and experiment may be carried out as follows. First we find the Fermi speed 



which indicates a close connection between the zero temperature gap 




6. Summary and Discussion
We have developed a theory regarding MgB2 as a member of GIC. We start with the lattice configuration with all ions locations specified, and find that each B-plane contains B’s forming a honeycomb lattice of the nearest neighbour distance (lattice constant) 

We obtain a linear dispersion relation 


Figure 4. The molar heat capacity C for 2D free massless bosons (after Ref. 14, Figure 6.3).
calculated without introducing the averaging. The superconducting energy gap 

Canfield and Crabtree have discussed two energy gaps, which is strange since there is one superconducting state at 0 K. We shall discuss this topic in a separate publication.
Cite this paper
S. Fujita,A. Suzuki,Y. Takato, (2016) Quantum Statistical Theory of Superconductivity in MgB2. Journal of Modern Physics,07,1546-1557. doi: 10.4236/jmp.2016.712141
References
- 1. Nagamatsu, J., Nakagawa, N., Muranaka, T., Zenitani, Y. and Akimitsu, J. (2001) Nature, 410, 63.
http://dx.doi.org/10.1038/35065039 - 2. Canfield, P.C. and Crabtree, G.W. (2003) Physics Today, 56, 34.
http://dx.doi.org/10.1063/1.1570770 - 3. Hinks, D.G., Claus, H. and Jorgenson, J.D. (2001) Nature, 411, 457.
http://dx.doi.org/10.1038/35078037
Kortus, J., et al. (2001) Physical Review Letters, 86, 4656.
http://dx.doi.org/10.1103/PhysRevLett.86.4656 - 4. Uchida, S. (2015) High Temperature Superconductivity. Springer, Japan, 9.
- 5. Bardeen, J., Cooper, L.N. and Schrieffer, J.R. (1957) Physical Review, 108, 1175.
http://dx.doi.org/10.1103/PhysRev.108.1175 - 6. Ashcroft, N.W. and Mermin, N.D. (1976) Solid State Physics. Saunders, Philadelphia, 6-7, 216-217, 228-229.
- 7. Fujita, S., Takato, Y. and Suzuki, A. (2011) Modern Physics Letters B, 25, 223.
http://dx.doi.org/10.1142/S021794911025675 - 8. Fujita, S. and Suzuki, A. (2010) Journal of Applied Physics, 107, 013711.
http://dx.doi.org/10.1063/1.3280035 - 9. Kang, N., Lu, L., Kong, W.J., Hu, J.S., Yi, W., Wang, Y.P., Zhang, D.L., Pan, Z.W. and Xie, S.S. (2003) Physical Review B, 67, 033404.
http://dx.doi.org/10.1103/PhysRevB.67.033404 - 10. Zhang, S.C., Hansson, T.H. and Kivelson, S. (1989) Physical Review Letters, 62, 82.
http://dx.doi.org/10.1103/PhysRevLett.62.82 - 11. Ehrenfest, P. and Oppenheimer, J.R. (1931) Physical Review, 37, 333.
http://dx.doi.org/10.1103/PhysRev.37.333
Bethe, H.A. and Jackiw, R.W. (1989) Intermediate Quantum Mechanics. 2nd Edition, Benjamin, New York, 23.
Fujita, S. and Morabito, D.L. (1998) Modern Physics Letters B, 12, 1061. - 12. Cooper, L.N. (1956) Physical Review, 104, 1189.
http://dx.doi.org/10.1103/PhysRev.104.1189 - 13. Fujita, S. and Godoy, S. (2001) Theory of High Temperature Superconductivity. Kluwer, Dordrecht, 54-58, 96-98, 107-109, 164-167, 230-231.
http://dx.doi.org/10.1007/0-306-48216-9 - 14. Fujita, S., Ito, K. and Godoy, S. (2009) Quantum Theory of Conducting Matter—Superconductivity. Springer, New York, 79-83.
http://dx.doi.org/10.1007/978-0-387-88211-6
Appendix: Linear Dispersion Relation and Bose-Einstein Condensation
We consider the case of a 2D superconductor. The phonon exchange attraction is in action for any pair of electrons near the Fermi surface. In general the bound pair has a net momentum, and hence, it moves. Such a pair is called a moving pairon. The energy 

which is Cooper’s equation in 2D, Equation (1) of his 1956 Physical Review paper [12] . The prime on the 



Equation (41) can be solved simply. We briefly review the calculations and results here. We assume that the energy 

Then,


where

is k-independent. Introducing Equation (43) in Equation (44), and dropping the common factor

We now assume a free-electron model in 2D. The Fermi surface is a circle of the radius (momentum)

where 


The prime on the k-integral in Equation (45) means the restriction:

We may choose the z-axis along 
The k-integral in Equation (45) can then be expressed by

where 

After performing the integration and taking the small-q and small-

Figure 5. The range of the interaction variables 

where

is the pairon ground state energy.
As expected, the zero-momentum pair has the lowest energy


Such a linear dispersion relation is valid for pairs moving in any dimensions (D). However the coefficients slightly depend on the dimension as follows:

where 

The velocity 


The velocity magnitude 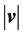
We consider a system of free bosons having a linear dispersion relation: 


where n is the 2D boson density. The 





where










