Open Journal of Civil Engineering
Vol.06 No.03(2016), Article ID:67887,7 pages
10.4236/ojce.2016.63042
Earth Pressure of Retaining Structure Induced by Subgrade under Rainfall
Yuwei Zhang, Junzhi Lin, Jun Zhao
College of River and Ocean Engineering, Chongqing Jiaotong University, Chongqing, China

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 31 May 2016; accepted 27 June 2016; published 30 June 2016
ABSTRACT
This article selects the retaining wall as the research object, introducing the rainfall infiltration model, considering the infiltration of rainwater into the groundwater recharge, analysizing the variation of earth pressure in the subgrade retaining wall. On this occasion, the back of retaining wall produces stable seepage water and compares with the non drainage water body. The results show that, with the infiltration of rainwater into the groundwater recharge, the greater the active earth pressure under the condition of rainfall appears, more quickly the active earth pressure of the retaining wall with the drainage body increases. The matrix suction of unsaturated soils, which is infiltrated into soil of subgrade, has a positive effect on the shear strength of the earth pressure.
Keywords:
Subgrade Engineering, Matrix Suction, Limit Equilibrium Method, Active Earth Pressure, Retaining Wall, Rainfall Infiltration

1. Introduction
Rainfall is one of the main causes of instability and failure of roadbed and other projects. After rainfall, surface runoff is formed on the road, and some of rainwater by the drainage system flows on both sides of the road, and part of rain water through the cracks of pavement structural the roadbed. On the one hand, it leads to the soil mass increases, and the original stress state in the soil of subgrade is changed, the shear strength of the soil is weakened, and the shear stress is increased; on the other hand, the effect of seepage in the soil can also promote this phenomenon. Retaining wall is one of the commonly used supporting structures in subgrade engineering. It is very necessary to analyze the strength of the back of the subgrade retaining wall to ensure the stability and durability of the roadbed. Earth pressure is one of the main loads. Drainage system is usually arranged in the design of retaining wall, in order to reduce the water content to a certain extent. When the roadbed water content is too large, poor drainage may lead to soil strength decreased and then lead to a series of disasters. Li Liang et al. [1] simplified Bishop method being applied to unsaturated soil, and the stability formula of subgrade under the condition of rainfall seepage is derived. Li Xia [2] analyzed the effect of rainfall seepage on the moisture content of subgrade. Zhao Minghua et al. [3] discussed the mechanism of the Instantaneous Event Model and Finite Duration Event Model rainfall model, and deduced the formula of the depth of the rain water infiltration Zhang Chang-guang, Zhao Junhai [4] [5] , etc. On the basis of the double stress state variable of unsaturated soil, considering the intermediate principal stress effect, the unified solution of shear strength and soil pressure of unsaturated soil is established. Wang Junjie et al. [6] analysed the earth pressure, under the action of load and seismic load, and considering the steady seepage of saturated soil. The existing theory of non-saturated soil and unsaturated soil pressure under the premise combined with the relevant research results of rainfall infiltration model. In this article, reaching and analyzing the earth pressure acting on the wall of the subgrade retaining wall, under the condition of rainfall, the infiltration of rainwater into the roadbed is a certain depth, which is influenced by the underground water level.
2. Research Question and Basic Assumptions
2.1. Description of Research Questions
Taking the retaining wall as the research object, based on the level of the embankment fill surface after the wall, in the condition of rainfall, infiltration into the wall after the rain water in the soil is different, so the value of the earth pressure on the retaining wall is changing, when arrangement drainage system on the back of wall. As shown in Figure 1(a), where H is the high of wall, h0 is the Initial height of groundwater level, 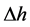 is Variation of groundwater level and
is Variation of groundwater level and 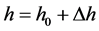 is water level line. After the rain into the permeability to fill soil water fails to reach the groundwater or make groundwater level rise, the magnitude of active earth pressure in water line h, above the water line for non-saturated soil, below the water line for saturated soil.
is water level line. After the rain into the permeability to fill soil water fails to reach the groundwater or make groundwater level rise, the magnitude of active earth pressure in water line h, above the water line for non-saturated soil, below the water line for saturated soil.
2.2. Basic Assumption
In practical engineering, the calculation of earth pressure on the supporting mechanism is very complicated. In order to simplify the calculation, it is necessary to make the specific problem to be idealized:
1) To meet the basic assumption of the Coulomb earth pressure theory;
2) Rigid retaining wall back is upright, and filling surface level. The soil is homogeneous and isotropic which material parameters such as friction angle, porosity and permeability coefficient are constant;
3) The retaining wall is located on the foundation with infinite horizontal extension and equipped with drainage system. Without considering other hydraulic diffusion, the groundwater flow through the drainage body to stabilize and comply with Darcy’s law, as shown in Figure 1(b) [7] .
3. Transient Water Distribution Model of Soil after Rainfall Infiltration
The rainfall infiltration process is a typical representative of the unsaturated fluid solid coupling. We first need to understand the influence of the seepage field and the water content of the roadbed after the infiltration of rain-

Figure 1. Rainfall analysis of simplified mode.
fall, considering the transient flow field distribution ,when analysis of soil pressure on retaining structure of unsaturated soil under rainfall condition. Many scholars at home and abroad have studied the rainfall infiltration in different aspects, such as IVERSON [7] , Leonardo Cascini [8] , Tung-Lin Tsai [9] . In this paper adopted the Zhao Minghua’s [3] academic achievements that the mechanism of the rain infiltration of the FDEM rainfall model based on the general equation of seepage, saturated unsaturated seepage formula of unsaturated soil subgrade is derived under the condition of rainfall:
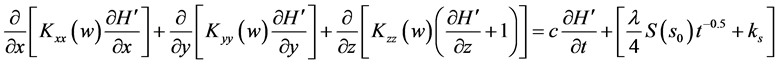 (1)
(1)
where 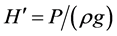 is atmospheric pressure head, P is atmospheric pressure, Kxx(w), Kyy(w), Kzz(w) is the permeability coefficient of the soil in 3 directions respectively, S(s0) is water uptake rate of soil, s0 is moisture content of soil before rainfall, ks is Saturated permeability coefficient, λ is porosity of soil, t is duration of rainfall.
is atmospheric pressure head, P is atmospheric pressure, Kxx(w), Kyy(w), Kzz(w) is the permeability coefficient of the soil in 3 directions respectively, S(s0) is water uptake rate of soil, s0 is moisture content of soil before rainfall, ks is Saturated permeability coefficient, λ is porosity of soil, t is duration of rainfall.
Under the condition of continuous rainfall model, starting from the surface, the rain infiltration depth of subgrade 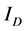 may be written as:
may be written as:
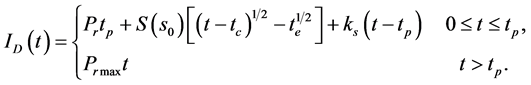 (2)
(2)
where Pr is seepage rate of soil, te is the time of rainfall rate is greater than that of the soil's infiltration rate Pr, tp is the time for the actual production of water and 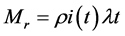 is the increment of the water content in the soil mass of the foundation wall after the rainfall,
is the increment of the water content in the soil mass of the foundation wall after the rainfall,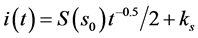 .
.
4. The Calculation of Active Earth Pressure on the Wall
4.1. Unsaturated Soil Shear Strength
Bishop based on the effective stress principle of unsaturated soils, which is a typical representative of the shear strength theory of unsaturated soils, the equation may be written as:
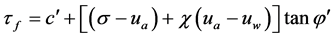
Definition: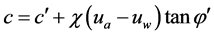 (4)
(4)
Obtain: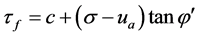 (5)
(5)
where 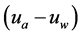 is matrix suction,
is matrix suction,  is normal net stress,
is normal net stress, 



where S is saturation, 

The type (6) into the form (4):

4.2. Calculation of Water Pressure in the Back of the Wall with Drainage Body
As shown in Figure 2, filling wall surface level, the initial water level is 

Figure 2. The groundwater steady flow field sketch.

where

where 




4.3. The Calculation of Active Earth Pressure
According to the Coulomb earth pressure theory,when the wall backfill soil reaches the active limit state will produce a theta angle with the horizontal plane of the fracture surface and through the wall to heel. As shown in
Figure 3(a), the plane OB and the rupture angle θ [5] [13] [14] . In order to simplify, the rupture angle

body to be subjected to force analysis. The calculation diagram as shown in Figure 3(b).
(1) The Underground water table of the sliding mass ABCD weight 




(2) Cohesive force C on the fracture surface of unsaturated soils, which is the above rainfall infiltration underground water level and the earth fill surface in the following sections of plane DB:

The type (7) into the Equation (14) to C:


Figure 3. Analysis of earth pressure chart of retaining wall back.
(3) Due to the drainage water of the wall, pore water pressure of saturated soil below ground water level is produced on the fracture surface. Total pore water pressure U along the fracture surface OD, and the formula (8) combined with type (6) for the integration, the result is [6] :

(4) As shown in Figure 3(b), the sliding soil wedge OAB is mainly affected by the soil pressure of the wall’s back, W is the wedge weight, along the failure surface shear T and effect on the plane DB unsaturated soil cohesive force, normal stress N perpendicular to the fracture surface and total pore water pressure U acting on OD. According to the condition of the static limit equilibrium of the rigid body:


where 



5. Example Analysis
This section takes a retaining wall as an example. Analysis the change of active earth pressure on the back of retaining wall, which influence of the water level of the underground water table under the condition of rainfall infiltration. See Table 1 for parameter values.
Rainfall intensity is 13.0 mm and the maximum infiltration depths respectively as 1.8 m, 2 m and 2.4 m when duration of rainfall 2 d 6 d and 10 d The Formula (3) was used to calculate the penetration depth of 1.8 m,


From the calculation results, the groundwater level is compensated when the depth of rainwater infiltration is greater than the distance from the surface of the water level, which make the water level rise and meanwhile the retaining wall of earth pressure are increased. Earth pressure should be much larger, when equipped with drainage body. The specific results are shown in Figure 4.
Figure 4. Effects of groundwater recharge on.
Table 1. Parameter selection table.
Table 2. Calculation result.
6. Concluding Remarks
In the design of retaining wall, in order to reduce the ground water level of back of wall, it needs to be set drainage body.
Sometimes, although the earth pressure of the retaining wall is relatively small when the water drainage body is not arranged, the softening and infiltration of groundwater to the soil after the embankment wall will aggravate the damage of the roadbed. Therefore, the drainage body must be set to carry out drainage treatment. From the analysis of this paper, with the infiltration of rainwater into the groundwater recharge, the greater the active earth pressure under the condition of rainfall, and the active earth pressure of the retaining wall with the drainage body increases more quickly.
Cite this paper
Yuwei Zhang,Junzhi Lin,Jun Zhao, (2016) Earth Pressure of Retaining Structure Induced by Subgrade under Rainfall. Open Journal of Civil Engineering,06,495-501. doi: 10.4236/ojce.2016.63042
References
- 1. Li, L., Liu, X.W. and Zhao, L.H. (2007) Factors Analysis of Subgrade Stability Due to Rainfall Infiltration. Journal of Railway Science and Engineering, 4, 19-23.
- 2. Li, X. (2010) Rainfall Seepage Effect of Subgrade Moisture Content Analysis. Journal of Highways & Automotive Applications, 4, 114-116.
- 3. Zhao, M.H., Liu, X.P., He, W. and Jiang, C. (2009) Unsaturated Soil Subgrade Rainfall Seepage Analysis. Journal of Highway and Transportation Research and Develop, 3, 49-53.
- 4. Zhang, C.G., Zhang, Q.H. and Zhao, J.H. (2010) Unified Solutions of Shear Strength and Earth Pressure for Unsaturated. Journal of Rock and Soil Mechanics, 6,1871-1876.
- 5. Zhao, J.H., Liang, W.B., Zhang, C.G., et al. (2013) Unified Solution of Coulomb’s Active Earth Pressure for Unsaturated Soils. Rock and Soil Mechanics, 34, 609-614.
- 6. Wang, J.J. and Chai, H.J. (2008) Active Earth Pressure Induced by Saturated Subgrade under Vehicle Load. Chinese Journal of Geotechnical Engineering, 30, 372-378.
- 7. Iverson, R.M. (2000) Landside Triggering by Rain Infiltration. Water Resources Research, 36, 1897-1910.
http://dx.doi.org/10.1029/2000WR900090 - 8. Leonardo, C., Sabatino, C. and Manuel, P. (2010) Modeling of Rainfall-Induced Shallow Landslides of the Flow-Type. Journal of Geotechnical and Geoenvironmental Engineering, 136, 85-98.
http://dx.doi.org/10.1061/(ASCE)GT.1943-5606.0000182 - 9. Tsai, T.-L. and Yang, J.-C. (2006) Modeling of Rainfall-Triggered Shallow Landslide. Environmental Geology, 50, 525-534.
http://dx.doi.org/10.1007/s00254-006-0229-x - 10. Vanapalli, S.K. and Fredlund, D.G. (2000) Comparison of Empirical Procedures to Predict the Shear Strength of Unsaturated Soils Using the Soil-Water Characteristic Curve. Advances in Unsaturated Geotechnics, 99, 195-209.
- 11. Van Genuchten, M.T. (1980) A Closed-Form Equation for Predicting the Hydraulic Conductivity of Unsaturated Soils. Soil Science Society of America Journal, 44, 892-898.
http://dx.doi.org/10.2136/sssaj1980.03615995004400050002x - 12. Barros, P.L.A. (2006) A Coulomb-Type Solution for Active Earth thrust with Seepage. Geotechnique, 56, 159-164.
http://dx.doi.org/10.1680/geot.2006.56.3.159 - 13. (2004) JTGD60—2004 General Code for Design of Highway Bridges and Culverts. The Ministry of Communications of China, Beijing. (In Chinese)
- 14. Harr, M.E. (1962) Groundwater and Seepage. McGraw-Hill, New York.







