Journal of Modern Physics
Vol.4 No.12(2013), Article ID:41484,7 pages DOI:10.4236/jmp.2013.412205
The Large Numbers in a Quantized Universe
52, 43 Dimitrova Street, Zagoryansky, Shchelkovo District, Moscow, Russia
Email: yanryazantsev@gmail.com
Copyright © 2013 Yan Ryazantsev. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received October 3, 2013; revised November 5, 2013; accepted November 29, 2013
Keywords: Large Numbers Hypothesis; Hubble Sphere; Eddington Number; Cosmological Constant
ABSTRACT
The article relates to a decades-old problem of the mysterious coincidence between various large numbers of the magnitude ranging from 1040 to 10120 which sometimes appears in cosmology and quantum physics. Using well known classical relations as well as the ideal Schwarzschild solution the exact relations of various large numbers, the fine structure constant ![]() and
and ![]() were found. The new largest number law is claimed. The hypothetical approximations of the Hubble parameter—68.7457(82) km/s/Mpc, Hubble radius—14.2330(17) Gly, and some others were proposed. The exact formulae supporting P. Dirac’s large number hypothesis and H. Weyl’s proposition were found. It is shown that all major physical constants with the length dimension (from the Compton wave length of universe through the Planck and atomic scale up to the Hubble sphere radius) could be derived from each other, and the table of the specific conversion rules has been developed. The model shows that the Eddington-Weinberg relation can be transformed to precise identity. It is shown that both Bekenstein universal entropy bound and Bekenstein-Hawking Black Hole entropy bound are proportional to the largest number doubled.
were found. The new largest number law is claimed. The hypothetical approximations of the Hubble parameter—68.7457(82) km/s/Mpc, Hubble radius—14.2330(17) Gly, and some others were proposed. The exact formulae supporting P. Dirac’s large number hypothesis and H. Weyl’s proposition were found. It is shown that all major physical constants with the length dimension (from the Compton wave length of universe through the Planck and atomic scale up to the Hubble sphere radius) could be derived from each other, and the table of the specific conversion rules has been developed. The model shows that the Eddington-Weinberg relation can be transformed to precise identity. It is shown that both Bekenstein universal entropy bound and Bekenstein-Hawking Black Hole entropy bound are proportional to the largest number doubled.
1. Introduction
The problem of Large Numbers dates back decades. The first problem statement and attempts at resolving it can be found in the studies by H. Weyl [1-3] and Sir A. Eddington [4,5], who drew attention to the incredibly large numbers of the dimensionless physical constants found in the cosmology, electrodynamics and quantum mechanics. The magnitude of these constants is so big 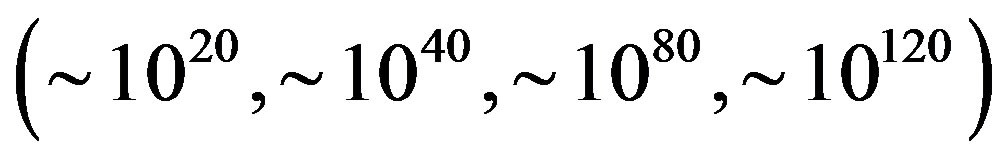 as compared with the conventional mathematical constants, like
as compared with the conventional mathematical constants, like 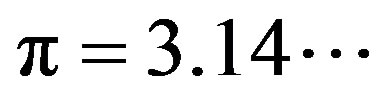 and Euler’s constant
and Euler’s constant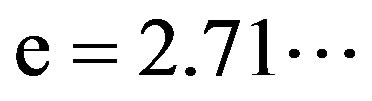 , that boggles imagination as P. Davies [6] noted.
, that boggles imagination as P. Davies [6] noted.
The first of the two most popular dimensionless large numbers is the classical ratio between the gravitational force and the electromagnetic force in any given distance, called by H. Weyl [2] “even more mysterious than the fine structure constant ![]() “:
“:
 (1)
(1)
were:
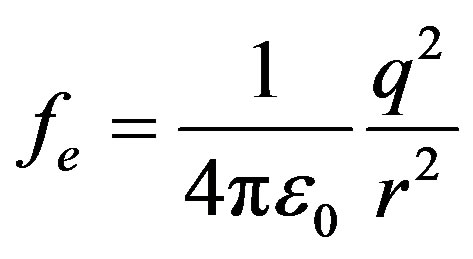 (2)
(2)
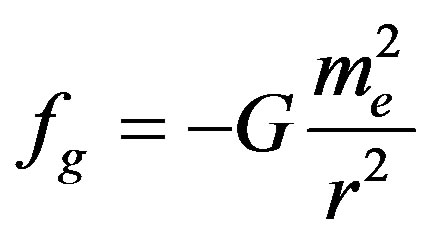 (3)
(3)
The second is the ratio of the universe radius to the classical electron radius:
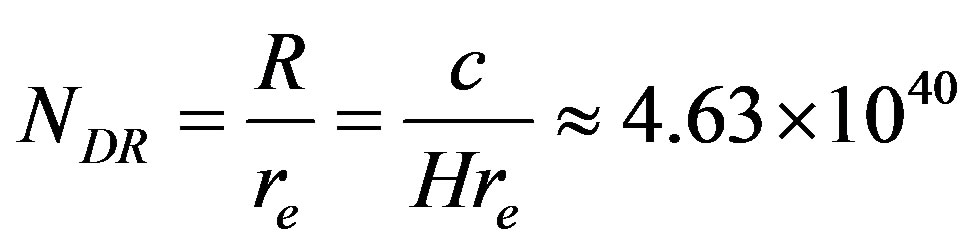 (4)
(4)
where  is Hubble’s constant,
is Hubble’s constant,![]() —classical electron radius,
—classical electron radius, —Hubble sphere radius or radius of event horizon,
—Hubble sphere radius or radius of event horizon, —gravitational electron radius,
—gravitational electron radius,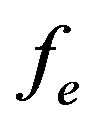 —electrostatic force between two electrons at a distance r,
—electrostatic force between two electrons at a distance r,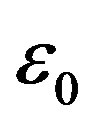 — permittivity of vacuum,
— permittivity of vacuum, —electron charge,
—electron charge,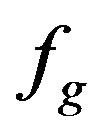 — gravitational force between two electrons at a distance r,
— gravitational force between two electrons at a distance r,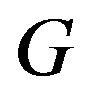 —Newton gravitational constant,
—Newton gravitational constant,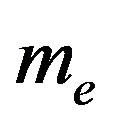 —electron mass.
—electron mass.
The proximity of magnitude of 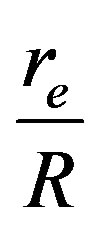 and
and 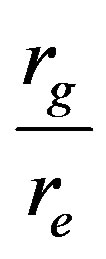 values led H. Weyl to the idea that the incredible weakness of gravitational interaction may be due to the ratio of the electron and the universe radiuses or to the total quantity of particles in the universe—the Eddington number [5].
values led H. Weyl to the idea that the incredible weakness of gravitational interaction may be due to the ratio of the electron and the universe radiuses or to the total quantity of particles in the universe—the Eddington number [5].
We refer everybody interested in the history of studying the problem of large numbers to the reviews by S. Ray, U. Mukhopadhyay, P. P. Ghosh [7] and K. A. Tomilin [8].
The hypothesis by P.A.M. Dirac is one of the best known hypotheses put forward to explain the problem of large numbers [9-11]. He supposed that the reason for appearance of great magnitudes of dimensionless values is their reliance on the equally large value, so-called “cosmological time”. This led P. Dirac to the hypothesis of dependence of the gravitational constant and the universe mass on time:
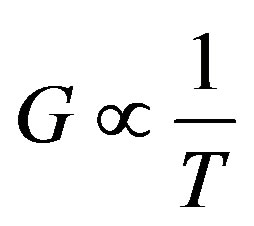 (5)
(5)
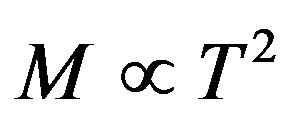 (6)
(6)
where 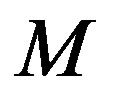 is the mass of universe,
is the mass of universe, ![]() is cosmological time.
is cosmological time.
The idea of time-varying constants was developed, in particular, by E. A. Milne [12]. To establish the ratios and laws between modern values of the fundamental constants, we suggest studying values of large numbers at the current point of time, without taking into account their time derivative. P. Dirac’s assumption that Newton’s gravitational constant and the mass of universe are not true constants but change over time gave rise to an array of scientific discussions, experimental and theoretical studies devoted to verification of the fundamental constants in subsequent decades. No reliable proofs of variability of the physical constants were found yet.
In this article, we will use the Hubble time, the parameter inverse to the Hubble constant, as approximation of the cosmological time:
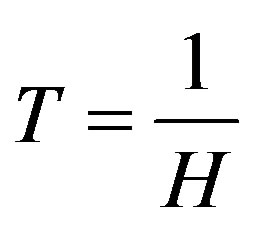 (7)
(7)
We referred to the simplest mathematics in narrating the article; however, the results we obtained provide quite good approximation to the most precise and generally accepted values of physical parameters, such as  and
and![]() , taking into account their uncertainties. In particular, CODATA 2010 [13] as well as the measurements of Mission Planck 2013 [14] were used.
, taking into account their uncertainties. In particular, CODATA 2010 [13] as well as the measurements of Mission Planck 2013 [14] were used.
2. Revealing the Large Numbers Ratios
To discover the correlation between large numbers in our epoch, let’s begin with the well known vacuum solution to the Einstein’s equations for spherically symmetric and static universe. According to Schwarzschild’s solution, the universe’s radius 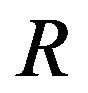 that coincides with the Black Hole radius with the mass of
that coincides with the Black Hole radius with the mass of 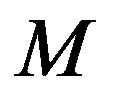 is determined by a well-known formula (Schwarzschild radius):
is determined by a well-known formula (Schwarzschild radius):
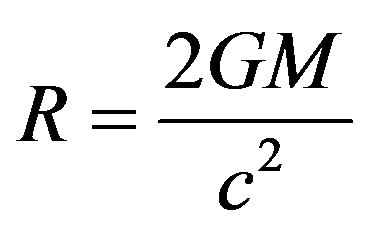 (8)
(8)
On the other hand, we know the formula for the electron gravitational radius that includes more or less precisely measured physical values:
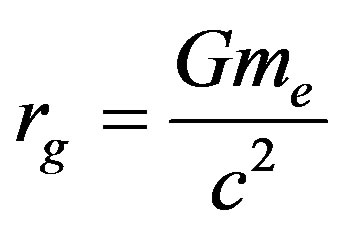 (9)
(9)
Therefore, the formula for the classical electron radius that uses 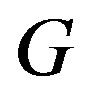 can be easily obtained from (8) and (9):
can be easily obtained from (8) and (9):
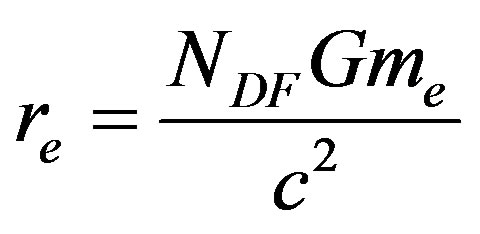 (10)
(10)
We will not discuss now if the value of the classical electron radius has any real physical significance. It is enough that it is one of the energy status representations of an electron, a particle with the minimum self-energy among all charged particles.
For transition to energy values, we will use a large mass number introduced by H.Weyl:
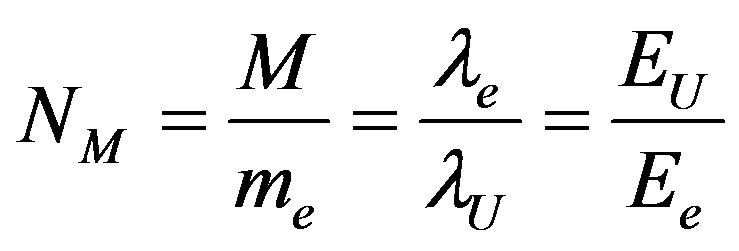 (11)
(11)
where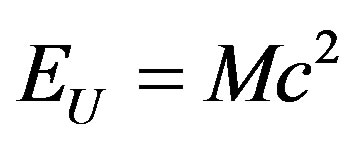 —the universe self-energy,
—the universe self-energy, 
—the electron self-energy,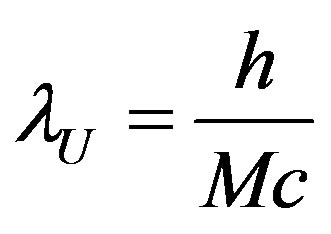 —Compton wave length of the universe,
—Compton wave length of the universe,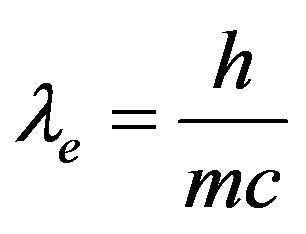 —Compton wave length of the electron.
—Compton wave length of the electron.
By dividing (8) by (10), one can get the following ratio:
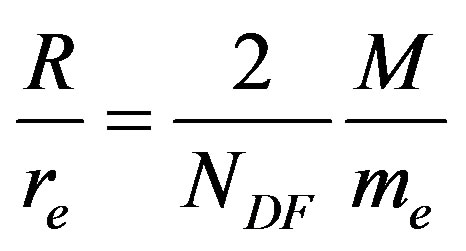 (12)
(12)
Thus, we obtain the precise correlation among the three large numbers out of (4), (11) and (12):
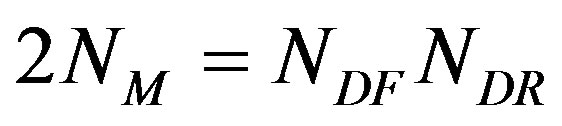 (13)
(13)
Besides the above large numbers, we will use a large energy number 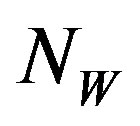 representing the ratio of the universe’s self-energy
representing the ratio of the universe’s self-energy 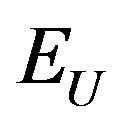 and “the minimum vacuum energy”
and “the minimum vacuum energy” 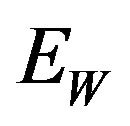 as proposed by J. Casado [15]:
as proposed by J. Casado [15]:
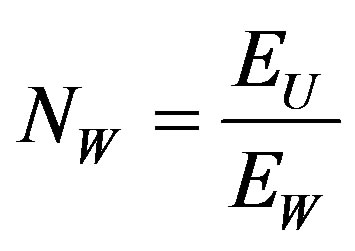 (14)
(14)
where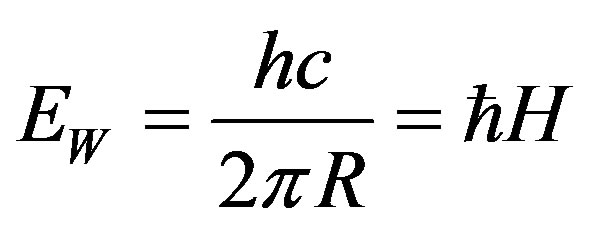 —quantum of energy with wave length
—quantum of energy with wave length ,
,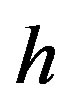 —Planck’s constant;
—Planck’s constant;
We will also need another large number equal to the cube of ![]() value:
value:
 (15)
(15)
Introduction of a new designation for a large number 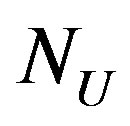 is quite justified, because, as we will see below, this number has a particular and substantial value. One of the simplest classical interpretations of this number: “the large number
is quite justified, because, as we will see below, this number has a particular and substantial value. One of the simplest classical interpretations of this number: “the large number 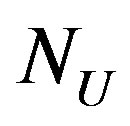 represents the total number of, say, ‘elementary clusters’ in the universe—the ratio between volume
represents the total number of, say, ‘elementary clusters’ in the universe—the ratio between volume 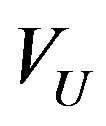 of ball-like universe and the region
of ball-like universe and the region 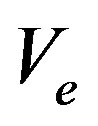 folded by sphere of radius
folded by sphere of radius![]() ”. Taking into account that this number has the greatest magnitude as compared with other large numbers
”. Taking into account that this number has the greatest magnitude as compared with other large numbers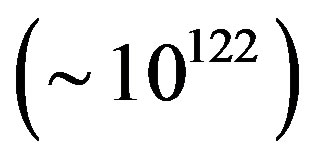 , we suggest calling it “the Largest number”.
, we suggest calling it “the Largest number”.
3. The Largest Number Law and Revealing of Dirac’s Proportionalities
Now let us use well known physical parameters with their uncertainties (see Table 1). The parameters listed in Table 1 enable us to calculate large numbers values (see Table 2). To obtain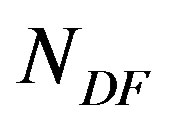 , we use (10):
, we use (10):
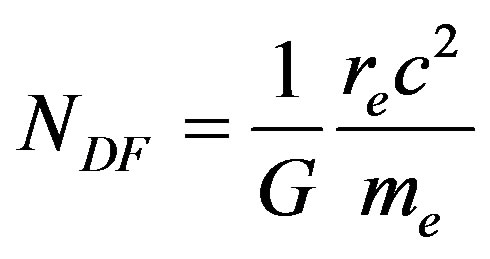 (16)
(16)
We should pay an attention to the very close values of 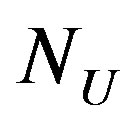 and
and 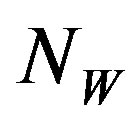 of the magnitude
of the magnitude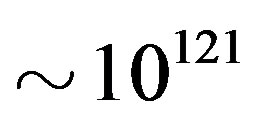 . The ratio between this two large numbers is
. The ratio between this two large numbers is
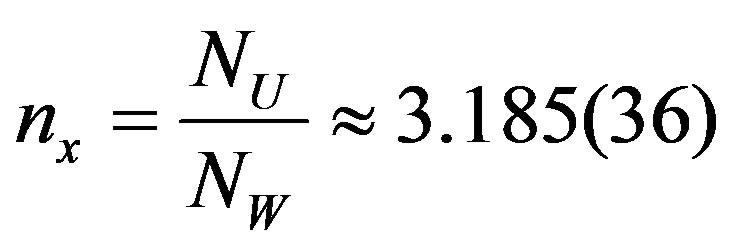 (17)
(17)
This is quite remarkable, taking into account the extremely large magnitudes of the numbers involved. Therefore, we can assume that this ratio is not just a coincidence but some specific physical law. In order to reveal the meaning of the ratio (17) one can rewrite it as follows:
 (18)
(18)
By simply grouping the cosmological parameters in
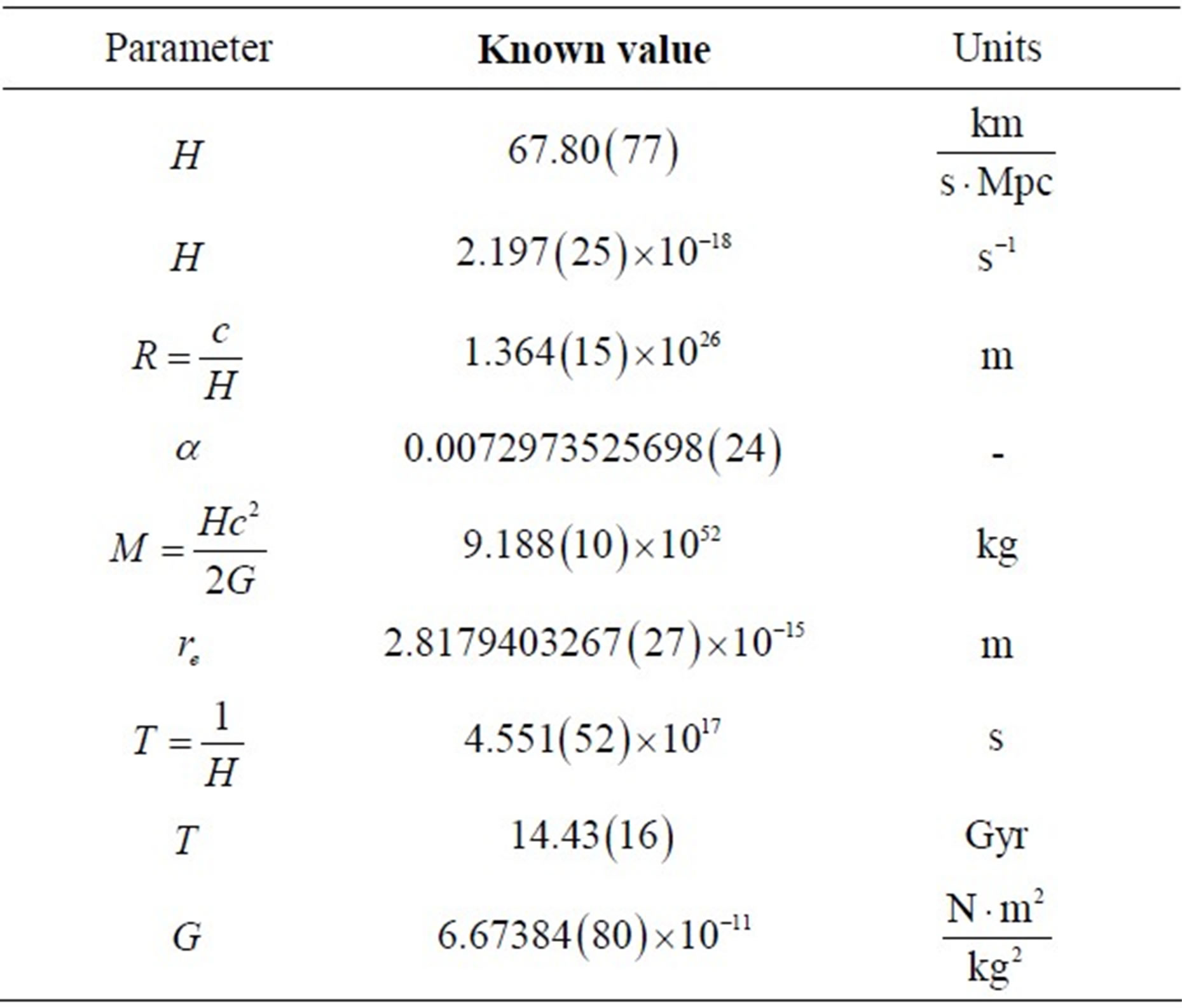
Table 1. Known physical parameters.
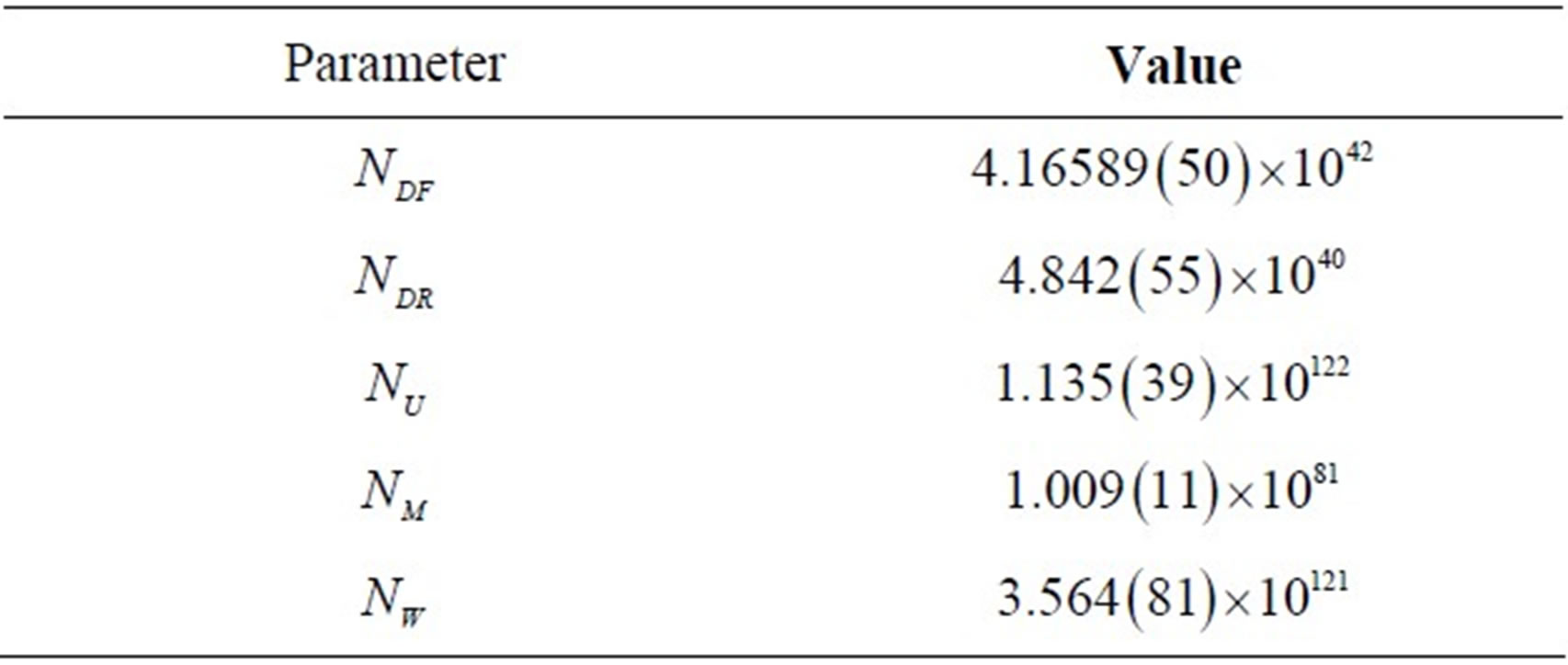
Table 2. Calculated Large numbers values.
the left-hand side, and the quantum ones in the righthand one the ratio (17) can be transformed into the following relation:
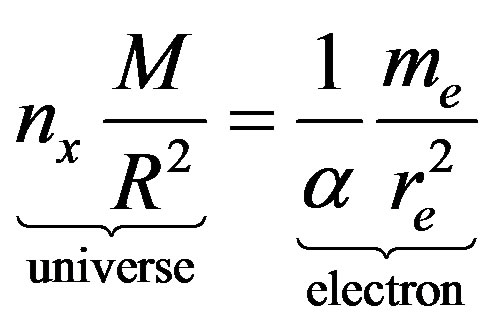 (19)
(19)
The question is—what kind of a phisical law the last equation represents?
In order to find out the answer we would like to propose quite a simple classical model. We can apply the classical approach because we are dealing with constant macroscopic physical parameters and those ratios. Let us consider a really large number of non-interacting quanta. All quanta are moving in all directions with a speed of light, i.e. there are photons. Obviously, the set must be confined inside the Black Hole with a radius 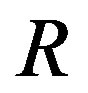 If the total energy of the whole quanta set is equal to
If the total energy of the whole quanta set is equal to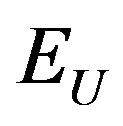 . We have to conclude that absolutely every photon must be reflected by the sphere’s bound in certain time. In other words the inner side of the Hubble sphere plays a role of an ideal diffusely reflecting surface. In a period of time
. We have to conclude that absolutely every photon must be reflected by the sphere’s bound in certain time. In other words the inner side of the Hubble sphere plays a role of an ideal diffusely reflecting surface. In a period of time 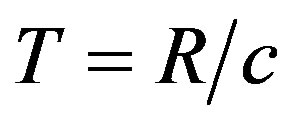 we will see that ultimately all quanta had experienced a reflection from the bound. It means that the inner side of the Hubble sphere looks like a Lambertian light emitter for an internal observer. Thus the constant radiant emittance from the inner side of our Black Hole can be expressed by the formula:
we will see that ultimately all quanta had experienced a reflection from the bound. It means that the inner side of the Hubble sphere looks like a Lambertian light emitter for an internal observer. Thus the constant radiant emittance from the inner side of our Black Hole can be expressed by the formula:
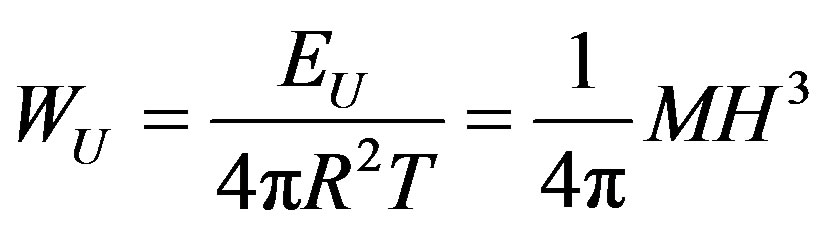 (20)
(20)
Now let us consider the observer-a spherical body in a vacuum with radius 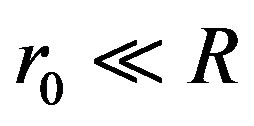 placed at the center of the Hubble sphere. The observer will find out a constant isotropic quanta flow that is falling to an every surface area
placed at the center of the Hubble sphere. The observer will find out a constant isotropic quanta flow that is falling to an every surface area  from a spatial hemisphere above the area.
from a spatial hemisphere above the area.
According to the Lambert’s cosine law a radiant energy flux  through the area
through the area  (i.e. irradiance) will be:
(i.e. irradiance) will be:
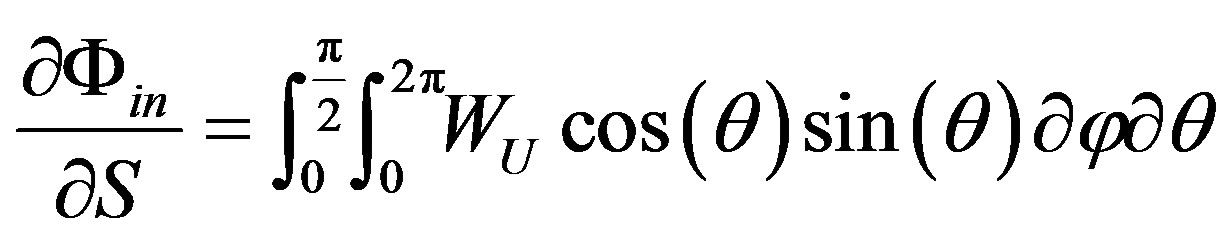 (21)
(21)
where  is the angle between the beam and a line normal to the surface area
is the angle between the beam and a line normal to the surface area .
.
The total radiant flux  related to the entire surface of our spherical observer:
related to the entire surface of our spherical observer:
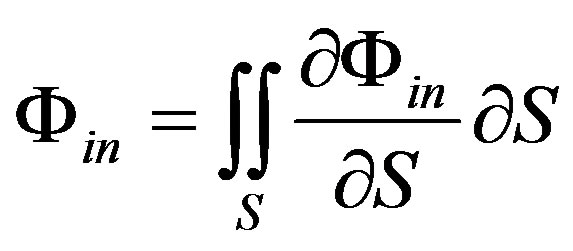 (22)
(22)
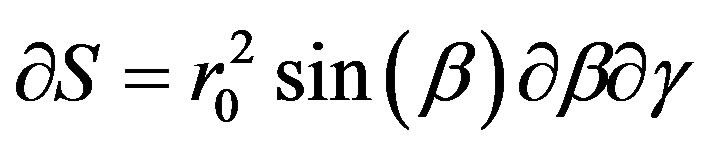 (23)
(23)
where 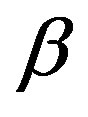 and
and 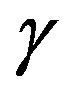 are spherical coordinates of the area
are spherical coordinates of the area 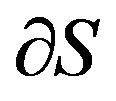 on observer’s surface. Thus:
on observer’s surface. Thus:
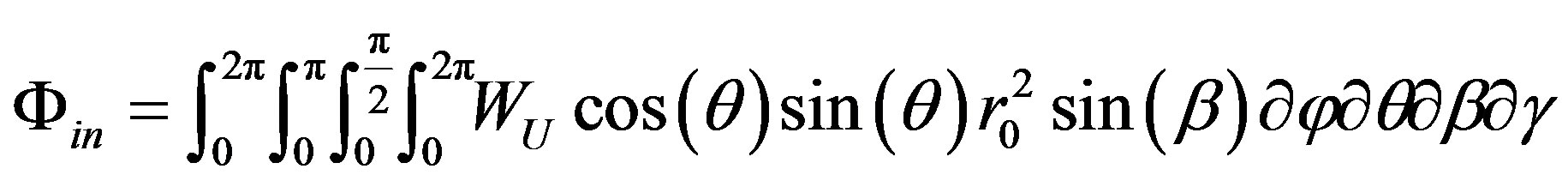 (24)
(24)
The last integral (24) can be simplified as follows:
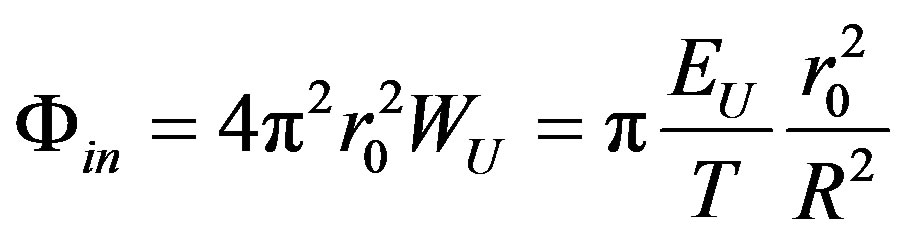 (25)
(25)
The total amount of energy 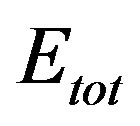 entered inward (or reflected by) the observer during the period of time
entered inward (or reflected by) the observer during the period of time ![]() is:
is:
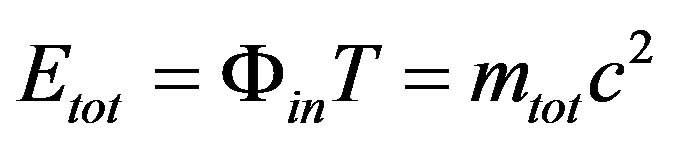 (26)
(26)
where 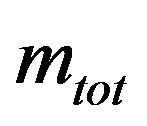 is a total mass which our observer should have at present time if he absorbs (or reflects) the incoming energy flux
is a total mass which our observer should have at present time if he absorbs (or reflects) the incoming energy flux 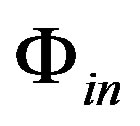 completely. Thus we can write down the following equation:
completely. Thus we can write down the following equation:
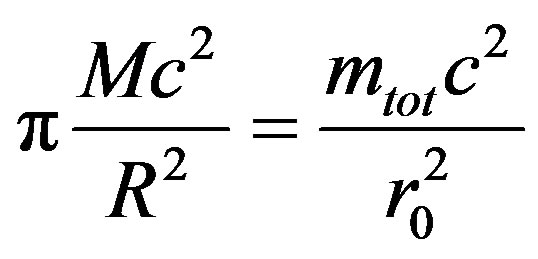 (27)
(27)
Now, making a comparison of the Equations (27) and (19) one would ultimately conclude that if the coefficient 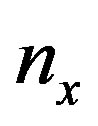 in the (19) equals exactly to
in the (19) equals exactly to ![]() then
then 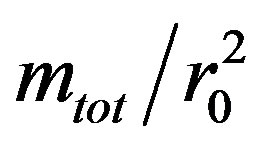 must be equal to
must be equal to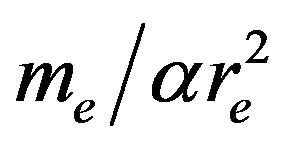 . Thus:
. Thus:
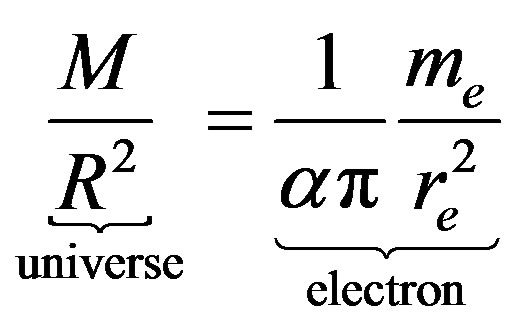 (28)
(28)
Using (4), (11) and (28), we immediately obtain a noteworthy ratio between Weyl-Eddington-Dirac large numbers :
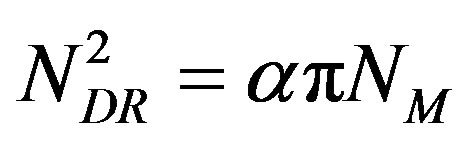 (29)
(29)
Hence, we can obtain the formula for the universe mass via the Hubble time:
 (30)
(30)
The last one represents the proportional relation between 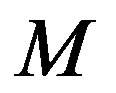 and
and 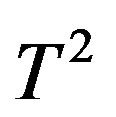 which was hypothesized by P. Dirac almost 80 years ago.
which was hypothesized by P. Dirac almost 80 years ago.
As we see from (29), both fundamental constants ![]() and
and ![]() are deeply involved in large number relations and thus we can assume that it is an evidence that cosmological and quantum parameters of our model of the universe are closely connected through geometry.
are deeply involved in large number relations and thus we can assume that it is an evidence that cosmological and quantum parameters of our model of the universe are closely connected through geometry.
The above Equations (13) and (29) readily yield the correlation of the best known large Weyl-Eddington-Dirac numbers, which include both the geometrical constant ![]() and the fine structure constant
and the fine structure constant![]() :
:
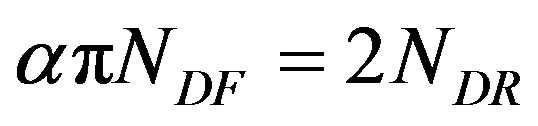 (31)
(31)
This correlation is very notable. It enables one to calculate the approximations for Hubble sphere radius and the Hubble parameter via the correlation of gravitational and electrostatic forces:
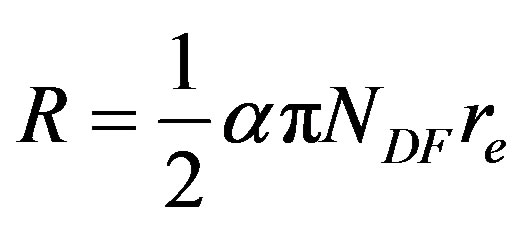 (32)
(32)
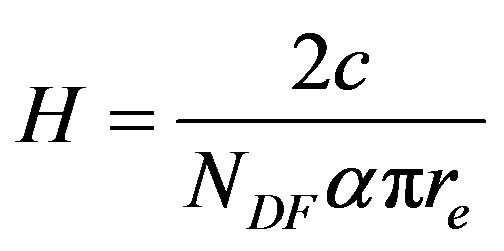 (33)
(33)
Now let us introduce the “big” angular momentum of the Hubble sphere measured along any given direction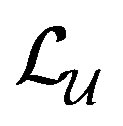 :
:
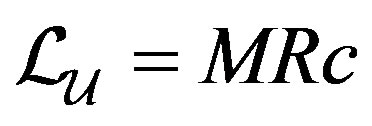 (34)
(34)
Thus, multiplying both sides of the Equation (28) by![]() , we would propose the exact Largest number law in the following form:
, we would propose the exact Largest number law in the following form:
 (35)
(35)
The total sum of 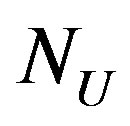 fundamental quanta of the angular momentum
fundamental quanta of the angular momentum 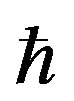 in universe equals exactly to the angular momentum of the Hubble sphere multiplied by
in universe equals exactly to the angular momentum of the Hubble sphere multiplied by![]() . This is a direct consequence of geometrical Lambert’s cosine law and rotational symmetry of space (conservation of angular momentum).
. This is a direct consequence of geometrical Lambert’s cosine law and rotational symmetry of space (conservation of angular momentum).
The following expression, as well as expressions (29) and (35), represent just another form of this law:
 (36)
(36)
With the help of large numbers ratios and Largest number law, one can get various representations of the Newton constant of gravitation 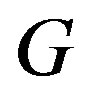 using initial expression:
using initial expression:
 (37)
(37)
The most elegant cases, in our opinion, are:
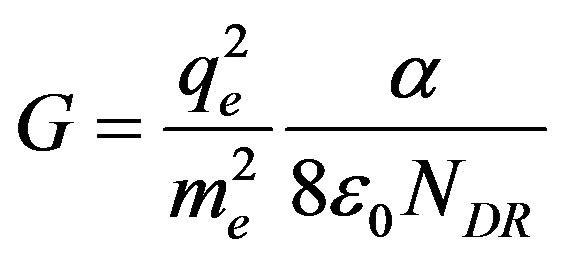 (38)
(38)
and:
 (39)
(39)
The last one represents the reverse proportional relation between 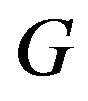 and
and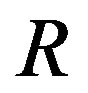 , which was hypothesized by H.Weyl and between
, which was hypothesized by H.Weyl and between 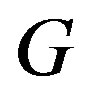 and
and ![]() which was hypothesized by P. Dirac.
which was hypothesized by P. Dirac.
By means of (39), one can get representation of the cosmological constant :
:
 (40)
(40)
where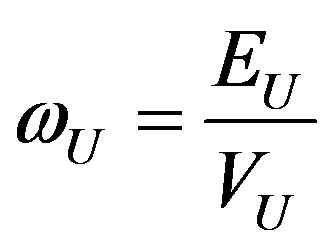 —energy density of the universe.
—energy density of the universe.
The last one represents the de Sitter space—a vacuum solution of Einstein’s equation with cosmological constant-for the 4-dimensional case. Hence, we can obtain the formula for the cosmological constant  via the fine structure constant
via the fine structure constant![]() , Weyl-Eddington-Dirac large number
, Weyl-Eddington-Dirac large number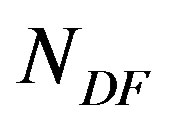 , classical radius of electron
, classical radius of electron ![]() and
and![]() :
:
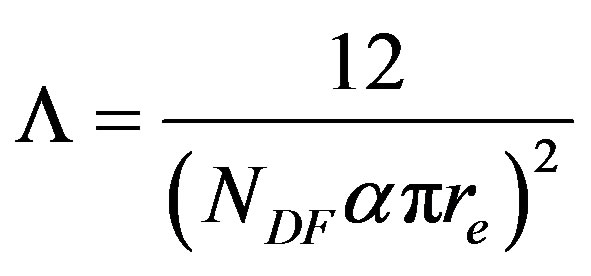 (41)
(41)
4. Calculations
The ratios between large numbers as described in Sections 2 to 3 enable us to calculate many cosmological parameters in the proposed model. The calculations has been carried out (by using the constants ) as follows:
) as follows: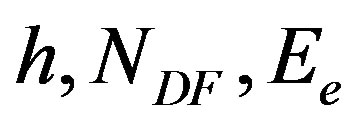 , then
, then![]() , then
, then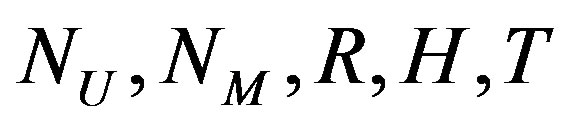 , then
, then 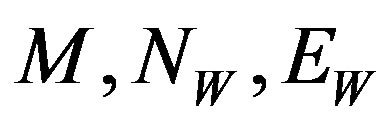 and then
and then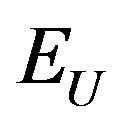 .
.
The results of calculations of the constants based on referential data and information from the most recent measurements are shown in Table 3.
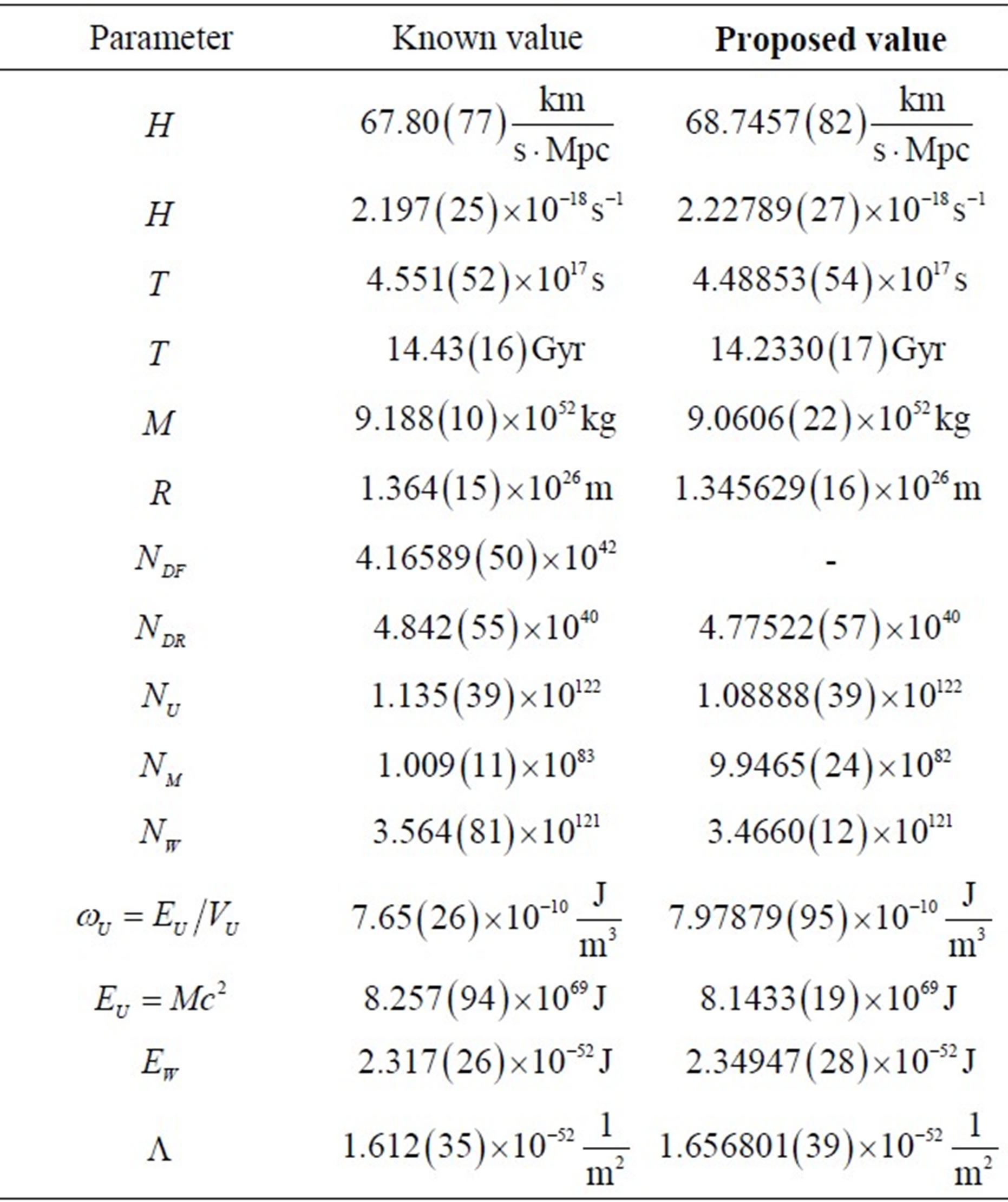
Table 3. Proposed values based on the the Largest number law.
The ratios of large numbers described in the previous sections enable to link the Hubble volume radius and other constants with the length dimensions, including such parameters as the electron gravitational radius and the Planck’s length. All such constants can be calculated one from the other, via the fine structure constant![]() , Weyl-Eddington-Dirac large numbers
, Weyl-Eddington-Dirac large numbers 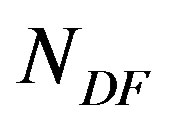 or
or ![]() and
and![]() . The Tables 4 and 5 show the mutual conversion ratios of the constants—from the Compton wave length of the universe to the Hubble sphere radius. To obtain the value of the top line parameter, one should multiply the initial parameter in the respective column by the formula in the cell at their crossing.
. The Tables 4 and 5 show the mutual conversion ratios of the constants—from the Compton wave length of the universe to the Hubble sphere radius. To obtain the value of the top line parameter, one should multiply the initial parameter in the respective column by the formula in the cell at their crossing.
In Table 5, one can find one more elegant expression-the ratio between largest and smallest distances in universe-radius of the Hubble sphere and the Compton wave length of the entire universe. It is proportional to the Largest number:
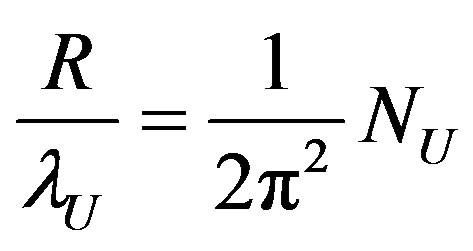 (42)
(42)
5. Examples of Applying the Large Number Ratios
The previous sections contain a rather simple derivation of the inter-dependence of all main large numbers. The Largest number law that links the Weyl-Eddington-Dirac numbers enable validation of whether the known hypothetic equations and inequations conform to the large numbers combination we proposed or not. Below are several examples.
Example 1. J. Teller proposed [16] an interesting ratio between Planck’s values, the fine structure constant and Hubble’s cosmological parameter:
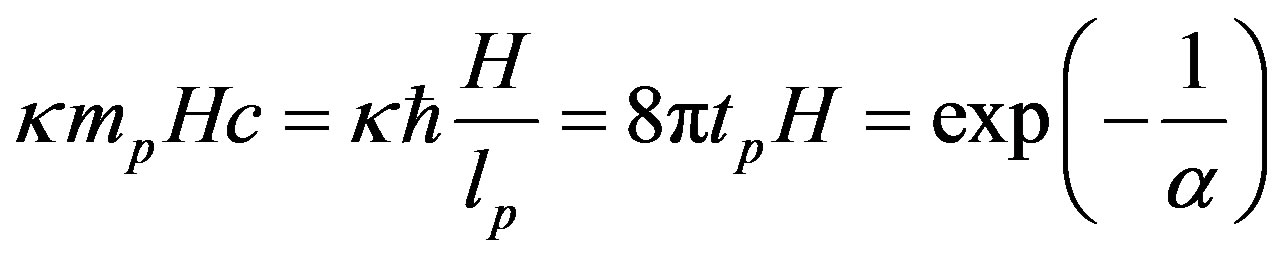 (43)
(43)
where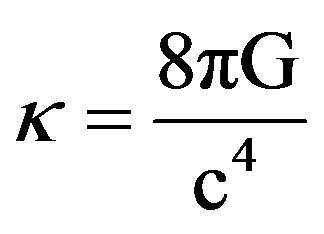 —Einstein constant,
—Einstein constant,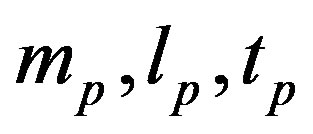 —Planck units.
—Planck units.
Having very large magnitude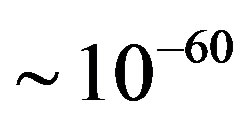 , the left and right parts of the formula give us values which differ from each other by only 1.5%. It is really remarkable but it is about 70 times more uncertain than other values calculated by us earlier:
, the left and right parts of the formula give us values which differ from each other by only 1.5%. It is really remarkable but it is about 70 times more uncertain than other values calculated by us earlier:
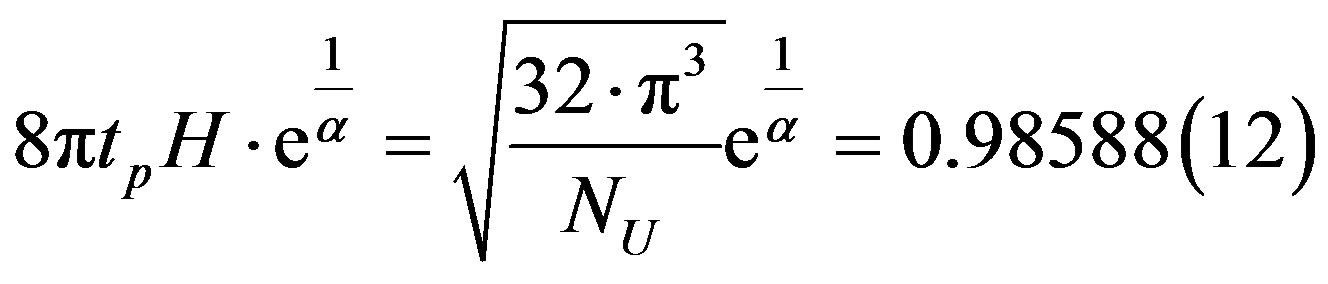 (44)
(44)
Teller’s formula cannot be recognized exact and expressing a fundamental physical law as it does not provide any strict derivation yet. A. Eddington’s contemplative assumptions on the quantity of particles in the universe, which is supposedly equal to precisely ,
,
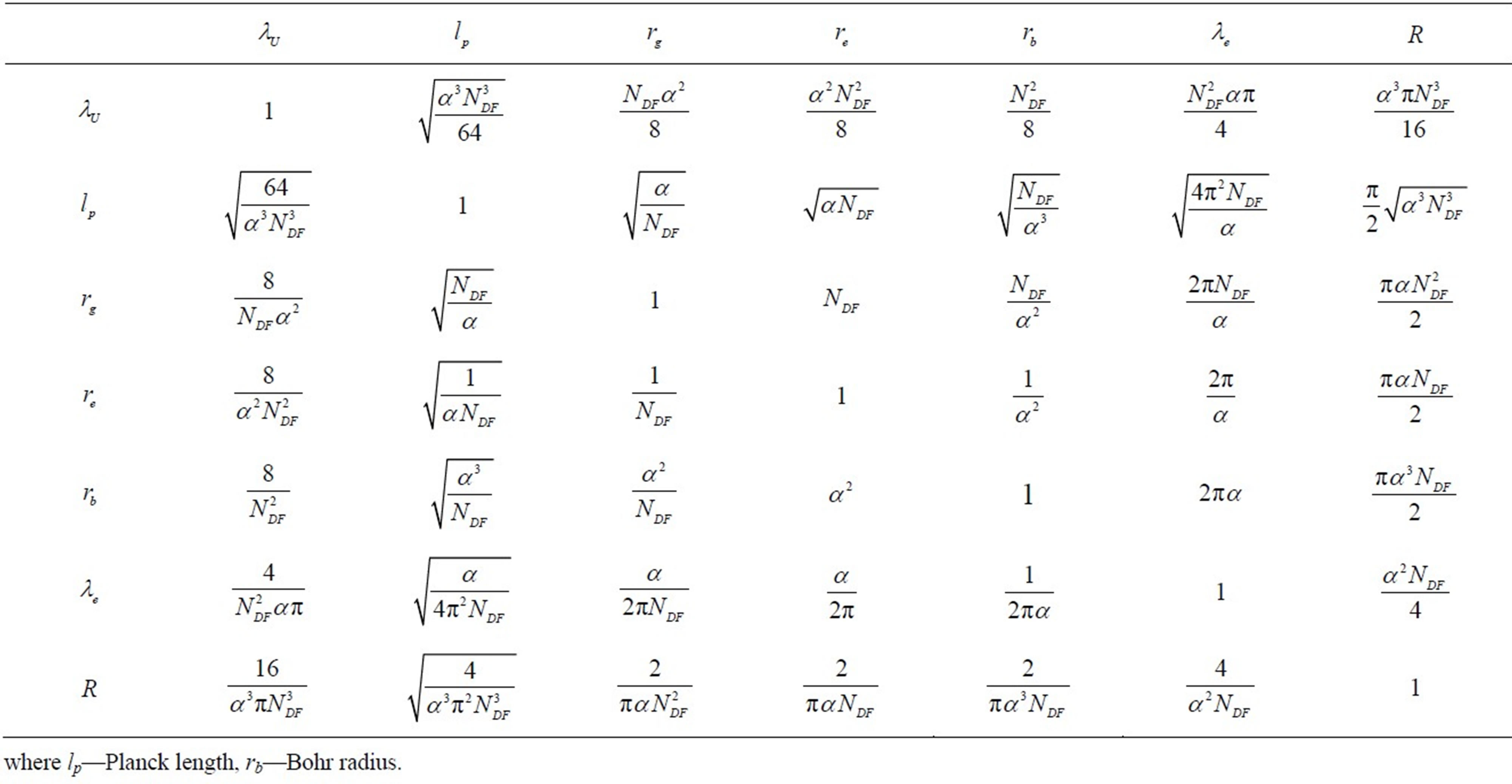
Table 4. Calculating parameters with a length dimension via![]() .
.
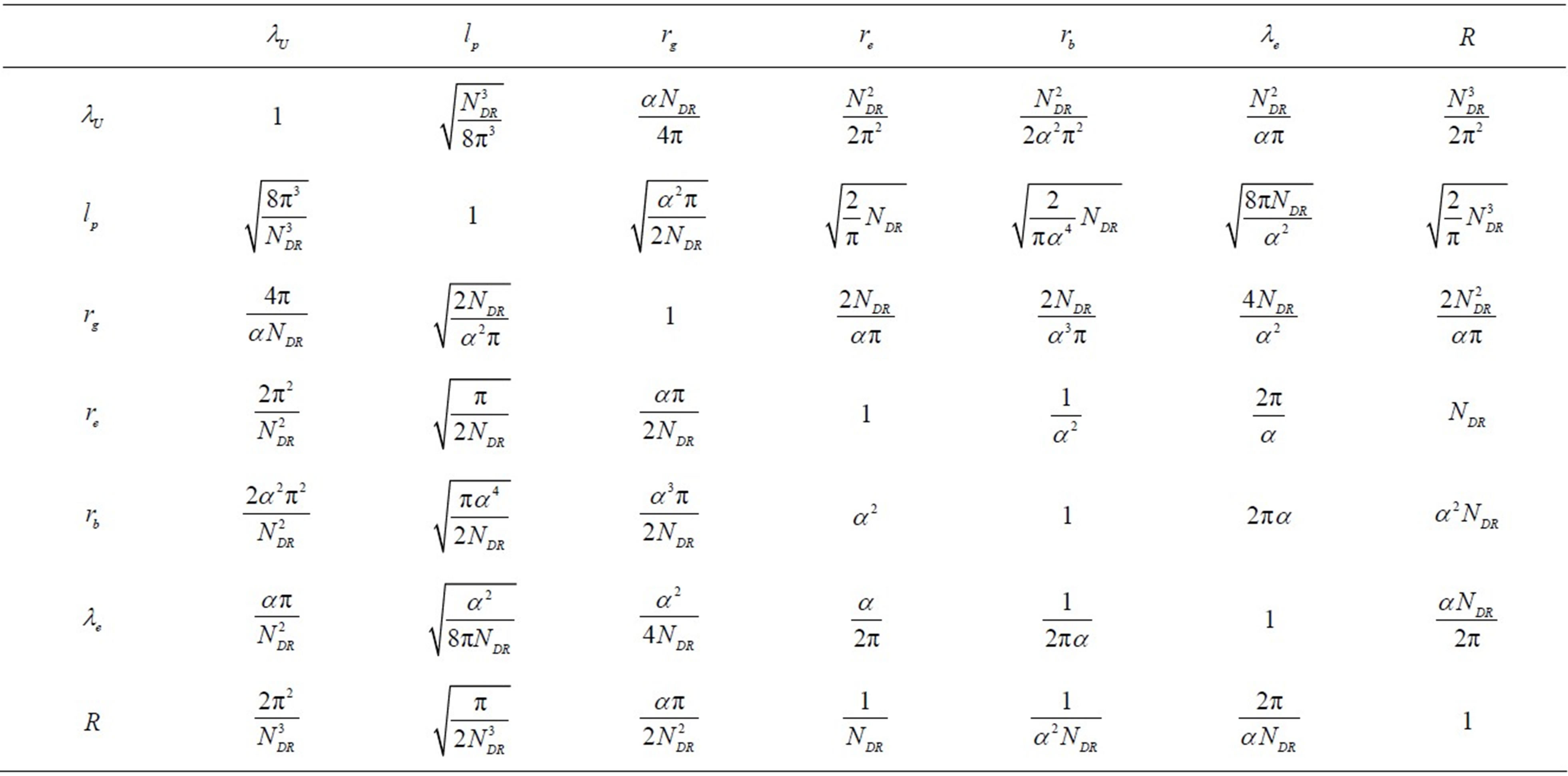
Table 5. Calculating parameters with a length dimension via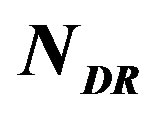 .
.
are even further from the reality.
Example 2. There is known Eddington-Weinberg approximate relation [17]:
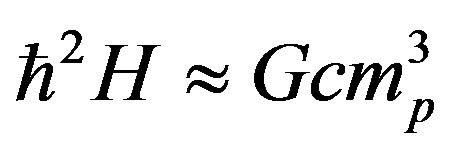 (45)
(45)
where —proton mass (CODATA 2010).
—proton mass (CODATA 2010).
Using the approximation of  calculated above (Table 3) one can get the ratio, which is quite far from expected
calculated above (Table 3) one can get the ratio, which is quite far from expected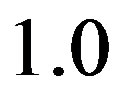 :
:
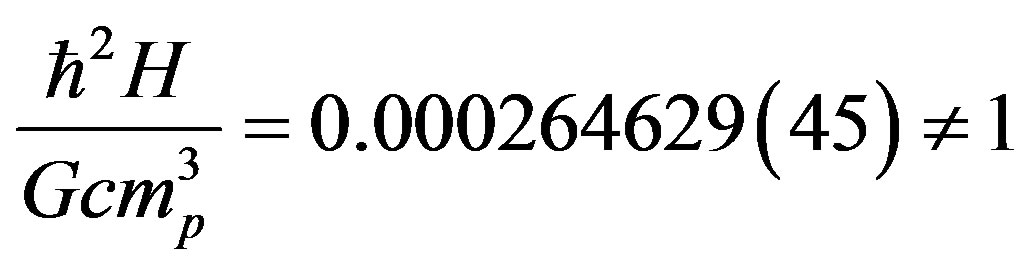 (46)
(46)
Thus we can conclude that Eddington-Weinberg approximate identity is not confirmed in our model. But, we should say that Eddington-Weinberg formula does have physical meaning in a nutshell. One can get the exact identity by replacing 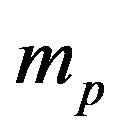 by
by 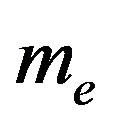 and applying the large number ratios to this hypothetical formula:
and applying the large number ratios to this hypothetical formula:
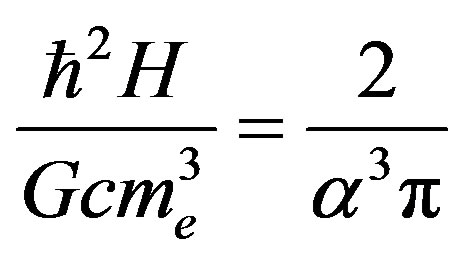 (47)
(47)
Example 3.
J. Bekenstein proposed [18] universal entropy bound for a complete physical system whose total mass-energy (in our case) is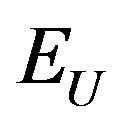 , and which fits inside a sphere of radius
, and which fits inside a sphere of radius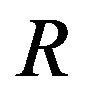 . Applying the large numbers ratios, we can get the universal entropy bound value:
. Applying the large numbers ratios, we can get the universal entropy bound value:
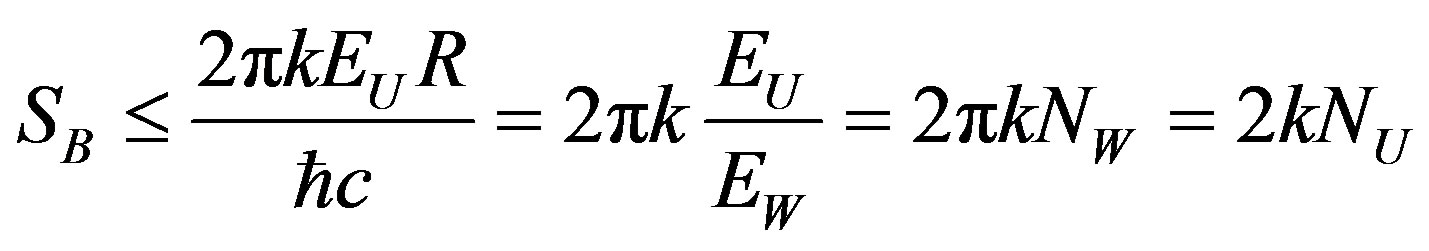 (48)
(48)
Where 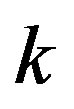 is Boltzmann constant.
is Boltzmann constant.
On the other hand, the Bekenstein-Hawking entropy bound for the Black Hole with radius 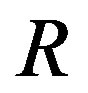 is:
is:
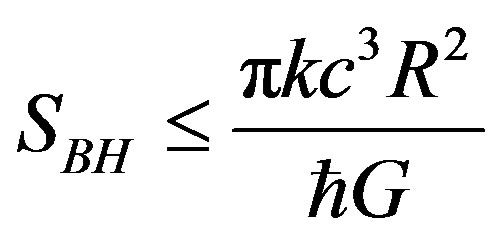 (49)
(49)
By dividing (48) by (49) and using (8) one can get:
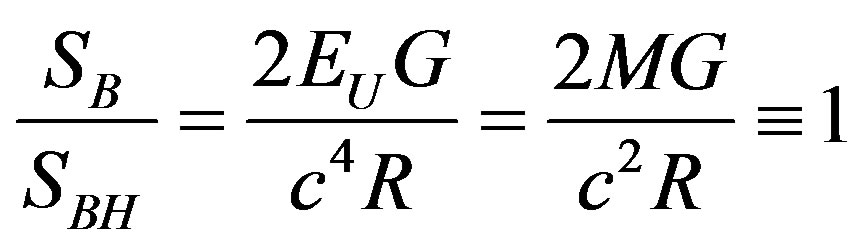 (50)
(50)
The last one says that both Bekenstein universal entropy bound and Bekenstein-Hawking Black Hole entropy bound have the same value in our model and equal exactly to :
:
 (51)
(51)
Using the large number ratios it is also easy to express the Hawking radiation 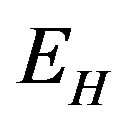 of our Black Hole with energy
of our Black Hole with energy  via Largest number
via Largest number 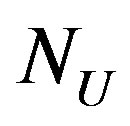 or Hubble parameter:
or Hubble parameter:
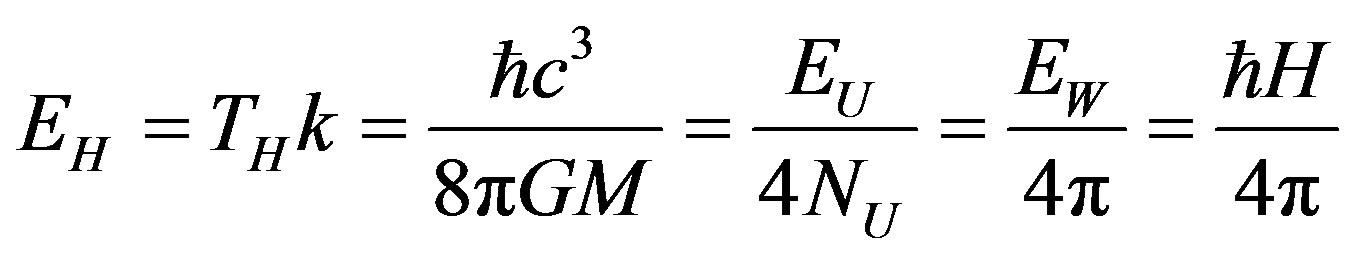 (52)
(52)
where  is Hawking radiation temperature.
is Hawking radiation temperature.
6. Discussion and Conclusions
The two currently prevailing physical theories, i.e. quantum mechanics and the general relativity, describe the reality very precisely, each in its range of energy and spatial scale. It is presumed that sometimes in the future, the value of  will be obtained in theory directly as a direct result of consolidation of gravitation with other known interactions, strong and electroweak.
will be obtained in theory directly as a direct result of consolidation of gravitation with other known interactions, strong and electroweak.
The fruitless attempts at explaining the proximity of  and
and ![]() resulted in a broad application of the term of “coincidence” that somehow highlights the randomness of the event. As shown in previous sections it is not random.
resulted in a broad application of the term of “coincidence” that somehow highlights the randomness of the event. As shown in previous sections it is not random.
As we see, the large number ratios proposed in the article provide the powerful means for finding relations among various information and physical parameters of our model universe. However, it does not help answer the main question: where do these enormous numbers come from in physics? Hopefully, the law and hypotheses proposed in this article will let find the correct answer in the foreseeable future.
The ratios we suggested impose rather many stringent limitations on the way physical constants may change over time. We must note that the sharply tuned combination of large numbers, including the mentioned approximations for Hubble time![]() , mass of the universe
, mass of the universe  and Hubble limit
and Hubble limit 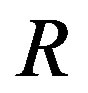 correspond to the values of the very precisely measured physical constants of quantum scale. Looking at (39) and (40), it is obvious that the gravity is closely connected with the Hubble radius, Hubble time and the properties of electron. However, there are rather reliable measurements that establish a very low limit for the Newton constant change rate in the long-term
correspond to the values of the very precisely measured physical constants of quantum scale. Looking at (39) and (40), it is obvious that the gravity is closely connected with the Hubble radius, Hubble time and the properties of electron. However, there are rather reliable measurements that establish a very low limit for the Newton constant change rate in the long-term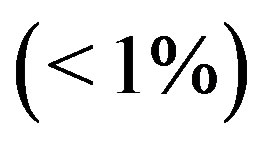 . Thus, if Hubble parameters
. Thus, if Hubble parameters ![]() varies with time then the corresponding variation of
varies with time then the corresponding variation of ![]() or/and electron energy
or/and electron energy 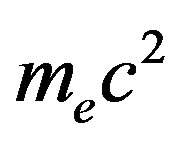 should preserve the constancy of
should preserve the constancy of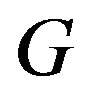 .
.
If the entire universe energy 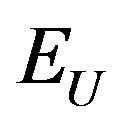 comprises (or once comprised) quantums of the minimum energy
comprises (or once comprised) quantums of the minimum energy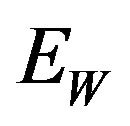 , we should make the conclusion that number (14) is a natural number. The same is true about (15). Hence, we can establish the hypothesis that all large numbers in the real (not infinite) universe should be naturals or rationals. However, the denominator and the numerator in these fractional numbers are so great that one can confidently presume the real large numbers approximate some perfect limit of fundamental importance infinitely closely. Taking into account the transcendentalism of (29), (31) and (35), we dare to express one more hypothesis: all Large numbers are infinitely approaching their limits and these very limits are transcendent mathematical constants.
, we should make the conclusion that number (14) is a natural number. The same is true about (15). Hence, we can establish the hypothesis that all large numbers in the real (not infinite) universe should be naturals or rationals. However, the denominator and the numerator in these fractional numbers are so great that one can confidently presume the real large numbers approximate some perfect limit of fundamental importance infinitely closely. Taking into account the transcendentalism of (29), (31) and (35), we dare to express one more hypothesis: all Large numbers are infinitely approaching their limits and these very limits are transcendent mathematical constants.
The Schwarzschild solution is one of the well known models of our universe, representing a Black Hole. Avoiding creation of new essences, we just consider the interaction between internal radiation and a small spherical observer. It appears that such a simple model reveals a lot of interesting identities and conservation laws. For example, the value of 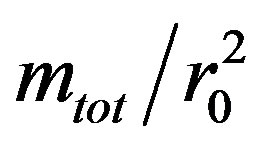 should be conserved for a sphere with a radius
should be conserved for a sphere with a radius![]() . Furthermore we found that identity
. Furthermore we found that identity  is valid for a sphere with classical electron radius
is valid for a sphere with classical electron radius![]() .
.
The last derivation allowed us to propose a list of exact ratios between well known Eddington-Weyl-Dirac large numbers which are listed in Table 2. The ratios (30) and (39) reveals the exact formulae supporting P. Dirac’s hypothesis and propositions which were announced many years ago and never were written in a clear mathematical form.
Using these large numbers ratios we have claimed the new Largest number law (35) which is based on Lambert’s cosine law and the rotational symmetry of space. It is quite important that this law precisely unites the major cosmological and quantum parameters of our universe. It could be interpreted as follows: our universe comprises of the mathematically determined number of elementary spatial clusters which can be matched up to energy quanta and angular momentum quanta. We should note that basic parameters of our model universe have been obtained form the properties of electron, speed of light and fine structure constant.
If Equations (13), (28) and (31) are valid inside the Hubble sphere, which is very probable, we should make a conclusion that all cosmological parameters are fully and unambiguously determined by quantum and mathematical constants. Simply put, all of us are very likely to live in an extremely precisely self-tuned quantized universe.
REFERENCES
- H. Weyl, Annalen der Physik, Vol. 359, 1917, pp. 117- 145. http://dx.doi.org/10.1002/andp.19173591804
- H. Weyl, “The Open World Yale,” Oxbow Press, Oxford, 1989.
- H. Weyl, “Space Time Matter,” Methuen, Dover, 1952.
- A. S. Eddington, “The Math. Theory of Relativity,” Cambridge University Press, Cambridge, 1924.
- A. S. Eddington, “Fundamental Theory,” Cambridge University Press, Cambridge, 1946.
- P. C. W. Davies, “The Accidential Universe,” Cambridge University Press, Cambridge, 1982.
- S. Ray, U. Mukhopadhyay and P. P. Ghosh, Large Number Hypothesis: A Review, 2007.
- K. A. Tomilin, Issledovaniya po Istorii Fiziki i Mekhaniki, Vol. 141, 1999.
- P. A. M. Dirac, Nature, Vol. 139, 1937, p. 323. http://dx.doi.org/10.1038/139323a0
- P. A. M. Dirac, Proceedings of the Royal Society A, Vol. 165, 1938, p. 199.
- P. A. M. Dirac, Nature, Vol. 1001, 1937.
- E. A. Milne, Proceedings of the Royal Society A, Vol. 158, 1937, p. 324.
- P. J. Mohr, B. N. Taylor and D. B. Newell, The 2010 CODATA Recommended Values of the Fundamental Physical Constants, National Institute of Standards and Technology, Gaithersburg, 2011. http://physics.nist.gov/constants
- C. Planck, P. A. R. Ade, N. Aghanim, C. Armitage-Caplan, M. Arnaud, et al., Planck 2013 Results, Cosmological parameters, 2013.
- J. Casado, Connecting Quantum and Cosmic Scales by a Decreasing-Light-Speed Model, 2004.
- E. Teller, Physical Review, Vol. 73, 1948, pp. 801-802. http://dx.doi.org/10.1103/PhysRev.73.801
- S. Weinberg, “Gravitation and Cosmology,” Wiley, New York, 1972.
- J. D. Bekenstein, Physical Review D, Vol. 23, 1981, pp. 287-298. http://dx.doi.org/10.1103/PhysRevD.23.287
NOTES
*Yan Ryazantsev, Independent researcher.

