Theoretical Economics Letters
Vol.2 No.1(2012), Article ID:17374,4 pages DOI:10.4236/tel.2012.21015
Weak and Strong Time Consistency
Department of Economics, University of Mumbai, Mumbai, India
Email: romarcorrea10@gmail.com
Received October 21, 2011; revised November 20, 2011; accepted November 30, 2011
Keywords: information sets; discretion
ABSTRACT
We motivate and provide proofs of Başar and Olsder’s (1995) theorems on the subject. The context is the increasing appreciation that the neoclassical framework is not the only model of the economy.
1. Introduction
The longstanding critique of the Dynamic Stochastic General Equilibrium (DSGE) model has gained widespread publicity as a consequence of the recent financialreal meltdown [1]. Of interest to us are an assumption and a theorem. The assumption of rational expectations is that agents access all available information. DSGE theorems leave no room for inefficient outcomes. Some of the profession’s most respected scholars at the Hearings referred to drew attention to the weight of evidence suggesting that the choice of information sets by people is a nontrivial matter. Secondly, an outcome might be a Keynes equilibrium like involuntary unemployment. A suite of orientations is available to incorporate these insights [2,3].
A corollary of DSGE results is the time inconsistency of optimal policy. A game is played by the monetary authorities (MA) and the private sector. A Nash equilibrium in the inflation rate will exist in the present. However, in any following period, the MA has an incentive to generate a higher rate of inflation in order to stimulate activity. The gain is short term. In the long run, the economy will tend to the natural rate of unemployment with the higher rate of inflation. Consequently, the task is to remove discretion from the MA and subject monetary policy committees to rules.
Although the language of differential games is used, the nuance of the title of the paper will not be found in the economics literature. We exploit the distinction to show, under specified conditions, that discretion is not inferior to rules.
2. The Result
The following account is drawn from [4]. The loop model of dynamic games allows for two possible equilibrium solutions. In the prior commitment mode of play, decisions are made at the outset. Feedback games, on the other hand, are of the delayed commitment type. Each player waits to find out the current value of the state vector and then announces her action. Time inconsistency might be expected in prior commitment decisions. In the absence of devices that tie the players’ hands in advance, there is an incentive for any one to recompute her strategy in each period based on the information that is forthcoming. In the macroeconomic illustrations, the payoff to the government (leader) by “cheating” thereby increases and the payoffs to members of the private sector (follower) fall. In what follows, therefore, we confine ourselves to the Feedback Nash Equilibrium (FNE) Solution.
Definition 1. A two-person discrete-time deterministic infinite dynamic game of fixed duration involves:
1) An index set 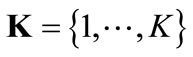 denoting the stages of the game.
denoting the stages of the game.
2) An infinite set X with some topological structure called the state space of the game to which the state of the game xk belongs for all .
.
3) An infinite set 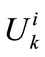 with some topological structure defined for each
with some topological structure defined for each  and player i called the action set of Pi at stage k. Its elements are permissible actions
and player i called the action set of Pi at stage k. Its elements are permissible actions 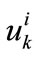 of Pi at stage k.
of Pi at stage k.
4) A function 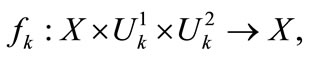 defined for each
defined for each
 , so that
, so that 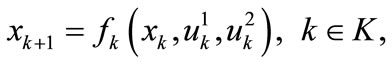 for some
for some
 called the initial state of the game. The difference equation above is called the state equation of the dynamic game.
called the initial state of the game. The difference equation above is called the state equation of the dynamic game.
5) A finite set 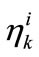 defined for each
defined for each  and player i as a subcollection of
and player i as a subcollection of

which determines the information gained and recalled by Pi at stage k. Specification of 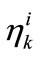 for all stages k characterizes the information structure of Pi and the collection over both players of their information structures is the information structure of the game.
for all stages k characterizes the information structure of Pi and the collection over both players of their information structures is the information structure of the game.
6) A set 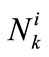 defined for each
defined for each  and player i as an appropriate subset of
and player i as an appropriate subset of
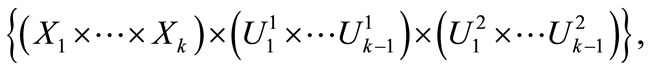
compatible with 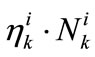 is called the information space of Pi at stage k.
is called the information space of Pi at stage k.
7) A prespecified class 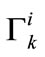 of mappings
of mappings 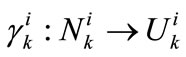 which are the permissible strategies of Pi at stage k. The aggregate mapping
which are the permissible strategies of Pi at stage k. The aggregate mapping 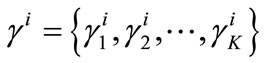 is a strategy for Pi in the game and the class Gi of all such mappings gi so that
is a strategy for Pi in the game and the class Gi of all such mappings gi so that 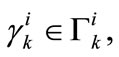
 , is the strategy space of Pi.
, is the strategy space of Pi.
8) A functional
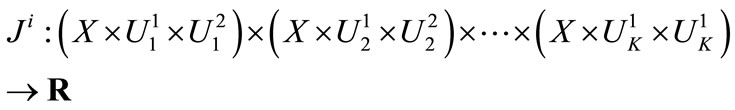
defined for each player i called the cost functional of Pi. The cost functional is said to be stage-additive if there exist , (
, ( ), so that
), so that

where 
The information structures of relevance are as follows:
Definition 2. Pi’s information structure is said to be open-loop (OL) if 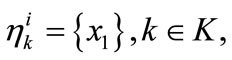
closed-loop perfect state (CLPS) if
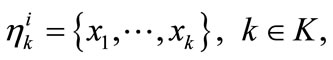
memoryless perfect state (MPS) if
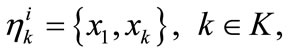
feedback perfect state (FB) if 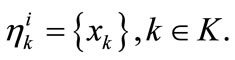
An important distinction, for our purposes, rests between the CLPS and MPS structures, on the one hand, and the FB on the other. Rational expectations is consistent with the former. History matters. If, on the other hand, agents suffer from memory loss or choose not to access past data because of cognitive or out-of-pocket costs, the latter prevails. In the buildup to the present recession, for instance, people seem to have forgotten previous recessions and history given by the complete cycle.
The Noncooperative (Nash) Equilibrium Solution is given by Definition 3. A pair of strategies  with
with
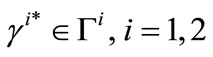 is said to constitute a Nash Equilibrium Solution if, and only if, the following inequalities are satisfied for all
is said to constitute a Nash Equilibrium Solution if, and only if, the following inequalities are satisfied for all 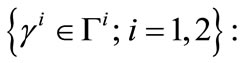
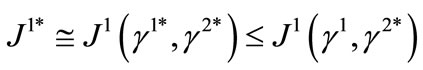
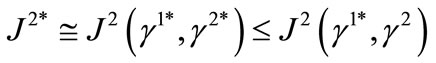
In the next section we restrict our attention to feedback games where at the time of her act each player has perfect information concerning the current level of play. In that case, the set of inequalities above is rewritten as Definition 4. A pair of strategies  constitutes a Feedback Nash Equilibrium Solution if it satisfies the following inequalities for all
constitutes a Feedback Nash Equilibrium Solution if it satisfies the following inequalities for all 

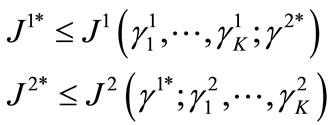
On any pair of Nash equilibrium strategies that satisfies the above inequalities, impose the further restriction that it satisfies the following K inequalities:
At stage K 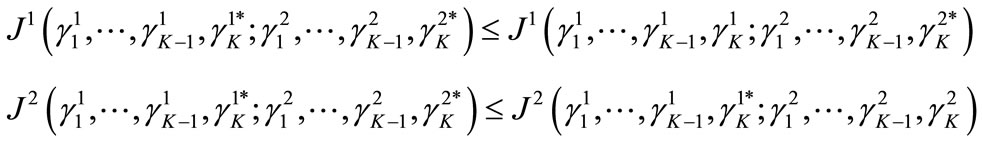
At stage K – 1 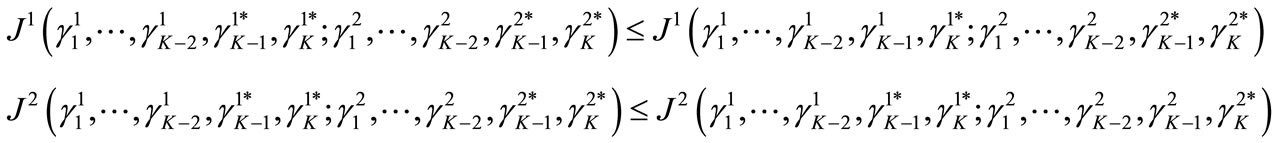
At stage 1 
For appreciating the notion of time consistency, the following notation and definitions are required. Following Başar and Jan Olsder, we abuse notation by using the denoting the discrete time period by the continuous time period. Thus,  Let
Let

denote a two-person dynamic game where Г is the product strategy space, 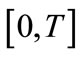 is the decision interval and N stands for the Nash solution concept. Also, let
is the decision interval and N stands for the Nash solution concept. Also, let
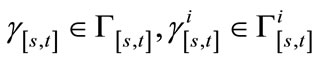
denote the truncations of 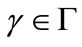 and
and  respectively, to the time period
respectively, to the time period 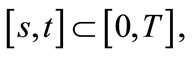 and let
and let

denote a version of 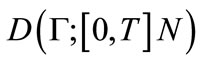 where the strategies of both players i in the intervals
where the strategies of both players i in the intervals 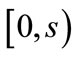 and
and 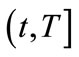 are fixed as
are fixed as 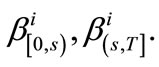 In that case, we have Definition 5. A pair of strategies
In that case, we have Definition 5. A pair of strategies 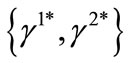 solving the dynamic game
solving the dynamic game  is said to be weakly time consistent (WTC) if its truncation to the interval
is said to be weakly time consistent (WTC) if its truncation to the interval 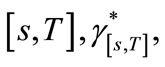 is the solution of the truncated game
is the solution of the truncated game 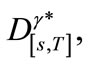 for all
for all  If a solution
If a solution  is not WTC, it is time inconsistent.
is not WTC, it is time inconsistent.
Definition 6. A pair of strategies 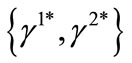 solving the dynamic game
solving the dynamic game 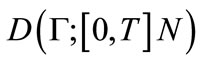 is said to be strongly time consistent (STC) if its truncation to the
is said to be strongly time consistent (STC) if its truncation to the  is the solution of the truncated game
is the solution of the truncated game 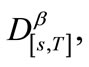 for every
for every
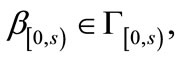 this being so for every
this being so for every 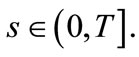
In the case of Definition 5 there is no basis at k for cheating at future stages only if the past actions are consistent with the original solution, whereas in the instance of Definition 6, this is true even if there have been deviations in the past from the actions dictated by the optimal strategy.
Definition 7. A strategy 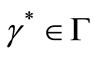 is said to be a representation of another strategy
is said to be a representation of another strategy  if 1) they both generate the same unique state trajectory and 2) they both have the same open-loop value on this trajectory.
if 1) they both generate the same unique state trajectory and 2) they both have the same open-loop value on this trajectory.
The importance of the notion of representations is that it enables the construction of equivalence classes of equal open-loop value strategies in the general class of closedloop strategies. The procedure is as follows. For the system defined in Definition 1, first determine the set of all elements of Г which are strategies that depend only on the initial state x1. The set thus constructed is the class of permissible open-loop controls in Г. Now let
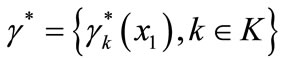
be an element of the set of open-loop controls which generates by substitution into the state equation of Definition 1, a unique trajectory 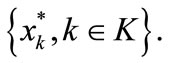 Then, consider all elements
Then, consider all elements  of Г with the properties 1) γ generates the same trajectory as
of Г with the properties 1) γ generates the same trajectory as 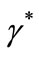 and 2)
and 2) 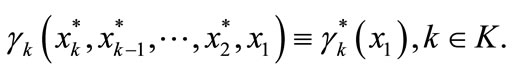
If this procedure is repeated for every element of the set of open-loop controls, the construction of all equivalence classes of Г becomes complete. In our terms, outcome space is dense with macroeconomic configurations. The DSGE class is one. The analyst is free to choose from a multiplicity of models.
The basic proposition is as follows:
Theorem 1) The FNE Solution under the CLPS or MPS information pattern is strongly time consistent.
2) The FNE Solution under the FB information pattern is weakly time inconsistent.
Proof. 1) Consider a FNE solution 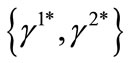 till stage k. Note that the star is only used for notational brevity and does not rule out the possibility of different equilibria for subgames preceding stage k. At that stage, P1, say, decides to renege on this solution. It must be the case that for some strategy
till stage k. Note that the star is only used for notational brevity and does not rule out the possibility of different equilibria for subgames preceding stage k. At that stage, P1, say, decides to renege on this solution. It must be the case that for some strategy 

The defection under CLPS or MPS implies entry into an information equivalence class where

Accordingly, the evolution of the state till stage k is given by  It turns out, therefore, that for agent P1, either
It turns out, therefore, that for agent P1, either
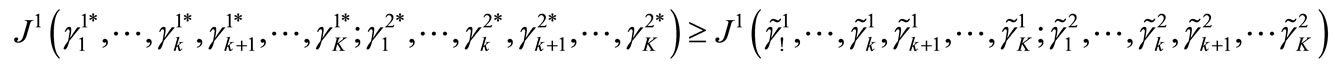
or

If the first inequality holds, the assumption that the agents were parties to the FNE solution under the CLPS or MPS information pattern is violated. If the second inequality is valid, the assumption of the deviation from the FNE solution does not hold.
2) On the other hand, suppose the players were operating under the FB information pattern. At stage k, the players decide to recommit. Their inducement to do so must be
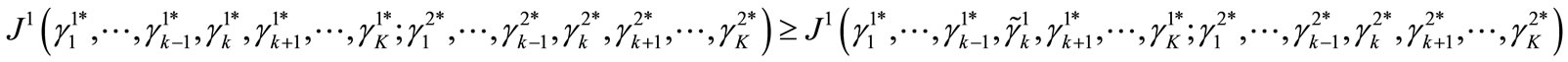
and
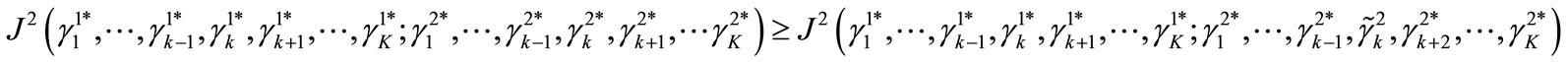
where  is the feedback strategy under the FB information pattern. Their actions determine a new initial condition for the game
is the feedback strategy under the FB information pattern. Their actions determine a new initial condition for the game 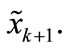 We can design the set of strategies which only depend on this new initial state and the time periods that follow. This would be the class of open-loop controls. An element would be
We can design the set of strategies which only depend on this new initial state and the time periods that follow. This would be the class of open-loop controls. An element would be
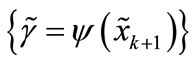 which generates by substitution into the state evolution equation a unique trajectory
which generates by substitution into the state evolution equation a unique trajectory  We can proceed to construct an equivalence class of representations all of which have the same value
We can proceed to construct an equivalence class of representations all of which have the same value 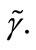
3. Conclusions
If agents access all the information they can command and the economy is described by a dynamic general equilibrium model, there is no incentive for any player to ‘cheat’ on the equilibrium. The DSGE model applies. On the other hand, if, under the same information conditions, the economy is characterized by a Keynesian model, agents will be locked into a “bad” equilibrium. Only a regime change will transfer the system into a superior equivalence class of solutions.
If, for reasons like myopia, agents are bound by the information provided in the present and the solution of the state equation of the game is an unemployment equilibrium, all of them are better off reneging on the Nash outcome into a dominating equivalence class of solutions. The mainstream response would that it is precisely when agents do not look to the past and, a fortiori, the future, that they are tempted out of the noncooperative equilibrium. However, no player is worse off in these circumstances. Credibility and a good reputation, in this instance, are at a discount.
REFERENCES
- United States House of Representatives, “Building a Science of Economics for the Real World,” website for the Committee on Science and Technology, 20 July 2010.
- D. Colander, “How Did Macro Theory Get So Far off Track and What Can Heterodox Macroeconomics Do to Get Back on Track?” Middlebury College Economics Discussion Paper No. 09-11, 2009.
- P. Omerod, “Keynes, Hayek and Complexity,” In: M. Faggini and T. Lux, Eds., Coping with the Complexity of Economics, Springer-Verlag, Rome, 2009, pp. 19-32. doi:10.1007/978-88-470-1083-3_2
- T. Başar and G. J. Olsder, “Dynamic Noncooperative Game Theory,” Academic Press, London, 1995.

