Received 15 June 2016; accepted 26 August 2016; published 29 August 2016

ABSTRACT

In this paper, we investigate the asymptotic behavior of the following quasilinear difference equations
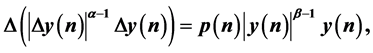(E)
where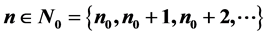,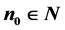. We classified the solutions into six types by means of their asymptotic behavior. We establish the necessary and/or sufficient conditions for such equations to possess a solution of each of these six types.

Keywords:

Asymptotic Behavior, Positive Solutions, Homogeneous, Quasilinear Difference Equations

1. Introduction

Recently, the asymptotic properties of the solutions of second order differential equations [1] [2] difference equations of the type (E) and/or related equations have been investigated by many authors, for example see, [3] - [19] and the references cited there in. Following this trend, we investigate the existence of these six types of solutions of the Equation (E) showing the necessary and/or sufficient conditions can be obtained for the existence of those solutions. For the general backward on difference equations, the reader is referred to the monographs [20] - [24] .

In 1996, PJY Wang and R.P. Agarwal [25] considered the quasilinear equation

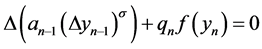(1)

and obtained oscillation criteria for the Equation (1).

In 1996, E. Thandapani, M.M.S. Manuel and R.P. Agarwal [26] have studied the quasi-linear difference equation

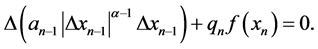(2)

In 2000, Pon Sundaram and E. Thandapani [27] considered the following quasi-linear functional difference equation

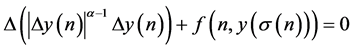(3)

and they have established necessary and sufficient conditions for the solutions of Equation (3) to have various types of nonoscillatory solutions. Further they have established some new oscillation conditions for the oscillation of solutions of Equation (3).

In 1997, E. Thandapani and R. Arul [28] studied, the following quasi-linear equation

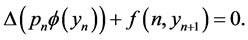(4)

They established necessary and sufficient conditions for the solutions of (4) to have various type of nono- scillatory solutions.

In 2004, E. Thandapani et al. [29] studied the equation

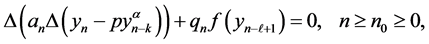(5)

and established conditions for the existence of non-oscillatory solutions.

S.S. Cheng and W.T. Patula [30] studied the difference equation

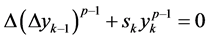(6)

where 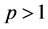and proved an existence theorem for Equation (6).

In 2002, M. Mizukanmi et al. [1] discussed the asymptotic behavior of the following equation

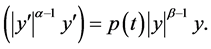(7)

Discrete models are more suitable for understanding the problems in Economics, genetics, population dynamics etc. In the qualitative theory of difference equations asymptotic behavior of solutions plays a vital role. Motivated by this, we consider the discrete analogue of (7) of the form

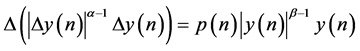(8)

where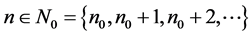, 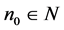and 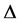is the forward difference operator defined by

We assume the following conditions on Equation (8)

1) and are positive constants

2) is a real sequence such that for all.

For simplicity, we often employ the notation

interms of which Equation (8) can be expressed in

By a solution of Equation (8), we mean a real sequence, together with exists and satisfies Equation (8) for all.

We here call Equation (8) super-homogeneous or sub-homogeneous according as α < β or α > β If α = β Equation (8) is often called half-linear. Our attention is mainly paid to the super-homogeneous and sub-homo- geneous cases, and the half-linear is almost excluded from our consideration.

2. The Classification of All Solutions of Equation (8)

To classify all solutions of Equation (8), we need the following lemma.

Lemma 1. Let be a local solutions of Equation (8) near and, be its right

maximal interval of existence. Then we have either near w or near w. That is does

not charge strictly its sign infinitely many times as.

The classification of all (local) solutions of Equation (8) are given on the basis of Lemma 1. Since the proof is easy, we leave it to the reader.

Proposition 1. Each local solution of Equation (8) falls into exactly one of the following six types.

1) Singular solution of the first kind: type there exist a such that

2) Decaying solution: type (D), can be continued to ¥, and satisfies for all large n, and

3) Asymptotically constant solution: type (AC) can be continued to ¥, and satisfies for all large n and

4) Asymptotically linear solution: type (AL) can be continued to ¥ and satisfies for all large n and

5) Asymptotically super-linear solution: type (AS) can be continued to ¥ and satisfies for all large n and

6) Singular solution of second kind: type has the finite escape time; that is, there exists a such that

3. Main Results for the Super-Homogeneous Equations

Before we list our main results for the case. Throughout this section we assume that

Theorem 2. Equation (8) has no solution of type.

Theorem 3. Equation (8) has a solution of type (D) if and only if

(9)

Theorem 4. Equation (8) has a solution of type (AC) if and only if

(10)

Theorem 5. Equation (8) has a solution of type (AL) if and only if

(11)

Theorem 6. Equation (8) has a solution of type (AS) if (11) holds.

Theorem 7. Equation (8) does not have solutions of type (AS) if there are constants and satisfying

(12)

and

(13)

Remark 1. The set of all pairs satisfying inequalities (13) is not empty. In fact, the pair

belongs to it.

Theorem 8. Equation (8) has a solutions of type.

Remark 2. Theorem 7 has the same conclusion that these are not solutions of type (AS). However, Theorem 7 is still valid for the case that p is nonnegative. For example, it is formed by this extended version of Theorem 7 that the equation

does not have solutions of type (AS).

Example 1 Let, consider the Equation (8) with

(14)

For this equation, we have the following results:

1) Equation (14) has a solution of type (D) if and only if (Theorem 3).

2) Equation (14) has a solution of type (AC) if and only if (Theorem 4).

3) Equation (14) has a solution of type (AL) if and only if (Theorem 5).

4) Equation (14) has a solution of type (AS) if and only if (Theorem 6).

4. Main Results for the Sub-Homogeneous Equation

Below we list our main results for the case. Throughout this section we assume that.

Theorem 9. Equation (8) has a solutions of type.

Theorem 10. Equation (8) has a solution of type (D) if

(15)

Theorem 11. Equation (8) does not have solutions of type (D) if

(16)

Theorem 12. Equation (8) does not have solutions of type (D) if there are constants and satisfying

(17)

and

(18)

Remark 3. The set of all pairs satisfying inequalities (18) is not empty. In fact, the pair

belongs to it.

Theorem 13. Equation (8) has a solution of type (AC) if and only if (15) holds.

Theorem 14. Equation (8) has a solution of type (AL) if and only if

Theorem 15. Equation (8) has a solution of type (AS) if and only if

(19)

Theorem 16. Equation (8) has no solutions of type.

Example 2. Let and consider the Equation (14) again.

We have the following results:

1) Equation (14) has a solution of type (D) if and only if (Theorem 10 and 11).

2) Equation (14) has a solution of type (AC) if and only if (Theorem 14).

3) Equation (14) has a solution of type (AL) if and only if (Theorem 15).

4) Equation (14) has a solution of type (AS) if and only if (Theorem 16).

5. Auxillary Lemma

In this section, we collect axillary lemmas, which are mainly concerned with local solution of Equation (8). A comparison lemma of the following type is useful, and will be used in many places.

Lemma 2. Suppose that are such that for. Let and be solutions of the equations

respectively. If and, then and for a < n ≤ b.

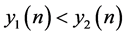
Proof. We have

(20)

(21)

By the hypotheses we have in some right neighborhood of a. If for some point in a < n ≤ b, we can find a c such that a < c ≤ b satisfying for a < n < c and. But, this yields a contradiction, because

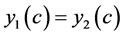
Hence we see that for. Returning to (20), we find that for. The proof is complete.

The uniqueness of local solutions with non-zero initial data can be easily proved. That is, for given, and, Equation (8) has a unique local solution satisfying, provided that. The uniqueness of the trivial solution can be concluded for the case.

Lemma 3. Let α ≤ β and. If is a local solution of Equation (1) satisfying then for.

Proof. Assume the contrary. We may suppose that for. Then, we can find such that satisfying and for. Summing (8), we obtain

We therefore have

(22)

(23)

Put. We see that for and w is nondecreas- ing. From (22) and (23), we can get

Let. Then from this observation we see that

where

Consequently, we have

(24)

If α = β, from (24), we have,. This is a contradiction because. If α < β, from

(24) we have,. This is also a contraction because.

The proof is complete.

Lemma 4. Let. Then all local solutions of Equation (8) can be continued to ¥ and, that is, all solutions of Equation (8) exist on the whole interval.

Proof. Let be a local solution of Equation (8) is a neighborhood of. Suppose the contrary that the right maximal interval of existence of is of the form,. Then, it is easily seen that. Summing (8) twice, we have

where and. Accordingly,

Put. Then,

Put moreover. Then, as in the proof of Lemma 3, we have

(25)

where. Since, there is a such that such that for. Therefore it follows from (25) that

(26)

Let. Then, using discrete Gronwall’s inequality, we see that, which is a contradiction.

Next let. Then (26) implies that

Since, we have. This is a contradiction too. Hence can be continued to ¥. The continuability to the left end point is verified in a similar way. The proof is complete.

The following lemma establishes more than is stated in Theorem 8. Accordingly the proof of Theorem 8 will be omitted.

Lemma 5. Let and and be given. Then there exists an such that the right maximal interval of existence of each solution of Equation (1) satisfying and

is a finite interval, , and.

Proof. Let be fixed, and put. There is an satisfying

We first claim that the solution of Equation (8) with the initial condition, does not exist on; that is blow up at some. To see this suppose the contrary that exists at least. By the definition of m, we have

Summing the inequality form N to yields

and hence

Finally, summing the above inequality both sides from N to, we obtain

which is a contradiction to the choice of M. Hence must blow up at some,.

If and, then Lemma 2 implies that on the common interval of existence of y and z and therefore blows up at some point before. The proof is complete.

6. Nonnegative Nonincreasing Solutions

The main objective of this section is to prove the following theorem.

Theorem 17. For each, the problem

has exactly one solution such that is defined for and satisfies

(27)

Furthermore, if is a solution for of Equation (1) satisfying and

then

Remark 4.

1) In the case, employing Lemma 3, we can strengthen (27) to the property that

(28)

2) In the case, all local solutions of Equation (8) can be continued to the whole interval Hence in this case property (6.2) always holds for all solutions with and [resp].

The property of nonnegative nonincreasing solutions described in Theorem 17 will play important roles through the paper. This section is entirely derided to proving Theorem 17. To this end we prepare several lemmas.

Lemma 6. Let 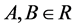and t be a bounded function on. Then, the two point boundary value problem

(29)

has a solution.

Proof. Let be a constant such that

We first claim that with each, we can associate a unique constant satisfying

(30)

Further this satisfies

(31)

To see this let be fixed, and consider the function

If, then. If, then. Since

I is a strictly increasing continuous function, there is a unique constant satisfying, namely (30). Then (31) is clearly satisfied.

By (31), we see that there is a constant satisfying for all. Choose so large that

Consider the Banach space B_N of all real sequences with the supernum norm.

Now we define the set and the mapping by

and

respectively. Then the boundary value problem (29) is equivalent to finding a fixed element of. We show that F has a fixed element in Y (via) the Schavder fixed point theorem

Hence F maps Y into itself.

Next, to see the continuity of F, assume that be a sequence converging to uniformly in. We must prove that converges to uniformly in. As a first step, we show that

. Assume that this is not the case. Then because of the boundedness of,

there is a subsequence satisfying for some finite value. Noting the relation

We have

This contradicts the uniqueness of the number. Hence. Then we find similarly that uniformly on.

It will be easily seen that the sets

are uniformly bounded on. Then is compact.

From the above observations we see that F has a fixed element in Y. Then this fixed element is a solution of boundary value problem (29) is easily proved. The proof is now complete.

Lemma 7. Let and. Then the two point boundary value problem

(32)

has a solution such that and for.

Proof. Define the bounded function f on by

By Lemma 6, the boundary value problem

has a solution y.

We show that y satisfies for. If this is not the case, we can find an interval such that on and. The definition of f implies that y

satisfies the equation on. Hence is a linear function on.

Obviously that this is a contradiction. We see therefore that on.

Since and on, by the definition of t, we find that

on. Hence, which implies that y is a desired solution of problem (32). The proof is complete.

Proof of Theorem 17. The uniqueness of satisfying the properties mentored here is easily established as in the proof Lemma 2. Therefore we prove only the existence of such a.

By Lemma 7, for each, we have a solution of the boundary value problem

satisfying and for let us extend each over the interval by defining for. Below we show that contains a subsequence converging to a desired solution of (8).

As a first step, we prove that

(33)

In fact, if this is not case, then for some i. Since. Lemma 2 implies that for. Putting, we have a con-

tradiction. Accordingly (33) holds, and so exists, since on

for any, is uniformly bounded on each compact subinterval of. Noting that is nondecreasing and nonpositive on, we have

Hence is equicontinuous on each compact subinterval of. From these consideration we

find that there is a subsequence and a function satisfying uni-

formly on each compact subinterval of. Finally we shall show that is a desired solution of Equation (8). Let be fixed arbitrarily. For all sufficiently large’s we have

letting, we obtain

Taking difference in this above equality, we are that solves Equation (8) on. That satisfies (27) is evident. The proof of Theorem 17 is complete.

7. Proofs of Main Results for the Super-Homogeneous Equations

Throughout this section, we assume that.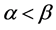

Proof of Theorem 2. The theorem is an immediate consequence of the uniqueness of the trivial solution (Lemma 3).

Proof of Theorem 4. Necessity Part: Let be a positive solution of Equation (8) for of type

(AC). It is easy to see that and as. Hence summing (8) twice, we have

from which we find that

This is equivalent to (10).

Sufficiency Part: Let (10) hold. Fix an and choose so that

We introduce the Banach space of all bounded, real sequences with norm.

Define the set and the mapping by

We below show via the Schauder-Tychonoff fixed point theorem that F has at least one fixed element in Y. Firstly, let. Then

Thus, and hence. Secondly, to see the continuity of F, let be a sequence in Y covering to uniformly on each compact subinterval of since is bounded for and

The Lebesgue dominated convergence theorem implies that uniformly on each compact subinterval of since for,

The set is uniformly bounded on. This implies that is compact.

From there observations we find that F has a proved element y in Y such that. That this y is a solution of Equation (1) of type (AC) is easily proved. The proof is complete.

Proof of Theorem 3. Sufficiency Part: Let be a solution of Equation (8) satisfying for. The existence of such a solution is ensured by Theorem 17. Obviously, is either of type (D) or type (AC). Theorem 4 shows that under assumption (9), Equation (8) does not posses solutions of type (AC). Hence must be of type (D).

Necessity Part: Let be a positive solution of Equation (8) for of type (D). Clearly satisfies

To verify (9), suppose the contrary that (9) fails to hold. Then, nothing that is decreasing for, we have

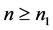
Accordingly,

The left hand side tends to ¥ as because of, where as the right hand side tends to 0 as. This contradiction verifies (9). The proof is complete.

Proof of Theorem 5. Necessity Part: Let be a positive solution of Equation (8) near ¥ of type (AL). There is a constant and satisfying

(34)

Summation of Equation (8) from to yields

Since, this in equality implies that

(35)

Combining (35) with (34), we find that (11) holds.

Sufficiency Part: We fix arbitrarily, and choose large enough so that

Let be the Banach space as in the proof Theorem 4. Define the set as follows

The mapping defined by

As in the proof of the sufficiency part of Theorem 4, we can show that F has a fixed element by the Schavder-Tyehonoff fixed point Theorem

Taking D twice for this formula we see that is a positive solution of Equation (8) for.

L’Hospital’s rule shows that. Thus is a solution of Equation (8) of type (AL). The proof

is complete.

Lemma 8. Let. If (11) holds, then there is a positive solution of Equation (8) for of type (AL) satisfying.

Proof. By Theorem 5, there is an (AL)-type positive solution of Equation (8) defined in some neigh-

borhood of. Let be a positive solution of Equation (8) for

satisfying and, for. Take a such that and

for. By Lemma 2 if is sufficiently elver to, then the solution

of Equation (8) with and exists at least on and satisfies

Then Lemma 2 again implies that as long as exists. Since and exists for, this means that exists for and satisfies,. Then we have

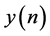
Noting that is the unique solution of (8) satisfying and passing through the point we have. Therefore is of type (AL). The proof is complete.

Proof of Theorem 6. For, we denote by, the unique solution of Equation (8) with in initial condition and. The maximal interval of existence of may be finite or infinite.

Define the set by

We know by Lemma 8 that and by Lemma 5 that for all sufficiently large. Hence exists. For there are three possibilities:

1)

2) and is of type (AS)

3) and is of type.

To prove the theorem, we below show that case (b) occurs. For simplicity, we write for below.

Suppose that the case (a) occurs. Then and. By condition

(11) we can find a satisfying

Choose close enough to so that exists at least on and. Then, for such a, can be extended to, and satisfies. In fact, if this is not the case, there is satisfying for and. It follows therefore that for. Summing the Equation (8) (with) for yields

This contradiction implies that exists for and satisfies. These observations show that, which contradicts the definition of. Hence case (a) does not occur.

Next, suppose that case (c) occurs. Let be the point such that. By Le- mma 5, there is an such that solution of Equation (8) satisfying, must

blow up at some finite. For sufficiently small, we have

. Then if is sufficiently close to, then can be continued at least to, and satisfies,. Then, even through can be continued to N, blows up at some finite point by the definition of M. This fact shows that such a does not belong to S, contradicting the definition of, again. Consequently case (b) occurs, and hence the proof of Theorem 6 is complete.

Proof of Theorem 7. The proof is done by contradiction. Let be a solution of Equation (1) of type (AS). We suppose that exists for and satisfies

(36)

Put . Then

Now, we employ the Young inequality of the form

(37)

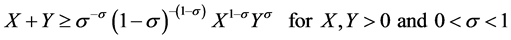
in the last inequality. It follows therefore that

where is a constant. We rewrite is inequality as

Noting (7.3) and condition (13), we obtain

where is a constant. Dividing both sides by and summing from n to

¥, we have

because. Consequently, we have

Letting, we get a contradiction to assumption (12). This completes the proof.

As was mentioned in Section 5, the proof of Theorem 8 is omitted. In fact, a more general result is proved in Lemma 5.

8. Proofs of Main Results for the Sub-Homogeneous Equations

Throughout this section, we assume that.

Proof of Theorem 9. Let be fixed so that and put

Then there are constants and satisfying

and

Consider the Banach space of all real sequences with sup norm. Define

the subset Y of by

and

We show that the hypothesis of the Schavder fixed point theorem is satisfied for Y and F. Let. Then, obviously for. Moreover

Hence. The continuity of F and the boundedness of the sets FY and can be easily established. Accordingly there is a satisfying. By taking difference twice, we find that is a solution of Equation (1) for and that for and. Now, we put

It is easy to see that is a solution of equation (8) for and is of type. The proof is complete.

Theorems 14 and 15 can be proved easily as in the proofs of Theorems 4 and 5 respectively. We therefore omit the proofs.

Proof of Theorem 10. By our assumption we can find a positive solution of Equation (8) sat-

isfying Since, we see by Lemma 4 that each exists for. We show that the

sequence has the limit function, and it gives rise to a positive solution of Equation (8) of type (D).

We first claim that

(38)

If this is not true, then for some and. This means however that there are two nonnegative nonincreasing solutions of Equation (8) passing through the point. This contradiction to

Theorem 17. We therefore have (38) and so exists observe that satisfies

Letting, we obtain via the dominated convergence theorem

We see that is a nonnegative solution of Equation (8) satisfying. It remains to prove that for n ≥ n₀ Fix N > n₀ arbitrarily. The proof of Theorem 2 implies that there is a solution for and for. We claim that

(39)

In fact, if this fails to hold, then

By this means, as before, that there are two nonnegative nonincreasing solution fo Equation (8) passing through the point. This contradiction shows that (39) holds. Hence by letting in (39) we have for. Since is arbitrary, we see that for. The proof is complete.

Proof of Theorem 11. The proof is done by contradiction. Let be a positive solution of equation (8) for of type (D). Using (16). We obtain from Equation (8)

(40)

where is a positive constant. We fix a arbitrary and consider inequality (42) only on the interval for a moment. A summation of (42) from n to 2N, given

From which, we have

(41)

We can find a constant satisfying

Therefore (43) implies that

from which we have

Letting, we have a contradiction. The proof is complete.

Proof of Theorem 12. The proof is done by contradiction. Let be a solution of Equation (8) of type (D). We notice first that

(42)

In fact, since, we can compute as follows

Therefore (42) holds.

We may suppose that for some and

(43)

But. Then

proceeding as in the proof of Theorem 8, we obtain

where is a constant. We obtain from (43) and assumption (18)

where is a constant. Dividing both sides by and summing from n to ¥, we have

that is,

Letting, we get a contradiction to assumption (17) by (42). The proof is complete.

Proof of Theorem 16. Sufficiency Part: By Theorem 17 and (2) of Remark 6.2, there is a positive solution of equation (8) satisfying. This is either of type (AL) or of type (AS). But by Theorem 15, we see that must be of type (AS).

Necessity Part: Let be a positive solution of Equation (8) for of type (AS). To prove (19), we

suppose the contrary that. As in the proof of Lemma 5.3, we have

where and let. It follows that

where is a constant. put. We then have

Since is of type (AS), is unbounded for and so is. Accordingly, there is a, satisfying for. Thus

Since, this implies the boundedness of w, which is a contraction. Hence, we must have (19). The proof is complete.

Theorem 16 is clear because of all solutions of equation (8) with exist for [see Lemma 5].

Cite this paper

Vadivel Sadhasivam,Pon Sundar,Annamalai Santhi, (2016) On the Asymptotic Behavior of Second Order Quasilinear Difference Equations7403287. Applied Mathematics,07,1612-1631. doi: 10.4236/am.2016.714139

References