Journal of High Energy Physics, Gravitation and Cosmology
Vol.02 No.01(2016), Article ID:63188,29 pages
10.4236/jhepgc.2016.21013
Does a Randall-Sundrum Brane World Effective Potential Influence Axion Walls Helping to Form a Cosmological Constant Affecting Inflation?
Andrew Walcott Beckwith
Chongqing University Huxi Campus, Chongqing, China

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 8 December 2015; accepted 26 January 2016; published 29 January 2016
ABSTRACT
In 2003, Guth posed the following question in a KITP seminar in UCSB. Namely “Even if there exist 101000 vacuum states produced by String theory, does inflation produce overwhelmingly one preferred type of vacuum states over the other possible types of vacuum states”? This document tries to answer how a preferred vacuum state could be produced, and by what sort of process. We construct a di quark condensate leading to a cosmological constant in line with known physical observations. We use a phase transition bridge from a tilted washboard potential to the chaotic inflationary model pioneered by Guth which is congruent with the slow roll criteria. This permits criteria for initiation of graviton production from a domain wall formed after a transition to a chaotic inflationary potential. It also permits investigation of if or not axion wall contributions to inflation are necessary. If we reject an explicit axion mass drop off to infinitesimal values at high temperatures, we may use the Bogomolnyi inequality to rescale and reset initial conditions for the chaotic inflationary potential. Then the Randall-Sundrum brane world effective potential delineates the end of the dominant role of di quarks, and the beginning of inflation. And perhaps answers Freeman Dysons contention that Graviton production is unlikely given present astrophysical constraints upon detector systems. We end this with a description in the last appendix entry, Appendix VI, as to why, given the emphasis upon di quarks, as to the usefulness of using times before Planck time interval as to modeling our physical system and its importance as to emergent field structures used for cosmological modeling.
Keywords:
Branes, Axion Walls, Bogomolnyi Inequality, Four- and Five-Dimensional Cosmological Constant

1. Introduction
It is well known through conventional calculations via QCD that there is a huge disconnection between what is calculated for a cosmological constant [1] , and the de facto observed phenomenological data which purports to support a cosmological constant far lower in magnitude than what is presumed to be an artifact of vacuum polarization [2] . This paper in part is an answer to this odd divergence and a summary of a different tack to giving a bound to the cosmological constant problem than one supplied by conventional applications of QCD. I contend that not only a difference would take on the cosmological constant yield dividends, but it would also yield an experimentally falsifiable model as to graviton production, as an answer to Freeman Dysons challenge to the astrophysics community to come up with detector schemes realistically capable of detecting spin two gravitons with our present level of detector technology.
As a general point, when we say S.H.O. I am referring to the acronym of simple harmonic oscillator. This paper is dedicated to physics way beyond the S.H.O. (simple harmonic oscillator) approximation and is dedicated to the premise that nonlinear contributions, which are not fully treated in the conventional literature are of decisive importance and this paper is a first attempt to include in effects which are often neglected for the same of convenience.
Note also that an acronym WKB is referred to as a semi-classical tunneling approximation in this paper. Semi-classical approximations abound all over physics, and what we are saying is that the construction we are emphasizing allows us to incorporate more quantum mechanical procedures in our treatment of cosmology. The reason for refinements of our protocol about tunneling, as in the initial phases of cosmology lies in our treatment of what is known about zero point fluctuations, which will be brought up next. We in looking at quantum tunneling, as in the WKB idea, and it is a way to form vacuum states, initially, in cosmology and also the concept of a vacuum energy density value, which will be the reason for Equation (1) in the paper below. More about the WKB approximation will be in the 2nd to last appendix entry of this document.
The vacuum state idea is brought up, in our document because a quantum state is said to be a vacuum state if the expectation value of the Hamiltonian in a given theory is a local minimum (the Hamiltonian of course being part of the data that defines the theory). We do not necessarily have a Hamiltonian for our modeling of cosmology, although both loop quantum gravity and Wheeler De Witt theory do refer to cosmological states, although incompletely. Needless to state, if we can define a Hamiltonian for cosmological conditions, not impossible to do, then we can go to the next idea, which is that of a vacuum energy density, which is one of the biggest problems in physics today. To a large degree, this document is an attempt to explain what are the consequences of extremely large theoretically calculated vacuum energy density values, and how to ameliorate the serious problems such calculations bring to the study of cosmology.
Zero point fluctuations of quantum fields contribute to an enormous vacuum energy density value 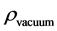 But the reality as seen in solar system based measurements and then in galactic astrophysics data sets have lead to a de facto observed [3]
But the reality as seen in solar system based measurements and then in galactic astrophysics data sets have lead to a de facto observed [3]
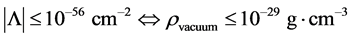 (1)
(1)
What we are doing is to give a physical model as to what could be an alternative mechanism physically to the vacuum fluctuation model used in QCD calculations to give a bound to the cosmological constant which avoids having a value roughly 10120 times too large [1] . In addition, this same value included in the cosmological constant model would help give an upper bound to graviton production in branes [4] which is a subject which will be done according to putting in the absolute magnitude of the cosmological constant in to a brane world bound to graviton production in a de Sitter, and ANTI de Sitter cosmological metric model [4] [5] .
Recent field theory calculations have lowered the overshoot factor to being of the order of 1043 times larger than what is inferred experimentally [6] . This is a significant improvement, but still beggars the question as to what is really needed to get the calculated results in line with known experimental data as referred to in Equation (1) above.
The model which in the end I turned to is similar in part to what was attempted by Weinberg et al. in the early 1980s [7] , with one tunneling event through a potential barrier. This was finally discarded, and what I am doing now is to in part re introduce part of the same idea but with a different scalar potential field than was attempted by Weinberg nearly two decades ago.
Dr. Abbot as of the mid 1980s attempted to lower a calculated cosmological constant value via application of a tilted Washboard potential for a scalar field [8] -[10] . I am using this method in part for just ONE tunneling event, plus a different constituent scalar field than used by either Weinberg or Abbot to try to get a more realistic value for the cosmological constant.
QCD is the theory of quantum chromodynamics, which is well understood. What we are doing is to try to make QCD as commensurate to quantum gravity, and quantum gravity via the idea of use of di quarks, which show up in this paper.
If as I suspect traditional QCD calculations have missed any essential details, it is in a di quark condensate as a template [11] for a nucleation of initial states contributing to inflation as viewed by Guth, et al. [12] in their papers. In addition, partly for reasons presented in this document, I also believe that the methodology I tried to refine from Abbot’s initial paper, as presented, is useful as a template for synthesis of gravitons. This is also due in part to Axion fields described as a “squeezer” to a di quark condensate [11] [13] .
We link an initial di quark configuration to instantons, spalererons, and the early universe. Since spalerons are not well understood, this will necessitate a discussion of spalerons and a thermal bath of temperature T [14] . It is also noteworthy that the bridge between the two potential systems mentioned in this document comes from increasing temperature removing a (1-cos {phi}) phase contribution to a tilted potential to what is an eventual typical Guth style quartic potential [15] . Disappearance of axion walls, or their equivalent would be in tandem with increase of temperature, leading to conditions at the start of start of inflation which would at that time, past the first instant of Planck time, match necessary and sufficient conditions as to slow roll potential systems used in early universe cosmology.
We argue all of this will set up conditions for describing in detail an effective cosmological constant contribution to brane world production of gravitons in the early universe. This will be done, assuming that the final input of a cosmological constant is significantly less than what the initial cosmological tilted well potential would give, effectively leading to a large burst of gravitons in an early universe.
Doing this though will necessitate an additional fifth dimension. Usually in mathematics, this is done via the circle group, denoted by T (or by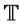 ), is the multiplicative group of all complex numbers with absolute value 1. The name comes from the fact that these numbers lie on the unit circle in the complex plane.
), is the multiplicative group of all complex numbers with absolute value 1. The name comes from the fact that these numbers lie on the unit circle in the complex plane.
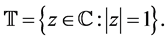 (1a)
(1a)
The circle group forms a subgroup of C×, the multiplicative group of all nonzero complex numbers. Since C× is Abelian, it follows that T is as well.
The notation T for the circle group stems from the fact that Tn (the direct product of T with itself n times) is geometrically an n-torus. The circle group is then a 1-torus. As electromagnetism can essentially be formulated as a gauge theory on a fiber bundle, the circle bundle, with gauge group U (1). Once this geometrical interpretation is understood, it is relatively straightforward to replace U (1) by a general Lie group. Such generalizations are often called Yang-Mills theories. If a distinction is drawn, then it is that Yang?Mills theories occur on a flat space-time, whereas Kaluza-Klein treats the more general case of curved space-time. The base space of Kaluza- Klein theory need not be four-dimensional space-time; it can be any (pseudo-) Riemannian manifold, or even a supersymmetric manifold or orbifold. All this will show up in our discussion of how to relate a Randall-Sundrum effective potential to a four dimensional potential system which incorporate axion walls which collapse as we reach an interval of Planck’s time 
This paper will finally conclude with a discussion of the relative merits of a space borne system as discussed by the DETF, like the JDEM project [3] [15] [16] , which would then not only help us identify early universe candidates for dark matter-dark energy, but also increase the chance of detecting gravitons. We make this final step by assuming that the dark matter-dark energy contribution is tied into the (1-cos {phi}) phase contribution to a tilted phase dependent potential spoken of, earlier, which blends into Guths typical inflationary potential for the reasons mentioned earlier on. Readers may peruse several condensed matter applications of false vacuum nucleation to condensed matter current calculations as to get a flavor of the starting point of the di quark nucleation [17] which will be used later on in this document [18] [19] . One of the key differences in the condensed matter approach from the cosmological one, is that the condensed matter approach explicitly uses the Bogomolnyi in equality as a topological charge vanishing in order to drop the contribution of a least action integral to primarily a quartic potential system [20] [21] , while the di quark equivalent system in the initial potential system explicitly appeals to an axion domain wall contribution acting as a squeezer, which is temperature dependent, and vanishing in mass as a given temperature radically increases. Aside from that the initial structure bears striking similarities, as being part of Sidney Coleman’s false vacuum nucleation [21] . Conceivably though, one could make a crude analogy with baryogenesis arguments though, with the contributions due to di quarks [12] largely vanishing. So the Bogomonyi inequality could be approximately cited, but this has yet to be conclusively proven [21] .
In any case, our work is taking a very physical interpretation of what was proved in “The embedding of the spacetime in five dimensions: an extension of Campbell-Magaard theorem” where the statement is made by F. Dahia et al. [22] that “We extend Campbell-Magaard embedding theorem by proving that any n-dimensional semi-Riemannian manifold can be locally embedded in an (n+1)-dimensional Einstein space. We work out some examples of application of the theorem and discuss its relevance in the context of modern higher-dimensional space-time theories”. Our analogy equivalent to embedding is to consider the relative magnitude of an effective potential derived from Randall Sundrum branes in a 5 dimensional setting, which is reduced by employing the circle group to being isomorphic with regards to a four dimensional tilted washboard potential of the sort just mentioned. Doing so if we reject an axion mass temperature dependence will lead, perhaps to a higher five dimensional analogue of the Bogomolnyi inequality [23] being used. This is a detail which we will expand upon later in this document.
2. Inflation, and the Conundrum It Creates for Dark Matter Searches
Beginning with Guth’s eternal inflation paradigm [12] , there are a few issues which need to be reviewed as to the importance of eternal inflation, and how it may be modified by observational data.
Let us now consider how eternal inflation is set up, and how variations in step size as concluded in this paradigm may affect a first order transition alluded to in this specific methodology first introduced by Guth in the 1980s [12] . As stated by Guth, in a recent talk at KITP, UC Santa Barbara [24] there are a few basic assumptions which can be considered as axioms as to the construction of inflation:
1) Quantum fluctuations are important on small scales, if and only if one is working with a static space time (i.e. no expanding universe)
2) For inflating space times, quantum fluctuations are “expanded” to be congruent in magnitude with classical sizes (classical fluctuations)
3) Simple random walk picture: In each time interval of , the average field
, the average field 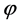 receives an increment with root means squared, of
receives an increment with root means squared, of . This increment is super imposed upon the classical motion, which is downward.
. This increment is super imposed upon the classical motion, which is downward.
4) Quantum fluctuations are equally likely to move field  “up or down” the well of a “harmonic” style potential.
“up or down” the well of a “harmonic” style potential.
Those who read the presentation should note the conclusion which is something which raises serious questions: i.e.
5) In equations, the probability of an upward fluctuation exceeds  if
if
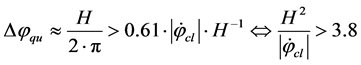 (1b)
(1b)
But
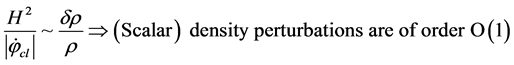 (2)
(2)
6) Guth closes his presentation with a statement to the effect that
“Even if there are 101000 vacuum states produced by String theory, then perhaps inflation produces overwhelmingly one preferred type of vacuum states over the other possible types of vacuum states”
The question then arises, How could this preferred type of vacuum state be produced, and by what sort of process?
Prior attempts to model the early stages of the universe by near ideal gas analogies for so called “super cooling” lead to thermodynamically oriented arguments which we will reproduce here [25] , and dissect with commentary as to what is to be expected in the first moments of creation.
To whit, from starting with the assumption that we are restricting ourselves to a non dark matter-dark energy regime of matter to be measured experimentally [13]
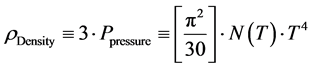 (3)
(3)
This is assuming that we are working with a degree of freedom for Fermionic and Bosonic matter contributions of the form [13] (neglecting dark matter and dark energy):
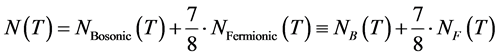 (4)
(4)
This lead to, assuming , and a critical temperature for electro weak transitions of
, and a critical temperature for electro weak transitions of

This would lead to

As well as a temperature dependent volume behaving as [13]

The radius of this “volume” is directly proportional to 

Note that the expressions so put up are highly dependent upon the degree of freedom parameter
This leads to the 2nd consideration of this document, which is how to put in explicit order calculations as to the order of the electroweak phase transition, which Trodden claims is crucial Trodden presents the following arguments [26] :
For continuous transitions, the associated departure from equilibrium is insufficient to lead to relevant baryon number production. For a first order transition, quantum tunneling occurs about
At a particular temperature below
The final, and important point Trodden makes is what happens in the immediate aftermath of baryogenesis, there is an alteration of a Higgs field, with the Higgs VEV changing from 


At this juncture, we wish to address how to incorporate a more accurate reading of phase evolution and the minimum requirements of phase evolution behavior in an evolving potential system which permits baryogenesis, and also incorporates dark energy production as well. This then answers the question raised earlier25, namely does “inflation produces overwhelmingly one preferred type of vacuum states over the other possible types of vacuum states” so one can have not only baryogenesis, but that we also have inflation as well.
To do this, we start off with an embedding in higher dimensions for answering in what context we may have baryogenesis, and when this baryogenesis ceases to be a dominant factor. In addition, we will also, as a side note, answer the question of graviton production in a brane structure arising in pre Planckian physics cosmology. This in particular is not too different from what Wald and others have argued [27] in, with the difference that we are assuming a potential structure for the regime of physics smaller than a Planck’s length
This investigation is attempting to show that the fifth dimension postulated by Randall-Sundrum theory helps give us an action integral which leads to a minimum physical potential we can use to good effect in determining initial conditions for the onset of inflation. The 5th dimension of the Randall-Sundrum brane world is of the genre [28] , for

This lead to an additional embedding structure for typical GR fields, assuming as one may write up a scalar potential “field” with 

This scalar field makes its way to an action integral structure which will be discussed later on, which Sundrum used to forming an effective potential. Our claim in this analysis can also be used as a way of either embedding a Bogomolyni inequality, perhaps up to five dimensions [22] , or a straight forward reduction in axion mass due to a rise in temperature [29] helped reduced effective potential in this structure, with the magnitude of the Sundrum potential forming an initial condition for the second potential of the following phase transition. Note that we are referring to a different form of the scalar potential, which we will call ϕ, which has the following dynamic [30] [31] . We explain the justification for a possible pre-Planck time, as given here, in the last appendix, Appendix VI, which is referenced in the conclusion with a long discussion. Suffice to say though that the pre Planck time condition is put in the document as to allow for changing mass of the graviton, which we will bring up in our Appendix VI entry. It is a last appendix entry, due to its contribution to the conclusion of our document.

The potentials



We should keep in mind that 


This in the context of the fluctuations having an upper bound of

Here,

3. Brief Sketch of Randall Sundrum Effective Potential and Its Links to Equation (13a) and Equation (13b)
The consequences of the fifth dimension mentioned in Equation (10) above show up in a simple warped compactification involving two branes, i.e. a Planck world brane, and an IR brane [28] . The IR brane can be thought of as similar to a Casmir physics plate model, parallel to the usual Universe brane, with a small separation between the two branes. This construction with the physics of this 5 dimensional system allow for solving the hierarchy problem of particle physics, and in addition permits us to investigate the following five dimensional action integral [28] .

This integral, will lead to the following equation to solve

Here, what is called 

This uses [33] (assuming 

This is for a compactification scale, for

We then obtain after a non trivial vacuum averaging [28]
Figure 1. Initial configuration of the domain wall nucleation potential used in Equation (6a) which we claim eventually becomes in sync with Equation (6b) due to the phase transition alluded to by Dr. Edward Kolbs model of how the initial degrees of freedom declined from over 100 to something approaching what we see today in given flat Euclidian space models of space time (i.e. the FRW metric used in standard cosmology).


This is leading to an initial formulation of [28]

Now, if one is looking at an addition of a 2nd scalar term of opposite sign, but of equal magnitude [29]

This is for when we set up an effective Randall-Sundrum potential looking like [29]

This above system has a meta stable vacuum for a given special value of 
4. How to Compare the Randall-Sundrum Effective Potential Minimum with an Effective Potential Minimum Involving the Potential of Equation (3) Above
We are forced to consider two possible routes to the collapse of a complex potential system to the chaotic inflationary model promoted by Guth [7] .
The first such model involves a simple reduction of the axion wall potential [13] as given by, especially when N = 1

The simplest way to deal with Equation (13) is to set, when Kolb [13] writes

i.e. to declare that the axion “mass” vanishes, and to let this drop off in value give a simple truncated version of chaotic inflationary potentials along the lines given by a transition from Equation (3a) to Equation (3b) We should note that 

has a bearing on this situation. Not to mention the problems inherent in several proposed fixes to the cosmological constant problem [34] .
Now if we want an equivalent explanation, which may involve baryogenesis, we need to look at the component behavior of each of the terms in Equation (23) without assuming

We then have to present a varying in magnitude value for the “scalar” 

There has been credible work with instantons in higher dimensions, starting with Hawking’s 1999 article [31] . This, however, addresses a way of linking an instanton structure with baryogenesis, dark energy, and issues of how Randall-Sundrum brane structure can be used to formation of initial conditions of inflationary cosmology.
Clarifying what can be done with an instanton style quantum nucleation in multiple dimensions [36] may help us with more acceptable models [6] [37] as to estimating, roughly, a quantum value for the cosmological constant, as an improvement in recent calculations. I refer interested readers to Appendix II on this matter, but for now will restrict this discussion to a qualitative derivation done for condensed matter currents for motivational purposes only. Start with a wave functional


where

This leads, if done correctly to the quadratic sort of potential contribution as given by [37] 
This is for his chaotic inflation model using his potential; I call the 2nd potential
Let us now view a toy problem involving use of a S-S’ pair which we may write as [17] - [19]

This is for a di quark pair along the lines given when looking at the first potential system, which is a take off upon Zhitinisky’s color super conductor model [11] .
5. Summarizing Different Approaches to Forming a Cosmological Constant
We should note what a common misconception as to the cosmological constant is. In dimensional terms we often see it referred to as a “natural” cosmological constant value in terms of Planck Energy values. This is similar to the problems one observes in a Quantum Field theoretic vacuum summation of zero point energy bosonic fields up to Planck energy values [3] .

V.G. Gurzadyan, and She-Sheng Lue wrote a world scientific paper [30] giving a derivation to the effect that one can calculate a realistic value for the cosmological constant based upon a wave number based upon a vacuum fluctuation model which gives a Fourier style de composition of vacuum fluctuation wave modes such that if we assume no angular momentum “twisting” and a flat FRW metric

Needless to say though, that any energy density so accumulated would be far, far less than what was assumed in the typical bosonic field calculation, above, especially since if 

This equation would get dramatically smaller for increasing age of the universe to present conditions, with the initial values of it to be similar in “form” to the enormous values of initial energy density outlined above for an initial nucleating universe, especially if we model the initial energy as proportional to the square of Equation (30c) above. This, however, has a serious defect in that it does not give a genesis, or origins reference as to how the cosmological constant could evolve from initial big bang conditions. Mainly due to it being extremely difficult to form 

Part of the misconception which I think is endemic in this field with respect to forming a cosmological constant which is consistent with known astrophysics observations lies in the difficulty of forming of an effective Field theoretic Hamiltonian for calculation of vacuum energy, i.e. for quasi particles making sense of

G.E. Volovick writes a candidate for an acceptable Hamiltonian in this above equation as having a chemical potential addition [3] , i.e.

This assumes that one can actually define a number operator for quasi particles, i.e.

Again, for early universe conditions, how does one form 

6. Abbot’s 1985 Attempt to Obtain Low Values for the Cosmological Constant
As of the mid 1980s, Abbot [8] initiated using a tilted washboard potential model for 



As Abbot admitted though, this model, while giving certain qualitatively attractive features involved an unacceptably long period of final tunneling time based upon [8]

with

This idea assumes as Abbot postulated a cascading series of minimum values of 

Equation (4) lead to a cascading series of local minimum values, where Abbot scaled the local minimum values via setting his scalar field as

This assumes 

Typical values for the constants above were
This lead to, for final values of tunneling time of the order of 



We looked at Ariel Zhitnitsky’s formulation of how to form a condensate of a stable instanton style configuration of cold dark matter as a starting point for how an axion field can initiate forming a so called QCD ball [11] The QCD ball forms instanton configurations which we use to help compute an upper bound to the cosmological constant which we claim is pertinent to the release of gravitons from a brane [28] .
Note that in classical GR, potential diagrams have proved useful in analyzing orbits of particles and photons in Schwartzschild geometry. Here, we use the di quark model so formed here as to add in a physically realistic upper bound to forming a cosmological constant input into analyzing when gravitons could be released from an early universe brane. This is similar in part to the use of potential diagrams in investigating Lorentz violations in 





The important thing to keep in mind, as brought up in a review of an earlier proposal made on this topic is that the di-quark potential satisfies the slow roll condition for inflation. Does one want to have inflation at 100 MeV? This is not impossible but difficult to achieve and it is necessary for a presenter of any such di quark model how to get the right amplitude of fluctuations [30] . The answer in part to this issue is that scalar field pertubations lead to a fluctuation of the scalar field at the end of inflation which is heavily dependent upon the initial value of the potential at the start of di quark nucleation of the scalar field itself. This will be elaborated upon in the conclusion of this document and will constitute part of a follow up of the ideas of this document for future research inquiry. This happens in spite of a transition from a tilted washboard potential to a potential materially similar to Guths quadratic chaotic inflationary potential [7] .
We also claim that the process of forming instantons as spoken of in the formation of di quark pairs in itself is useful for the later formation of cold dark matter in the form of QCD (Quantum Chromodynamics) balls, as is elucidated upon later in this document. In addition is the datum that the main part of the potential for forming the single tunneling washboard potential used in the formation of di quark pairs is extremely similar to a cosmic axion potential which is known to have a temperature dependence. This axion potential, as is stated in one of the appendix entries disappears as temperature increases, leading to the more typical Guth chaotic inflationary potential [7] which is then used as a template to fine tune the fluctuation of scalar potential values toward the end of the cosmic inflationary period [7] . We assert that this period of transformation would lead to extensive graviton production in an early universe, as well as dark matter production in a way which is observable via the proposed JDEM satellite system.
7. Introduction of Spalerons for the Early Universe as a Generalization of Cosmological Instantons for a Re Do of Abbots Procedure
As referred to in Mukhanov’s book on foundations of cosmology, spalerons are a way to introduce motion of a “quasi particle” in a Euclidian metric via use of Wick rotations 


This assumes

Next

This assumes

When we have the energy of the system close to Equation (35bi), we are in the realm of a first order approximation of escape probability of constituents of a scalar field 

This assumes that the energy (mass) of a sphaleron is defined via

The exact particulars of forming an appropriate instaton 

8. Zhnitinisky’s QCD Balls, and Their Contribution to Cosmological Instantons
We looked at Zhitnitsky’s formulation of how to form a baryon condensate to initiate forming a so called QCD ball [11] . This QCD ball could in fact be the template for the initial expansion of a scalar field leading to false vacuum inflationary dynamics in the expansion of the universe. Zhitnitsky’s formulation uses quarks in a non-hadronic state of matter, but which in the beginning can be in di quark pairs. In doing so, Zhitnitsky’s calculations for quarks being squeezed by a so called QCD phase transition due to the violent collapse of an axion domain wall should be used. The axion domain wall would be the squeezer to obtain a soliton (anti soliton) configuration. This pre supposes that we are using a multiple dimensional generalization of the baryon number contribution

And, Zhitnitsky [11] further gives a criteria for absolute stability by writing a region of stability for the QCD balls dependent upon the inequality occurring for 

He furthermore states that stability, albeit not absolute stability is still guaranteed with

This is done in such a manner as to use Figure 1 as a condensed matter physics template which is analogous to the instanton behavior of di quark pairs. Furthermore I also claim that it gives a reason for the inclusion of axion physics as seen in the first part of Equation (6a) with respect to the axion walls alluded to in Appendix II below. We should note though that Kolb’s “The Early Universe” book [13] also gives a temperature dependence of axions which is of the form of

It is important to note that Abbots potential [8] as given above has multiple cascading false vacuum minimum. We are assuming just one minimum, with a final value as given for the first potential system above. Also, if the initial tunneling system is started off with the value of the exponent of Equation (32)

Then I claim that the tunneling problem Abbot spoke of can be scaled out as to obtain tunneling times not incongruent with respect to a reasonable age of the universe.
We should note that 
Why in this model did I work with a 2nd potential system as well? The reason is because that the first potential system in its emergent fashion is congruent only with the first slow roll condition assumed as necessary for inflation, i.e. if we look at

We find that 


However, if we work with 
9. Estimation of Tunneling Time for New Potential System Given in Equation (13a) and Equation (13b)
An easy, straightforward manner to calculate tunneling time in the case of a false vacuum is to use a WKB type bounce calculation for forming an energy based tunneling [31]

We need now to do this for a potential system given in part by Equation (5) above, and to do it consistently. Assuming that 

Here, 

If one defines the minimum of the potential as being due to the 1st tilted washboard potential 

If


This explicitly assumes that one is using the tilted washboard potential of Equation (13a)
10. Necessary Conditions for Axion Wall Potential Contribution to Equation (13a) Disappearing
What suffices to initiate chaotic inflation? Note that a potential

Involves a change of scale factor of the form [14]

What is involved is a reduction of the degrees of freedom of the initial physical system which permitted the di quark condensate to form in the first place. This would cause the physical system to shift from the 1st to the final Guth chaotic potential [8] form alluded to above which is involving a drop off of higher order terms as seen in Equation (6b) above. Equation (10) if representative of an axion wall contribution to our problem would cease being a factor and largely disappear with increasing temperature, which would be part of the nucleation process. As the mass of Equation (40) largely disappeared, this would be in tandem with higher powers of n disappearing, leading to

This would be in tandem with [14]

11. Using Our Bound to the Cosmological Constant to Obtain a Conditional Escape of Gravitons from an Early Universe Brane
In forming this problem, we offer an initial review of the basic physics we are assuming leads up to this problem. The physics shown in Equation (13a) to Equation (13b) above, corresponds to a phase transformation from 

Our discussion in part is a brief introduction to the. brane structure built by Leach, and Lesame in their article about escape of gravitons from a brane [46] . It is noticeable that their contribution makes use of an effective potential for gravitons which includes cases where the brane would conceivably move at the same speed as escaping gravitons, and the more desirable case that gravitons moved faster than the brane itself, and escaped to propagate through inter galactic space in the way we see today. Our use of di quarks would be for an emergent structure filling in the bridge between the 1st and 2nd potentials of Equation (5) above, and our analysis of the eventual bound to the cosmological constant is commensurate with an early universe producing most of gravitons we would expect in our present universe. Our candidate for graviton production would be when we are producing cold dark matter in the form of QCD balls as written up by Zhitinisky in his model of QCD color super conductors as a cold dark matter candidate [11] . We also believe that we are tying in this initial period of graviton production with cold dark matter, and that further analysis will lead to refining how to effectively search for gravitons. i.e. the place to start would be in early universe objects, perhaps like quasars.
To begin we present using the paper written by Leach et al. on conditions from a FRW brane [28] in early universe cosmology, assuming that the cosmological constant input is formed as a result of the immediate consequences of the phase transition indicated in Equation (13) above. This also assumes that what we call the cosmological constant was likely initially greater than the final value given for inflationary potentials of the 2nd type given in Equation (13b).
We are assuming explicitly that we can use a Friedmann-Lemaître-Robertson-Walker brane world at or near the end of inflation, and that it is embedded in a structure similar to a 5-dimensional anti-de Sitter Schwartzshield bulk [28] . Why are we doing this? Frankly to utilize the effective graviton potential argument built up by J. Leach et al. as to how a cosmological constant plays into would be release of gravitons from a brane. I believe this criteria is unique, and deserves further analysis.
We shall briefly summarize their findings and put in our work on di quark contributions to an upper bound to the cosmological constant as an enabler of graviton production from this brane structure. A further unstated assumption is that the gravitons were likely released in a brief instant after di quark nucleation. This will be discussed in lieu of the presumed experimental difficulties as to graviton detection via conventional detectors, with recommendations as to how to find gravitons experimentally if they are as I suspect primarily released in early universe inflationary expansion.
Leach et al. obtain a condition for a gravitational signal to be going through a FRW brane via use of an effective potential of a graviton, which is defined via a potential impact parameter [46]

Also there exists an “impact parameter”

This leads to, practically, a condition of “accessibility” via [28]

Note, that in this definition, R so defined is with respect to “bulk dimensions” and from considerations of a five dimensional anti de Sitter Schwartzshield metric

Here, k = 0 for flat space, k = −1 for hyperbolic three space, and k = 1 for a three sphere, while we also set an anti-de Sitter radius of curvature [28]

This assumes a negative bulk cosmological constant 


Then we have a maximum effective potential of gravitons defined via

This leads to a bound with respect to release of a graviton from an anti De Sitter brane as defined by Leach et al. as

How do we link this to our problem with respect to di quark contributions to a cosmological constant? Here I make several claims.
Claim 1.
It is possible to re define 

Here, I am defining 


Claim 2.


Claim 3.
Equation (5) has a 1st potential which tends to be for a di quark nucleation procedure which just before a defined Planck’s time 


With furthermore

So then that there would be a great release of gravitons at or about time
Claim 4.
Few gravitons would be produced significantly after time
Before we reach our conclusion, we should note that negative bulk cosmological constant used in brane world with the sort of metric referred to above assumes that there exist positive and negative tensions 



Still though this assumes as in Randall-Sundrum brane-worlds that the negative tension brane world has the standard model fields in the negative tension brane [5] . Also that one is able to use a finite distance between the branes to get a discrete KK spectrum, and that a distance between the branes is fixed, to recover standard GR four dimensional space time for low energy systems, which are assumed to be dominant after di quark nucleation.
12. Connection to Investigating a Tie in of a Brane World Least Action Integral with a Di Quark Least Action Integral
Now for the question the paper is raising, Can we realistically state the following for initial conditions of a nucleating universe? If so, then what are the consequences?

The right hand side of Equation (20) can be stated as having

We can insist that this 

So, this leads to the following question. Does a reduction of axion wall mass for the first potential system given in Equation (13a) being transformed to Equation(13b) above give us consistent physics, due to temperature dependence in axion “mass”, or should we instead look at what can be done with S-S’ (soliton-anti soliton) instanton physics and the Bogolmyi inequality [22] , in order to perhaps take into account Baryogenesis? Also, can this shed light upon the Wheeler De Witt equations [13] [33] modification by Ashtekar [47] in early universe quantum bounce conditions?
Finally, does this process of baryogenesis, if it occurs lend then to the regime where there is a bridge between classical applications of the Wheeler De Witt equation to the quantum bounce condition raised by Ashtekar [24] ?
13. Tie in with Di Quark Potential Systems, and the Classical Wheeler De-Witt Equation
Ashtekar’s quantum bounce [47] gives a discretized version of the Wheeler De Witt equation. Let us first review classical De Witt theory which incidentally ties in with inflationary n = 2 scalar potential field cosmology. This will be useful in analyzing consequences of the wave functional so formed in Equation (18) and suggest quantum bounce analogies we will comment upon later.
In the common versions of Wheeler De Witt theory a potential system using a scale radius


Here we have that if 

As well as

Now, Henriques [32] presents a way in which one can obtain a Wheeler De Witt equation based upon

Using a momentum operator as give by

This is assuming a real scalar field 



As well as an energy term


This is for a “cosmic” Schrodinger equation as given by
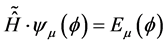
This has

And

Here 



Now Ashtekar in his longer arXIV article [47] make reference to a revision of this momentum operation along the lines of basis vectors 

With the advent of this re definition of momentum we are seeing what Ashtekar works with as a sympletic structure with a revision of the differential equation assumed in Wheeler-De Witt theory to a form characterized by [47]



Also, and more importantly this 

This is for a crucial “momentum” value

and

Which leads to, for an initial point in “trajectory space” given by the following relation 
So that if we consider eignfunctions of the De Witt (difference) operator, as contributing toward

With each 

This equation above has a “symmetry” as seen in Figure 1 of Ashtekar’s PRL article [47] about
14. Conclusion
Our model is in part a response to an assertion by Dr. Steinbeck about the feasibility of information exchange in a cyclic universe model. As stated in Appendix III, [24] , there is for standard spherically symmetric metrics no prior information exchanged between prior and the present day universe expansion and contraction cycles. What we have formulated so far in this paper is a start toward analyzing initial conditions permitting a cosmological constant without the huge 10120 magnitude overshoot of current QCD vacuum state values which are reported all over the literature without using his cyclic universe model. QCD balls [11] are a way to introduce a dark matter candidate in directly for formulating this problem and do permit, up to a point adherence to the false vacuum state to a true vacuum value, as postulated by Coleman [20] .
Furthermore, we also argue that the semi classical analysis of the initial potential system as given by Equation (6) above and its subsequent collapse is de facto evidence for a phase transition to conditions allowing for cold dark energy to be created at the beginning of inflationary cosmology. And that this production of dark energy would be at the same time as a surge in early universe graviton production this is done with Zhitinisky’s QCD ball condensate construction [11] as a multiple dimensional generalization of instanton physics.
Any further work in analyzing this problem will require reconciling the modification of Abbot’s hypothesis stated above with what is manifestly wrong in the ideas presented in standard quantum chromodynamics references [1] and furthermore it permits reformulating standard initial conditions for Wheeler-De Witt equation [24] which may permit time dependent effects in the beginning of cosmic nucleation of inflationary universe initial states.
Let us now briefly review what we can say now about standard graviton detection schemes. As mentioned earlier, Rothman states that the Dyson seriously doubts we will be able to detect gravitons via present detector technology [4] . The conundrum is as follows, namely if one defines the criterion for observing a graviton as

Here,

This has 
ton source, to a detector. Furthermore, 




My entire point is that Dysons conjecture will be true for most candidates for graviton production, but that if we can define early universe graviton production more succinctly via a refinement of the above presented arguments that in due time, most likely by space detection systems looking for dark energy, that we may in fact be able to identify gravitons from an early universe environment. In addition, how the tunneling occurs should be investigated further via a refinement of the bounce solutions and vacuum tunneling procedures used by J. C. Hackworth and E.J. Weinberg in October 2004 [44] .
We can point out via a heuristic argument a linkage between the di quark scalar field and fluctuations of a scalar field toward the end of the inflationary era which we do believe will support a proposal for refining this inquiry as to getting appropriate data sets from planned JDEM space borne instrument platforms. As pointed out by Zhitinisky [11] , in 2002, di quark condensates are a good candidate for the formation of cold dark matter. We can relate amplitude fluctuations from the formation of di quark scalar fields to later fluctuations via the following procedure.
Let us state for the beginning of the inflationary era, where the di quark pairs were already synthesized an amplitude for admissible fluctuations to be written as reaching a peak value when

This assumes that 



This assumes we define

This is assuming k so defined is given by Equation (69) above, while

This last equation represents the value of the Hubble parameter at the end of the inflationary epoch, with
This leads to fluctuations for a quadratic potential as assumed by Guth

As Mukhanov [14] states, which is crucial, if the initial scale of the scalar potential 


could be detected in the 
beginning of the manuscript, a base line requirement as to the development of realistic inflationary models, tying into the amplitude of the initial inflationary model is that for inflation to be on going, due to a di quark nucleation at the beginning, with a mass m defined in part by Equation (32)

All this needs to be vetted and confirmed by space borne observations.
A final consideration to be investigated, for the sake of how we define scale factors lies in an issue that Ellis raised recently in “On Horizons and the Cosmic Landscape” [43] . His point was about the problems of vetting the multi universe hypothesis put in by Susskind. His most cogent point was in defining the existence of an event horizon (assuming that we could consider an initial universe construction as analogous to a black hole) at an arbitrary time 

or

If as I assert that we can not realistically define 
While checking Dr. Corda’s supposition [37] , we will need to use the techniques and instruments as cited in [38] as to the most efficient way to perform appropriate data analysis, so as to investigate if we can experimentally confirm the relevance of General relativity to early universe conditions, Dr. Corda’s choice of either scalar tensor gravity or traditional general relativity as the causative agent for instrumental data will be have to be investigated via the instrumentation as written up in [38] , and care must be taken to avoid the Bicep 2 disaster, which was due to dust from galaxies, which effectively generated multiple sources. We want a single causative agent as to relic gravitational waves, for our data.
Acknowledgements
This work is supported in part by National Nature Science Foundation of China grant No. 11375279.
In addition: the author wishes to thank Dr. Steinhardt for his elucidation of the importance of the Abbot model in the UCLA 2006 dark matter convention. Previously, the author had no awareness of this work, or of its deep significance as to parameterization of a realistic value to the cosmological constant. In addition, several researchers at the Kalvi institute Eastern gravity conference in March 2006, noticeably Dr. Thorsten Battefeld stated that much of what I presented was a re hash of Guth and Weinberg’s inflationary models, which forced me to enunciate graviton production and detection as crucially important. Finally, reviewers in South Africa pointed out the necessity of linking inflationary magnitude to a given specific upper bound which I claim in part is partly answered in the conclusion leading up to Equation (34) above.
In addition, Dr. Xi Yang is thanked for motivating me to continue physics, in spite of every hardship put my way.
Cite this paper
Andrew WalcottBeckwith, (2016) Does a Randall-Sundrum Brane World Effective Potential Influence Axion Walls Helping to Form a Cosmological Constant Affecting Inflation?. Journal of High Energy Physics, Gravitation and Cosmology,02,125-153. doi: 10.4236/jhepgc.2016.21013
References
- 1. Brown, L.S. (1992) Quantum Field Theory. Cambridge University Press, Cambridge. http://dx.doi.org/10.1017/CBO9780511622649
- 2. Carrol, S., Press, W.H. and Turner, E.L. (1992) The Cosmological Constant. Annual Review of Astronomy and Astrophysics, 30, 499-542. http://dx.doi.org/10.1146/annurev.aa.30.090192.002435
- 3. Volovik, G. (2006) Vacuum Energies: Myths and Reality. International Journal of Modern Physics D, 15, 1987-2010. http://dx.doi.org/10.1142/S0218271806009431
- 4. Rothman, T. and Boughn, S. (2006) Can Gravitons Be Detected? Foundations of Physics, 36, 1801-1825. http://dx.doi.org/10.1007/s10701-006-9081-9
- 5. Maartens, R. (2005) Brane-World Cosmology. In: Papantonopoulos, E., Ed., The Physics of the Early Universe, Lecture Notes in Physics, Vol. 653, Springer Verlag, Berlin, 213-249.
- 6. In the D4-D5-E6-E7-E8 VoDou Physics Model, Gravity and the Cosmological Constant Come from the MacDowell-Mansouri Mechanism and the 15-dimensional Spin(2,4) = SU(2,2) Conformal Group.
- 7. Guth, A.H. and Weinberg, E. (1983) Could the Universe Have Recovered from a Slow First-Order Phase Transition? Nuclear Physics B, 212, 321-364. http://dx.doi.org/10.1016/0550-3213(83)90307-3
- 8. Abbot, L. (1985) A Mechanism for Reducing the Value of the Cosmological Constant. Physics Letters B, 150, 427-430. http://dx.doi.org/10.1016/0370-2693(85)90459-9
- 9. Private Communications with Dr. Steinhardt at the UCLA Dark Matter Conference, 2006.
- 10. Presentation Given by Dr. Steinhardt at the UCLA Dark Matter Conference.
- 11. Zhitinisky, A.R. (2002) Dark Matter as Dense Color Superconductor. Nuclear Physics B—Proceedings Supplements, 124, 99-102. http://dx.doi.org/10.1016/S0920-5632(03)02087-5
- 12. Guth, A. (1981) Inflationary Universe: A Possible Solution to the Horizon and Flatness Problems. Physical Review D, 23, 347-356. http://dx.doi.org/10.1103/PhysRevD.23.347
- 13. Kolb, E.W. and Turner, M.S. (1990) The Early Universe. Westview Press, Boulder.
- 14. Mukhanov, (2005) Physical Foundations of Cosmology. Cambridge University Press, Cambridge.
- 15. Beckwith, A.W. (2006) “How S-S” Di Quark Pairs Signify an Einstein Constant Dominated Cosmology, and Lead to New Inflationary Cosmology Physics. Electronic Journal of Theoretical Physics, 9, 7-33.
- 16. Kadota, K., Dodelson, S., Hu, W. and Steward, E.D. (2005) Precision of Inflaton Potential Reconstruction from CMB Using the General Slow-Roll Approximation. arXIV:astroph/0505185 v1 9 May 2005
- 17. Beckwith, A.W. (2006) A New Soliton-Anti Soliton Pair Creation Rate Expression Improving upon Zener Curve Fitting for I-E Plots. International Journal of Modern Physics B, 20, 849-861.
- 18. Beckwith, A.W. (2005) Making an Analogy between a Multi-Chain Interaction in Charge Density Wave Transport and the Use of Wave Functionals to Form S-S’ Pairs. International Journal of Modern Physics B, 19, 3683-3703. http://dx.doi.org/10.1142/S0217979205032358
- 19. Beckwith, A.W. (2006) An Open Question: Are Topological Arguments Helpful in Setting Initial Conditions for Transport Problems in Condensed Matter Physics? Modern Physics Letters B, 29, 233-243. http://dx.doi.org/10.1142/s0217984906010585
- 20. Coleman, S. (1977) The Fate of the False Vacuum. Physical Review D, 15, 2929-2936. http://dx.doi.org/10.1103/PhysRevD.15.2929
- 21. Zee, A. (2003) Quantum Field Theory in a Nutshell. Princeton University Press, Princeton, 279-280.
- 22. Dahia, F. and Romero, C. (2002) The Embedding of the Space-Time in Five Dimensions: An Extension of Campbell-Magaard Theorem. Journal of Mathematical Physics, 43, 5804-5814. http://dx.doi.org/10.1063/1.1507824
- 23. Lee, J. and Sorkin, R.D. (1988) Derivation of a Bogomolnyi Inequality in Fivedimensional Kaluza-Klein Theory. Communications in Mathematical Physics, 116, 353-364. http://dx.doi.org/10.1007/BF01229199
- 24. http://online.itp.ucsb.edu/online/strings_c03/guth/pdf/KITPGuth_2up.pdf
- 25. Banerjee, B. and Gavai, R.V. (1993) Supercooling and Nucleation in Phase Transitions of the Early Universe. Modern Physics Letters A, 11, 615-622. http://dx.doi.org/10.1142/S0217732396000631
- 26. Trodden, M. (1999) The Cosmology-Particle Physics Connection. arXiv:hep-ph/0605284.
- 27. Hollands, S. and Wald, R.M. (2002) Comment on Inflation and Alternative Cosmology. arXiv:hep-th/0210001.
- 28. Sundrum, R. (2005) In Three Lectures Given in SSI, 2005: The First One Being: Extra Dimensions, by R. Sundrum, Monday, 1 August 2005, 10:30 a.m.-11:30 a.m., The Second One Being: Extra Dimensions, Ctd, by R. Sundrum, Tuesday, 2 August 2005, 10:30 a.m.-11:30 a.m. And the Final One Being Extra Dimensions, Ctd, by R. Sundrum, Wednesday, 3 August 2005, 10:30 a.m.-11:30 a.m.
- 29. Beckwith, A.W. (2006) How One Can Use Di Quark Scalar Fields Permitting a Cosmological Constant Permitting Gravitons Escaping from Early Universe Branes. 36th COSPAR Scientific Assembly, Beijing, 16-23 July 2006.
- 30. Gurzadyan, G. and Xue, S.-S. (2003) On the Estimation of the Current Value of the Cosmological Constant. Modern Physics Letters A, 18, 561-568. http://dx.doi.org/10.1142/S0217732303008405
- 31. Hawkings, S.W. and Real, H.S. (1999) Inflation, Singular Instantons and Eleven Dimensional Cosmology. Physical Review D, 59, Article ID: 023502. http://dx.doi.org/10.1103/PhysRevD.59.023502
- 32. Henriques, A.B. (2006) Loop Quantum Cosmology and the Wheeler-De Witt Equation. General Relativity and Gravitation, 38, 1645-1659. http://dx.doi.org/10.1007/s10714-006-0330-1
- 33. Dalarsson, M. and Dalarsson, N. (2005) Tensors, Relativity, and Cosmology. Elsevier Press, Amsterdam.
- 34. Griffiths, D. (2004) Introduction to Quantum Mechanics. 2nd Edition, Prentice Hall, Princeton.
- 35. http://hitoshi.berkeley.edu/221a/WKB.pdf
- 36. Beckwith, A. (2016) Gedanken Experiment for Fluctuation of Mass of a Graviton, Based on the Trace of GR Stress Energy Tensor-Pre Planckian Conditions That Lead to Gaining of Graviton Mass, and Planckian Conditions That Lead to Graviton Mass Shrinking to 10-62 Grams. Journal of High Energy Physics, Gravitation and Cosmology, 2, 19-24. http://dx.doi.org/10.4236/jhepgc.2016.21002
- 37. Corda, C. (2009) Interferometric Detection of Gravitational Waves: The Definitive Test for General Relativity. International Journal of Modern Physics D, 18, 2275-2282. http://arxiv.org/abs/0905.2502 http://dx.doi.org/10.1142/s0218271809015904
- 38. Van Den Broeck, C., et al. (2015) Gravitational Wave Searches with Advanced LIGO and Advanced Virgo. http://arxiv.org/pdf/1505.04621v1.pdf
- 39. Cowen, R. (2015) Gravitational Waves Discovery Now Officially Dead: Combined Data from South Pole Experiment BICEP2 and Planck Probe Point to Galactic Dust as Confounding Signal. http://www.nature.com/news/gravitational-waves-discovery-now-officially-dead-1.16830
- 40. Cowen, R. (2014) Full-Galaxy Dust Map Muddles Search for Gravitational Waves. http://www.nature.com/news/full-galaxy-dust-map-muddles-search-for-gravitational-waves-1.15975
- 41. Beckwith, A. (2016) Non Linear Electrodynamics Contributing to a Minimum Vacuum Energy (“Cosmological Constant”) Allowed in Early Universe Cosmology. Journal of High Energy Physics, Gravitation and Cosmology, 2, 25-32. http://dx.doi.org/10.4236/jhepgc.2016.21003
- 42. Beckwith, A. (2016) Gedanken Experiment for Degree of Flatness, or Lack of, in Early Universe Conditions. Journal of High Energy Physics, Gravitation and Cosmology, 2, 57-65. http://dx.doi.org/10.4236/jhepgc.2016.21006
- 43. Ellis, G. (2006) Comment—On Horizons and the Cosmic Landscape. arXIV astro-ph/0603266 v 2 15 March, 2006. http://120.52.73.79/arxiv.org/pdf/astro-ph/0603266.pdf
- 44. Hackworth, C. and Weinberg, E.J. (2004) Oscillating Bounce Solutions and Vacuum Tunneling in de Sitter Spacetime. Physical Review D, 71, Article ID: 044014.
- 45. Dyson, F. (2012) Is a Graviton Detectable? http://publications.ias.edu/sites/default/files/poincare2012.pdf
- 46. Leach, J. and Lesame, W. (2005) Conditional Escape of Gravitons from the Brane. arXIV gr-qc/0502109 v3 26 Apr 2005. http://arxiv.org/abs/gr-qc/0502109v4
- 47. Ashtekar, A., Pawlowiski, T. and Singh, P. (2006) Quantum Nature of the Big Bang: An Analytical and Numerical Investigation. arXIV gr-qc/0604013. http://arxiv.org/abs/gr-qc/0604013
- 48. Thieumann, T. (1998) QSD V : Quantum Gravity as the Natural Regulator of Matter Quantum Field Theories. Classical and Quantum Gravity, 15, 1281-1314. http://arxiv.org/abs/gr-qc/9705019
Appendix I
How we show phase evolves in both Equation (13a) and Equation (13b) above
Appendix I A
First, the physics of how phase evolves due to potential Equation (13b) leading to chaotic inflation.
We shall make a plausibility argument linking the two potentials mentioned above in Equation 13a and Equation 13b. This will be using a slow roll approximation which is de rigor in inflationary cosmology, namely using 

To start off, we will be using a nearly constant Hubble value of H in an early universe nucleation scheme. The reason for doing this will be considering

This will be done in spite of the well known relationship of

Furthermore, as a very rough approximation, we will be setting 

Let us now review how a di quark phase 


Then, what I write as Equation (1) may be viewed as

From now on, we will write

If so we can write Equation (1d) above as

We re write this Equation (1f) as

This leads to a general equation solution of

This leads to an equation for 

Next, let us view the particular solution as given by

So, then we can look at the general situation created by the chaotic potential given by Equation (1b), as given by

This assumes we may write 

This in the very small time limit is similar to what Guth wrote up in the 1980s.
Appendix I B
When we look at Equation (6a) of the main text
Next, let us review the far more complicated situation arising with the tilted washboard potential given in Equation (13a) we will write up as, with

This has a derivative value of the form

Which we write as

Let us first look at the first to third order contributions to this potential, and then get a slow roll equation for evolution of

This has a general solution equation we may write up as

If we pick an exponential value for the general phase, and solve for

Implies, if we set the time unit as

This would lead for very small time intervals

This is almost always negative, since it would require the following for it to be positive, namely
If so then for the re do of the washboard potential mentioned, we have an exponentially growing

A particular solution for this phase for the washboard potential would lead to the cubic equation of the form

In general this leads to the di quark phase 
This allows us to form a bridge between Equation (1b) and Equation (2). This is incidentally in line with the following graphs given in Dr. Kolb’s book, namely Figure 8.1 which gives a schematic illustration of an inflationary potential. In addition, Figure 8.7 gives the non barrier version of Figure 8.1, whereas we assume we do have a barrier for tunneling.
Appendix II
Kolb’s book [13] has a discussion of an Axion potential given in his Equation (10.27)

Here, he has the mass of the Axion potential as given by 

Appendix III
As given by Mirjana Dalarsson and Nils Dalarsson [33] , we can cite wave function of the universe arguments in order to give a base line as to what constitutes a Plancks length and Planck’s time for the initial starting point of inflationary expansion. The radial turning point is usually cited as

With a corresponding time length of

This however is due to a wave functional argument for the universe which is time independent and depends only on the space time geometry. We do not have a way to include in an evolutionary history of states of matter contributing to these order of magnitude estimates other than a probalistic argument about space time geometry and the matter field content.
The di quark hypothesis, is intended to be a start to giving more definition to the Wheeler-De Whitt equation, for a wave function of the universe 

This is where z is a dimensionless scale variable and

And

The enormous initial energy densities given in forming the bounce radius effectively preclude information exchange in prior universe cycles with our own, at least from the stand point of entropy of information at such high energy values assumed in the formation of our present universe.
Appendix IV. Brief Statement as to the WKB Approximation
As given by Griffiths [34] we have the following for the WKB approximation, namely that if we are considering a second-order homogeneous linear differential equation that the procedure as given in [35] actually holds, namely as given by Equation (17) of reference [40] which we give as, if E is equal to the Hamiltonian, with

This is the solution to what is actually a Hamilton-Jacobi equation. The solution given above, with no requirement that E be the energy operator of the Schrodinger equation is inherently semi classical. And an approximation used in tunneling problems.
Appendix V. Working with Astrophysical Conundrums
We take the following discussion as quoted directly from [41] namely
Quote: from [41] we ask that the readers read this directly from the text, and to fill it in, from there. This section 4, from [41] , completely fills in the essential background. [39] is also quoted in the below copy from reference [42] . Keep in mind that the references, as given in the below quote are from [42] and that the reader is urged to this section from [41] directly. Here it is.
Re-Examining Relic Gravitational Wave Models as to What Relic Gravitational Waves Could Tell Us about the Origins of the Early Universe. As Given in an Earlier Paper by the Author
Quoting from [8] we write the following. We reproduce this, because of the centrality of Equation (27) which is basic. It is very noticeable that in [9] we have that the following quote is particularly relevant to consider, in lieu of our results
“Thus, if advanced projects on the detection of GWs will improve their sensitivity allowing to perform a GWs astronomy (this is due because signals from GWs are quite weak) [1] , one will only have to look the interferometer response functions to understand if General Relativity is the definitive theory of gravity. In fact, if only the two response functions (2) and (19) will be present, we will conclude that General Relativity is definitive. If the response function (22) will be present too, we will conclude that massless Scalar-Tensor Gravity is the correct theory of gravitation. Finally, if a longitudinal response function will be present, i.e. Equation (25) for a wave propagating parallel to one interferometer arm, or its generalization to angular dependences, we will learn that the correct theory of gravity will be massive Scalar-Tensor Gravity which is equivalent to f(R) theories. In any case, such response functions will represent the definitive test for General Relativity. This is because General Relativity is the only gravity theory which admits only the two response functions (2) and (19) [4] [7] . Such response functions correspond to the two “canonical” polarizations h+ and h×. Thus, if a third polarization will be present, a third response function will be detected by GWs interferometers and this fact will rule out General Relativity like the definitive theory of gravity”
We argue that a third polarization in Gravitational waves from the early universe may be detected, if there is proof positive that in the pre Planckian regime that the Corda conjecture [10] as given below, namely if the following analysis is part of our take on relic gravitational waves, is supported by the kinetic energy being larger than the potential energy, namely what if
“The case of massless Scalar-Tensor Gravity has been discussed in [4] [12] with a “bouncing photons analysis” similar to the previous one. In this case, the line-element in the TT gauge can be extended with one more polarization, labelled with Φ (t + z), i.e. …”
This ends our recap of the section given in [8] which we think is important.
What we are arguing for is that the choice of the vacuum energy as given by Equation (27) may give conclusive proof as to satisfy the Corda conjecture and his supposition as to the existence of an additional polarization [9] . We will, in the future try to extend our results so as to determine if Equation (27) either falsifies or supports the existence of a 3rd polarization. Which will be a way to determine the final disposition of GR as THE theory of Cosmology, or open up the possibility of alternate theories. It is an issue which we think will require extreme diligence. While ending our query as to the possible existence of a third polarization we should mention what would be the supreme benefit of our upcoming analysis of Equation (27), namely how to avoid the conflating of dust, with gravitational waves, i.e. the tragic Bicep 2 mistake [10] - [13]
End of quote from reference [41] which answers questions as to cosmological questions as to what is necessary for physics interpretation of both bicep 2, and the relative strength of polarization.
Which indicate if we have a scalar-tensor theory of gravity, or something else as is discussed in [37] . We also need to avoid the problems alluded to in references [39] which are due to Gravitational wave signals generated due to galactic dust, and can be seen to be due to multiple point sources in generated Gravitational wave signatures.
Appendix VI. Why Pre Planck Time is Mentioned at All in Our Document
What we are referencing in Equation (12) in the main text is a situation for which there is a pre-Planckian space time for emergent phenomena. The Equation (12) is part of using a publication, [41] , which would allow the formation of a graviton, with varying mass, which finally settles upon a rest mass of 10−62 grams after 10−62 grams. We pick this procedure as part of our also wanting to know how and why there would be a high degree of flat space, as opposed to curved space, right in the aftermath of Planck time, and an emergent space-time construction would be instrumental in terms of understanding the background as given in publication [41] . We submit that [41] gives evidence as to the feasibility and importance of Pre-Planckian space time to understand more fully the dynamics of space-time as well as our model of emergence given in Equation (12).









