Received 18 March 2015; accepted 24 May 2015; published 28 May 2015

ABSTRACT

Two methods of stability analysis of systems described by dynamical equations are being considered. They are based on an analysis of eigenvalues spectrum for the evolutionary matrix or the spectral equation and they allow determining the conditions of stability and instability, as well as the possibility of chaotic behavior of systems in case of a stability loss. The methods are illustrated for nonlinear Lorenz and Rössler model problems.

Keywords:

Nonlinear Dynamical Systems, Stability Analysis Methods, Dynamical Chaos, Lorenz and Rössler Model Problems

1. Introduction

The work is dedicated to the methods of practical stability analysis for systems described by nonlinear autonomous equations. The analysis of such systems is of a particular interest due to the dynamical chaos phenomena, which can be observed in cases of stability loss [1] -[3] . A stability analysis of nonlinear Lorenz and Rössler systems [1] [3] is used as an example, illustrating the possibilities of the suggested methods.

2. Stability Analysis Methods

The more common methods of system stability investigation are the spectral methods, which consist of dynamics spectrum analysis for small perturbations. The problem is defined in the following way.

Let’s assume that the system state is defined by a combination of macroscopic characteristics 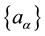complying with the motion equations

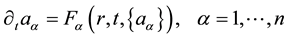. (1)

The linearization of these equations leads to a system of equations describing the dynamics of small perturbations of the initial state

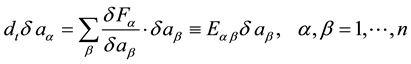. (2)

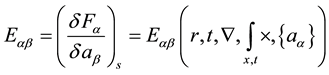
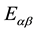―are the elements of the evolutionary matrix operator, which in general depend on initial state parameters, coordinates―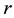, time―, gradients―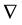, integral operators of space and time convolution type―.

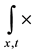The condition of solvability of the system (2) which is its spectral equation―(SE)

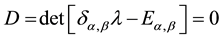(3)

defines the eigenvalues spectrum―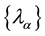of the evolutionary operator and the stability of the initial state―.

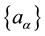In the Fourier-Laplace transform for the perturbations in case of stationary initial states and also in cases when the initial dependences are weak in comparison with the high-speed and high-gradient perturbations (method of local dispersion relation (LDR)), the spectral equation acquires polynomial form

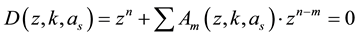, (4)

,―are the parameters of the Fourier-Laplace transform,―are the complex coefficients of SE.

In the classical posing of the stability analysis problem, it comes down to the analysis of spectral equation roots.

The indication of an instability is the presence of a SE root with positive real part Re. If all the SE roots have negative real parts, the initial state described by―is stable.

Due to the fact that the exact solution of Equation (4) with complex coefficients for is impossible to obtain, fixed sign property criteria of real part of the root are being used-Routh-Hurwitz criterion, D-decompo- sition method, frequency criteria. However, their effectiveness is not very high because of their crockness and calculation complexity, especially during the analysis of significantly instable systems. In actual practice, it is more common to use numerical methods or look for special criteria applied to several problem types (energy in the theory of plasma stability and hydrodynamics, criteria for negative differential conductivity in problems on the carrier drift stability in strong energy fields/4/). But any specific criterion is not universal; numeric solutions do not allow obtaining stability conditions in analytical form and viewing their dependencies on parameters. They require a large amount of calculations.

In studies [4] [5] two methods of practical stability analysis for systems described by Equation (1) are suggested. They are called-the neutrality criteria. In studies [4] [6] [7] examples of their appliance is demonstrated. The essence of these methods lies in the determination of the neutral surface―a border separating the areas of stability and instability in the parametric space―using the dynamical equations coefficients (2) or the spectral equations coefficients (4).

1) NSE (neutrality, separation, exclusion) method is based on the spectral Equation (4) and it is implemented according to the following outline

(5.1), (5.2), (5.3), (5.4)

(5.1) Neutrality―the condition of the real part of SE roots being equal to zero;

(5.2) The separation of the spectral equation into two if the neutrality condition is fulfilled;

(5.3) The exclusion of frequency or one of the parameters from the equations and obtaining of a neutral surface―and the critical frequency―;

(5.4) Indication of stability and instability areas in relation to the neutral surface.

The NSE outline is fully realized for the polynomial SE. The general neutrality conditions (3.3) in this case are given by

, (6)

―the resultant, а NOD-GCD―the greatest common divisor of polynomials and.

Specifically for a third order system the conditions (6) are

. (7)

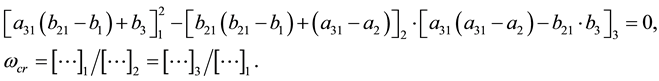
2) The―criterion method is expressed by the determinant of block matrix

, (8)

which consists of the evolutionary matrix and its coefficients unity matrix,―complex conjugate elements of the matrix,―its eigenvalues.

The neutrality criterion and the equation for the critical frequencies in this method have the following form

(9)

The commutation of matrix blocks allows operating them the same way as numbers and in particular reducing the order of its determinant. As a result, the―criterion comes down to the following form

,―are the coefficients of the spectral equation.

3. Nonlinear Systems

Assuming that Equation (1), which describe the system that is being analyzed in terms of stability, represent a combination of nonlinear autonomic equations

. (10)

The perturbation dynamics of system (10) in this case are described by Equation (11)

, (11)

―are the elements of evolutionary matrix, which depend on dynamical variables and time―.

If all the time derivatives in (11) are negative, the perturbations attenuate and the system is Lyapunov stable. If there is at least one positive derivative, the solution curves scatter; the system is not stable. The correlation of derivative signs allows to determine the possibility of chaotic behavior and the formation of complex localized structures―strange attractors [3] (see Figure 1 and Figure 2) in the phase space.

In these cases, the spectral equation method (4) and NSE method (5) for the stability analysis in their classical forms are not applicable.

Due to the fact that the -criterion is directly expressed by the dynamical equation coefficients, it is possible to generalize it for the stability analysis of nonlinear systems by introducing generalized eigenvalues of the evolutionary matrix. At this, the former will be time functions, the sign of which is determined unequivocally by the sign of the time derivatives and which automatically become normal eigenvalues in case of stationary states. This way, the form and the meaning of -criterion in regard to the generalized eigenvalues are reserved.

. (12)

Specifically, the criterion (12) being equal to zero corresponds to the presence of zero-order derivatives (eigen- values), the criterion sign change-corresponds to a sign change of time derivatives in dynamical Equation (11). As a result, the multiplication factor analysis in (12) represents an analysis of evolutionary matrix eigenvalues spectrum for a nonlinear system and therefore, an analysis of time derivatives signs in Equation (11).

Such generalization can technically be conducted for the NSE (5) and spectral Equation (4) methods, but in that case, are not the parameters of Laplace transform, which is not applicable in these circumstances, they are the generalized eigenvalues.

So now we will use the generalized NSE and -criterion methods for a stability analysis of nonlinear Lorenz and Rössler model systems and we also will evaluate the possibilities of the suggested methods to determine the presence of dynamical chaos.

4. Lorenz Model Problem [1] [3]

The Lorenz problem is of a particular interest because nonlinear equations of Lorenz model result from the dynamics equations of a whole range of physical systems: The convection inside a fluid layer heated from underneath, a single-mode laser, water-wheel and other. Besides that, it demonstrates the formation of chaotic dyna- mics (Figure 1).

, ,

The Lorenz model equations have the following form

, (13)

, ,―are the dynamical variables,―are the parameters, where the controlling parameter, representing the intensity is.

In the phase space of variables the system state can be represented by a velocities vector,―the divergence of which characterizes the dissipativity of the system and can be one of the stability conditions.

. (14)

If the phase volume decreases, the trajectories come closer to each other. If the phase volume increases, the trajectories scatter―the system looses stability. From Equation (13) we have

i.e. the Lorenz system is dissipative.

The system (13) has two stationary solutions-stationary states, ,

. (15)

The linearization of system (13) in relation to a solution, for which any, including stationary, solution can be chosen, produces a system of equations for perturbations (11), where the evolutionary matrix is

. (16)

The spectral equation and its coefficients in a stationary case are

;

. (17)

(It should be noted that the dissipation condition coincides in absolute value with the first coefficient of the spectral equation and is equal to the sum of eigenvalues of the evolutionary matrix. It may be shown that there is a common result.)

The NSE method for SE (17) produces two critical-neutral modes

1), (18)

2). (19)

The mode (18) occurs for the first stationary state and corresponds to its instability when, the eigenvalues in this case are

. (20)

The mode (19) occurs for the second stationary state and for the classical values of Lorenz parameters the critical values of frequency and the parameter are

. (21)

When the second stationary state becomes unstable, in this case the real part of the second and third eigenvalues becomes positive, i.e. slow-growing oscillations appear. For example, when

,.

The -criterion for states (15) correspondingly produces expressions

, (22)

. (23)

As could be expected, the criterion (22) shows an instability of the first stationary state (15) when. From (23) it follows that the instability of the second stationary state (existing when) occurs when and that it complies with the NSE criterion and the spectrum. In the criterion (23) there are three multiplier factors changing their sign, which corresponds to the signs of three time derivatives in the initial equations of the system (13). When the first multiplier factor is positive, the second one equals to zero, the third one is negative. Therefore, the combination of derivative signs corresponds to the occurrence condition for chaotic dynamics in the system, see [3] . Specifically, if the eigenvalues signs are

, (24)

The dynamical mode is correspondingly

1) A stable point;

2) A boundary cycle;

3) An attractor (of chaotic dynamics).

This way, in the Lorenz system with up to a chaotic mode with a phase portrait exists Figure 1. When the third derivative changes sign and the system fully looses stability entering the field of complex irregular dynamics.

It should be noted that the criterion (23) includes the first critical mode related to the instability of the first stationary state; also it can be shown that the critical frequency calculated according to formula (7) coincides with the value determined using the spectrum (21).

5. Rössler Model Problem

A nonlinear problem which has an evidentially expressed field of chaotic behavior with an attractor presented in Figure 2, see [1] [3] .

The Rössler model equations have the following form

. (25)

, ,―are the dynamical variables;, ,―the parameters,―the controlling parameter. The diver- gence of velocities vector characterizing the system dissipativity is

. (26)

From (26) it follows that the Rössler system is dissipative only in a limited field (Figure 2).

The system (25) has two stationary solutions-stationary states

, (27)

which are possible under the condition. For Rösslerparameters, , the stationary solutions take the following form

. (28)

The linearization of Equation (25) in relation to the solution, for which any, including stationary, solution can be chosen, produces a system of equations for perturbations (11), where the evolutionary matrix is

. (29)

The spectral equation of the system (25) and its coefficients for the stationary states are correspondingly

;

. (30)

The NSE criterion for SE (30) produces two critical-neutral modes (18, 19), which in this case take the following form

. (31)

The analysis of conditions (31) combined with stationary conditions (27) shows that when both conditions (31) coincide and the SE roots (30) are

. (32)

The first critical mode occurs only for the second stationary state, which, when becomes unstable, and the SE roots, for example, when are correspondingly

. (33)

The second critical mode occurs for the first stationary state, which is also unstable when. And when the SE roots are

. (34)

This way both stationary states are unstable in different ways.

The L-criterion (12) regarding arbitrary solutions after several developments takes the following form

. (35)

For stationary solutions (27) the criterion (35) is rearranged into

. (36)

As one would expect, the -criterion contains both critical modes acquired using the NSE method (31), at this, one of the multipliers in the criterion coincides with the dissipation condition. The -criterion unlike the NSE method, the same way it was in the Lorenz problem, contains an additional-third multiplier which corresponds to the sign of the third time derivative in the initial equations. From (36) it follows that, for the Rössler model, depending on the (r, e, d) parameters, the occurrence of all three modes is possible (see (31)). For example when the multipliers signs in (36) and correspondingly the signs of time derivatives in the initial system are

which indicates a chaotic behavior of the system with a phase portrait of the type illustrated in Figure 2.

6. Conclusion

In conclusion, the use of modified NSE methods and the -criterion for the stability analysis of nonlinear systems allows not only acquiring the stability and instability conditions, but also predicting the possibility of chaotic dynamics in the former.

References