Received 4 March 2016; accepted 23 April 2016; published 26 April 2016

ABSTRACT

Subjective, non-verifiable information (such as satisfaction rating) is often used to supplement objective, verifiable information (such as financial numbers) in contracting. Empirical research finds that non-verifiable information can be used in contracting as a subjective performance measure or as subjective weighting on an objective performance measure. The differences between the two options have not yet been thoroughly explored analytically. This paper considers a multi-task setting where the non-verifiable performance signal provides incremental information about an aspect of the agent’s action. The research finds that when the agent has unlimited liability, both contracting schemes deliver the same payoff to the principal. However, the principal may strictly prefer a subjective weighting scheme in a setting where the agent has limited liability.

Keywords:

Subjective Performance Evaluation, Implicit Contract, Non-Verifiable Information

1. Introduction

Compensation is an important tool to help align employees’ interests with shareholders’ interests. Conventionally, compensation contracts are based on objective, verifiable performance measures such as financial and accounting numbers. While objective performance measures can provide useful information about an employee’s work effort, they are often imperfect in the sense that they may not capture all relevant information and they may be affected by random events beyond an employee’s control. In addition, some of the objective measures (such as accounting profit) may be subject to manipulation (Baker et al., 1988 [1] ). Because objective performance measures are imperfect, in practice, firms often supplement the use of objective performance measures with subjective performance evaluations (such as supervisor or peer evaluation and satisfaction rating). A subjective performance evaluation is useful because it can provide incremental information about an employee’s actions or uncontrollable events affecting the objective measures (Bol, 2008 [2] ; Gibbs et al., 2004 [3] ; Murphy and Oyer, 2004 [4] ; Woods, 2012 [5] ). When the objective measures (such as accounting numbers) are subject to manipulation, a subjective assessment of the actual performance can be used to undo the manipulation (Bol, 2008 [2] ; Gibbs et al., 2004 [3] ; Woods, 2012 [5] ).

While subjective, non-verifiable information is useful for contracting; previous analytical literature provides little guidance on how it should be used in a contract. Subjectivity can be introduced into a compensation contract in various ways. For example, consider a setting where both the output quantity and quality are important to an employer and an employee is rewarded based on both the quantity and quality of the production output. The output quantity is an objective performance measure, which can be verified by a third party. However, assume that the output quality is based on an evaluator’s subjective assessment. The employer can pay the employee based on the output quantity with a fixed piece rate and pay an extra bonus based on the output quality. In this way, the output quality is introduced into a compensation contract as a subjective performance measure. Alternatively, the employer can use the subjective information (quality) to determine the weight on the objective measures (quantity), i.e., pay the employee based on the output quantity with a piece rate determined by the output quality (the better the quality, the higher the piece rate).

Although the analytical literature which investigates how subjective, non-verifiable information should be used in contracting is scarce, empirical research on subjectivity in compensation contracts is growing (Bol, 2008 [2] ) and recent empirical studies start to examine different ways in which subjectivity can be introduced into a compensation contract (Bushman et al., 1996 [6] ; Ederhof, 2010 [7] ; Gibbs et al., 2004 [3] ; Höppe and Moers, 2011 [8] ; Ittner et al., 2003 [9] ; Murphy and Oyer, 2004 [4] ; Wood, 2012 [5] ). This paper aims to fill the gap in the analytical literature and to provide guidance for future empirical research in this area. This paper contributes to the literature by investigating the two different ways in which subjective, non-verifiable performance information can be used in compensation contracts: as a subjective performance measure or as a subjective weight on an objective performance measure. Intuitively, which approach is better should depend, at least to some extent, on the reasons for using a subjective performance evaluation (e.g., because the subjective performance evaluation provides incremental information about the agent’s action, about the noise in other performance measures, or about the manipulation of the objective measures, etc.). This paper considers the situation where the subjective performance evaluation provides incremental information about the agent’s action. The research question is, given that the non-verifiable information is related to the agent’s action, how should the non-verifiable information be used in contracting: as an additional performance measure or as an adjustment to the weight of the existing measure?

Following previous studies, this paper adopts the principal-agent framework in which a principal (an employer) hires an agent (an employee) to perform certain tasks. The agent’s effort is not observable to the principal. The principal, however, observes objective and subjective performance signals, which can be used for contracting. This paper considers a two-task setting where the verifiable performance measure captures the information about one task and the non-verifiable information is related to another task. Assume that the agent is risk neutral. The research finds that when the agent has unlimited liability, the two contracting schemes deliver the same payoff to the principal (given that some regularity condition holds). When the agent has limited liability and the lower bound on the fixed compensation is sufficiently large, the principal prefers a subjective weight contract. Intuitively, the reason is as follows. When a subjective weight is used on an objective measure, the bonus is multiplicative in the realized objective and subjective signals, and therefore, the agent’s ex ante expected payoff is multiplicative in the efforts allocated to the two tasks. The complementarity of efforts in the agent’s payoff function is useful because it helps motivate efforts in both tasks. Given the same amount of variable pay, the subjective weight contract provides greater incentives to the agent. Therefore, the expected amount of bonus needed to induce a certain action is lower and the limited liability rent is smaller under the subjective weight contract. The research also finds that the incremental payoff from using the subjective weight scheme rather than the subjective measure scheme is larger as the marginal benefits of effort from the two actions increase; the degree of complementarity between the two actions in the production function is greater, and the commitment problem is less severe.

The research findings imply that if the subjective performance evaluation is used to provide incremental information on the agent’s action, the use of subjective weighting schemes is more likely observed among low- level employees than high-level employees because the low-level employees tend to have a lower reservation wage. Hence, it is more likely that the limited liability constraint will be binding for the low-level employees. The research also implies that with limited liability problems, the subjective weight scheme tends to deliver a greater payoff to an employer for a job with greater degree of complementarity between various actions and for a job where the employee’s effort is more crucial for success (e.g., a labor-intensive job as compared with a capital-intensive job).

The rest of the paper is organized as follows. Section 2 reviews the literature. Section 3 describes the model. Section 4 considers a benchmark setting where the agent has unlimited liability. Section 5 considers the setting with a limited liability constraint. Section 6 concludes the paper.

2. Literature Review

This paper is related to the literature on implicit contract (a contract based on non-verifiable information). Earlier research in this area considers the setting where only non-verifiable information is available for contracting. The key issue is the commitment problems which arise due to the non-verifiability of the information. The moral hazard problem is double-sided. The agent may shirk, and the principal may renege by not paying the bonus based on the non-verifiable performance evaluation. For example, to avoid paying bonuses, the principle may intentionally understate the performance assessment results. There are several ways in which the use of non- verifiable information in contracting can be sustained: by considering an infinitely repeated game (as in the literature on cartel agreements), by assuming the agent is not certain about the principal’s type (as in Kreps and Wilson, 1982 [10] ), or by using a bonus pool as a commitment tool (e.g. Baiman and Rajan, 1995 [11] ). Earlier work adopts the first approach and considers a trigger strategy where the agent will no longer cooperate once the principal reneges. The key for an implicit contract to be self-enforceable is that the long-term gain from contract continuation to the principal must be greater than the gain from reneging. (See, for example, Bull (1983 [12] , 1987 [13] ), Levin (2002 [14] , 2003 [15] ), and MacLeod and Malcomson (1989) [16] .)

Subsequent research considers the setting where both verifiable and non-verifiable information are available for contracting. Baker et al. (1994) [17] , for example, consider the setting where both an objective performance measure (based on verifiable information) and a subjective performance measure (based on non-verifiable information) are available for contracting. The authors show that a bonus based on the subjective measure cannot be sustained when the distortion in the objective measure is sufficiently low. The authors also investigate the use of non-verifiable information as a subjective weight on the objective performance measure when the agent can “game” the compensation system. Schöttner (2008) [18] extends Baker et al. (1994) [17] to a multi-task setting.

Instead of considering a super game with an infinite horizon, another branch of literature investigates the use of a bonus pool where the principal can commit to pay a bonus pool based on verifiable performance measures to a group of agents. The total compensation (“bonus pool”) to be shared among the agents is determined by the verifiable measures, but the allocation of the bonus pool to each individual agent is based on both the verifiable measure and the subjective measure(s) observed by the principal. Assume no renegotiation, no influence activity, and no collusion. Once committed to the bonus pool amount through an explicit contract, the principal has no incentive to renege. Baiman and Rajan (1995) [11] show in a binary, two-agent setting that the principal is better off when the subjective measure can be used in an implicit contract. Rajan and Reichelstein (2009) [19] extend Baiman and Rajan (1995) [11] to more general single- and multi-agent settings. Rajan and Reichelstein (2006) [20] show that the principal assigns less weight to the non-verifiable measure while relying more on the objective measure when the agents’ non-verifiable measures are negatively correlated. Budde (2007) [21] extends Rajan and Reichelstein (2006) [20] by considering a setting where the agent has limited liability.

In summary, the previous literature has investigated the conditions under which an implicit contract is self- enforceable, and examined when and how a subjective measure should be used in contracting when an objective performance measure is also available. In most cases, researchers consider the setting where subjective, non- verifiable information is introduced into a compensation contract as an additional performance measure. The principal chooses the weight on the objective and subjective measures ex ante. However, as Ittner et al. (2003) [9] suggest, an alternative way to use non-verifiable information is to make the weight on an objective performance measure dependent on the subjective, non-verifiable signal (a subjective weighting scheme). The interesting question is whether the two approaches (subjective measure VS subjective weight) are equivalent. If not, under which conditions is the firm better off choosing one approach over the other? This paper aims to answer these questions in a setting where subjective performance evaluation provides incremental information about the agent’s action.

3. Model Description

Traditionally, principal-agent models are based on an assumption that an agent is risk averse. To simplify analyses, researchers have recently assumed instead that an agent is risk neutral but has limited liability. Following previous work such as Sappington (1983) [22] and Innes (1990) [23] , this paper assumes that an agent is risk neutral for model tractability. Consider a moral hazard model in which a risk-neutral principal hires a risk-neu- tral, work-averse agent.¹ The principal moves first to offer a contract to the agent, who then decides whether to accept the job offer. The agent will accept the job offer if the payoff from the contract is no less than the agent’s reservation utility denoted by U. After the agent accepts the contract, she chooses the level of effort to exert on each of two productive tasks. Let 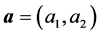where, i = 1, 2 denote the agent’s action. Assume

that the agent’s action is not observable to the principal and is costly to the agent, with

representing the agent’s disutility from working.

The production outcome, x, is a random variable with mean 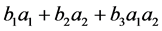and variance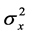. The agent’s effort in each task increases the production outcome in expectation. Assume that the production outcome is not contractible (for example, the outcome from the manager’s action may not be known until the future period). However, there are two performance measures that are observable to both contracting parties. Let 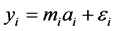denote the performance measure that is informative about a_i, i = 1, 2. The noise in performance measure e_i is a random variable with mean zero and variance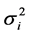, i = 1, 2. Assume that y₁ is verifiable and hence can be used for explicit contracting. Assume further that y₂ is observable to both contracting parties but is not verifiable to the court. In other words, y₂ represents subjective performance information while y₁ is an objective performance measure. The subjective performance signal y₂ provides incremental information regarding the agent’s action a₂, which is not reflected in the measure y₁. To simplify the analysis, assume that 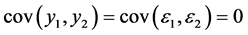, i.e., y₂ is not informative about the noise in y₁ and vice versa.

In this paper, I consider two ways in which subjectivity can be introduced into contracting: 1) through the use of a subjective performance measure with a predetermined weight, and 2) through the use of a subjective weight on an objective performance measure. For simplicity, I consider a linear contract. For the first contracting choice, the bonus is determined by a formula based on one objective measure and one subjective measure, i.e., the contract is of an additive form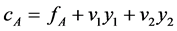. The agent receives a fixed payment f_A and a bonus v₁y₁ + v₂y₂. This contractual form is consistent with the one documented in an empirical study by Höppe and Moers (2011) [8] , which finds that 147 (out of 1753) contracts studied include subjective performance measures with explicit weights on both the objective (financial) and subjective measures.

Another way to use non-verifiable information for contracting is to use the information to determine the weight on another performance measure. Höppe and Moers (2011) [8] find that 416 (out of 1753) contracts studied involve the use of subjective weights (on objective and/or subjective measures). Let 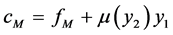denote the contract with a subjective weight on an objective measure. The agent receives a fixed payment f_M and a bonus μ(y₂)y₁. The subjective weight function μ(y₂) can take various forms. To simplify the analysis, I adopt a simple form where the subjective weight function is linear in y₂, i.e., 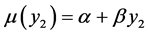so that the contract is represented by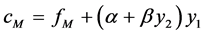. Note that the variable a is included in the contract so that the principal has an option to contract based on y₁ alone by choosing β = 0. With the subjective weight on an objective measure, the compensation is multiplicative in y₁ and y₂.

Compared with the payoff from the subjective measure contract c_A, if under a certain condition the principal is better off with the simple linear subjective weight contract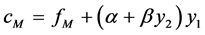, then the principal will also be better off with a more general subjective weight scheme. Therefore, the conditions under which the principal prefers the simple subjective weight scheme c_M with 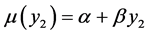over the subjective measure scheme c_A is a sufficient condition for the principal to also prefer a more general subjective weight scheme over the linear subjective performance measure scheme.

Although the subjective performance information can be useful for contracting, commitment problems arise in this setting because a court cannot enforce a bonus based on a subjective evaluation. To account for this commitment problem, I adopt the Kreps and Wilson (1982) [10] approach for tractability. Assume that there are two types of principals, honest and dishonest, and the agent ex ante does not know the principal’s type. The agent’s prior belief is that the principal is honest with probability r Î (0, 1). An honest principal will never cheat. He will always pay the bonus based on non-verifiable information as promised. A dishonest principal will cheat by paying the lowest bonus possible no matter what the realized y₂ is. (Assume that the dishonest principal will renege on y₂ but not on y₁ because y₁ is verifiable to the court so that reneging on y₁ can result in a costly lawsuit.) Let 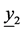denote the lower bound of y₂. The agent’s expected utility from the contracts c_A and c_M are denoted by the following, respectively.

.

.

The principal’s expected utility is denoted by where.

The analyses below consider pooling equilibriums where a dishonest principal offers the same contract as an honest principal. By mimicking the honest principal, the dishonest principal can benefit from the agent’s effort in action a₂ without paying for the service ex post. By offering a different contract, however, the dishonest principal reveals his type. Learning that the principal is dishonest, the agent will not supply any effort on the action a₂ because the agent anticipates that the dishonest principal will renege ex post. The dishonest principal is thus better off mimicking the honest principal than offering a different contract and not being able to induce the effort for action a₂. Thus, this paper considers pooling equilibriums in which both the honest and dishonest principals offer the same contract to the agent. As discussed above, this paper follows the previous literature and assumes the agent’s risk neutrality to simplify analyses. Below, I start with a benchmark case in which the agent is assumed to have unlimited liability in Section 4. Section 5 considers the setting of interest in which the agent has limited liability.

4. The Agent Has Unlimited Liability

As a benchmark case, in this section, assume that the agent is risk neutral and has unlimited liability. In the optimization problem below, I adopt the two-stage approach as in Grossman and Hart (1983) [24] . The first step is to derive the least-cost contract, which can induce the agent to accept the contract and to choose an arbitrary action. The second step is to choose the action that maximizes the principal’s payoff.

4.1. A Formula-Based Contract with a Subjective Performance Measure

Assume for simplicity that. The agent’s expected utility from the formula-based contract with subjective measure is represented by

.

The agent’s maximization problem is as follows.

The agent will choose the action that maximizes her expected payoff. The first-order conditions to the agent’s problem are shown below.

(4.1.1)

(4.1.2)

The second-order conditions for the agent’s problem are satisfied for all action choices because and. The agent’s action choice is therefore

.

Assume that the principal is honest. The least-cost contract, which can induce the agent to accept the contract and to choose an arbitrary action, , can be found by solving the following minimization problem.

subject to

(PC)_A,

(IC)_A

.

To induce the agent to select the action, given the contract offered, the agent must not be better off choosing any other action, i.e., the incentive compatibility constraint (IC)_A must be satisfied. The incentive rate (v₁,v₂)

thus must be such that the agent’s first-order conditions (4.1.1) and (4.1.2) are satisfied, i.e., and. With, the fixed payment that can induce the agent to accept the contract must satisfy

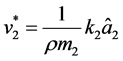
the participation constraint (PC)_A, i.e.,. The fixed payment that minimizes the compensation cost is thus. The minimized cost of inducing is

(4.1.3)

Note that the minimized compensation cost above is the first-best cost plus the additional cost from the commitment problem. Consistent with intuition, it becomes more costly to induce a certain action when the commitment problem is more severe in the sense that the probability of an honest principal r is smaller.

The second step is to find the action that maximizes the principal’s payoff. The principal’s optimization problem is shown below.

,

where C_A(a) is as defined in (4.1.3).

The principal’s payoff thus is equal to, which is the expected production outcome net of the first-best contracting cost, subtracted by the additional cost from the commitment problem. Assume that the cost parameters k₁ and k₂ are sufficiently large so that the second-order conditions² are satisfied. The solution to the above problem and the principal’s maximized payoff follow.

. (4.1.4)

. (4.1.5)

Consistent with intuition, the principal induces greater effort as the marginal benefit of effort in each action increases and the cost of effort decreases, i.e., , i = 1, 2, increases as b_i and b₃ increase or k_i decreases. Also, as r increases, the commitment problem becomes less severe and it is less costly to induce effort for a₂ so that increases.

When b₃ = 0, i.e., there is no complementarity between a₁ and a₂ in the production function, is independent of b₂ and k₂ and vice versa. In addition, is not affected by a change in r. When a₁ and a₂ are complementary, i.e., b₃ > 0, however, a₁ increases as b₂ and r increase or k₂ decreases. This is because when b₂ and r increase or k₂ decreases, the principal wants to induce more effort for a₂. Since a₁ and a₂ are complementary, the principal optimally increases a₁ as well. For similar reasoning, a₂ increases as b₁ increases or k₁ decreases.

4.2. A Contract with Subjective Weighting

With a subjective weight contract, the agent’s expected utility from this contract is represented by

The agent’s maximization problem follows.

The first-order conditions are shown below.

(4.2.1)

(4.2.2)

With, the second-order conditions for the agent’s problem are satisfied when

. (4.2.3)

Assume that the second-order conditions are satisfied. From (4.2.1) and (4.2.2), the agent’s action choice is therefore

.

The honest principal solves the following minimization problem to find the least-cost contract that can induce the agent to accept the contract and to choose an arbitrary action.

subject to

(PC)_M,

(IC)_M

.

To be able to induce an arbitrary action, the incentive weight (a, b) must be such that the first-order conditions (4.2.1) and (4.2.2) and the second-order conditions (4.2.3) are satisfied at. The incentive weight that satisfies first-order conditions (4.2.1) and (4.2.2) at is shown below.

Assume that the second-order conditions (4.2.3) are satisfied.³

With (a^*, b^*), the fixed payment that will induce the agent to accept the contract must satisfy the participation constraint (PC)_M, i.e.,. The fixed payment that minimizes the compensation cost is thus. The minimized contracting cost to induce the action is

(4.2.4)

Again, the minimized compensation cost to induce using the subjective weight contract is the first-best cost plus the additional cost from commitment problems. Note that the minimized contracting cost under the two schemes are equal, i.e.,. This implies that.

The second step is to find the action that maximizes the principal’s payoff. The principal’s optimization problem is shown below.

,

where C_M(a) is as defined in (4.2.4).

Assume that the cost parameters k₁ and k₂ are sufficiently large such that the second-order conditions are satisfied for all a.⁴

The solution to the above problem is

. (4.2.5)

From (4.2.5) and (4.1.4), the principal induces the same action under both contracting schemes. This is because the minimized costs of inducing an arbitrary action under the two contracting schemes are equal when the agent has unlimited liability (given that the regularity condition holds). The principal can use either contract to achieve the same payoff. The results above are summarized in the proposition below.

Proposition 1: Assume that the agent is risk neutral with unlimited liability. The principal induces the same action and receives the same payoff under the two contracting schemes.

It should be noted that while the total contracting costs are equal under the two schemes, the variable component is larger and the fixed component is smaller under the subjective measure scheme as shown in Table 1.

5. The Agent Has Limited Liability

In the previous section, I assume that the agent has unlimited liability. In practice, there may be a limited liability constraint on the amount of the fixed payment. Minimum wage requirements, for example, are imposed worldwide and salaries that we observed in the real world are usually non-negative. Bankruptcy clauses in contracts can also result in corporate stakeholder’s limited liability. Following previous work such as Sappington (1983) [22] and Innes (1990) [23] , I introduced a limited liability constraint in this section. Assume that the fixed payment has to be no less than the lower bound F. In other words, in addition to the participation constraint and the incentive compatibility constraint, there is a limited liability constraint in the principal’s cost- minimization problem.

(LL)_i

Recall that when there is no limited liability constraint, the optimal fixed payment under the subjective measure scheme c_A is, while the optimal fixed payment under the subjective weight scheme c_M is

. With the limited liability constraint, there are three possible cases to consider.

1) The limited liability constraint is not binding under both contracting schemes, i.e., and.

2) The limited liability constraint is binding under both contracting schemes, i.e., and.

3) The limited liability constraint is binding under the subjective measure scheme only, i.e., but.

The solution to case 1 is as characterized in the previous section. In case 3, because the lower bound F is such that, it is optimal to use the contract c_M and the optimal contract is as characterized in Section 4.2. Below, I discuss the solutions to case 2.

When the limited liability constraint is binding under both contracting schemes, the characterizations of least-cost contracts to induce the action with a binding limited liability constraint (case 2 above) and the minimized compensation costs to the principal are shown below.

With the regularity condition holds,⁵, i.e., the subjective weight contract is cheaper. Intuitively, this is because the use of the subjective weight contract causes the actions a₁ and a₂ to become complementary for the agent. The marginal benefit (increase in bonus) from the action a₁ increases when a₂ increases, and vice versa. This implies that, compared with c_A, the principal does not need to use as much incentive pay to induce the desired action with c_M. The fixed payment to satisfy the participation constraint is hence higher for c_M than c_A. With the binding limited liability constraint, the limited liability rent is thus lower with c_M than with c_A.

5.1. A Formula-Based Contract with a Subjective Performance Measure

Given the contracting cost in Table 2, the principal’s maximization problem is as shown below.

.

Assume that the cost parameters k₁ and k₂ are sufficiently large so that the second-order conditions⁶ are satisfied. The solution to the above problem and the principal’s maximized payoff are as follows.

. (5.1.1)

. (5.1.2)

The comparative static results for are similar to the comparative statics results for the subjective measure contract with unlimited liability, i.e., increases as b_i and b₃ increase or k_i decreases, and if b₃ > 0, also increases as b_j increases and k_j decreases, for i, j = 1, 2 and i ≠ j. In addition, as r increases, the principal induces more effort for (and for as well if b₃ > 0).

5.2. A Contract with Subjective Weightings

With characterized in Table 2, the principal’s optimization problem is shown below.

.

Assume that the cost parameters k₁ and k₂ are sufficiently large so that the second-order conditions⁷ are satisfied for all a. The solution to the above problem and the principal’s maximized payoff are as follows.

. (5.2.1)

(5.2.2)

The comparative static results for are similar to the comparative statics results for above. Note that the principal tends to induce greater effort under the subjective weight scheme, i.e.,

for b₃ > 0, and

.

Proposition 2: Assume that the agent is risk-neutral and the lower bound on fixed compensation is sufficiently large (such that the limited liability constraint is binding no matter which contractual form is used). The principal induces greater effort for action a₂ under the subjective weight scheme than under the subjective mea- sure scheme. If b₃ > 0, the principal also induces greater effort for action a₁ under the subjective weight scheme.

Compare the principal’s payoff from the subjective measure scheme (5.1.2) and the payoff from the subjective weight scheme (5.2.2), the principal is better off with the subjective weight scheme. The incremental payoff the principal receives from using the subjective weight scheme rather than the subjective measure scheme is

. (5.2.3)

Proposition 3: Assume that the agent is risk-neutral and the lower bound on fixed compensation is sufficiently large (such that the limited liability constraint is binding no matter which contractual form is used). The principal prefers the subjective weight contract c_M to the subjective measure contract c_A.

Under the subjective weight scheme, the contract is multiplicative in y₁ and y₂. The incentives for a₁ and a₂ hence mutually reinforce each other. As a result, the variable pay needed to implement a certain action is lower under the subjective weight scheme. In other words, with the same amount of variable pay, the subjective weight scheme provides greater incentives. The fixed payment to satisfy the participation constraint is thus higher under the subjective weight scheme. When the lower bound on fixed compensation is sufficiently large so that the principal bears the rent from the agent’s limited liability, the principal incurs a lower rent under the subjective weight scheme.

Note that the incremental payoff increases as the marginal benefits of effort from the two actions and the degree of complementarity between the two actions increase (i.e., b₁, b₂, and b₃ increase respectively). The incremental payoff also increases as the commitment problem is less severe in the sense that the probability of an honest principal r is larger.

6. Conclusions

This paper investigates the question whether the non-verifiable information about the agent’s action should be introduced into the compensation contract as a subjective performance measure or as a subjective weight on an objective performance measure. When the agent has unlimited liability, the two contracting schemes deliver the same payoff to the principal and the principal optimally induces the agent supply the same level of effort under the two schemes. However, it should be noted that while the minimized total compensation cost to induce an arbitrary action is the same under the two contracting schemes, the variable pay is smaller and the fixed pay is larger under the subjective weight scheme. This is because the subjective weight contract is such that the agent’s ex ante expected payoff is multiplicative in the two actions. The incentives for the two actions mutually reinforce each other. With the same amount of variable pay, the subjective weight scheme provides greater incentives to the agent. This is useful in a setting where the agent has limited liability. With less incentive pay needed, the fixed payment to satisfy the participation constraint becomes larger and the limited liability rent becomes smaller with the subjective weight contract. Since the limited liability constraint is more likely to be binding when the agent’s reservation utility is smaller, empirically, one can expect to see greater use of subjective weight contracts for low-level employees with lower reservation wages than for high-level employees.

This paper investigates the setting where subjective performance signals provide incremental information about the agent’s action. As mentioned in previous literature (Bol, 2008 [2] ), subjective performance information may be related to the noise in objective measures or related to the manipulation of objective measures. Subjectivity can also be viewed as ex post contracting to account for the change in environment or to account for the new information, which was not previously available. If the purposes for the use of a subjective performance evaluation are different, the answer to the question whether the non-verifiable information should be used as a subjective performance measure or as a subjective weight may be different as well. Future research that investigates the use of non-verifiable information for other purposes will contribute greatly to our knowledge in this field.

Acknowledgements

The author is very grateful for comments and suggestions of Robert Göx, Suresh Radhakrishnan, Sudipta Basu, and other workshop participants at City University of Hong Kong and the EAA Annual Congress 2011. Part of the work in this paper was done when the author was at City University of Hong Kong and the author would like to gratefully acknowledge financial support from City University of Hong Kong (Project No. 7002169).

Cite this paper

Pattarin Adithipyangkul, (2016) The Use of Non-Verifiable Information Regarding the Agent’s Action in Compensation Contracts. Theoretical Economics Letters,06,234-245. doi: 10.4236/tel.2016.62026

References

NOTES

¹While the agent is risk-neutral, assume that the agent has limited liability and the agent does not have sufficient wealth to buy the firm from the principal.

²The second-order conditions for the principal’s problem are satisfied when.

³With (a^*, b^*), the second-order condition (4.2.3) is not restrictive when the following condition holds:

⁴The second-order conditions for the principal’s problem are satisfied when.

⁵The regularity condition is

⁶The second-order conditions for the principal’s problem are satisfied when.

⁷The second-order conditions for the principal’s problem are satisfied when.