Journal of Modern Physics
Vol.06 No.11(2015), Article ID:59751,13 pages
10.4236/jmp.2015.611159
Complex Riemannian Geometry―Bianchi Identities and Einstein Tensor
Richard A. Hutchin
Optical Physics Company, Calabasas, CA, USA
Email: rahutchin@opci.com
Copyright © 2015 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 28 July 2015; accepted 18 September 2015; published 21 September 2015
ABSTRACT
Riemannian geometry has proved itself to be a useful model of the gravitational phenomena in the universe, but generalizations of it to include other forces have so far not been successful. Here we explore an extension of Riemannian geometry using a complex Hermitian metric tensor. We find that the standard electromagnetic field naturally appears along with two additional fields, which act as mass and charge sources. A first paper set up the basic geometry and derived the Christoffel symbols plus the E&M field equation. This paper continues development with the generalized Riemann curvature tensor, Bianchi identities and the Einstein tensor, laying the basis for field equations. A final paper will then present the field equations.
Keywords:
Unified Field Theory, Gravity, Electromagnetism, Complex Riemannian Geometry, Riemannian Geometry

1. Introduction
There have been numerous attempts to embed electromagnetism into Riemannian geometry, of which the most well known are Weyl’s gauge field [1] , Kaluza’s fifth dimension [2] and Eddington’s affine geometry [3] . Even exploration of making the metric tensor complex (as we propose here) was investigated almost a hundred years ago by Mie [4] and Reichenbacher [5] . These two did not have general relativity available at that time, and their theories led nowhere.
We explore here the implications of assuming a complex Riemannian geometry as a generalized theory of gravity. We find that the electromagnetic field naturally appears as the imaginary part of the metric tensor and that two other fields also emerge, denoted as S and W. The flow of the development has 5 steps listed below. The first paper [6] set up the complex geometry and completed Step 1. This paper completes Steps 2-4, and a third paper will explore the field equations.
1) Derivation of the Christoffel symbols leading to the E&M field equation;
2) Derivation of the Riemann curvature tensors;
3) Symmetries of the curvature tensors including Bianchi identities;
4) Derivation of the Einstein tensor;
5) Field equations for all four fields.
2. Short Summary of the First Paper
We provide here a summary of the first paper [6] , which assumed a complex Riemannian geometry as a generalized theory of gravity. We found that the electromagnetic field naturally appeared as the imaginary part of the metric tensor and that two other fields also emerge, denoted as S and W. That paper presented the concepts of complex Riemannian geometry and derives the Christoffel symbols, the E&M field equation and the two additional fields. While the overall geometry is based on complex numbers, each of the four fields is pure real. The current paper derives the Riemann curvature tensor, Bianchi identities and the generalized Einstein tensor which can be used to provide field equations.
Since general relativity has been a successful theory of gravity, any generalization should reduce to the standard theory in certain approximations. Hence in exploring complex Riemannian geometry, there is strong motivation to include an axiomatic structure which closely parallels the familiar Riemannian geometry. A reference text on General Relativity will be useful to some readers to fill in details of the steps, for the structure of that formulation applies in broad terms. An example of a suitable text would be Introduction to General Relativity by Adler, Bazin and Schiffer (ABS) [7] , but there are many available.
We began with a complex Hermitian metric tensor 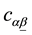 which defined an inner product between covariant complex vectors. The barred index is meant to indicate a complex-conjugate index, so that the inner product of a vector with itself will be real as defined in Equation (2.1), where * indicates complex conjugation.
which defined an inner product between covariant complex vectors. The barred index is meant to indicate a complex-conjugate index, so that the inner product of a vector with itself will be real as defined in Equation (2.1), where * indicates complex conjugation.
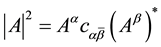 (2.1)
(2.1)
This form of 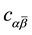 can be written as a sum of its real and imaginary parts as shown in Equation (2.2a) with its Hermitian inverse
can be written as a sum of its real and imaginary parts as shown in Equation (2.2a) with its Hermitian inverse 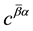 also decomposed into real and imaginary parts as shown in Equation (2.2b). We note that since the two indices are typed for the complex metric tensors
also decomposed into real and imaginary parts as shown in Equation (2.2b). We note that since the two indices are typed for the complex metric tensors 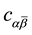 and
and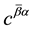 , the indices can be in any order without confusion such as
, the indices can be in any order without confusion such as 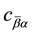 and
and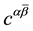 ―a convention that will simplify some of the later equations. We will also use the same convention for
―a convention that will simplify some of the later equations. We will also use the same convention for  and its raised form
and its raised form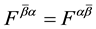 . Since the real part of
. Since the real part of 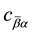 and
and  are symmetric, typing is not required for those two (
are symmetric, typing is not required for those two (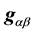 and
and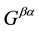 ).
).
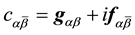 (2.2a)
(2.2a)
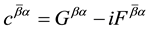 (2.2b)
(2.2b)
Here g is the usual symmetric metric tensor and f is an antisymmetric tensor. We will use bold font for those two basic fields, (









Equation (2.2f) shows that 




Using this result, Equation (2.2g) shows that 









Raising and Lowering Indices: The metric tensor is customarily used for raising and lowering indices of vectors and tensors, and this property also applies to the Hermitian metric with one caveat―the conjugate quality of the index switches. Thus 






We also assume that one can convert between barred and unbarred indices by complex conjugation, i.e. you can create a new tensor with indices of flipped type by taking the complex conjugation of a previous tensor.


3. Covariant Derivative
We begin with scalar differentiation. In classical relativity, the partial derivative and the covariant derivative of a scalar field 



Vector Differentiation: Complex geometry also introduces covariant differentiation with Christoffel symbols in the standard fashion, where there are now two different types of indices for the vector and two for the derivative resulting in four different types of Christoffel symbols shown in Equations (3.2a)-(3.2d).




We note that the Christoffel symbols for the lowered indices can be derived from Equations (3.2a)-(3.2d) using 




Note that with all derivatives of a vector, the vector index is always contracted with one of the first two indices of the Christoffel symbol, which two indices are always of the same type (barred or unbarred). The last index is always the derivative index.
We also require that covariant differentiation maintain the conjugation symmetry. This last statement means that:

and

Equations (3.4a) and (3.4b) imply conjugation symmetry on the Christoffel symbols, namely:


We will frequently need to use the explicit difference S between the barred and unbarred Christoffel symbols and thus give it its own symbol as defined in Equation (3.6a) and (3.6b). Since the difference between two Christoffel symbols transforms as a tensor under coordinate transformations, we know that S must be a tensor, and we proved in the first paper [6] that S is pure real. We also showed that S switches sign when the type of one of its indices switches, as it does in Equation (3.6).

We note one immediate corollary to this definition of S in (3.7a) or equivalently in Equation (3.7b).


Christoffel Symmetry Condition: Since we want this new geometry to reduce to classical Riemannian geometry when the metric tensor is pure real, we will want to impose symmetry conditions on the Christoffel symbols which map to the classical symmetry in the last two indices. We chose the condition shown in Equations (3.8a)-(3.8c), where the Christoffel is unchanged when the last two indices switch. As will be shown below, this condition leads to generalized Bianchi conditions and resulting Einstein tensor. An alternate symmetry, which used complex conjugation in Equation (3.8c), was also explored, but it was found to produce only a constant E&M field―useless for this geometry.



Zero Derivatives for the Metric Tensor: The last requirement we want to make on the Christoffel symbols is that the covariant derivative of the metric tensor vanishes as usual. Since there are two types of covariant derivative, we get two equations―which are just complex conjugates of each other (with a flip of indices).


By taking the average of these two equations, we note that there is a third derivative form in Equation (3.9c) that also gives a zero derivative for the metric tensor and thus may have a place in the geometry. Since this derivative is the average of barred and unbarred derivatives, we use a different slashed symbol. This form of derivative will be essential to derive the Riemann curvature tensor. All the forms of the barred, unbarred and slashed Christoffel symbols are summarized in Equation (3.13).
We note as a caution that the derivation of the Christoffel symbols in the first paper [6] required the selection of a fully real Christoffel symbol to make the complex Christoffel symbols correctly invariant under coordinate transformations. We chose the classical Christoffel symbols for convenience and because they gave an elegant non-recursive formula for the Christoffel symbols. However, as a note for the future, we could also have chosen the fully slashed Christoffel symbols, which are also pure real and are more naturally embedded into this complex geometry. This would change some of the higher order terms in the field equations. Assuming this basic unification process is correct, then only experimental observations will be able to determine which path to take.


The E&M Field Equation:
Once we have the transpose symmetry for the Christoffel symbols (Equations (3.8)) and the zero derivative Equations (3.9), we can derive the desired E&M field equation by antisymmetrizing Equation (3.9a) i.e. summing over all signed permutations of




Expanding that metric tensor in (3.11), we get further simplification in Equation (3.12) since the symmetric 

We note that this field Equation (3.11) is well known to imply that the E&M field tensor 



As a note on the indexing convention used here: A Greek index carries a type, either barred or unbarred, while a roman index indicates a more general index of either type. This convention makes many of the equations much simpler. For instance, the general solution for a lowered Christoffel symbol is shown in Equation (3.13a), with the only condition being that indices a and b must have the opposite type. The bold green Christoffel symbols are the standard ones from classical general relativity, and the bold green covariant derivatives use the classical covariant derivatives. With the classical derivatives and the barred and unbarred derivatives, this complex Riemannian geometry has a total of three different types of derivatives―each of which has its purpose. It is fascinating that the classical Christoffel symbols evolve intrinsically in this complex geometry―as explained in the first paper [6] .
The same expression for the raised Christoffel symbols is given in Equation (3.13b). Note that the barred or unbarred type of index a is used to determine the sign of the f terms. In Equation (3.13a), if index a is barred, then that index position is barred in the two f terms to give






For easy reference we summarize the symmetries of the S and W tensors here, derived in the previous paper.
The S tensor changes sign if the type of the first two indices flip (which must be opposite types) or the third index flips.

If types are fixed, then S is invariant under all 6 permutations of the index values.

The W tensor changes sign if the type of the first two indices switch types, but index type makes no difference to the sign in the last index for W.

Just like S, if types are fixed, then W is invariant under all 6 permutations of the index values

With these symmetries, we note that the sum of two Christoffel symbols which switch type in the first two indices is twice the classical Christoffel symbol.
4. Scalar Curvature Tensor
Now we can deduce some general properties of this type of geometry. We start with the scalar curvature tensor where a scalar function is twice differentiated. When both derivatives are of the same type, then derivatives commute as usual as shown in Equations (4.1a) and (4.1b). However, when they are of different types, then they don’t commute in general as shown in Equation (4.1c). Note that since all derivatives of a scalar field are identical, we can write Equation (4.1c) using the slashed derivative, which is obviously a tensor equation since the difference between two Christoffel symbols is a tensor under coordinate transformations.



The general result is given in Equations (4.2a) and (4.2b), where for convenience in writing these equations we also define the scalar curvature tensor


where

Since the slashed derivative has been established as a third valid type of covariant derivative with its own Christoffel symbols, we point out that indices a and b in Equation (4.2b) can be slashed as well as barred or unbarred. The slashed scalar curvature tensor is simply and intuitively related to the unslashed scalar curvature tensor as shown in Equation (4.3)

5. Riemann Curvature Tensor
We next derive the Riemann curvature tensor and explore its symmetries. It is defined as usual by taking the antisymmetrized double derivative of a vector―but in this derivative the vector may be barred or unbarred and each derivative may be barred or unbarred thereby creating 8 different curvature tensors. While this may seem like a large number, the symmetries will reduce them to 3 types.
Derivatives of the Same Type: To make this process explicit, we start with the simplest curvature tensor derivation, where all indices are of the same type. Begin with the definition of covariant derivative of an arbitrary twice differentiable vector field 








We note that the type of index 






Derivatives of Different Types: The derivation with mixed types of derivatives begins the same way in Equations (5.2a)-(5.2c), but the difference in Equation (5.2d) has two extra terms, marked in yellow because there are two types of derivatives―one barred and one unbarred. We must keep track of those two terms when doing mixed derivatives.




To find the curvature tensor we need to separate the right side of Equation (5.2d) into a tensor component multiplying 


To make this work, we need to use the slashed derivative as we did in Equation (4.1c), which is half way between the barred and unbarred derivatives. The algebra is shown in Equations (5.3a)-(5.3c). In Equation (5.3a) we expand the covariant derivatives. In Equation (5.3b), we add and subtract slashed Christoffel terms, which we regroup in Equation (5.3c). Finally we substitute that result into Equation (5.2d) to get Equation (5.3d), which has clearly separated out a covariant component (the same difference of Christoffel symbols we found in Equation (4.1c)) that multiplies the covariant derivative, leaving the rest to be the Riemann curvature tensor term.
For compactness we defined






where

Since the left side and the last term in Equation (5.e) are obviously tensors, then so is the first term which multiples



We also note a second alternative form of the curvature tensor in Equation (5.4c) which uses covariant derivatives rather than partial derivatives. It is easily verified by expanding the covariant derivative, and is sometimes more useful in derivations because it immediately gives covariant derivatives. It also allows the Riemann curvature to be written with all indices lowered without much change in the form as shown in Equation (5.4d) and then expanded out with simple derivatives in Equation (5.4e). This same technique works with the classical Riemann curvature tensor as well.



One immediate question that arises is how many independent curvature tensors do we have? Since indices a, c and d can be barred or unbarred, we begin with 8. (The type of index b depends on the type of index a and thus is not an independent degree of freedom.) Complex conjugation switches barred and unbarred types and thus divides the number of degrees of freedom in half to 4. Finally, if the last indices are of different type, switching their order simply changes the overall sign of the tensor. This reduces the number of different curvature tensors to 3.
Curvature Tensors for Lowered Indices: As customary, lowered indices also have their own mixed derivative relationships, which use the same curvature tensors as defined in (5.4). To get the lowered curvature tensors, we simply twice differentiate the scalar function

After some algebra, we get the familiar result in Equation (5.5d) with the expected additional term using the scalar curvature tensor.

Using the definition of the Riemann curvature above in Equation (5.3a) and reordering terms, we get Equation (5.5c).

Since Ba is an arbitrary twice differentiable field, we can remove it from the equality to get Equation (5.4d). This equation is identical to the classical equation except for the addition of the scalar curvature tensor term K.

Thus we see that the curvature tensor for lowered indices is the same curvature tensor as for raised indices with a switch in sign―the familiar classical result.
6. Symmetries of the Riemann Curvature Tensor
Now we get to the critical discussion of the symmetries on the Riemann curvature tensor which will allow us to construct the Einstein tensor and field equations. Symmetries come in two versions. One version has the types moving with the indices, and the other version has types remaining in their fixed position with the indices moving. In classical relativity, all indices are the same type, so the difference never appears.
Last two indices: The most obvious symmetry for the Riemann curvature tensor is the antisymmetry in the last two indices, which trivially comes from the definition of the curvature tensor. This symmetry requires that types move with indices. Thus this symmetry is expressed simply as Equation (6.1).

First two indices: We can derive another symmetry relationship for the complex Riemann tensor using techniques similar to ABS. Begin with the scalar function 






Shuffling some indices and combining terms, we get the Equation (6.3a), which becomes the familiar antisymmetric relationship in Equation (6.3b) when we realize that 



The exact same logic holds when 


We also see that the same symmetry holds with mixed type derivatives. We define the scalar function 



Next we expand 


Realizing that the K terms subtract away and then switching index names to combine terms, we get the Equation (6.5a), which becomes the familiar antisymmetric symmetry relationship in Equation (6.5b) when we realize that 



The final summarized result is that the antisymmetry in the first two indices holds for all possible combinations of barred and unbarred indices as stated in Equation (6.6a).

Three-fold symmetry: Another immediately obvious symmetry is a familiar three-fold symmetry but (caution!) here the types do not move with the indices. Such symmetries have not been found to be very useful compared to symmetries where the types move with the indices, but we list it here for completeness. This symmetry follows immediately from inspection of the expressions for the curvature tensor in (5.3c). Since every Christoffel symbol is symmetric in the last two indices, every term immediately zeroes out under the signed sum of permutations. To differentiate clearly between the cases where the types are fixed to positions and where the types are fixed to the indices, we annotate each equation and also use the equation format 




Bianchi Identities: From here on, all the symmetries will use typed indices. The Bianchi identities can now be gotten using the following straightforward technique. We simply begin in Equation (6.8a) and (6.8b) with two trivial identities, where the first term of each equation produces the same 6 terms as the second but with opposite signs since two of the indices are switched.


Subtracting Equation (6.8b) from Equation (6.8a), we get Equation (6.8c). This becomes Equation (6.8d) when we use the Riemann curvature Equation (5.4d).


Now we expand the 



We note that the first term in the first line subtracts out the second term in the second line using the antisymmetric sum

Note that if indices b, c and d were all the same type, then all the K terms would vanish and we would have only two terms left as shown in Equation (6.8g).

Since an arbitrary field and its covariant derivative are two independent degrees of freedom, both components must separately vanish to give the familiar Bianchi identities as the coefficient of An and the familiar three-index symmetry of the Riemann curvature tensor as the coefficient of





The logic supporting this principle goes as follows: The first insight is to point out that the partial derivative 


Bianchi Identities:
Triplet Symmetry:
Dropping the now proven zero middle term in Equation (6.8f), we can rewrite Equation (6.8f) as (6.9c). Then using Equation (6.9b), the terms multiplying the derivatives 


We then add and subtract terms in Equation (6.9d) to get Equation (6.9e).

Since we have already proven that the first term is identically zero in Equation (6.9b), so is the second term. Also recognizing the difference of the Christoffel symbols from Equation (5.3e) as

Given that Ae is an arbitrary field and a is an arbitrary index, as long as the S field is even microscopically non-zero everywhere, then we conclude the very simple result in Equation (6.9g).

The final results of this analysis are given in Equations (6.10).
Bianchi Identities: 
Triplet R Symmetry: 
Triplet K Symmetry: 
We can also lower the n indices to get an equivalent form for the Bianchi and triplet identities assuming that only barred and unbarred indices are used. We are, however, cautious about extending the results to slashed indices
Bianchi Identities: 
Triplet R Symmetry: 
Modified Bianchi Identities: To put these equalities into a slightly different form we can use the Equation (6.10b) (with a lowered first index), which says that the sum over all six signed permutations of the last three indices of Rnabc equals 0. By keeping one term on the left and putting the other 5 terms with opposite sign on the right, we can rewrite the Bianchi identities in Equations (6.10b) as (6.11a). (Note that the c and d index names have been switched here to make the relationship look simpler.)
Since we are antisymmetrizing with the indices (b, c, d), the first term on the right changes sign when we flip the cb indices, which means it can be added to the left side―keeping everything equal to zero. Similarly the second and fourth terms on the right simply double, and the third and fifth terms also double. Dividing everything by two, we end up with Equation (6.9b).


Now we can use the antisymmetric property in the last two typed indices (Equation (6.1)) combined with the antisymmetry in the 



Note that if 
Einstein Tensor: With this background, we can now show the generalization of the zero divergence condition of the Einstein tensor, which will lay the basis for the field equations. The symmetries required for the field equations apply only to a particular set of barred and unbarred indices, namely





Note that two of the double contractions are equal i.e.

Now to get the generalization of the zero divergence Einstein tensor, begin with Equation (6.15a) (a modified Bianchi identity) and write out all the terms explicitly in Equation (6.15a). Note that the generalized Latin indices have been made specific typed indices here. Also note that here all the permuted terms are the same type, which means that the K term is zero, resulting in a very traditional and familiar result.

Now contract Equation (6.15a) with 


This can be rewritten as follows when we remember that

This then will satisfy a zero divergence criterion if we define the generalized Einstein tensor as

The generalized Einstein tensor can also be written in the more compact (and = familiar looking) form of Equation (6.16b) where the contracted curvature tensor Rs is a sum of the four different contracted forms as shown in Equation (6.16c).

where

Since the sum 




The final result of zero divergence is then summarized in Equation (6.17), which looks exactly like the familiar classical conservation equation except for the bar above the first index.

Overview of the Field Equations: In classical relativity, the Einstein tensor is set equal to a general stress- energy-momentum tensor that also satisfies the zero divergence condition. This was an ad hoc assumption extrapolated from known gravitational laws at the time, and its solutions have occupied a significant number of scientists for many different scenarios. Equation (6.18) is thus expected to be one of the field equations of the generalized Riemannian geometry. However, it does contain more than gravity. It contains four different types of field: gravity



7. Summary
In this paper we have explored complex Riemannian geometry further. In particular, we have derived the Riemann curvature tensors (three different ones) and the symmetries that go with them. Using those results, we found the generalized Einstein tensor which satisfies the familiar zero divergence condition suitable for field equations. The next paper will propose and explore field equations for all four of the geometric fields, gravity, electromagnetism and two others that provide mass and charge sources.
8. Conclusion
We see that complex Riemannian geometry inherently has a metric with a real gravity field and an imaginary part resembling the electromagnetic field plus two wholly new fully symmetric third rank tensor fields 


Cite this paper
Richard A.Hutchin, (2015) Complex Riemannian Geometry—Bianchi Identities and Einstein Tensor. Journal of Modern Physics,06,1572-1585. doi: 10.4236/jmp.2015.611159
References
- 1. Weyl, H. (1918) Gravitation und Elektrizität. Sitzungsberichte der K öniglich Preussischen Akademie der Wissenschaften, 465.
- 2. Kaluza, T. (1921) Zum Unittsproblem in der Physik. 2nd Edition, Math. Phys, Berlin, 966-972.
- 3. Eddington, A.S. (1924) The Mathematical Theory of Relativity. 2nd Edition, Cambridge University Press, Cambridge.
- 4. Mie, G. (1912) Annals of Physics, 37, 511-534.
http://dx.doi.org/10.1002/andp.19123420306 - 5. Reichenbächer, E. (1917) Annals of Physics, 52, 134-173.
http://dx.doi.org/10.1002/andp.19173570203 - 6. Hutchin, R.A. (2015) Journal of Modern Physics, 6, 749-757.
http://dx.doi.org/10.4236/jmp.2015.66080 - 7. Adler, R., Basin, M. and Schiffer, M. (1975) Introduction to General Relativity. McGraw Hill, New York.

