ABSTRACT

A new form of Dirac equation of a second order partial differential equation is found. With this wave equation the quivering motion (Zitterbewegung) is satisfactorily explained. A quaternionic analogue of Dirac equation is presented and compared with the ordinary Dirac equation. The two equations become the same if we replace the particle rest mass, m₀, in the latter by im₀. New space and time transformations in which these two equations represent a massless particle are found. The invariance of Klein-Gordon equation under these transformations yields the Dirac equation. The electron is found to be represented by a superposition of two waves with a group velocity equals to speed of light in vacuum.

1. Introduction

In 1928 Dirac introduced his relativistic wave equation [1]. He had wanted to find an equation for the electron that would be consistent with special relativity and describe the known fine structure frequency spectrum of hydrogen. In technical terms the equation was Lorentz-covariant. It correctly described the fine structure of hydrogen. When Dirac examined the solutions to his equation, he found that, much to his surprise, it also predicted the electron’s spin and its magnetic moment. Perhaps even more surprising, his equation also suggested a positively charged particle with the mass of the electron could exist. Based on his equation, in 1931 Dirac predicted what he called the anti-electron (a positron) and confirmed experimentally in 1932. In 1933 he shared with Schrodinger the Nobel Prize for physics for his work in quantum mechanics.

In developing his equation, Dirac assumed that the electron was a point-like particle with an electric charge equal to the experimentally measured charge of the electron. Dirac however did not try to develop visualizable physical models as aids to picturing or understanding his mathematical results.

There are several results of Dirac’s equation which were problematical, despite the equation’s general success. It was mentioned that Dirac did not in this period attempt to make a physical model of the electron and did not support such attempts. However, many of the properties of the Dirac electron, including these problematical properties, lend themselves to a physical modeling approach, which may help resolve some of these problematical properties.

The Zitterbewegung is a theoretical rapid motion of elementary particles, in particular electrons, that obey the Dirac equation. The existence of such motion was first proposed by Erwin Schrodinger in 1930 as a result of his analysis of the wave packet solutions of the Dirac equation for relativistic electrons in free space, in which an interference between positive and negative energy states produces what appears to be a fluctuation (at the speed of light) of the position of an electron around the median, with a circular frequency of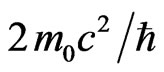, or approximately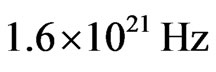. This very rapid motion of the electron means we cannot localize the electron extremely well and gives rise to the Darwin term. This motion never been observed for a free electron, but the behavior of such a particle has been simulated with a trapped ion, by putting it in an environment such that the non-relativistic Schrodinger equation for the ion has the same mathematical form as the Dirac equation (although the physical situation is different) [2,3]. Thus, if we measure the velocity component in any direction, we should either get plus or minus c. This seems quite surprising, but we should note that a component of the velocity operator does not commute with momentum, the Hamiltonian, or even the other components of the velocity operator. If the electron were massless, velocity operators would commute with momentum. In more speculative theories of particles, electrons are actually thought to be massless, getting an effective mass from interactions with particles present in the vacuum state. Barut and Bracken [4] analyzed Schrodinger’s Zitterbewegung results and proposed a spatial description of the electron where the Zitterbewegung would produce the electron’s spin as the orbital angular momentum of the electron’s internal system, while the electron’s rest mass would be the electron’s internal energy in its rest frame. The states of definite momentum are not eigen-states of velocity for a massive electron. The velocity eigen-states mix positive and “negative energy” states equally.

Thus, while momentum is a constant of the motion for a free electron and behaves as it did in non-Quantum Mechanics, the velocity behaves very strangely in the Dirac theory, even for a free electron. While Dirac equation is a first-order differential equation in space and time, Klein-Gordon equations is a second-order in space and time. The two equations are invariant under Lorentz transformations.

We would like in this paper to write Dirac equation as a second—order differential equation in space and time mimicking the standard wave equation. In doing so, we have found new space and time transformations under which Dirac equation represents a particle with zero mass. Moreover, the ordinary Dirac equation is invariant under these transformations. Interestingly, the invariance of Klein-Gordon equation under these transformation yields Dirac equation. The new Dirac equation has many interesting consequences. As in the de Broglie theory, the electron is described by a wavepacket whose group velocity is equal to the speed of light in vacuum. The nature of this wave may explain the Zitterbewegung exhibited by the electron as found by Schrodiner.

2. Quaternionic Quantum Mechanics: Dirac-Like Equation

Consider a particle described by the quaternion wavefunction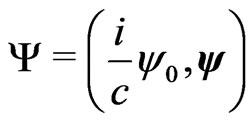. This is equivalent to spinor representation of ordinary quantum mechanics which we have recently developed [5]. The evolution of this quaternion wavefunction is defined by the three equations [5]

(1)

(2)

and

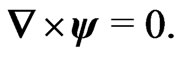(3)

Equations (1)-(3) yield the two wave equations

(4)

and

(5)

Using the transformation

(6)

Equations (1) and (2) become

(7)

Employing Equation (6), Equations (4) and (5) are transformed into the wave equations

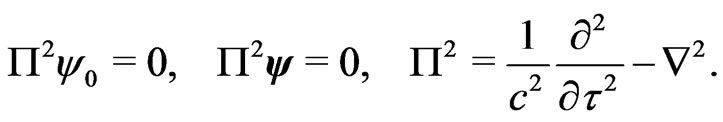(8)

The physical meaning of the vector 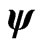is still not clear. But I argue that it can be associated with fundamental particles (eg., quarks) that making up all hadrons, since it has three components. In this way may represent a scalar particle (boson). Notice that Equations (4) and (5) can be obtained from the energy equation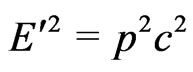, where, 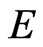is the total energy of the particle, and using the familiar quantum mechanical operator replacements, viz., 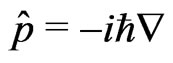and

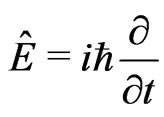. is an energy equation for a massless particle. Thus, it is interesting that a massive particle can be transformed into a massless particle using Equation (6). Since energy is a real quantity, this equation is physically acceptable if it describes a particle with imaginary mass. In this case the energy equations split into two parts; one with and the other with energy. Such energies can describe the state of a particle and antiparticle. A hypothetical particle with an imaginary mass moving at a speed higher than speed of light in vacuum is known as tachyon [6]. Hence, our above equation can be used to treat the motion of tachyons. This implies that our equations, Equations (4) and (5), can be applied to tachyons. Some scientists propose that neutrino can be a tachyonic fermion [7]. This is in favor of the experimental finding that the squared mass of neutriono is negative [8]. We know that Cherenkov radiation is emitted from a particle moving in a medium with speed greater than speed of light in vacuum. When the speed exceeds the speed of light in vacuum, the extra energy acquired by the particle is transformed in radiation. This can happened momentarily for a particle keeping its total energy conserved. Thus, the excess energy (speed) is such that it compensate the dissipations.

3. Ordinary Quantum Mechanics: Dirac’s Equation

Dirac’s equation can be written in the form [1]

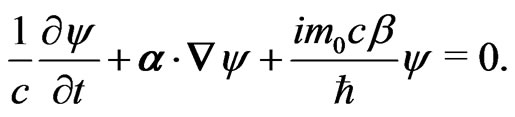(9)

where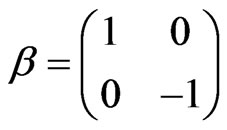, , and

are the Pauli matrices. From Equation (9) one has

. Squaring this equation yields,

(10)

Employing Equation (9) once again yields,

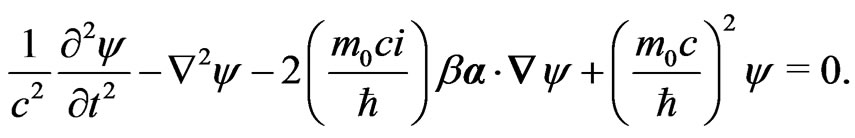(11)

This can be written as

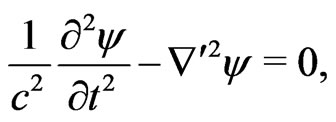(12)

where

(13)

Equation (10) can be obtained from Equation (9) by squaring it. These forms of Dirac equation, Equations (9) and (10), have not been obtained before. They exhibit clearly the wave nature of the electron. It can be compared with the Klein-Gordon equation of spin-0 particles

(14)

Equations (10) and (11) are another form of Dirac’s equation exhibiting the wave nature of spin-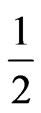 particles explicitly. Using the transformation

(15)

Equation (10) can be written as

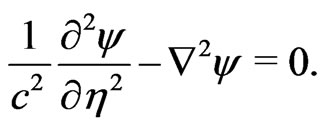(16)

This is a wave equation for a massless particle. Hence, 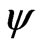can also represents a particle with zero mass when taken in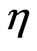—time. Equations (12) and (16) show that the particle is in state of continuous creation and annihilation. This happens after a time of 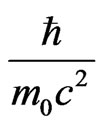and a distance of 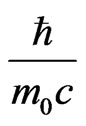which is traversed with speed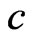, as evident from Equations (13) and (15).

It is interesting to see that the invariance of KleinGordon equation under the transformations defined by Equations (13) and (15) yields Dirac equation. Moreover, Dirac equation, viz., Equation (9), is also invariant under the transformations in Equations (13) and (15).

Since 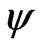is a four components spinor, we can write it in terms of two components doublets, viz.,.

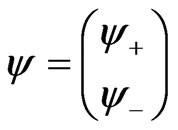Substituting these decomposed spinors in Equation (10), one obtains the two equations

(17)

and

(18)

Equations (17) and (18) imply two energy solutions, one with and the other with energy. Equation (10) can be obtained from Equations (4) or (5) by replacing 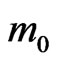by. Therefore, Equations (4) and (5) can represent imaginary massive bodies (tachyons). De Broglie hypothesis that all microparticles exhibit a wave nature. This is possible if we describe a particle by a wavepacket. In this way the group velocity of the wavepacket will be the velocity of the moving particle. However, a single wave can’t describe a particle. In Dirac’s theory the electron is found to move with speed of light and owing to Einstein’s relativity it must have a zero mass. Hence, this motion is problematic in Dirac’s theory. The negative energy equation, i.e., Equation (18), can be written as

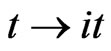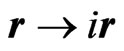which can be seen as a generalized Schrodinger’s equation. It can thus represent the motion of a positron. It is interesting to notice that if we rotate space and time, viz., and, in Equation (4) we will obtain the Dirac positive energy equation, i.e., Equation (17). Similarly, for anticlockwise rotation, viz., 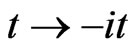and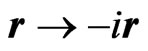, Equation (4) yields the Dirac negative energy equation, i.e., Equation (18). Hence, our Equation (4) is equivalent to Dirac equation in imaginary space-time.

The transformation equations, Equations (13) and (15), can be compared with the covariant transformation that is defined by

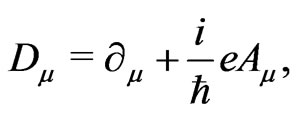(19)

where 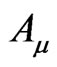is the gauge potential. Hence, one concludes that

(20)

This analogy dictates that 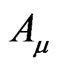to be a matrix. It is related to Dirac matrices by the relation

(21)

The plane wave solution of Equation (5) takes the form

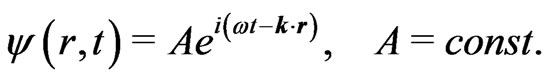(22)

Substituting this solution in Equation (10) yields the dispersion relation

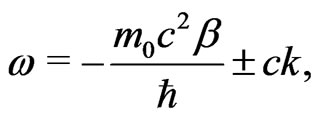(23)

and in Equations (17) and (18) yield the positive and negative frequencies

(24)

respectively. This implies that this plane wave is a collection of two waves of two different frequencies but same wavelength. It is thus a wavepacket. We may assume that a particle is a combination of two states: one moving with momentum 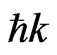in one directions and the other with momentum 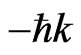is the opposite direction with velocity of light,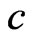. This may imply an internal circular motion resulting from the two states. Hence, in the electron rest frame the total momentum is zero. Does this circular motion give rise to the spin of the electron? Does this imply that the electron is not fundamental and consists of some substructure? Dirac’s theory of the relativistic electron did not include a model of the electron itself, and assumed the electron was a point-like particle. Notice that string theory postulated that the electrons and quarks within an atom are not 0-dimensional objects, but rather 3-dimensional oscillating lines (strings). The theory advocates that these strings can vibrate, thus giving the observed particles their charge, mass and spin.

According to Equation (21) a particle is in a positive energy state when its wavelength is greater than the Compton wavelength, 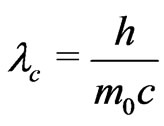, (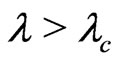), and in a negative energy state (antiparticle) when its wavelength is smaller than the Compton wavelength (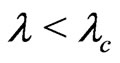). However, a particle existing in the negative energy state (antiparticle) having will be in a state of rest (trapped). Thus, the particle is in essence a superposition between its matter and antimatter states, these two contradictory sides of its personality should interfere, setting the particle quivering (Zitterbewegung). However, this phenomenon has never been observed experimentally because the motion is too small and too fast to detect in real quantum systems.

The group velocity of a (non)-relativistic particle is equal to its velocity. Equations (19) and (20) implies that the group velocity of the wavepacket is given by

(25)

This implies that not the electron as a whole that oscillates but its constituents that having zero mass. Hence real particles and tachyons are particles move with speed of light. However, the phase velocity of each wave comprising the wave packet is less than speed of light in vacuum, as evident from Equations (20) and (21). The group velocity does not necessarily require a particle to have, as evident from Equation (21). It is a transient property of the particle when seen in a different time frame (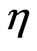). Hence, the Dirac’s velocity dilemma is now resolved.

4. Electromagnetic Wave in Conducting Medium

For a conducting medium with conductivity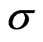, Maxwell equations read for electric and magnetic fields, 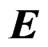, 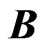the wave equation

(26)

and

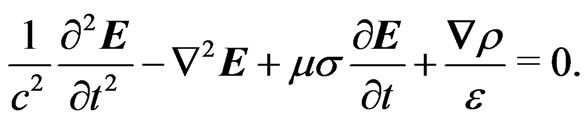(27)

Comparing this with Dirac equation, Equation (4), one obtains the relation

(28)

defining the effective mass (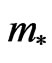) of the magnetic field when propagating in a medium. This is acceptable if we employ the wave-particle hypothesis of de Broglie. It is evident that this mass depends on the conductivity of the medium. Hence, the magnetic field inside a conducting medium behaves like a particle with mass of. Therefore, we see that when an electromagnetic field propagates in a conducting medium, it loses part of its energy so that the photon acquires a mass that is directly proportional to the conductivity of the medium. Moreover, if one now writes the last term in Equation (27) as

(29)

so that Equation (27) will be similar to Equation (17). If we take the divergence of both sides of Equation (29) and use Gauss law, we will obtain

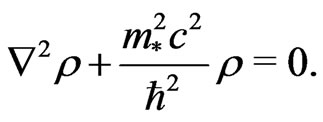(30)

Consider the reflection of positively charged spin-0 particle from a high Coulomb barrier for which the Klein-Gordon equation is [9]

,(31)

where the potential 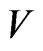is zero to the left of the barrier and a constant to the right. Hence, comparison with Equation (17) reveals that when (massless boson) in Equation (31) the resulting equation will be similar to Equation (17) for spin- particle. In this case, one finds the mass of the spin- particle will be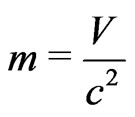. Hence, a massless boson behaves as a massive Dirac particle of an effective mass of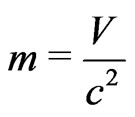. Thus, a massless meson encountering a potential of 0.511 MeV will acquire a mass equals to the electron mass, and therefore, will behave as an electron.

5. Concluding Remarks

We have written Dirac equation in terms of a second-order wave equation. Specific space and time transformations allow the mass term to disappear. When these transformations are applied to Klein-Gordon equation, Dirac equation is obtained. The solution of the Dirac equation yields two oscillating states with positive and negative energies. These states move with a group velocity equals to the speed of light in vacuum. The existence of such states resolved the quivery motion of the electron (Zitterbewegung) found by Schrodinger. This is because not the electron as a whole that oscillates but its constituents that having zero mass. It is quite interesting to notice that the quaternion analogue of Dirac equation yields the ordinary Dirac equation if we replace with.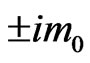

REFERENCES