ABSTRACT

The effect of resonance on the motion of two cylindrical rigid bodies has been studied in the light of Bhatnagar [1] [2] [3] and under some defined axiomatic restrictions. Here we have calculated variation in Eulerian angles due to resonance in terms of orbital elements and unperturbed Eulerian angles.

Keywords:

Inertia Ellipsoid, Ellipsoids of Revolution, Symmetrical Bodies, Orientation of the Bodies, Principal Axes, Eulerian Angles, Critical Points, Perturbations, Averaging of Hamiltonian, Resonance

1. Introduction

Russel [4] studied the motion of two spherical rigid bodies. In same way, Kopal [5] extended the previous work of Russel [4] ; Cowling [6] , Sterne [7] and Brouwer [8] generalized the work of previous authors by considering the lean angle and eccentricity as the small quantities. Johnson and Kane [9] extended the work of above authors by imposing some axiomatic restrictions as follows:

1) The inertia ellipsoids of two rigid bodies 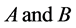for their respective mass centre 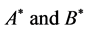are ellipsoids of revolution.

2) Either the distance between 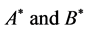is considerably greater than the greatest dimension of either body or the ellipticities of the inertia ellipsoids of 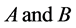are small.

3) The angular velocities of in an inertial frame of reference 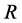are initially parallel to the symmetrical axes of 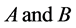respectively.

4) The mass centers 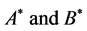move in plane whose orientation is fixed in.

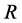Bhatnagar [3] , Elipe and Miguel [10] , Choudhary and Mishra [11] , Mercedes and Elipe [12] have discussed the problem similar to the works of the author of early thirties and forties. But Milution Marjanov [13] has discussed the problem on the cause of resonant motions of celestial bodies in an inhomogeneous gravitational field. He has shown that, when eccentricities of the orbits differ from zero and cross section of the ellipsoids of inertia with orbital plane differs from the circle, the two-cycle resonance is the most stable one. Further Milution Marjanov [13] has discussed the effect of resonance on the problem of two real bodies. He has shown that there are 22 periodic functions and all the variables are coupled. Moreover he established that the stability of the orbit i.e. periodicity of the motion requires 231 resonances.

In our present work, we have proposed to extend the work of Bhatnagar et al. [1] [2] [3] by taking into account the effect of resonance and imposing some modified axiomatic restrictions as follows:

1) The inertia ellipsoids 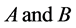for their mass centers 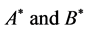are considered as general ellipsoids only but not the ellipsoids of revolution.

2) The angular velocities of are initially parallel to one of the principal axes, which is perpendicular to the orbital plane of.

3) Only the periodic terms are taken and other terms are neglected.

4) The two rigid bodies are symmetrical and cylindrical.

On taking axioms second and fourth under consideration 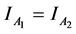for and for, more critical points are found than that found by Bhatnagar and Gupta [1] [2] .

2. Equations of Motion

Let 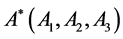be the mass center of the body in the rotating frame of reference having a variable orientation in the fixed frame of reference which is shown in Figure 1. Let be fixed right handed mutually perpendicular axes in. Let us suppose that are lines parallel to the principal axes of at. We assume that -plane is normal to the angular momentum of the system about the centre of mass. Let be the distance between, be the angle between and -axis. Let us assume that be the Eulerian angle with the help of the principal axes of the body at its centre of mass oriented with the fixed axes respectively. Similarly be the Eulerian angles with the help of the principal axes of the body at its centre of mass, oriented with the fixed axes respectively.

Let be generalized momenta corresponding to the generalized co-ordinates respectively. Let be the principal moments of inertia, and be the components of the angular velocities of body respectively. If and be the masses of the two cylinders respectively then the total kinetic energy of the system is given by

(1)

where, kinetic energy of and due to translation.

(2)

Sum of kinetic energy of and due to rotation about the principle axes.

(3)

If be the Eulerian angles shown in Figure 1 then the components of angular velocity are given by

(4)

Thus the combination of Equations (1), (2), (3) and (4) yields

(5)

Since for cylindrical bodies and hence from the Equation (5), we get

(6)

The generalized momenta corresponding to generalized coordinates are given by the relations

(7)

where,

i.e.

From, we get

From, we get

Introducing in the Equation (6), we get

(8)

Following Brouwer and Clemenc [14] the potential for the two bodies is given by

(9)

where is the distance between two elements and of the two bodies respectively and is the gravitational constant. The integration extends over total mass of two bodies.

From Equation (9), we get

(10)

where.

The Hamiltonian function is given by

where, unperturbed Hamiltonian

(11)

Perturbed Hamiltonian,

(12)

The Canonical equations of motion are given by

3. Unperturbed Solutions

The Hamilton-Jacobi Equation for the Hamiltonian is given by

The solution of the above equation is given by

(13)

Hence the solution of the problem can be given in term of the Keplerian elements as

(14)

Here are the usual Keplerian elements, is the eccentric anomaly, and are constants of integration, and are generalized momenta variables corresponding to and respectively.

4. Approximate Variational Equations Corresponding to Perturbed Hamiltonian

The set of approximate variational equations may be given by averaging the Hamiltonian. The averaged value of the Hamiltonian is given by

where is given by the Equation (12).

Here, we observe that by averaging the Hamiltonian, short-periodic terms are eliminated from the Hamilton-Jacobi equation. An approximate set of variational equations are given by

(15)

From the above equations, we get

From Equation (14), we have

(16)

Also,

(17)

(18)

For solving the Equations (17) and (18), we should know as function of time.

5. Solutions for Generalized Co-Ordinates

and are generalized co-ordinates.

For the solution, we will use the Lagrange’s equation of motion

(19)

where kinetic energy and Potential energy of the system given by the Equations (8) and (10) respectively.

From Equation (6), we get

(20)

and

(21)

(22)

From Equation (12), we have

(23)

For

The combination of Equations (19), (20), (21), (22) and (23) gives

(24)

This is the required Lagrange’s equation of motion in.

Again,

Thus the Lagrange’s equation of motion in is

(25)

Again,

(26)

Similarly for.

(27)

(28)

(29)

We have assumed that the angular velocities and of bodies are initially parallel to one of the principal axes which is perpendicular to the orbital plane. If we further assume that no torque (unperturbed motion) is acting on either of the two bodies then both the bodies will spin at a constant rate about that axes and the orientation with the axes will be fixed.

In terms of the Eulerian angles, we have

constant, constant,

constant, constant,

constant, constant,

,.

In the case of perturbed motion, let us suppose that

(30)

where are the constants corresponding to the torque-free solutions and are small quantities which are functions of time.

Since bodies are cylinders hence

(31)

where, radius of body A, length of body A, radius of body B, length of body B.

We replace and by their steady state value and respectively and using the Equation (30) and (31) in Equations (24), (25) and (26) and neglecting higher order terms, then from Equation (24), we have

(32)

From Equation (25), we have

(33)

From Equation (26), we have

(34)

Similarly for the body using Equations (30) and (31) in Equations (27), (28) and (29), we get

(35)

(36)

From Equation (29), we have

(37)

Integrating the Equation (36) and putting the value of in the Equation (37) and neglecting the secular terms, we get

(38)

where are constants independent of and.

Considering Kepler’s equation up to the 1^st order approximation, the solution of the Equation (38) is given by

(39)

Here we can see that if any one of the denominator vanishes, the motion is indeterminate at the point. It depends on the mean motion and the angular velocity of rotation of the body. There are many points at which resonance will occur but for discussion we have consider only one point and for other we can use the similar procedure. We further assume that is a small quantity and at the equilibrium point i.e. mean motion and angular velocity of the rigid body are in the ratio of 1:2. In order to study the behavior at this point we will follow the procedure of Brown and Shook [15] .

6. Resonance at the Critical Points

From right hand side of Equation (39), we have are the critical points. Here we consider for discussing resonance. Now we shall calculate the amplitude and period of vibration in the variable.

We may write the Equation (39) as

(40)

where,

The solution of the equation

(41)

is periodic and given by

. (42)

Let be the function of two independent variable i.e..

The Equation (41) may be written as

. (43)

Then

(44)

We want to replace from Equation (40) by two new variables and which are related to by Equation (42). As we are replacing one variable by other two co-relations between the new variables is at our choice. Let us choose it in such a way that

. (45)

Using Equations (44) and (45), we get

As are function of time, therefore differentiating it with respect to, we get

. (46)

Using Equations (40), (43) and (46), we get

. (47)

Also from the Equation (46), we get

. (48)

Obviously the Equations (47) and (48) are linear equations in.

So solving these equations for these variables, we get

(49)

(50)

where, is a function of only.

Also,

. (51)

As are function of only, we can write the Equation (51) into canonical form with new variables defined by

As so differentiating the Equation (50) and putting the value of and, we get

Neglecting higher powers of, we get

(52)

Here we observe that are present in only as the sum of the periodic terms with argument where are given constants, thus we have

The Equation (53) can be written

. (53)

Now we are considering here the case in which the critical argument is at the point then the affected Hamiltonian is given by

. (54)

Taking as the critical argument in our case so the Equation (53) becomes

. (55)

As the first approximation, if we put (All constants) then Equation (54) becomes

. (56)

This is the equation of motion of a simple pendulum. If co-efficient of is negative then

If the oscillation is small, we can take as oscillates about the value of 0 or. Then Equation (56) becomes

where

Its solution is given by

(57)

where and are arbitrary constants. Thus amplitude and period of vibration

are given by and respectively with similar approximation in the first relation

of Equation (50) and using the Equations (54) and (57), we get.

where can be determined from the equation as is known function.

7. Equilibrium Points for the Body A in Terms of Eulerian Angles

Now we calculate the libration in the variables.

Integrating the Equation (33) and ignoring secular terms, we get

where constants of integration are taken to be zero.

Putting the value of in Equation (32) and ignoring secular term, we get

where etc. are constants.

And the perturbed solution for is given by

(58)

Obviously in the case of one of the denominator becomes zero, the motion cannot be determined at that point, known as critical point and hence resonance arise at that point. In this case usual method fails to determine the motion, so for the present purpose the present purpose we will use the method as that of.

The equation for can be written as

On taking the first approximation, we can see that critical argument oscillates about

or. Also the solution for is given by

(59)

where and are arbitrary constant.

Thus amplitude and period of vibration are given by and respectively, where,

The solution for is given by

(60)

where can be determined from the equation as is a known function. From the Equation (34) it is obvious that depends on so that all the results of can be found in terms of.

8. Equilibrium Points for the Body B in Terms of Eulerian Angles

By proceeding exactly same as above case, we can find out the libration in the variables. Here, we assume that is a small quantity and at the equilibrium point i.e. mean motion and angular velocity of the body B are in the ratio of 1:2. Therefore at this point the resonance will arise. By taking

and the solution up to first order approximation of, we get

(61)

(62)

are arbitrary constants.

. (63)

Also we see that in the libration in the variable the critical argument variable

makes oscillation about the value and the period of libration is given by.

The solution of for small oscillation is given by, where and are arbitrary constant.

is a function of only.

Solution for is given by

Also when we consider the libration in the variable we see that the critical

argument will make oscillation about the value and the period of libration is given by.

The solution of for small oscillation in this case will be, where and are arbitrary constant.

And the solution for is given by

. (64)

where can be determined from the equation as is a known function.

From the Equation (37) it is obvious and depends on, so that the result of can be found in term of.

9. The Solution for the Generalized Momenta Variables Corresponding to Constants of Integration

We have from Equation (16),

Integrating the Equation (17) with respect to, we get

Initially at take and using the Equation (16), we get

where,.

Again from Equation (18), we have

Initially at take and using the Equation (14), we get

where,.

Now we find the time that elapses between the instant at which attains successive minima and the corresponding change in.

We have. Clearly attains it successive minima at.

Let when when. Then from Equations (14) and (34), we have

Again from the Equations (13) and (36), we get

Let when and when

The corresponding change in is given by

10. Conclusions

In the section of “Equations of motion”, we have derived the perturbed and unperturbed Hamiltonian and the canonical equations of motion with respect to the complete Hamiltonian H where are generalized co-ordinates and are the corresponding generalized momenta. In Section 3, unperturbed solutions can be derived by usual course from the Kepler’s equation of motion. For appropriate variational equation, the required generalized co-ordinates have been calculated in Section 5. In section 6, the effect of resonance has been shown in the solutions of the equations of motion of two cylindrical rigid bodies. In Section 7 and 8, equilibrium points have been calculated in terms of Eulerian angles for both the bodies.. Finally the appropriate variational equation in Section 4 has been completely solved in Section 9.

The tools obtained in different sections of the manuscript can be used to discuss the motion of cable connected two artificial satellites. Thus, we may conclude that this article is highly applicable in Astrophysics and Space Science.

Cite this paper

Hassan, M.R., Kumari, B., Hassan, Md.A., Singh, P. and Sharma, B.K. (2016) Effect of Resonance on the Motion of Two Cylindrical Rigid Bodies. International Journal of Astronomy and Astrophysics, 6, 555-574. http://dx.doi.org/10.4236/ijaa.2016.64040

References