Wireless Sensor Network, 2010, 2, 1-6
doi:10.4236/wsn.2010.21001 anuary 2010 (http://www.SciRP.org/journal/wsn/).
Published Online J
Evaluation of Downlink Performance of a Multiple-Cell, Rake
Receiver Assisted CDMA Mobile System
Ayodeji J. BAMISAYE, Michael O. KOLAWOLE
Department of Electrical and Electronics Engineering, The Federal University of Technology, Akure, Nigeria
Received August 15, 2009; revised September 7, 2009; accepted September 10, 2009
Abstract
In wireless Code Division Multiple Access (CDMA) system, the use of power control is indispensable to
combat near-far and fading problems. Signals transmitted over a multipath propagation channel which exhib-
its inter-path interference and fading. The receiver has to employ measures to mitigate these effects or it will
incur severe performance degradation. A classic approach in CDMA communications is the rake receiver. In
this paper, the downlink performance is estimated for a CDMA mobile system at the vertex of multiple ad-
jacent cells. At the base station the received signal is coherently dispread and demodulated using a rake re-
ceiver. The effects of power control, error correction and rake receiver were also investigated on the as-
sumption that the received signals undergo Rayleigh fading, lognormal shadowing, and frequency selective
fading. The evaluation of performance measures of base to mobile link (downlink) of a multiple-cell CDMA
mobile system is presented. This study demonstrates that significant performance improvements are achiev-
able with combined use of power control, rake receiver and error correction scheme.
Keywords: DS-CDMA, Power Control, Rake Receiver, Error Correction, Probability Density Function
1. Introduction
In wireless communication, fading is one of the prob-
lems that cause the signal fluctuation so it may cause
degradation of signal level at the receiver. CDMA as one
of the wireless communication types also experiences
fading. Although downlink channels in CDMA are tran-
smitted with codes that are orthogonal to one another;
that is, they are encoded for minimal mutual interference,
multipath propagation causes the downlink signal to be
“smeared” in time, destroying some of this orthogonality
[1]. Another problem that CDMA suffers is signal inter-
ference from other users because all users in CDMA
system use the same frequency. One of the solutions to
mitigate fading is diversity. In a fading environment, the
principal means for a direct-sequence (DS-CDMA) sys-
tem to obtain the benefits of diversity combining is by
using rake receiver—by coherently combining resolvable
fading. Diversity can improve the quality of the received
signal at the receiver. To combat other users’ interfer-
ence, power control is used to improve the CDMA sys-
tem performance because power control can minimize
the interference between users. Spread-spectrum signals
inherently exhibit frequency diversity. Due to the in-
creased bandwidth, a spectral null is less likely to affect
the entire signal spectrum. For the same reason, however,
the radio channel is likely dispersive. In order to exploit
frequency diversity, the receiver has to collect signal
energy from several multi-paths. For this purpose, a rake
receiver allocates so-called fingers to multi-paths; in
which each finger dispreads the receive signal synchro-
nized to the corresponding path delay, while the receiver
subsequently computes a weighted sum of the rake re-
ceiver fingers’ output.
The signal-to-interference ratio (SIR) in fading chan-
nels varies according to the channels’ response, and the
required SIR to achieve a certain bit-error rate (BER)
depends on the distribution of SIR. To keep the SIR
nearly constant at the desired level, power control can be
used [2]. The reverse link of a CDMA system employs
both open loop and closed loop power control. The open
loop power control is performed at the mobile station
based on the measurement of the downlink signals and
therefore, it can only compensate for the near far dis-
tance problem. The closed loop power control is per-
formed at the base station based on measurements of the
SIR experienced at the base station and therefore re-
quires information to be fed back from the base station to

A. J. BAMISAYE ET AL. 2
the mobile stations. The closed loop power control
scheme aims at reducing the channel fading effects and
the multi user interference experienced at the BS.
In the past many papers have analyzed the perform-
ance of single cell CDMA systems. This is a useful and
necessary step in the analysis of a complete CDMA sys-
tem, as these analyses give expressions for capacity and
system performance measures under a variety of condi-
tions. However, in order to evaluate the performance of a
complete cellular system, the analyses performed for
single cells have to be extended to multiple cells. This
paper analyses the performance of the base-to-mobile
link (downlink) of a multiple cell CDMA system.
This paper is arranged as follows. Subsection 1.1 de-
scribes the system analysis and outlines the derivation of
the probability density functions (pdfs) of the signal
variables. Appropriate system performance measures are
defined in Subsection 1.2. The role of error correction is
considered in Subsection 1.3, power control in Subsec-
tion 1.4, rake receiver concept in Subsection 1.5 and the
results from the analyses are presented in Subsection 1.6.
Finally, the conclusions drawn from this study are sum-
marized in Subsection 1.7.
1.1. System Model
The quality of radio reception by a mobile at the junc-
tion of adjacent cells in a CDMA cellular system is
estimated in this analysis, which is applicable to both
two and three dimensional cellular layouts and is in-
dependent of the cell shape. The signal from the base
station (BS) received at the mobile station (MS) is
assumed to suffer Rayleigh fading, lognormal shad-
owing and frequency selective fading [3]. In some
cases the wide band nature of CDMA channels may
result in fading that is not Rayleigh distributed but is
actually less severe. However [4] reported that signals
occupying a bandwidth of l MHz could suffer
Rayleigh like fades exceeding 10-30 dB. The signals
from the different base stations are assumed to be asyn-
chronous and would fade independently of each other.
II
I
III
Base station
mobile station
Figure 1. Mobile station at the junction of 3-cells.
All the signals (including those intended for other users
in the same cell) transmitted to by a particular BS oc-
cupy the same frequency spectrum and propagate
through the same multipath channel to arrive at an MS.
Consequently, the CDMA signals from a particular BS,
arriving at a given MS, fade in unison. The frequency
selectivity of the channel is modeled by the correlation
bandwidth of the channel. The time delay correspond-
ing to the correlation bandwidth may be measured as a
multiple of the chip period, Tc, [3]. All the users in the
system under analysis are assumed to employ coherent
binary phase shift key (BPSK) modulation and di-
rect-sequence (DS) spreading [5]. All the base stations
and mobile antennas are assumed to be omnidirectional.
The receiver employed in this CDMA system is as-
sumed to reject all but one of the multipath components
of the desired signal. Consider 3 cells: I, II and III,in
Figure 1, where it is assumed that the desired user is
connected to the BS in cell I and all the other base sta-
tions act as interferers. For simplicity it is assumed that
there are k+1 users in cell I and k users in the other in-
terfering cells (i.e. there are k interferers in each cell).
The mobile at the junction of adjacent cells will ex-
perience interference from a number of cells, but the
effect of the Interference from the adjacent cells will
dominate. Therefore in this analysis only interference
from the adjacent cells II and III is considered where
the combined interference is approximated by a Gaus-
sian random variable. When the user is at the junction
of adjacent cells the number of interferers is generally
large enough to make this approximation valid. Having
approximated the total interference, the momentary bit
error-rate


pBER can be approximated by the com-
plementary error function of the signal-to-interference
ratio


erfc SIR [5]. The SIR at the junction of L-adja-
cent cells is given by [5]

1
2222
123
() ()2
L
o
Lb
SIR N
ak bkE

 




(1)
where
a(k) represents the self-interference from the desired
BS. This comprises of unwanted multipath compo-
nents of the desired user’s signal and the interference
from signals intended for other users in the same cell.
b(k) represents the interference from users in the
other cells.
1
2o
N
b
E
is the spectral density of the double sided addi-
tive white Gaussian noise (AWGN). is the energy
per bit.

A. J. BAMISAYE ET AL. 3
1
is the signal strength received from BS I,

2

is
the signal strength received from BS II, etc. It is as-
sumed that 1

, 2

, 3

, ...
L

are each Rayleigh
distributed, and the means of the Rayleigh distribu-
tions are assumed to be log-normally distributed.
β is the voice activity factor. This factor is very im-
portant because although CDMA systems can reduce
self-interference by muting transmission during pauses,
in the downlink much more is required in terms of oc-
casional power control bits, to ensure effective trans-
mission during pauses, and
δ represents the interference reduction factor if power
control is used.
Hence, the momentary bit-error rate can be approxi-
mated as:



erfcSIRpBER (2)
where the complementary error function erfc(x) is the
probability that a normally distributed random variable
will be x or more standard deviations from its mean [6],
and almost linear for large interference variance. By as-
suming the 2
o
b
N
E



22
23
aa
2

term in (1) to be negligible, and
represent the -summation term as
, and substituting in (2) in view of (1), then the
expression for the momentary BER reduces to
( 
2
1
)
L
aa


1
() ()akbk
pBER erfc







 (3)
In order to determine the actual probability of error,
the probability density function of

; i.e.


pdf

,
must be determined using the Laplace transforms and
then evaluating i

terms using Mellin convolution [7].
Thus, pdf of the signal strength received from BS A, i.e.,
pdf of 1

, is given by [8]

2
1
1
1
1
2e




1
1
1
pdf 
(4)
where is the mean-square value of

, which again
is assumed to be log-normally distributed. Often, the
ratios of the mean-square values of the Rayleigh vari-
ables are preferred; i.e., 31
12
,,,
L
2
12



 


1
L



in an attempt to study the relative signal
strength variation as a consequence of adjacent cells in-
fluence. So the pdf of the ratios is expressed:
2
2
2
ln 4
exp 2
2
ii
i
i
ii
pdf






 



 





 














1, 2,,1iL


i
(5)
where

is the mean area of the ith cell (in dBm), i

is the standard deviation of the signal variability (in dB)
and constant


10 4.3429
ln(10)

av
P
 
0
av
PkpBERpdf d
.
1.2. Estimation o f S y stem Pe rformance Me asures
1.2.1. Short-Term Average Bit-Error-Rate (Ber)
The short-term average BER, (k), as a function of k
number of users, is defined as the momentary BER av-
eraged over the Rayleigh fading and may be expressed
by


 
av
SIR


(6)
And the short-term SIR average as a function of k
number of users, (k), can be defined as the mo-
mentary SIR averaged over the Rayleigh fading as

0
1
() ()
av
SIR kpdfd
ak bk


 


ser
P
max
BER

(7)
1.2.2. Service Reliability
The service reliability, (k), may be defined as the
percentage of time the momentary BER is below a
maximum desired level , which alternatively can
be defined as the probability of

pBER
max
BER
less than
. Specifically,




max
() (8)
s
er
Pk probpBERBER


For k users per cell, the service reliability can also be
expressed as




0ser k
P
kprobSIRSIR


0
SIR

0max
erfc SIRBER
0
SIR
0
(9)
where is given by .
For a given value of , a corresponding value of

can be found. Hence the service reliability can be
estimated by evaluating the probability that

0


av-
eraged over the lognormal shadowing, i.e.
 
 
0
0
0
0
1111
000
i
seri i
L
L
Pkpdfpdfdd
pdfpdfpdfd dd










 


(10)
C opyright © 2010 SciRes. WSN

A. J. BAMISAYE ET AL. 4
v(K)
1.2.3. Link Availability
The link availability, , is defined as the per-
centage of locations that the short-term average BER is
below a maximum desired level
link_a
P
s
hort
BER . The link
availability can be calculated by integrating the pdfs of
, …
1
η2 η1
L

 over all possible values for which the
short-term average BER—defined in (6)—is below the
threshold
s
hort
BER ; that is,
 
_0
0
link av
Pkpdf p
 
ii i
dfd d



1, 2,,1iL
123 1
,,,,


(11)
where
L

 

1213 1 ,,,, are values of
L

 


respectively for which the short-term BER is below the
threshold
s
ho
BER
1
,
rt . It should be noted that the values of
123
,,,
L

  
 are functions of all the variables of the
outer integrals.
1.3. The Role of Error Correction
If we assume an block code (i.e. for every m data
bits, error correcting bits are added) capable of
correcting all combinations of c and fewer errors, then
the average BER, , can be approximated by [9]

,lm
m
P
l
b

1li
i
o o
PP

 


e
P

1
1l
b
ic
l
Pi
i
l

 (12)
where is the corresponding BER in the absence of
error correction. If the data is to be transmitted at a par-
ticular rate then as error correcting codes are added to the
data the bandwidth occupied by the composite (baseband
signal) code increases. If only a limited bandwidth is
available for transmission, then the processing gain has
to be reduced to compensate for the greater bandwidth of
the baseband signal.
1.4. The Role of Power Control
Power control is an essential requirement of CDMA sys-
tems. An effective power control scheme—where a po-
wer increase command is sent to all users before a new
high data rate packet is transmitted—could improve the
quality of signal as well as the distribution of signals to
mobile users within BS coverage [10,11]. A simple
power control algorithm that could be implemented re-
quires that the power transmitted from the BS be propor-
tional to the distance between the BS and the user, raised
to the power of the path loss exponent [12]. If the users
are assumed to be uniformly distributed within a circular
cell of radius R, the pdf of the distribution of the mobile
system at a radius, r, from the BS may be expressed by
2
2
r
r
Pr R  (13)
Thus the power control factor ∂ can be written as

0
2
2
N
R
r
rPrdr
RN



 


l
(14)
1.5. The Role of Rake Receiver
The rake receiver consists of a bank of L-correlators or
matched filters (also called fingers) where each finger is
matched to a particular multipath component to com-
bine the received multipaths coherently. In this work,
the rake receiver is assumed to use the maximal ratio
combining (MRC) technique, where the amplitudes of
the received MPCs are estimated and used as weighting
vector

in each finger. Each l

matches the chan-
nel-fading coefficient l

of the received signal. Fol-
lowing [13], and given output signal-to-noise ratio
(SNRo) per bit γb, an approximate expression of bit er-
ror probability,


|eb
P


, conditioned on a particular
channel realization at the output of the rake, is written
thus:


2
1
|
22
1
L
bll
l
eb oL
no l
l
E
PQSNRQ



















no
(15)
where Q(·) is the standard Q function, which by defini-
tion Q(x) is the probability that a standard normal ran-
dom variable (zero mean, unit variance) exceeds x; and

is the noise standard deviation. We assume that the
channel fading coefficients l

are random, so we av-
erage (15) over the probability density function of γb; that
is,


 
b
p


b
pdf




|
0
eebbb
PP pd

to have


(16)

A closed-form expression of (16) is difficult to obtain.
A numerical method can be used to obtain a solution.
Often, some approximations are used in practice. For
instance, by estimating across the fading channel instan-
taneous output SNR per symbol, an average bit-error
probability Pe can be obtained.
1.6. Simulation Results
A simple three-cell system has been considered in this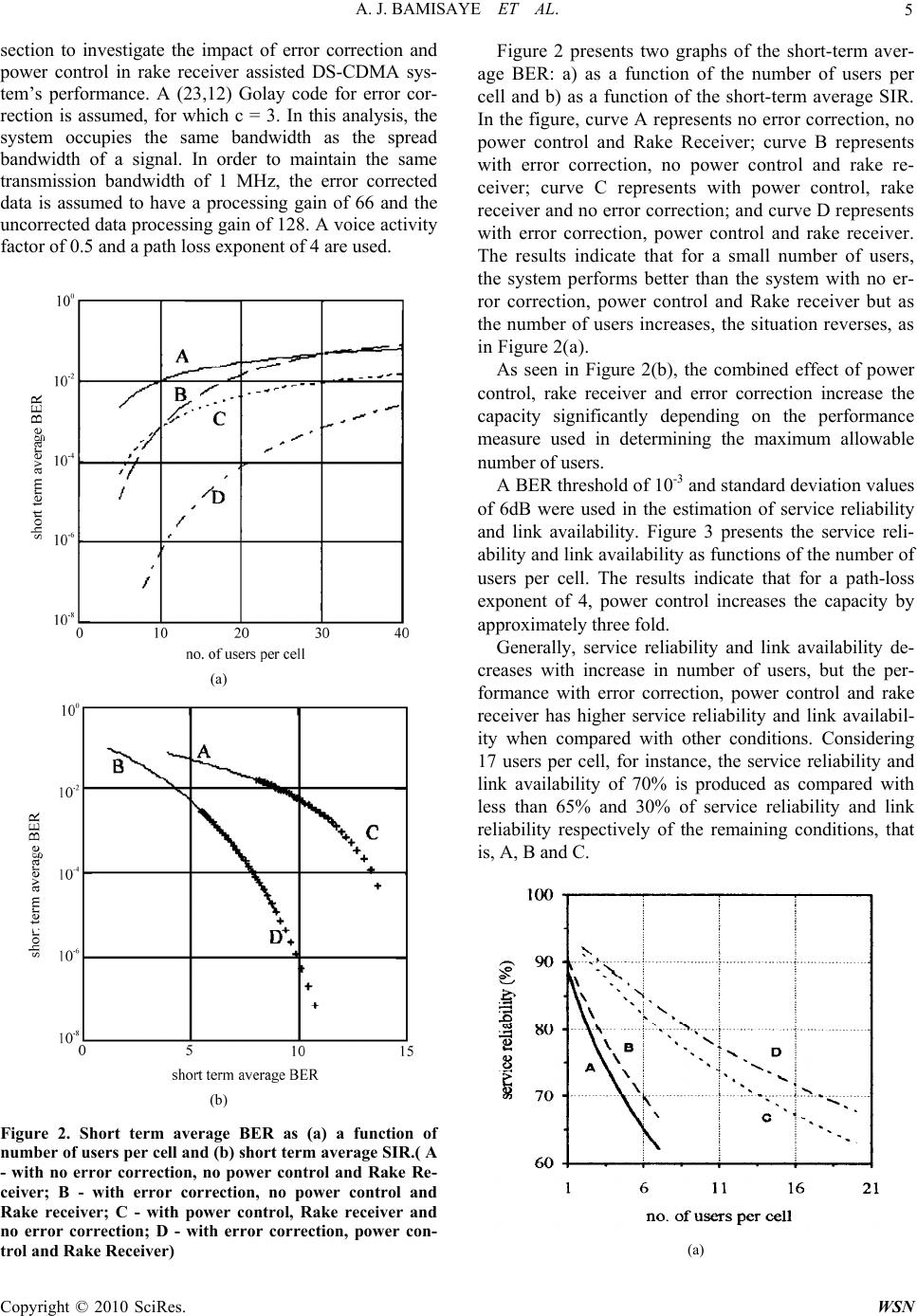

A. J. BAMISAYE ET AL. 5
section to investigate the impact of error correction and
power control in rake receiver assisted DS-CDMA sys-
tem’s performance. A (23,12) Golay code for error cor-
rection is assumed, for which c = 3. In this analysis, the
system occupies the same bandwidth as the spread
bandwidth of a signal. In order to maintain the same
transmission bandwidth of 1 MHz, the error corrected
data is assumed to have a processing gain of 66 and the
uncorrected data processing gain of 128. A voice activity
factor of 0.5 and a path loss exponent of 4 are used.
(a)
(b)
Figure 2. Short term average BER as (a) a function of
number of users per cell and (b) short term average SIR.( A
- with no error correction, no power control and Rake Re-
ceiver; B - with error correction, no power control and
Rake receiver; C - with power control, Rake receiver and
no error correction; D - with error correction, power con-
trol and Rake Receiver)
Figure 2 presents two graphs of the short-term aver-
age BER: a) as a function of the number of users per
cell and b) as a function of the short-term average SIR.
In the figure, curve A represents no error correction, no
power control and Rake Receiver; curve B represents
with error correction, no power control and rake re-
ceiver; curve C represents with power control, rake
receiver and no error correction; and curve D represents
with error correction, power control and rake receiver.
The results indicate that for a small number of users,
the system performs better than the system with no er-
ror correction, power control and Rake receiver but as
the number of users increases, the situation reverses, as
in Figure 2(a).
As seen in Figure 2(b), the combined effect of power
control, rake receiver and error correction increase the
capacity significantly depending on the performance
measure used in determining the maximum allowable
number of users.
A BER threshold of 10-3 and standard deviation values
of 6dB were used in the estimation of service reliability
and link availability. Figure 3 presents the service reli-
ability and link availability as functions of the number of
users per cell. The results indicate that for a path-loss
exponent of 4, power control increases the capacity by
approximately three fold.
Generally, service reliability and link availability de-
creases with increase in number of users, but the per-
formance with error correction, power control and rake
receiver has higher service reliability and link availabil-
ity when compared with other conditions. Considering
17 users per cell, for instance, the service reliability and
link availability of 70% is produced as compared with
less than 65% and 30% of service reliability and link
reliability respectively of the remaining conditions, that
is, A, B and C.
(a)
C opyright © 2010 SciRes. WSN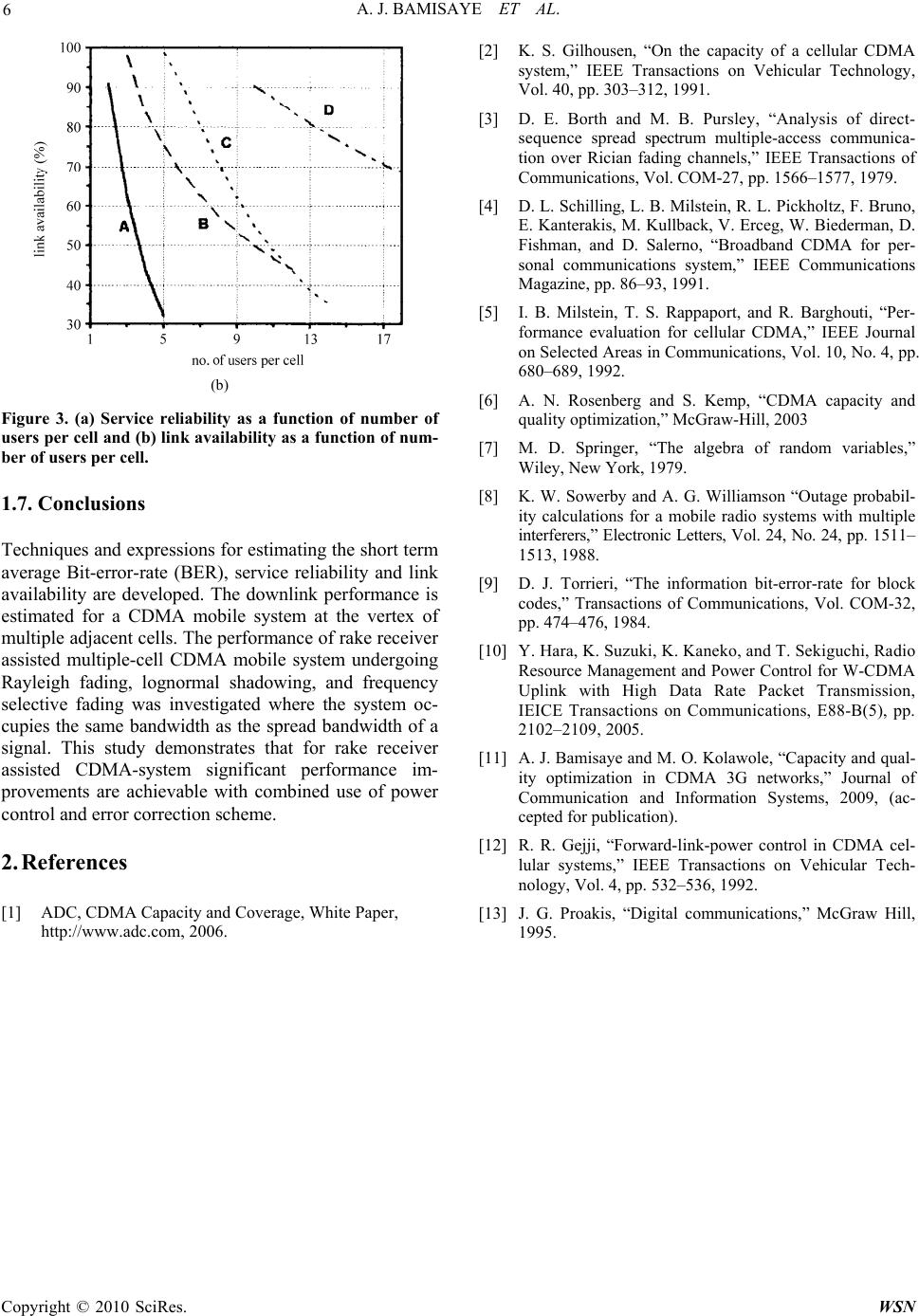

A. J. BAMISAYE ET AL. 6
[2] K. S. Gilhousen, “On the capacity of a cellular CDMA
system,” IEEE Transactions on Vehicular Technology,
Vol. 40, pp. 303–312, 1991.
[3] D. E. Borth and M. B. Pursley, “Analysis of direct-
sequence spread spectrum multiple-access communica-
tion over Rician fading channels,” IEEE Transactions of
Communications, Vol. COM-27, pp. 1566–1577, 1979.
[4] D. L. Schilling, L. B. Milstein, R. L. Pickholtz, F. Bruno,
E. Kanterakis, M. Kullback, V. Erceg, W. Biederman, D.
Fishman, and D. Salerno, “Broadband CDMA for per-
sonal communications system,” IEEE Communications
Magazine, pp. 86–93, 1991.
[5] I. B. Milstein, T. S. Rappaport, and R. Barghouti, “Per-
formance evaluation for cellular CDMA,” IEEE Journal
on Selected Areas in Communications, Vol. 10, No. 4, pp.
680–689, 1992.
(b)
[6] A. N. Rosenberg and S. Kemp, “CDMA capacity and
quality optimization,” McGraw-Hill, 2003
Figure 3. (a) Service reliability as a function of number of
users per cell and (b) link availability as a function of num-
ber of users per cell. [7] M. D. Springer, “The algebra of random variables,”
Wiley, New York, 1979.
[8] K. W. Sowerby and A. G. Williamson “Outage probabil-
ity calculations for a mobile radio systems with multiple
interferers,” Electronic Letters, Vol. 24, No. 24, pp. 1511–
1513, 1988.
1.7. Conclusions
Techniques and expressions for estimating the short term
average Bit-error-rate (BER), service reliability and link
availability are developed. The downlink performance is
estimated for a CDMA mobile system at the vertex of
multiple adjacent cells. The performance of rake receiver
assisted multiple-cell CDMA mobile system undergoing
Rayleigh fading, lognormal shadowing, and frequency
selective fading was investigated where the system oc-
cupies the same bandwidth as the spread bandwidth of a
signal. This study demonstrates that for rake receiver
assisted CDMA-system significant performance im-
provements are achievable with combined use of power
control and error correction scheme.
[9] D. J. Torrieri, “The information bit-error-rate for block
codes,” Transactions of Communications, Vol. COM-32,
pp. 474–476, 1984.
[10] Y. Hara, K. Suzuki, K. Kaneko, and T. Sekiguchi, Radio
Resource Management and Power Control for W-CDMA
Uplink with High Data Rate Packet Transmission,
IEICE Transactions on Communications, E88-B(5), pp.
2102–2109, 2005.
[11] A. J. Bamisaye and M. O. Kolawole, “Capacity and qual-
ity optimization in CDMA 3G networks,” Journal of
Communication and Information Systems, 2009, (ac-
cepted for publication).
[12] R. R. Gejji, “Forward-link-power control in CDMA cel-
lular systems,” IEEE Transactions on Vehicular Tech-
nology, Vol. 4, pp. 532–536, 1992.
2. References
[1] ADC, CDMA Capacity and Coverage, White Paper,
http://www.adc.com, 2006.
[13] J. G. Proakis, “Digital communications,” McGraw Hill,
1995.