Journal of Modern Physics
Vol.07 No.16(2016), Article ID:72940,9 pages
10.4236/jmp.2016.716204
New Expansion Dynamics Applied to the Planar Structures of Satellite Galaxies and Space Structuration
Jacques Fleuret
Independent Researcher, Antony, France

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: November 15, 2016; Accepted: December 20, 2016; Published: December 23, 2016
ABSTRACT
Recent observations of Dwarf Satellite Galaxies (DSG) show that they have a clear tendency to stay in particular planes. Explanations with standard physics remain controversial. Recently, I proposed a new explanation of the galactic flat rotation curves, introducing a new cosmic acceleration due to expansion. In this paper, I apply this new acceleration to the dynamics of DSG’s (without dark matter). I show that this new acceleration implies planar structures for the DSG trajectories. More generally, it is shown that this acceleration produces a space structuration around any massive center. It remains a candidate to explain several cosmic observations without dark matter.
Keywords:
Dwarf Satellite Galaxies, Dark Matter, Expansion, Flat Rotation Curves, Galaxies, Structure, Gravitation, Symmetry

1. Introduction
Dwarf Satellite Galaxies (DSG) have been discovered in the vicinity of Milky Way, M31 [1] - [7] and also near low redshift galaxies [8] . Recent observations have shown that these DSG and other globular clusters tend to stay in thin plane structures [5] [9] [10] , which seem to be co-rotating with the host galaxy [11] [12] . Most of these are orthogonal to the galactic plane, but some have been found with different angles [11] . In any case, they are clearly not isotropically distributed, and correlations are widespread [11] .
Several attempts have been made to explain these observations, introducing tidal effects [11] , past accretion processes [4] [10] [11] [12] , etc. But for several others, these observations seem to be inconsistent with the expected distributions deduced from classical standard cosmological models [5] [9] [13] [14] [15] . More precisely, the assumption of quasi spherical Dark Matter (DM) around galaxies implies the presence of hundreds of DSG’s which should be isotropically distributed around large galaxies. This is in conflict with observations. Other proposals have also been imagined, such as DM haloes, filaments or “superhighways” [11] [16] [17] to attempt to answer the question. But is-it not too ad-hoc to suppose linear DM structures having similar patterns than the observed patterns to be explained?
The problem has also been addressed without Dark Matter by the MOND theory [15] .
I propose here a new attempt to provide a model without DM.
2. Method
In a recent paper [18] , I have introduced a new Expansion Cosmic Acceleration (ECA) to explain the galactic flat rotation curves. This acceleration is proportional to the local expansion rate and to the velocity:
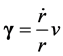 (1)
(1)
Then I showed [19] that this acceleration can be seen as a consequence of a rest-mass erosion theory, where space, time and mass are inter-dependent. It can also be considered as a consequence of SEC theory [20] [21] .
In the present paper, acceleration (1) is supposed to be applied to dwarf galaxies of a given host-galaxy. Solving the dynamics equations with this additional acceleration will give the DSG trajectories. It will then be seen that they remain in co-rotating thin plane structures. Finally, space structuration will be shown to result from the fundamental consequence of the ECA hypothesis, and is no more due to assumed DM structures.
3. The Dynamics Equations
Let-us choose a classical 3D coordinate system, with the host (plane) galaxy centered in the 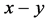 plane.
plane.
The velocity of a DSG located in 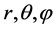 is made of three components (Figure 1):
is made of three components (Figure 1):
-radial component: 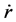
-z-axial rotation:
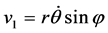 (2)
(2)
-transverse rotation:
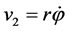 (3)
(3)
Then the well-known 3D dynamics equations can be written, for a DSG submitted to both Newtonian gravitation and to the cosmic acceleration (1):
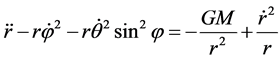 (4)
(4)
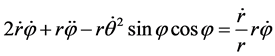 (5)
(5)
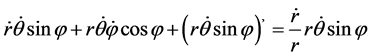 (6)
(6)
The DSG is supposed to be far away from the center of the host galaxy and the total mass M, up to radius , is approximately constant. Furthermore, attraction forces from other DSG’s are neglected here.
, is approximately constant. Furthermore, attraction forces from other DSG’s are neglected here.
Obviously, the purely Newtonian equations (without the last terms in (4), (5) and (6)) lead to the classical elliptic orbits, with no reason to stay in single planar structures.
With the cosmic acceleration terms, Equations (5) and (6) can be rewritten as:
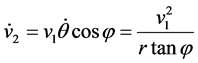 (7)
(7)
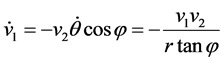 (8)
(8)
We then observe that:
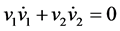 (9)
(9)
Or equivalently:
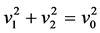 (10)
(10)
where  is constant.
is constant.
Consequently, Equation (4) can be rewritten as:
 (11)
(11)
This equation has the same form as the corresponding eq. for the trajectory of a star within the host galaxy plane, due to gravity and cosmic acceleration [18] . This “inside host-galaxy” case can be considered as a particular case of (11) when 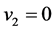 or
or  the flat rotation curve velocity.
the flat rotation curve velocity.
More generally, from (10), the two components of the constant velocity 

Figure 1.A small satellite galaxy is represented as a point, with its velocity vectors (the host-galaxy is in the xy plane).


4. The DSG Trajectories
From (12), (13) and (2), (3):

Then, derivating (13) and using the two Equations (7):

The 

Whose integration leads to:

where C is a constant (
The 


Let us introduce a constant k such that:

(18) can be integrated into:

where the integration constant has been chosen in such a way that 
From (17) and (20), it is easy to obtain the 

And, using (19), we get:

Incidentally, we observe that not all angular values are valid. As an instance, we have:


5. The DSG Planar Structures
The Cartesian coordinates of a DSG are:



From (22), it can be immediately deduced:

Physically, Equation (27) means that the DSG’s stay in a plane, cutting the galaxy plane along Oy (Figure 2). Its apex lies in the xz plane, where





It results that any DSG must have its apex in the same plane (xz), and it must cross the galactic plane along the same line (Oy). All DSG trajectory planes must cut the galactic plane in Oy.
In particular, two important cases can be considered, depending on the initial conditions:
1)
In this case, from (17), 

galactic plane. Then, 

2) C is small.
In this case, for a non-z coaxial trajectory (
according to Equation (17). Velocity 

is also approximately equal to
Figure 2. For a given C value, any DSG must stay in the plane Oyξ, where its velocities are shown, at apex and at intersection with the galaxy plane on Oy.
Clearly, from (27), the DSG stays in a plane, which is quasi-perpendicular to the galactic plane and intersects it along Oy.
An individual orbit is mainly driven by 


Since 



Finally, the DSG orbit parameters r and 
As an instance, in the case:

and if 
as:

and integrated into:

(only valid for large r and large t, according to (28)).
Then, from (3) and (13):

can be integrated, using Equations (17) and (19). In the simple case

which describes the falling motion of a DSG from 



More generally, Equation (27) describes a couple of planes, whose orientations depend on the C value.
To resume, there are several situations, depending on the initial ratio
If 


6. Discussion
If we recall that the host-galaxy mass 
In fact, introducing a cosmic force proportional to velocity creates a symmetry break (Figure 3).
The figure made of a probe mass m at a distance r from a centered mass M is highly symmetrical. But, when the velocity vector of m is added, the new figure is modified under planar symmetry: the probe mass does not turn in the same direction any more. (In the above development, changing 



This symmetry break does not allow any motion: it tends to give a structure to space (planar structures as described by Equation (27), and forbidden orientations such as (23)).
This conclusion is not only valid for planar host-galaxies, but for any massive attractive center. Consequently, our developments could be applied to any “small” massive objects in the vicinity of a huge massive center.
In this case, space structuration due to ECA can be seen in another way (Figure 4). Starting from a given unique massive center M placed at the point O, if a small probe mass 





Since planar structures are predicted in the vicinity of one “host” massive center, linear filaments (2 plane intersects) should be obtained around two massive centers. And in the vicinity of more than two hosts, matter accumulation in spots should happen (plane and line intersect). Could it be that the new ECA give some insight to the structure of the universe at larger scales?
In any case, the ECA hypothesis engraves a fundamental structuration into space. Whenever, for the DM hypothesis, this structuration had to be assumed to come from the DM repartition itself.
Figure 3. Symmetry break due to the cosmic acceleration. Left: Newtonian gravity is highly symmetrical. Right: with the additional cosmic force proportional to velocity, the mass probe and its image do not turn the same way.
Figure 4. Space structuration around a massive spot: the orbit plane of the 3rd mass does necessarily include the intersection (D) of the two first orbit planes.
7. Conclusions
I have shown that the hypothesis of the new cosmic acceleration (1) not only explains the flat rotation curve problem, but also leads to the conclusion that the DSG’s do stay in co-rotating planes with the host galaxy. Whenever their “falling down” velocity (
More generally, I have shown that the cosmic acceleration proportional to velocity introduces a symmetry break around any massive spot. This implies the formation of planar, linear or concentrated mass repartitions in space.
Of course, further observations and experiments will be needed to test the ECA hypothesis.
Close observations of DSG planes will have to be correlated with the proposed model. I also suggest introducing ECA into simulations of galaxy formation, galaxy collisions and also higher-level universe structures.
Cite this paper
Fleuret, J. (2016) New Expansion Dynamics Applied to the Planar Structures of Satellite Galaxies and Space Structuration. Journal of Modern Phy- sics, 7, 2357-2365. http://dx.doi.org/10.4236/jmp.2016.716204
References
- 1. Libeskind, N.I., et al. (2005) Monthly Notices of the Royal Astronomical Society, 363, 146-152.
https://doi.org/10.1111/j.1365-2966.2005.09425.x - 2. Belokurov, V., et al. (2006) The Astrophysical Journal, 647, L111-L114.
https://doi.org/10.1086/507324 - 3. Belokurov, V., et al. (2007) The Astrophysical Journal, 654, 897-906.
https://doi.org/10.1086/509718 - 4. Ibata, R.A. (2013) Nature, 62, 65.
- 5. Conn, R., et al. (2013) The Astrophysical Journal, 766, 120-135.
https://doi.org/10.1088/0004-637X/766/2/120 - 6. Koposov, S.E., et al. (2015).
https://doi.org/10.1088/0004-637X/805/2/130 - 7. Drlica-Wagner, A. (2015)
https://doi.org/10.1088/0004-637X/813/2/109 - 8. Ibata, N.G., et al. (2014) Nature, 511, 563-566.
https://doi.org/10.1038/nature13481 - 9. Kroupa, P., Theis, C. and Boily, C.M. (2004) A&A, 517-521.
- 10. Libeskind, N.I., et al. (2011) Monthly Notices of the Royal Astronomical Society, 411, 1525.
https://doi.org/10.1111/j.1365-2966.2010.17786.x - 11. Libeskind, N.I., et al. (2015) Monthly Notices of the Royal Astronomical Society, 452, 1052-1059.
https://doi.org/10.1093/mnras/stv1302 - 12. Ibata, R.A., et al. (2014) Astrophysical Journal Letters, 784, L6-L10.
https://doi.org/10.1088/2041-8205/784/1/L6 - 13. Pawlowski, M., Pflamm-Altenburg, J. and Kroupa, P. (2012) Monthly Notices of the Royal Astronomical Society, 423, 1109-1126.
https://doi.org/10.1111/j.1365-2966.2012.20937.x - 14. Pawlowski, M., Kroupa, P. and Jerjen, H. (2013) Monthly Notices of the Royal Astro-nomical Society, 435, 1928-1957.
https://doi.org/10.1093/mnras/stt1384 - 15. Kroupa (2012) International Journal of Modern Physics D, 14-35.
- 16. Lovell, M.R., Eke, V.R., Frenk, C.S. and Jenkins, A. (2011) Monthly Notices of the Royal Astronomical Society, 413, 3013-3021.
https://doi.org/10.1111/j.1365-2966.2011.18377.x - 17. Wang,J., Frenk, C.S., Navarro, J.F., Gao, L. and Sawala, T. (2012) Monthly Notices of the Royal Astronomical Society, 424, 2715-2721.
https://doi.org/10.1111/j.1365-2966.2012.21357.x - 18. Fleuret, J. (2014) Astrophysics and Space Science, 350, 769-775.
https://doi.org/10.1007/s10509-014-1797-y - 19. Fleuret, J., (2015) Astrophysics and Space Science, 357-368.
- 20. Masreliez, J. C., (2012) The Progression of Time. Appendix III.
- 21. Masreliez, J.C. (2004) Apeiron, 11, 99.
Abbreviations
ECA = Expansion Cosmic Acceleration
DSG = Dwarf Satellite Galaxy
DM = Dark Matter








