Journal of Modern Physics
Vol.3 No.2(2012), Article ID:17701,6 pages DOI:10.4236/jmp.2012.32028
The Structure Functions in the Weak and Semileptonic Decay of Meson B to the Mesons D and D*: A Computational Study
Institute for Advanced Studies, Tehran, Iran
Email: *mohammadalighorbani1983@yahoo.com
Received October 22, 2011; revised December 13, 2011; accepted January 4, 2012
Keywords: Bethe-Salpeter Equation; Heavy-Mesons Decay; Weak Decay; Quark; Meson; Semileptonic Decay; Hadron; Hadronic Decays
ABSTRACT
In this paper, first, we achieve the first-order wave function of the heavy mesons through the Bethe-Salpeter equation with a confining kernel. Afterwards, by means of this function, the structure functions of the decays of B to D and D* are obtained, and the structure functions’ slope and curve are computed. The obtained results are suitably consistent with the empirical values. The flows expressing transitions according to the invariance structure functions, that solely depend on the momentum transfer (q2) between hadrons, are stated to describe the dynamics of the quarks inside the hadrons and weak hadronic decays. One of the methods for obtaining the structure functions is to apply the BetheSalpeter wave function (B-S). In this work, by virtue of the zeroand first-order B-S wave functions, the structure functions for the semileptonic transitions of heavy mesons ( ) are achieved.
) are achieved.
1. Introduction
In this paper, we study the Beth-Salpeter equation for the bound state of two-piont particles with a confining kernel. Accordingly, we achieve the wave function of heavy meson in the framework of heavy quark effective theory assumptions. We compute the semileptonic weak decay of B to D and D* using the BS wave function to give q2- dependence of the form factors. The obtained results for the form factors, their slopes and curvature are consistent with the empirical data. The QCD as the strong-interactions theory has succeeded in describing the high-energy physics. The discovery of asymptotic freedom has made it possible to perturbationally calculate a substantial number of physical quantities. Nevertheless, the QCD behaves inappropriately at the infrared region. For instance, there is not a tool to describe the enclosure of quarks so that either the lattice gauge theory should be utilized to describe it or the QCD should be utilized to solve it through creating effective theories.
Nowadays, the weak decays of hadrons including heavy quarks are used for experimenting on the standard model and measuring its parameters. Especially, these decays are an appropriate tool to obtain the CKM matrix’s entries. Considering the QCD’s problems, the employment of completely independent ways of investigating such decays is of great significance [1-3].
To acquire the zero-order wave function, we conform to the references [1-3] that are based on the zero-order HQET. More, a confining kernel [4-6] is introduced for the Bethe-Salpeter equation; through utilizing this kernel, the first-order Bethe-Salpeter wave functions are achieved. Then, the structure functions of 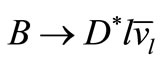 and
and 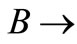
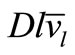 transitions are obtained by means of the zeroand first-order Bethe-Salpeter wave functions.
transitions are obtained by means of the zeroand first-order Bethe-Salpeter wave functions.
2. The Bethe-Salpeter Equation and Confining Kernel
In the momentum space, the Bethe-Salpeter equation for the two-fermion bound state (quarks) is as follows:
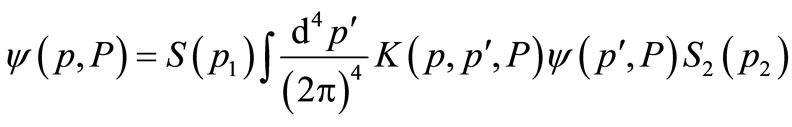 (1)
(1)
where 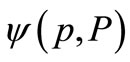 is the amplitude of B-S for the bound state with the momentum P,
is the amplitude of B-S for the bound state with the momentum P, 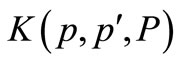 is a kernel of B-S equation, and the
is a kernel of B-S equation, and the 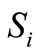 s are the free particle propagators. In general, the kernel K is a sum of all irreducible renormalized diagrams representing the Green’s function with two incident fields and two outgoing fields.
s are the free particle propagators. In general, the kernel K is a sum of all irreducible renormalized diagrams representing the Green’s function with two incident fields and two outgoing fields.
Therefore, if the amplitude of the zero-order wave function ( and the B-S equation’s kernel are available, the bound-state wave function can be achieved at every desirable order.
and the B-S equation’s kernel are available, the bound-state wave function can be achieved at every desirable order.
The references [1-3], that are based on the zero-order HQET, are conformed to obtain the zero-order wave function. The heavy quark is free and moves as fast as hadrons; consequently, it can be written as the form below:
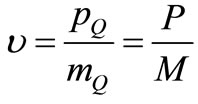 (2)
(2)
where P and M are respectively the meson’s momentum and mass, 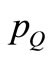 and
and 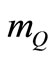 are the heavy meson’s momentum and mass, respectively. When the heavy quark is free, the quark spin can be generally indicated in a fourdimensional space of Dirac indexes. For example, a heavy meson is demonstrated by a two-index wave function (
are the heavy meson’s momentum and mass, respectively. When the heavy quark is free, the quark spin can be generally indicated in a fourdimensional space of Dirac indexes. For example, a heavy meson is demonstrated by a two-index wave function ( ). However, when the heavy quark moves as fast as meson,
). However, when the heavy quark moves as fast as meson, 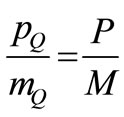 is true for the Bergman-Wigner equation.
is true for the Bergman-Wigner equation.
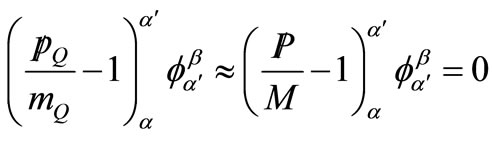 (3)
(3)
The above equation brings about the following quasiscalar wave function:
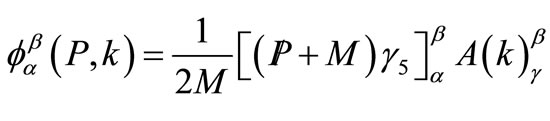 (4)
(4)
where 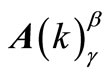 is a matrix indicating the small degrees of freedom (soft gluons and light quark) that is a Lorentz scalar matrix function of light quark momentum. Herein, we choose A(k) as follows:
is a matrix indicating the small degrees of freedom (soft gluons and light quark) that is a Lorentz scalar matrix function of light quark momentum. Herein, we choose A(k) as follows:
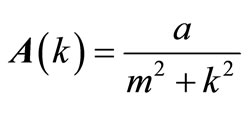 (5)
(5)
where a is a normalizing constant. k and m are the light quark’s momentum and mass, respectively. Hence, the zero-order wave function is written as the form below:
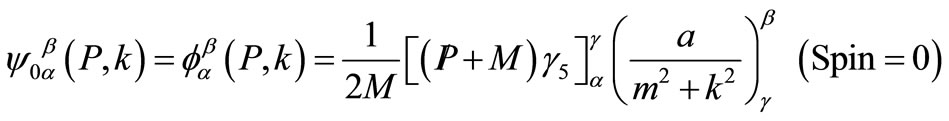 (6.1)
(6.1)
 (6.2)
(6.2)
Now, to obtain the first-order wave function, it is sufficient to have the kernel of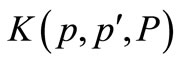 . In the reference [4-6], through taking a confining kernel, and after the Wick rotation, they achieved
. In the reference [4-6], through taking a confining kernel, and after the Wick rotation, they achieved 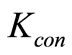 for the B-S equations the scalar kernel superposition in the momentum space:
for the B-S equations the scalar kernel superposition in the momentum space:
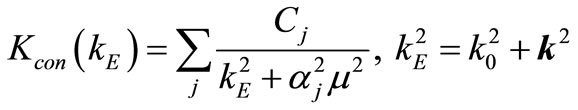 (7)
(7)
and applying the below conditions:
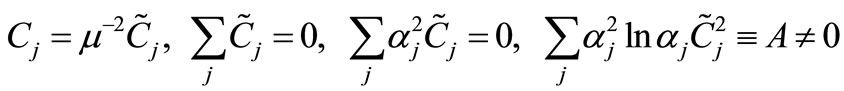 (8)
(8)
A kernel is obtained as the following form:
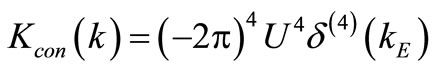 (9)
(9)
into which a new effective coupling constant (U), that has mass, is inserted to be simplified. The Fourier transform of the kernel of Equation (9) is a constant in the fourdimensional space (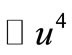 ). This means that it does not behave same as a non-relativistic constant potential for which the
). This means that it does not behave same as a non-relativistic constant potential for which the  like behavior is expected. Therefore, the kernel of Equation (9) is not exactly similar to the nonrelativistic constant potential, and the effective constant (
like behavior is expected. Therefore, the kernel of Equation (9) is not exactly similar to the nonrelativistic constant potential, and the effective constant ( ) does not relate to a constant of such potential.
) does not relate to a constant of such potential.
By the impact of the kernel of Equation (9) on the B-S equation of Equation (1), and under the Wick rotation, it can be written as:
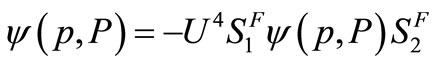 (10)
(10)
3. Results and Discussion
3.1. The Computation of Structure Functions Using the Zero-Order Bethe-Salpeter
In this paper, for the first time, we proposed a novel analytical approach toward the structure functions in the weak and semileptonic decay of meson B to the mesons D and D*. The matrix of the flow producing the transition of mesons is as follows:
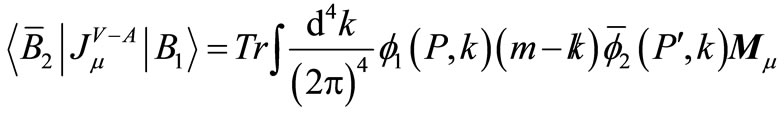 (11)
(11)
where 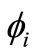 is the ith meson’s wave function that can be calculated at every desirable order by virtue of Equations (1) or (10) and (6);
is the ith meson’s wave function that can be calculated at every desirable order by virtue of Equations (1) or (10) and (6); 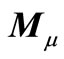 expresses the weak-vertex flow, and is proportional to the CKM matrix, and equals
expresses the weak-vertex flow, and is proportional to the CKM matrix, and equals 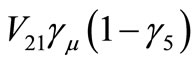 in which
in which 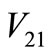 is the corresponding entry to this transition. Accordingly, the
is the corresponding entry to this transition. Accordingly, the 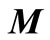 matrix of flow for
matrix of flow for 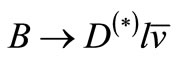 transitions can be written as follows:
transitions can be written as follows:
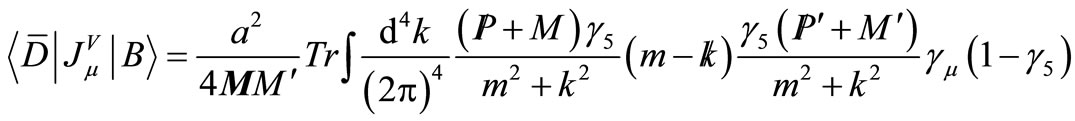 (12.1)
(12.1)
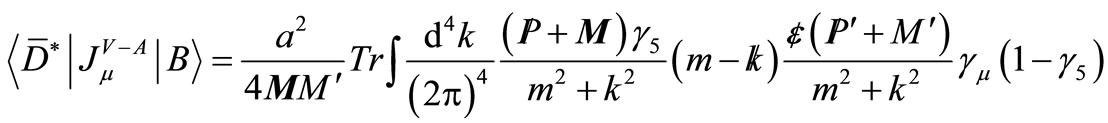 (12.2)
(12.2)
The integration of k is logarithmically divergent; therefore, it is regulated through the dimensional regularizetion. Performing this, and calculating the Trs, these equations can be easily written as the following forms:
 (13.1)
(13.1)
 (13.2)
(13.2)
In the normalization, the term of 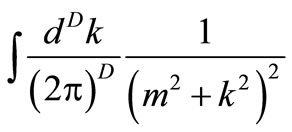 is removed by means of the Luke’s theorem, and it is not necessary to compute it. Hence, after the normalization, the structure functions at this state equal:
is removed by means of the Luke’s theorem, and it is not necessary to compute it. Hence, after the normalization, the structure functions at this state equal:
 (14)
(14)
This conclusion is predictable since the wave functions we chose were the static wave functions of extremely heavy quark; and as the HQET predicts for this state, the structure functions fulfill Equation (14) at the maximum recoil [7-14].
3.2. The Computation of Structure Functions Using the First-Order Bethe-Salpeter Wave Function
The zero-order wave functions (Equations (6.1) and (6.2)) are put into the first-order B-S equation with a confining kernel (Equation (10)) to obtain the first-order wave function (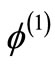 ) and actually to gain better results. Consequently, the first-order wave functions for mesons are as follows:
) and actually to gain better results. Consequently, the first-order wave functions for mesons are as follows:
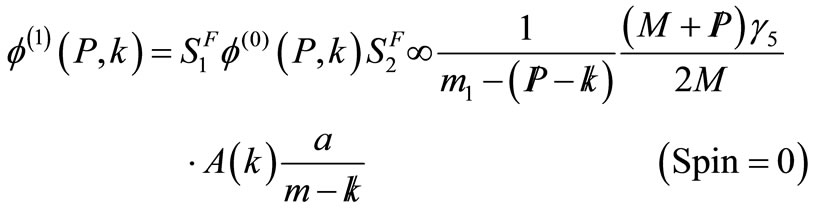 (15.1)
(15.1)
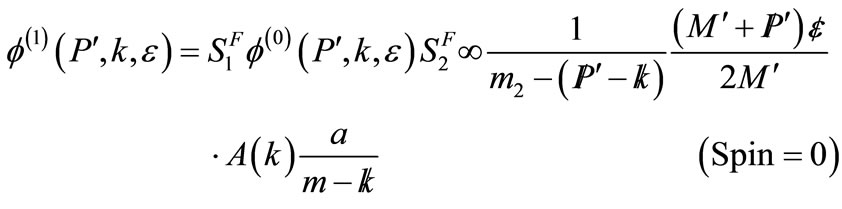 (15.2)
(15.2)
Through replacing the above equations with the matrixes of flow producing the 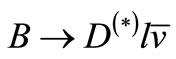 transitions and with regards to Equation (11), these matrixes are as follows:
transitions and with regards to Equation (11), these matrixes are as follows:
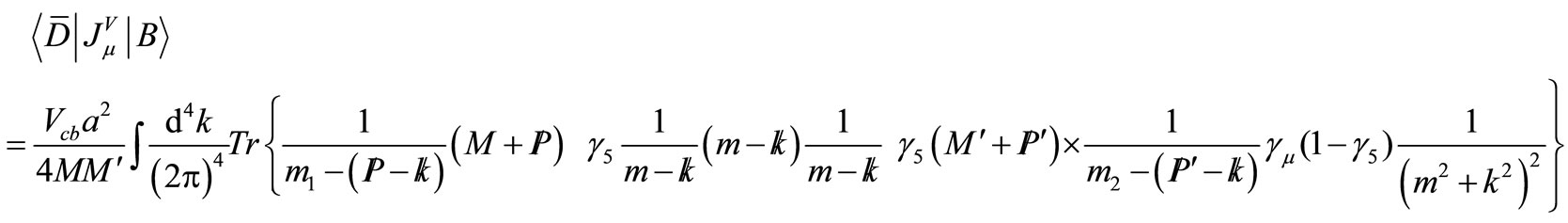 (16.1)
(16.1)
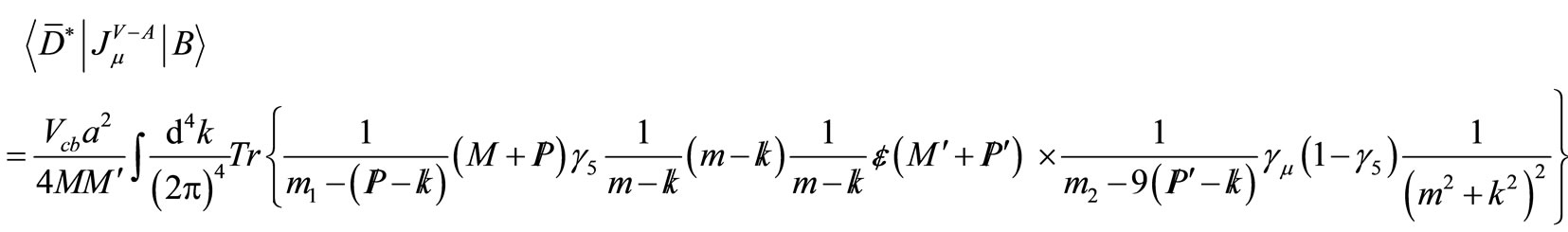 (16.2)
(16.2)
assuming that the heavy quarks are mass-shell:
 (17)
(17)
and through algebra the γs, they are simplified as the forms below:
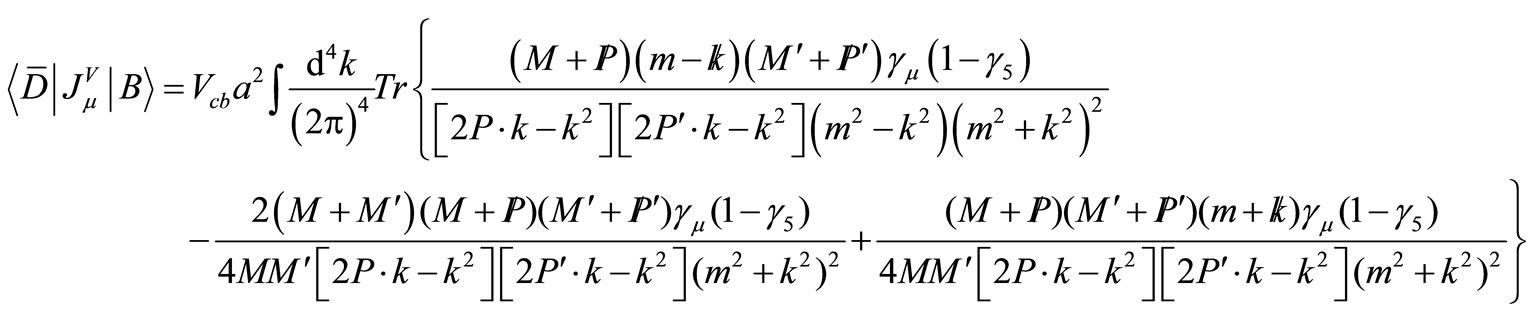 (18.1)
(18.1)
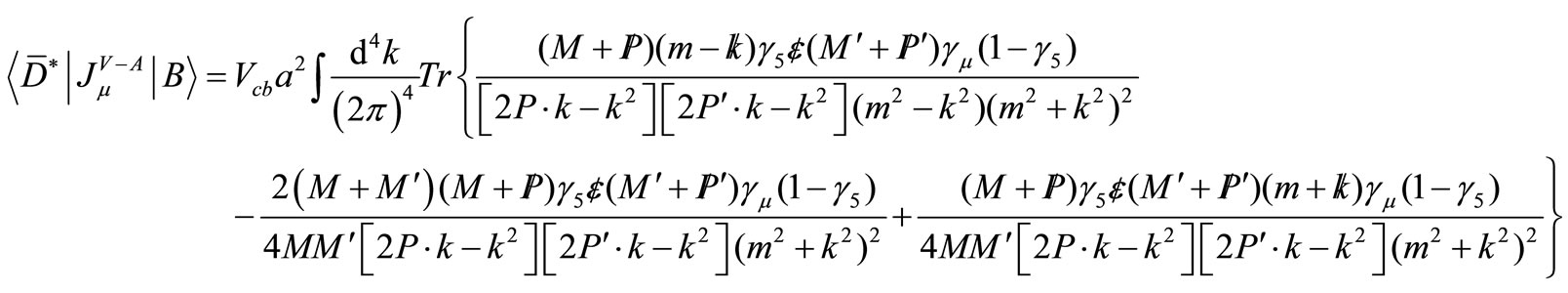 (18.2)
(18.2)
If the Feynman parameterization is applied:
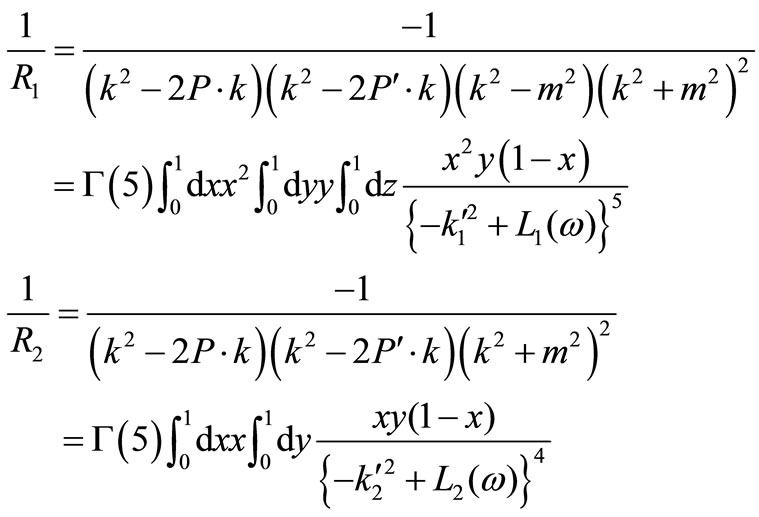 (19)
(19)
where the  s are the first and second (or third) fractions’ dominator. The
s are the first and second (or third) fractions’ dominator. The 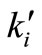 s and
s and 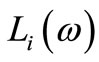 s are defined as follows:
s are defined as follows:
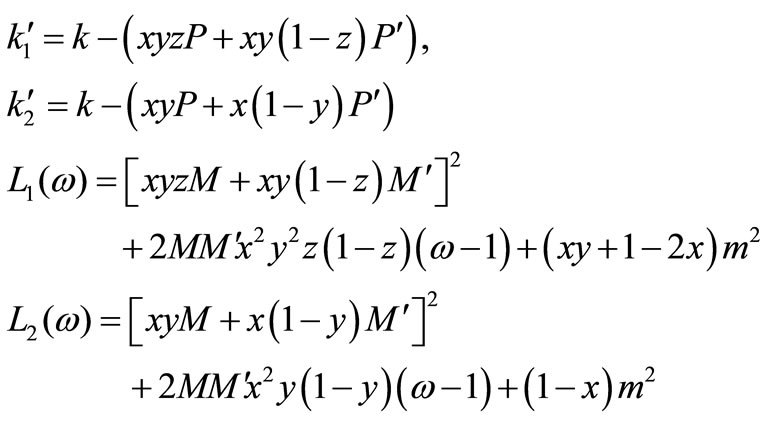 (20)
(20)
First, each of rejections in the integrand’s numerators (Equations (18.1) and (18.2)) is calculated. The numerator of the first fraction of Equation (18.1) equals:
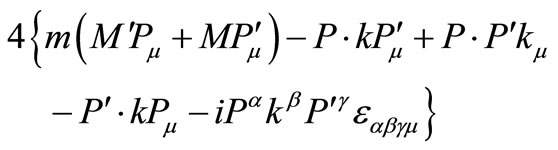 (21.1)
(21.1)
and the numerator of the first fraction of Equation (18.2) equals:
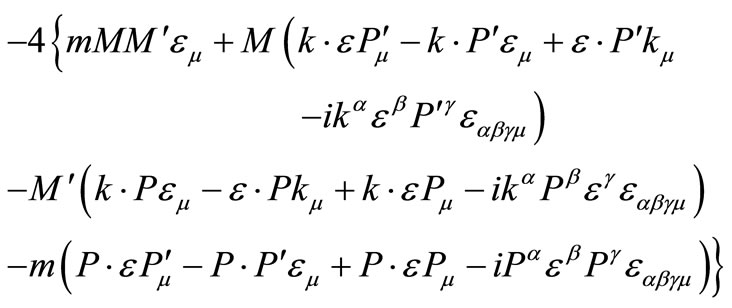 (21.2)
(21.2)
which through inputting 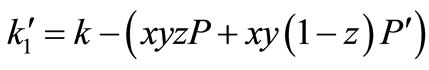 to the above equations, and removing the odd terms in proportion to
to the above equations, and removing the odd terms in proportion to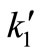 , they are respectively simplified as follows:
, they are respectively simplified as follows:
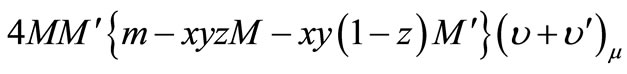 (22.1)
(22.1)
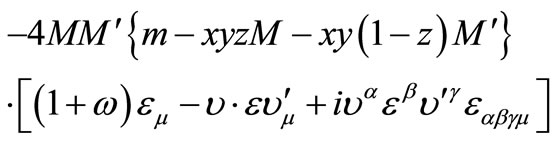 (22.2)
(22.2)
The second fractions’ numerators are easily achieved as the following forms:
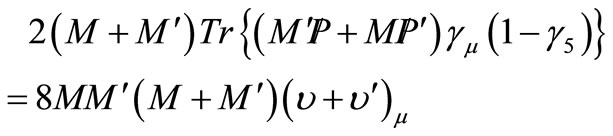 (23.1)
(23.1)
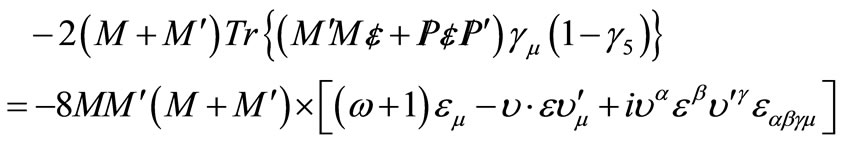 (23.2)
(23.2)
and the third fractions’ numerators are calculated as follows:
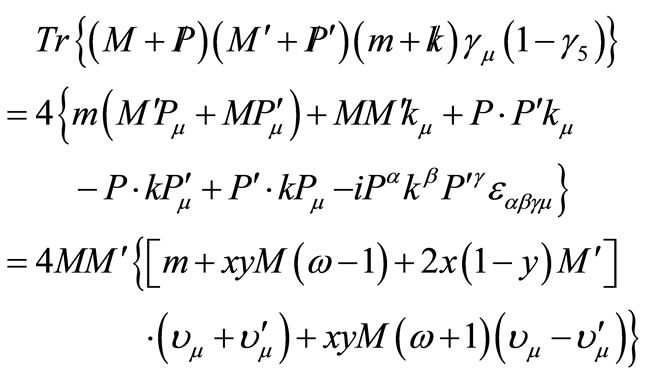 (24.1)
(24.1)
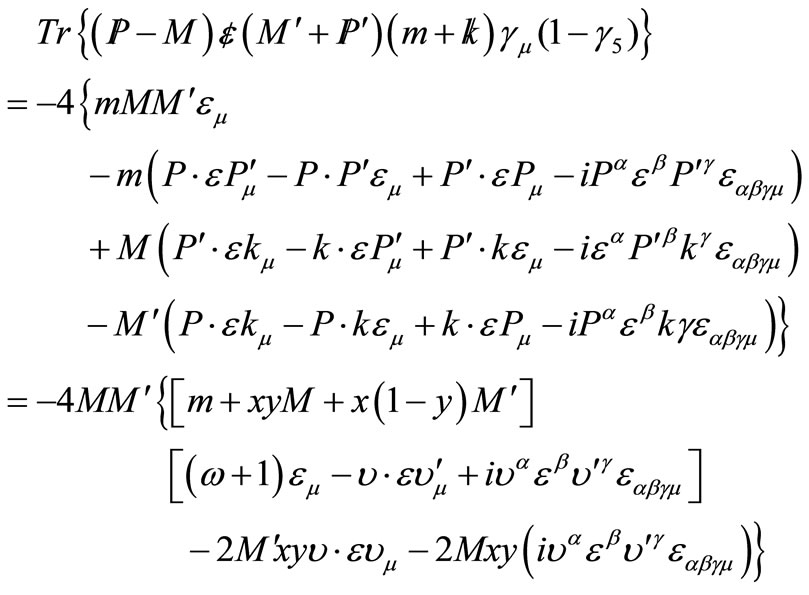 (24.2)
(24.2)
At the third step, the change of variable 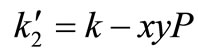
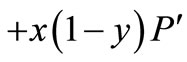 is applied to both above equations. Also, writing the odd terms with respect to
is applied to both above equations. Also, writing the odd terms with respect to 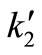 is neglected since the integration of them becomes zero. Therefore, the structure functions are transformed into the following forms:
is neglected since the integration of them becomes zero. Therefore, the structure functions are transformed into the following forms:
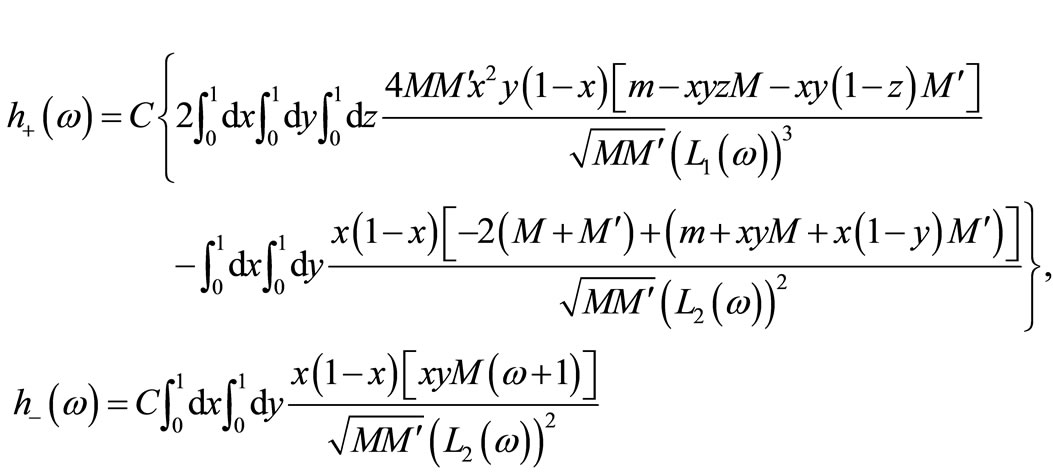 (25.1)
(25.1)
 (25.2)
(25.2)
where C and 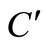 are the normalizing constants that are computed using the Luke’s theorem [15].
are the normalizing constants that are computed using the Luke’s theorem [15].
For the next computations, it is essential to have the structure functions as the functions of parameters without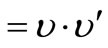
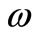 , while the structure functions achieved in Equations (25.1) and (25.2) are not only the functions of
, while the structure functions achieved in Equations (25.1) and (25.2) are not only the functions of 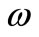 but also the functions of x and y which should be first integrated. However, since the Mathematica software is unable to parametrically solve these integrals, and also regarding the fact that the structure functions are normal merely up to
but also the functions of x and y which should be first integrated. However, since the Mathematica software is unable to parametrically solve these integrals, and also regarding the fact that the structure functions are normal merely up to  order, before the integration of x and y, they are expanded according to
order, before the integration of x and y, they are expanded according to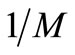 , and then x and y are integrated.
, and then x and y are integrated.
Generally, the product of 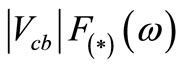 is determined as a function of
is determined as a function of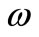 ; and
; and  is obtained through extrapolating the data at the recoil of zero (
is obtained through extrapolating the data at the recoil of zero (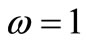 ) (
) (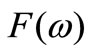 and
and 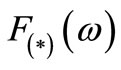 are a combination of
are a combination of 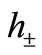 and
and  functionsrespectively). For this purpose, the values of
functionsrespectively). For this purpose, the values of 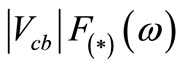 for various
for various 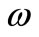 s are empirically achieved, a linear or square curve is fitted to them, and thereby obtaining
s are empirically achieved, a linear or square curve is fitted to them, and thereby obtaining 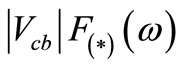 at
at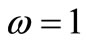 ; consequently,
; consequently, . Therefore, the structure functions’ slope and curve achieved theoretically are of importance. Accordingly, the main reason of inaccuracy in the theoretical computations for determining
. Therefore, the structure functions’ slope and curve achieved theoretically are of importance. Accordingly, the main reason of inaccuracy in the theoretical computations for determining 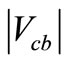 pertains to the value of
pertains to the value of  and the shape of
and the shape of  curve used for fitting the laboratory data. In other words, various values of slope and curve result in different values of
curve used for fitting the laboratory data. In other words, various values of slope and curve result in different values of . Hence, the structure functions are also written as an expansion around
. Hence, the structure functions are also written as an expansion around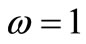 . Since the ω’s range of values available is narrow (
. Since the ω’s range of values available is narrow ( ), the expansion of structure functions around
), the expansion of structure functions around 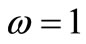 is logical [16]:
is logical [16]:
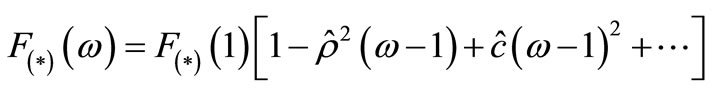 (26)
(26)
The slope 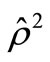 and the curve
and the curve 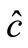 are considered as parameter.
are considered as parameter.
4. Conclusion
The results obtained using the zero-order Bethe-Salpeter wave function are similar to the results the HQET predicts for extremely heavy quark at the maximum recoil. Table 1 provides the results achieved using the first-order B-S wave function, some of empirical values, and the results obtained through other theoretical methods. As can be observed, the results acquired by means of the first-order B-S wave function for heavy-to-heavy transitions are appropriately consistent with the empirical values and values obtained in other theoretical methods.
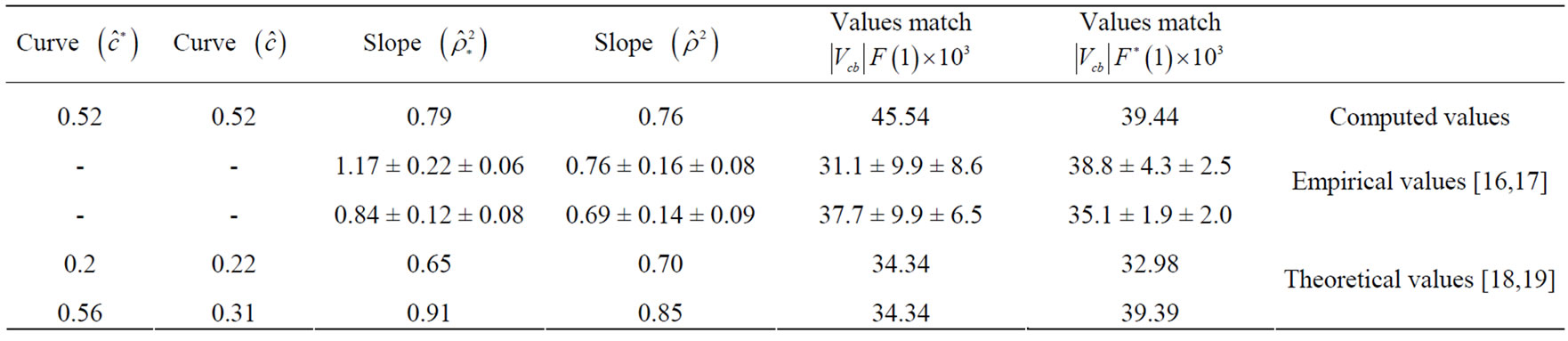
Table 1. The slope and curve values of 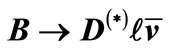 transition’s structure functions (Vcd = 0.039 for
transition’s structure functions (Vcd = 0.039 for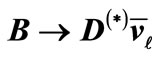 , Vcd = 0.034 for
, Vcd = 0.034 for ).
).
5. Acknowledgement
The work described in this paper was fully supported by grants from the Institute for Advanced Studies of Iran. The authors would like to express genuinely and sincerely thanks and appreciated and their gratitude to Institute for Advanced Studies of Iran.
REFERENCES
- F. Hussain, J. G. Körner and G. Thompson, “Relativistic SU(6) Wave Functions as the Basis of Modern Approaches to Hadronic Wave Functions,” Annals of Physics, Vol. 206, No. 2, 1991, p. 255. doi:10.1016/0003-4916(91)90228-Z
- F. Hussain, J. G. Körner and G. Thompson, “Relativistic SU(6) Wave Functions as the Basis of Modern Approaches to Hadronic Wave Functions,” Annals of Physics, Vol. 206, No. 2, 1991, pp. 334-367. doi:10.1016/0003-4916(91)90004-R
- F. Hussain, J. G. Körner, K. Schilcher, G. Thompson and Y. L. Wu, “Heavy-Light Hadrons and Current-Induced Transitions among Them,” Physics Letters B, Vol. 249, No. 2, 1990, pp. 295-302. doi:10.1016/0370-2693(90)91259-E
- A. Yu. Umnikov, F. C. Khanna and L. P. Kaptari, “Deep Inelastic Scattering on the Deuteron in the Bethe-Salpeter Formalism. II. Realistic NN Interaction,” Physical Review C, Vol. 56, No. 4, 1997, pp. 1700-1719. doi:10.1103/PhysRevC.56.1700
- A. Yu. Umnikov, F. C. Khanna and L. P. Kaptari, “On the Unfolding of the Neutron Structure Functions from the Deuteron Data,” Zeitschrift für Physik a Hadrons and Nuclei, Vol. 348, No. 3, 1994, pp. 211-215. doi:10.1007/BF01291919
- M. Neubert, “A Virial Theorem for the Kinetic Energy of a Heavy Quark Inside Hadrons,” Physical Review C, Vol. 53, No. 1, 1996, pp. 377- 384. doi:10.1103/PhysRevC.53.377
- M. Neubert, “A Virial Theorem for the Kinetic Energy of a Heavy Quark Inside Hadrons,” Physics Letters B, Vol. 322, No. 4, 1994, pp. 419-424. doi:10.1016/0370-2693(94)91174-6
- M. Neubert, “Heavy-Quark Symmetry,” Physics Reports, Vol. 245, No. 5-6, 1994, pp. 259-395. doi:10.1016/0370-1573(94)90091-4
- M. Neubert, “Analysis of the Photon Spectrum in Inclusive B→Xsγ Decays,” Physical Review D, Vol. 49, No. 9, 1994, pp. 4623-4633. doi:10.1103/PhysRevD.49.4623
- M. Neubert, “QCD-Based Interpretation of the Lepton Spectrum in Inclusive B¯
 Xulν¯ Decays,” Physical Review D, Vol. 49, No. 9, 1994, pp. 3392-3398. doi:10.1103/PhysRevD.49.3392
Xulν¯ Decays,” Physical Review D, Vol. 49, No. 9, 1994, pp. 3392-3398. doi:10.1103/PhysRevD.49.3392 - M. Neubert, “Theoretical Update on the Model-Independent Determination of |Vcb| Using Heavy Quark Symmetry,” Physics Letters B, Vol. 338, No. 1, 1994, pp. 84-91. doi:10.1016/0370-2693(94)91348-X
- M. Neubert and V. Rieckert, “New Approach to the Universal Form Factors in Decays of Heavy Quarks,” Nuclear Physics B, Vol. 382, No. 1, 1992, pp. 97-119. doi:10.1016/0550-3213(92)90080-U
- M. Neubert and B. Stech, “Quark-Quark Correlations and the λI = 1/2 Rule,” Physics Letters B, Vol. 231, No. 4, 1989, pp. 477-482. doi:10.1016/0370-2693(89)90697-7
- B. Stech, “Form Factor Relations for Heavy-to-Light Transitions,” Physics Letters B, Vol. 354, No. 3, 1995, pp. 447- 452. doi:10.1016/0370-2693(95)00661-4
- M. E. Luke, “Effects of Subleading Operators in the Heavy Quark Effective Theory,” Physics Letters B, Vol. 252, No. 3, 1990, pp. 447-455. doi:10.1016/0370-2693(90)90568-Q
- B. Grinstein and Z. Ligeti, “Heavy Quark Symmetry in
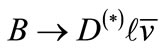 Spectra,” Physics Letters B, Vol. 526, No. 1, 2002, pp. 345-354. doi:10.1016/S0370-2693(01)01517-9
Spectra,” Physics Letters B, Vol. 526, No. 1, 2002, pp. 345-354. doi:10.1016/S0370-2693(01)01517-9 - M. Artuso and E. Barberio, “Present Status of Experimental Determination of Vcb,” High Energy Physics-Phenomenology, 2002, arXiv:hep-ph/0205163v1.
- D. Merten, R. Ricken, M. Koll, B. Metsch and H. Petry, “Instanton Effects in Meson Spectroscopy,” Czechoslovak Journal of Physics, Vol. 51, Suppl. 1, 2002, pp. B152- B159. doi:10.1007/s10582-001-0053-1
- D. Merten, R. Ricken, M. Koll, B. Metsch and H. Petry, “Weak Decays of Heavy Mesons in a Covariant Quark Model,” The European Physical Journal A, Vol. 13, No. 4, 2002, pp. 477-491. doi:10.1140/epja/iepja1357
NOTES
*Corresponding author.

