Journal of Applied Mathematics and Physics
Vol.04 No.08(2016), Article ID:70175,13 pages
10.4236/jamp.2016.48173
Forward (D) and Backward (Ñ) Difference Operators Basic Sets of Polynomials in 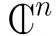 and Their Effectiveness in Reinhardt and Hyperelliptic Domains
and Their Effectiveness in Reinhardt and Hyperelliptic Domains
Saheed Abayomi Akinbode, Aderibigbe Sheudeen Anjorin
Lagos State University, OJO (LASU), Apapa, Nigeria

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).



Received 7 July 2016; accepted 26 August 2016; published 29 August 2016
ABSTRACT
We generate, from a given basic set of polynomials in several complex variables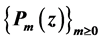 , new basic sets of polynomials
, new basic sets of polynomials 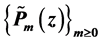 and
and 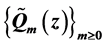 generated by the application of the Ñ and D operators to the set
generated by the application of the Ñ and D operators to the set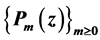 . All relevant properties relating to the effectiveness in Reinhardt and hyperelliptic domains of these new sets are properly deduced. The case of classical orthogonal polynomials is investigated in details and the results are given in a table. Notations are also provided at the end of a table.
. All relevant properties relating to the effectiveness in Reinhardt and hyperelliptic domains of these new sets are properly deduced. The case of classical orthogonal polynomials is investigated in details and the results are given in a table. Notations are also provided at the end of a table.
Keywords:
Effectiveness, Cannon Condition, Cannon Sum, Cannon Function, Reinhardt Domain, Hyperelliptic Domain

1. Introduction
Recently, there has been an upsurge of interest in the investigations of the basic sets of polynomials [1] - [27] . The inspiration has been the need to understand the common properties satisfied by these polynomials, crucial to gaining insights into the theory of polynomials. For instance, in numerical analysis, the knowledge of basic sets of polynomials gives information about the region of convergence of the series of these polynomials in a given domain. Namely, for a particular differential equation admitting a polynomial solution, one can deduce the range of convergence of the polynomials set. This is an advantage in numerical analysis which can be exploited to reduce the computational time. Besides, if the basic set of polynomials satisfies the Cannon condition, then their fast convergence is guaranteed. The problem of derived and integrated sets of basic sets of polynomials in several variables has been recently treated by A. El-Sayed Ahmed and Kishka [1] . In their work, complex variables in complete Reinhardt domains and hyperelliptical regions were considered for effectiveness of the basic set. Also, recently the problem of effectiveness of the difference sets of one and several variables in disc D(R) and polydisc 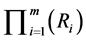 has been treated by A. Anjorin and M.N Hounkonnou [27] .
has been treated by A. Anjorin and M.N Hounkonnou [27] .
In this paper, we investigate the effectiveness, in Reinhardt and hyperelliptic domains, of the set of polynomials generated by the forward (D) and backward (Ñ) difference operators on basic sets. These operators are very important as they involve the discrete scheme used in numerical analysis. Furthermore, their composition operators form the most of second order difference equations of Mathematical Physics, the solutions of which are orthogonal polynomials [25] [26] .
Let us first examine here some basic definitions and properties of basic sets, useful in the sequel.
Definition 1.1 Let 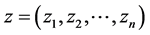 be an element of the space of several complex variables
be an element of the space of several complex variables  . The hyperelliptic region of radii
. The hyperelliptic region of radii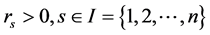 ), is denoted by
), is denoted by 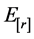 and its closure by
and its closure by 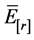 where
where
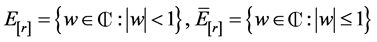
And
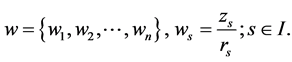
Definition 1.2 An open complete Reinhardt domain of radii 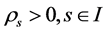 is denoted by
is denoted by 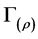 and its closure by
and its closure by
The unspecified domains 





Thus, the function 



where 

where 

t being the radius of convergence in the domain, 




where also, using the above function 




Hence, we have for the series


arbitrary near to
With 

Definition 1.3 A set of polynomials 


Thus, according to [4] , the set 











Thus, for the function 



is an associated basic series of F(z). Let 

Definition 1.4 A basic set satisfying the condition

Is called a Cannon basic set. If
Then the set is called a general basic set.
Now, let 






For any function 



For Reinhardt domains [24] ,

For hyperelliptic regions [1] .
2. Basic Sets of Polynomials in 
Now, we define the forward difference operator D acting on the monomial 
where E is the shift operator and 
So, considering the monomial
Hence
Since
Hence
where 

Ñ acting on the monomial 

Equivalently, in terms of lag operator L defined as


The Cannon functions for the basic sets of polynomils in complete Reinhardt domain and in hyperelliptical regions [1] , are defined as follows, respectively:
Concerning the effectiveness of the basic set 
Theorem 2.1 A necessary and sufficient condition [7] for a Cannon set 
1. effective in 

2. effective in 

Theorem 2.2 The necessary and sufficient condition for the Cannon basic set 
several complex variables to be effective [1] in the closed hyperelliptic 


The Cannon basic set 
if and only if

So, considering the monomial
Let’s prove the following statement:
Theorem 2.3 The set of polynomials 
Are basic.
Proof: To prove the first part of this theorem, it is sufficient to to show that the initial sets of polynomials 




For at least one i,
Hence, it follows that



In general, given any polynomial 
Hence the representation is unique. So, the set 
Theorem 2.4 The Cannon set 

in the closed complete Reinhardt domain 

Proof: In a complete Reinhardt domain for the forward difference operator D, the Cannon sum of the monomial 
Then
where 
which implies that
Then the Cannon function
But
Similarly, for the backward difference operator Ñ, the Cannon sum
Then
where 

But
Hence, we deduce that
Theorem 2.5 If the Cannon basic set 







be effective in
The Cannon sum 


where 
where 
where
So, by similar argument as in the case of Reinhardt domain we obtain
where

Such that the Cannon function writes as
But
Since the Cannon function is non-negative. Hence
3. Examples
Let us illustrate the effectiveness in Reinhardt and hyperelliptic domains, taking some examples. First, suppose that the set of polynomials 
Then
Hence
which implies
for

Now consider the new polynomial from the polynomial defined above:
Hence by Theorem 2.4,
where
where 
The Cannon function
which implies
where
and
Hence
Similarly, for the operator Ñ, we have
Since
Then
Table 1. Region of effectiveness: (1) Disc


Implication: The new sets are nowhere effective since the parents sets are nowhere effective. By changing



same condition of effectiveness as in Reinhart domain for both operators D and Ñ in the hyperelliptic domain.
The following notations are relevant to the table below.




Finally, for the classical orthogonal polynomials, the explicit results of computation are given in a Table 1 below.
Thus, in this paper, we have provided new sets of polynomials in C, generated by Ñ and D operators, which satisfy all properties of basic sets related to their effectiveness in specified regions such as in hyperelliptic and Reinhardt domains. Namely, the new basic sets are effective in complete Reinhardt domain as well as in closed Reinhardt domain. Furthermore, we have proved that if the Cannon basic set 

Cite this paper
Saheed Abayomi Akinbode,Aderibigbe Sheudeen Anjorin, (2016) Forward (Δ) and Backward (∇) Difference Operators Basic Sets of Polynomials in ![]() and Their Effectiveness in Reinhardt and Hyperelliptic Domains. Journal of Applied Mathematics and Physics,04,1630-1642. doi: 10.4236/jamp.2016.48173
and Their Effectiveness in Reinhardt and Hyperelliptic Domains. Journal of Applied Mathematics and Physics,04,1630-1642. doi: 10.4236/jamp.2016.48173
References
- 1. El-Sayed Ahmed, A. and Kishka, Z.M.G. (2003) On the Effectiveness of Basic Sets of Polynomials of Several Complex Variables in Elliptical Regions. Proceedings of the Third International ISAAC Congress, 1, 265-278.
- 2. Anjorin, A. and Hounkonnou, M.N. (2006) Effectiveness of the Difference Sets of One and Several Variables in Disc D(R) and Polydisc. (ICMPA) Preprint 07.
- 3. Lorente, M. (2001) Raising and Lowering Operators, Factorization and Differential/Difference Operators of Hypergeometric Type. Journal of Physics A: Mathematical and General, 34, 569-588.
http://dx.doi.org/10.1088/0305-4470/34/3/316 - 4. Hounkonnou, M.N., Hounga, C. and Ronveaux, A. (2000) Discrete Semi-Classical Orthogonal Polynomials: Generalized Charlier. Journal of Computational and Applied Mathematics, 114, 361-366.
http://dx.doi.org/10.1016/S0377-0427(99)00275-7 - 5. Kishka, Z.M.G. and El-Sayed Ahmed, A. (2003) On the Order and Type of Basic and Composite Sets of Polynomials in Complete Reinhardt Domains. Periodica Mathematica Hungarica, 46, 67-79.
http://dx.doi.org/10.1023/A:1025705824816 - 6. Kishka, Z.M.G. (1993) Power Set of Simple Set of Polynomials of Two Complex Variables. Bulletin de la Société Royale des Sciences de Liège, 62, 361-372.
- 7. New, W.F. (1953) On the Representation of Analytic Functions by Infinite Series. Philosophical Transactions of the Royal Society A, 245, 439-468.
- 8. Kumuyi, W.F. and Nassif, M. (1986) Derived and Integrated Sets of Simple Sets of Polynomials in Two Complex Variables. Journal of Approximation Theory, 47, 270-283.
http://dx.doi.org/10.1016/0021-9045(86)90018-3 - 9. Boss, R.P. and Buck, R.C. (1986) Polynomial Expansions of Analytic Functions II. In: Ergebinsse der Mathematika Hung ihrer grenz gbicle. Neue Foge., Vol. 19 of Moderne Funktionetheorie, Springer Verlag, Berlin.
- 10. Makar, R.H. and Wakid, O.M. (1977) On the Order and Type of Basic Sets of Polynomials Associated with Functions of Trices. Periodica Mathematica Hungarica, 8, 3-7.
http://dx.doi.org/10.1007/BF02018040 - 11. Makar, R.H. (1954) On Derived and Integral Basic Sets of Polynomials. Proceedings of the American Mathematical Society, 5, 218-225.
- 12. Mikhall, N.N. and Nassif, M. (1954) On the Differences and Sum of Simple Sets of Polynomials. Assiut Universit Bulletin of Science and Technology. (In Press)
- 13. Metwally, M.S. (1999) Derivative Operator on a Hypergeometric Function of the Complex Variables. Southwest Journal of Pure and Applied Mathematics, 2, 42-46.
- 14. Nasif, M. and Adepoju, J.A. (1984) Effectiveness of the Product of Simple Sets of Polynomials of Two Complex Variables in Polycylinders and in Faber Regions. Journal of Natural Sciences and Mathematics, 24, 153-171.
- 15. Nasif, M. (1971) Composite Sets of Polynomials of Several Complex Variables. Publicationes Mathematics Debrecen, 18, 43-53.
- 16. Mursi, M. and Makar, B.H. (1955) Basic Sets of Polynomials of Several Complex Variables ii. The Second Arab Sci. Congress, Cairo, 61-68.
- 17. Mursi, M. and Makar, B.H. (1955) Basic Sets of Polynomials of Several Complex Variables i. The Second Arab Sci. Congress, Cairo, 51-60.
- 18. Abul-Dahab, M.A., Saleem, A.M. and Kishka, Z.M. (2015) Effectiveness of Hadamard Product of Basic Sets of Polynomials of Several Complex Variables in Hyperelliptical Regions. Electronic Journal of Mathematical Analysis and Applications, 3, 52-65.
- 19. Abdul-Ez, M.A. (1996) On the Representation of Clifford Valued Functions by the Product System of Polynomials. Journal of Complex Variables and Applications, 29, 97-105.
- 20. Abdul-Ez, M.A. and Sayed, K.A.M. (1990) On Integral Operators Sets of Polynomials of Two Complex Variables. Bug. Belg. Math. Soc. Simon Stevin, 64, 157-167.
- 21. Abdul-Ez, M.A. and Constales, D. (1990) Basic Sets of Polynomials in Clifford Analysis. Journal of Complex Variables and Applications, 14, 177-185.
- 22. Sayed, K.A.M. and Metwally, M.S. (1998) Effectiveness of Similar Sets of Polynomials of Two Complex Variables in Polycylinders and in Faber Regions. International Journal of Mathematics and Mathematical Sciences, 21, 587-593.
- 23. Sayed, K.A.M. (1975) Basic Sets of Polynomials of Two Complex Variables and Their Convergences Properties. Ph D Thesis, Assiut University, Assiut.
- 24. Whittaker, J.M. (1949) Sur les séries de bases de polynômes quelconques. Quaterly Journal of Mathematics (Oxford), 224-239.
- 25. Adepoju, J.A. and Nassif, M. (1983) Effectiveness of Transposed Inverse Sets in Faber Regions. International Journal of Mathematics and Mathematical Sciences, 6, 285-295.
- 26. El-Sayed Ahmed, A. (2006) Extended Results of the Hadamard Product of Simple Sets of Polynomials in Hypersphere. Annales Societatis Mathematicae Polonae, 2, 201-213.
- 27. El-Sayed Ahmed, A. (2013) On the Convergence of Certain Basic Sets of Polynomials. Journal of Mathematics and Computer Science, 3, 1211-1223.
Appendix
Key Notations
1) 
2) 
3) 
4) 
5) 
6) 
7) 
8) 
9)
10)
11)
where 






Submit or recommend next manuscript to SCIRP and we will provide best service for you:
Accepting pre-submission inquiries through Email, Facebook, LinkedIn, Twitter, etc.
A wide selection of journals (inclusive of 9 subjects, more than 200 journals)
Providing 24-hour high-quality service
User-friendly online submission system
Fair and swift peer-review system
Efficient typesetting and proofreading procedure
Display of the result of downloads and visits, as well as the number of cited articles
Maximum dissemination of your research work
Submit your manuscript at: http://papersubmission.scirp.org/








































































