Received 30 November 2015; accepted 27 March 2016; published 30 March 2016

ABSTRACT

In this study, we develop an SIS model for two types of mosquitoes, a traditional one and one that is resistant to IRS and ITNs. The resistant mosquito develops behavioral adaptation to control measures put in place to reduce their biting rate. They also bite early before dusk and later after dark when people are outside the houses and nets. We determine the effect of the two types of mosquitoes on malaria transmission in Kenya. The basic reproduction number 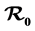is established as a sharp threshold that determines whether the disease dies out or persists in the population. Precisely, if 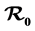≤ 1, the disease-free equilibrium is globally asymptotically stable and the disease always dies out and if 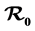> 1, there exists a unique endemic equilibrium which is globally stable and the disease persists. The contribution of the two types of mosquitoes to the basic reproduction number and to the level of the endemic equilibrium is analyzed.

Keywords:

Malaria, Insecticide Resistance, ITNs, IRS, Reproduction Number

1. Introduction

Malaria is one of the leading causes of morbidity and mortality in Kenya and it kills an estimated 34,000 children under five every year. Economically, it is estimated that 170 million working days in Kenya are lost each year because of malaria illness.

http://kenya.usaid.gov/programs/health/72.

After 1990, pyrethroids were promoted as insecticides of choice especially for Insecticides Treated Nets (ITNs) and Indoor Residual Spray (IRS) [1] [2] , due to high efficacy, rapid rate of knockdown, strong mosquito excito-repellence and low mammalian toxicity [3] .

In Kenya, ITNs have mainly been distributed to pregnant women and children under 5 years old by the Kenya Ministry of Health and non-governmental organizations [4] [5] . Currently, ITN coverage for children under 5 years old has increased rapidly from 7% in 2004 to 67% in 2006; this increase has been associated with a 44% reduction in malaria deaths [6] . However there is an increasing case resistance of mosquitoes to pyrethroid. The likely zoonotic nature of P. falciparum and the behavioral changes of mosquitoes are many new features which indicate that malaria control is not yet achieved [7] - [9] . The gains made from ITNs and IRS therefore are threatened by the development of physiological or behavioral resistance in the malaria vectors, which is widely documented [10] . Anopheline mosquitoes exhibit two major mechanisms of pyrethroid resistance, they are:

1) Increased level of metabolic detoxification of the insecticide,

2) Reduced sensitivity in the target sites of the insecticide. The target site of the pyrethroids is the voltage- gated sodium channel.

The second type of resistance is caused when a point mutation in the region II of the para-type sodium channel genes causes a change in affinity between the insecticide and its binding site or the sodium channel, and it induces a phenotype termed knock-down resistance (KDR) in a range of insecticides [11] - [14] . Insensitivity at the sodium channel target site also leads to cross-resistance between different classes of insecticides [15] .

Reports from literature confirm that use of ITNs and IRS has led to a substantial reduction in mosquitoes, reduced malaria transmission and a 44% reduction in malaria deaths [16] - [18] . However, although there was a global reduction in overall malaria transmission, 57% of the population continued to live in areas where transmission remained moderate to intense in Africa [19] . The ITNs and IRS intervention can reduce malaria transmission by targeting mosquitoes when they feed upon sleeping humans and/or rest inside houses, livestock shelters or other man-made structures. Despite high coverage, malaria spreading mosquitoes can maintain robust transmission because they develop resistance hence limiting the achievable impact [20] [21] . High and patchy resistance to pyrethroid insecticide has been confirmed in the endemic region of western Kenya, leaving the government with limited option but to seek other control measures [7] .

In this study, we develop a mathematical model with two types of vectors, one which is sensitive to the insecticides and a resistant type which adapts easily and survives despite the two types of intervention. We assume that the An. fambiae and the An. fenestus mosquito species are either sensitive or resistant to insecticides.

In section 2 we develop the model and equations. In section 3 the basic properties of the model are shown for positive invariance and computation of the basic reproduction number is also done. Section 4, we show the local and global stability of the Disease Free Equilibrium and section 6 is the conclusion.

2. The Model Formulation and Equations

We shall subdivide the mosquito population in Western Kenya into the traditional (non resistant) group and the new resistant group. This new resistance type has been termed as a “super mosquito”, but for the sake of terminology, we shall refer to them generally as “resistant” mosquito vectors. We shall use the subscripts “n” to represent non resistant traditional vectors, while, “r” represents the resistant vectors.

In this model 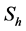will represent the susceptible human hosts while 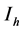will represent the infectious human population. The variable 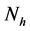representing the total human population will be given by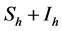. The non resistant susceptible (infectious) vectors will be represented by 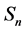(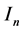), respectively, while the resistant vector population will be represented likewise as 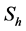(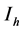), for the susceptible (infectious) population respectively. The total non resistant vector, therefore is given by 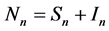and the resistant vector population by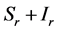. We shall use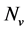, reservedly for the total resistant and non resistant vector populations respectively, hence.

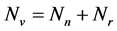When there are no malaria deaths, the host population dynamics is given by, and the total

human and mosquito population size approaches a carrying capacity for any non zero initial pop-

ulation size.

The non-resistant vector population defined by approaches a carrying capacity, while the resistant vector population approaches a carrying capacity.

Model assupmtions:

Ÿ the two types of vectors have different biting rates hence differentiated infectivity,

Ÿ the two types coexist and no vector changes status during the entire life span, i.e. not resistance vector becomes non-resistant or vice versa,

Ÿ The total vector and human populations are constant.

The following parameter symbols will be used in the equations:

Ÿ : The per capita rate of human birth,

Ÿ : The per capita rate birth rate of traditional vector and resistant vector respectively,

Ÿ : The proportion of infectious bites on hosts that produce a patent infection,

Ÿ : The proportion of bites by susceptible vectors on infectious hosts that produce a patent infection,

Ÿ : The per capita death rate for the human, traditional and resistant vectors, respectively,

Ÿ : Hosts rate of recovery,

Ÿ : The man biting rates of traditional and resistant vector, respectively.

The dynamics of our model will be governed by the following set of equations:

(1)

The term in the susceptible host’s compartment corresponds to a constant recruitment of susceptible hosts by natural birth.

The transmission term corresponds to frequency dependent infection of susceptible hosts by infectious non resistant mosquitoes, on infection they move to the infectious compartment.

The transmission term corresponds to frequency dependent infection of susceptible hosts by infectious resistant mosquitoes, on infection they also move to the infectious compartment.

The infected hosts who recover become susceptible again as malaria has no permanent immunity.

The last terms represents per capita deaths of the susceptible, infected hosts respectively.

In the susceptible vectors, represent the recruitment of susceptible non-resistant, (resistant) mosquitoes, respectively, by birth.

The term corresponds to the transmission of malaria to an susceptible non-resistant, (resistant) vectors, respectively, by an infected host.

Natural deaths affects all the groups as denoted by the parameter for the susceptible non- resistant, (resistant) vectors respectively, and for the infectious non-resistant, (resistant) vectors respectively.

Both resistant and non resistant vectors, once infected, are assumed to remain infected till death as mosquitoes do not recover or develop immunity from the parasite [22] [23] .

All the parameters in the model are non negative and the model equations are well posed.

Equation (1) is defined in feasible region

where denotes the non-negative cone of including its lower dimensional faces. It is clear that Ω is positively invariant with respect to (1). We denote the boundary and the interior of Ω by and respectively.

3. Well-Posedness of System

We use the relation and, and to reduce Equation (1), and therefore study the system

(2)

3.1. A Compact Positively Invariant Set

In this section we prove that the following set

is a positively invariant compact set for system (2) by barrier theorems (e.g. [24] [25] ). Moreover is a global attractor on the nonnegative orthant

Now we show that the vector field induced by the system is either tangent or entering on the boundary.

Ÿ ;

Ÿ when and we have.

Ÿ and;

Ÿ and;

Ÿ since we have;

Ÿ since we have;

Ÿ when and we have;

Ÿ when and we have;

We denote the demographic equilibria by, and.

The total human population is given by. In the absence of the disease and the equation becomes, which can be written as

The Integrating factor for this linear differential equation is given by

Thus

Integrating both sides we and applying the intial conditions () we have

The Equation for the Traditional non reistant mosquito is given by, which can be written as

The Integrating factor for this linear differential equation is given by

Thus

Integrating both sides we and applying the intial conditions () we have

Finally, the equation for the resistant mosquito given by, can be written as

The Integrating factor for this linear differential equation is given by

Thus

Integrating both sides we and applying the intial conditions () we have

Thus the feasible set for the model system (1) is given by, which is a positively invariant set. Hence the model is well posed and biologically meaningful.

3.2. Basic Reproduction Number

(3)

which can be simplified as

(4)

The expression is caled the basic reproduction number, with a biological meaning that is can be inter-

preted from terms under the square root sign. The first term, represents the number of secondary human infections caused by one infected resistant and one none resistant mosquito vector. The term represents the number of secondary mosquito infections caused by one infected human to an non-resistant vector, while represents the number of secondary infections to a resistant mosquito vector by a hu-

man host. The square root sign represent the two generations that the disease has to undergo from a mosquito to a human being and to a mosquito again or vice versa for the infection to take place. It is a number that determines the threshold for disease spread, as well as a control tool that whose parameters can be targeted for control.

4. Stability of Disease-Free Equilibrium Solution

Jacobian evaluated at disease-free equilibrium solution:

Characteristic polynomial:

(5)

where

it is easy to show that

which is positive if.

This means all the roots of the polynomial equations are negative, hence the system is locally asymptotically stable.

Global Stability of the DFE

The local dynamics of a general SIS and SI model is determined by the reproduction number. If, then each infected individual in its entire period of infectiousness will produce less than one infected individual on average. This means that the disease will be wiped out of the population. If, then each infected individual in its entire infectious period having contact with susceptible individuals will produce more than one infected individual implying that the disease persists in the population. If, and this is defined as the disease threshold, then one individual infects one more individual. For the disease free equilibrium is locally asymptotically stable while for the disease free equilibrium becomes unstable. By using the theory of Lasalle-Lyapunov function V, we will show the global asymptotic stability. The disease free equilibrium point is.

Theorem

If, then the disease-free equilibrium of the system is globally asymptotically stable on.

Proof

We construct the following Lasalle-Lyapunov function on the positively invariant compact set. Thus on, is continuous and non negative.

We define

The system of ordinary differential equations given by Equation (2) can be written as

(6)

This can be written as where

and.

If we define

then the derivative along the trajectories is given by as

We define the set. The largest invariant set is contained in the set E

for which or or. Thus when and. If or and, then. Thus by Lasalle’s invariance principle the disease free equilibrium is globally asymptotically stable on.

5. The Endemic Equilibrium, EE

5.1. Local Stability of the Endemic Equilibrium, EE

Theorem

The endemic equilibrium, and is locally asymptotically stable on.

Proof

The system of equations 5 can also be expressed as follows when we let

(7)

The Jacobian computed at the endemic equilibrium using the relations given by Equation (6) can be expressed as:

To determine the stability of the endemic equilibrium, we use the Routh-Hurwitz stability criteria on the characteristic equation of a third degree polynomial given by. We say that is Hurwitz iff and.

The coefficient, = sum of the determinants of all the principal minors of

and.

The trace of J will be given as

iff

iff

To prove the Routh-Hurwitz stability criteria we compute to obtain

iff

The requirements of Routh-Hurwitz stability criteria are satisfied hence this proves that the endemic equilibrium is locally asymptotically stable.

5.2. Global Stability of the EE

Theorem

The endemic equilibrium is globally asymptotically stable on if.

Proof

We will prove the global stability of the Endemic Equilibrium by using the following Lyapunov function proposed by Cai and Li (2007). Thus we have:

Then the derivative of, obtained by direct calculation along the solution of is given by

(8)

Substituting the expressions of the model system into the equation 7 above we get

can be written as

(9)

where F represents the positive terms of the equation above and G represents the negative terms of the said equation. The expression of F and G are as follows:

Thus from equation 8 if then we obtain that. We have that if and only if, and.

We define the set. Therefore the largest compact invariant set is the singleton

set which is the endemic equilibrium. By Lasalle Invariance principle is globally asymptotically stable on.

NB: In an upcoming article, we include a human protection factor and the development of mosquito resistance during their life time. Wa also allow some resistant vectors to become sensitive to insecticides.

6. Conclusion

In this study, we formulated a malaria model representing the transmission of malaria by two types of vectors; the traditional mosquito which is sensitive to insecticides in ITNS and IRS, and a resistant type which is able to survive despite the control measures aimed at shortening their life span and limiting the biting rate. The basic reproduction number is determined as a contribution of the two types of vectors. The model is shown to be positively invariant, hence well posed. The Disease Free Equilibrium and the Endemic equilibrium are shown to be locally and globally asymptotically stable when and, respectively. The development of resistance in sensitive mosquitoes and the loss of resistance in resistant mosquitoes will be done in an upcoming article.

Acknowledgements

We wish to thank Calistus Ngonghala for the contribution and advise he gave during the formulation of this model.

Cite this paper

Josephine Wairimu,Marilyn Ronoh, (2016) Modeling Insecticide Resistance in Endemic Regions of Kenya. Applied Mathematics,07,542-555. doi: 10.4236/am.2016.76050

References