Applied Mathematics
Vol.4 No.3(2013), Article ID:28858,5 pages DOI:10.4236/am.2013.43072
The Conservation Laws and Stability of Fluid Waves of Permanent Form
Department of Mathematics Technological Institute, Altai State Technical University, Biisk, Russia
Email: bta@bti.secna.ru
Received December 13, 2012; revised January 15, 2013; accepted January 23, 2013
Keywords: Nekrasov Integral Equation; Kellogg Method; Successive Approximations Method; Wave Mechanical Energy; Wave Stability
ABSTRACT
The solution of Nekrasov’s integral equation is described. By means of this solution the wave kinetic, potential, and full mechanical energies are defined as functions of fluid depth and wavelength.The wave obey the laws of mass and energy conservation. It is found that for any constant depth of fluid the wavelength is bounded from above by a value denoted as maximal wavelength. At maximal wavelength 1) the maximum slope of the free surface of the wave exceeds 38˚ and the value 45˚ is supposed attainable, 2) the wave kinetic energy vanishes. The stability of a steady wave considered as a compound pendulum is analyzed.
1. Introduction
This paper is based on Nekrasov’s integral equation solution obtained in [1,2]. This solution allows us to find the profile and velocity of a gravitational wave, and the calculation of the wave kinetic and potential energy is possible. At fixed depth of fluid the solution of Nekrasov’s integral equation exists on a limited segment of wavelength 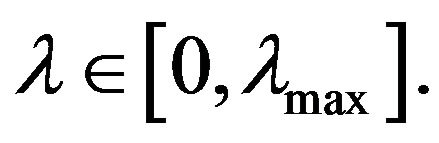 The potential and full mechanical energies are monotonically increasing functions on the segment
The potential and full mechanical energies are monotonically increasing functions on the segment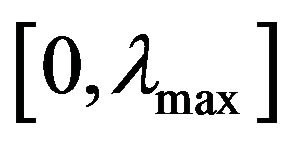 . From the law of the change of wave’s kinetic energy presented in [3] as mathematical theorem follows that the kinetic energy vanishes on the boundaries of the segment
. From the law of the change of wave’s kinetic energy presented in [3] as mathematical theorem follows that the kinetic energy vanishes on the boundaries of the segment 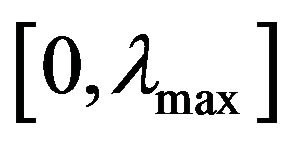 and has the maximum at a point
and has the maximum at a point 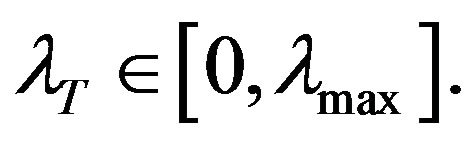 Thus we observe symmetry: at the points
Thus we observe symmetry: at the points 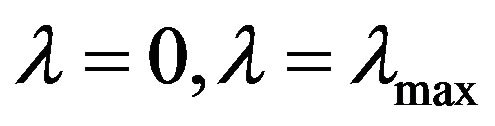 the full mechanical energy consists only of potential energy. The wave of constant shape may be considered as a compound pendulum with a suspension center in the origin of coordinates arranged on unperturbed surface of fluid.Then the wave stability is spotted as a compound pendulum stability.
the full mechanical energy consists only of potential energy. The wave of constant shape may be considered as a compound pendulum with a suspension center in the origin of coordinates arranged on unperturbed surface of fluid.Then the wave stability is spotted as a compound pendulum stability.
The plan of the paper is as follows. In Section 2, we describe the method of Nekrasov’s integral equation solution. Here this method has been used for evaluation of the maximum wavelength 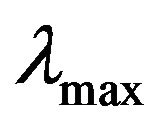 boundaries and for estimation of the maximum slope of the wave free surface.
boundaries and for estimation of the maximum slope of the wave free surface.
In Section 3, geometrical and energy properties of a wave are explored and the theorem about the change of the wave velocity on the segment 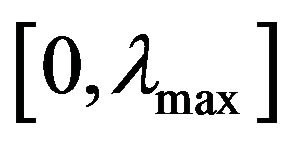 is proved. As a result we have gained the laws of the change of wave’s kinetic and potential energy. Here we have defined the wave’s center of mass as a function of depth-towavelength ratio and have made some suppositions concerning the wave stability considering it as a compound pendulum.
is proved. As a result we have gained the laws of the change of wave’s kinetic and potential energy. Here we have defined the wave’s center of mass as a function of depth-towavelength ratio and have made some suppositions concerning the wave stability considering it as a compound pendulum.
2. Solution of Nekrasov’s Integral Equation
Nekrasov’s integral equation describing steady state waves of unchangeable shape on the surface of a fluid with finite depth 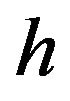 is written as [4]
is written as [4]
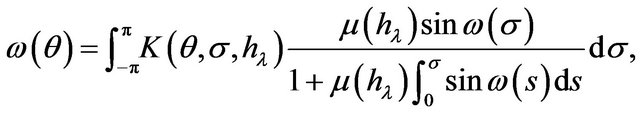 (1.1)
(1.1)
where  - is the polar angle,
- is the polar angle, 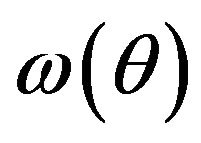 - is the angle that the wave surface makes with the horizontal,
- is the angle that the wave surface makes with the horizontal,  - is the wavelength,
- is the wavelength, 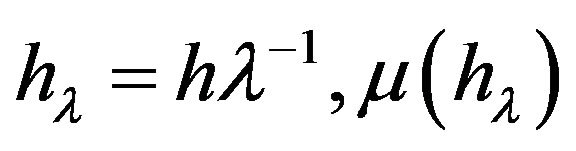 - is an arbitrary constant,
- is an arbitrary constant,
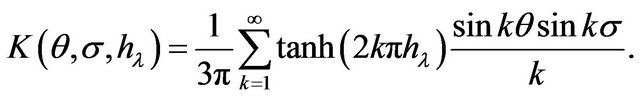
From Equation (1.1) follows that required function 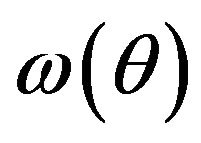 represents a trigonometric series
represents a trigonometric series
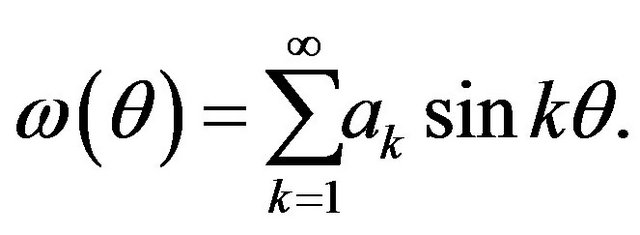 (1.2)
(1.2)
The evaluation of scalar product
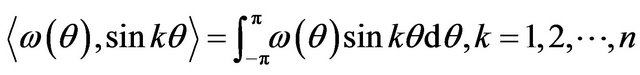
taking account of Equations (1.1) and (1.2) gives a system of 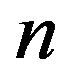 nonlinear integral equations
nonlinear integral equations
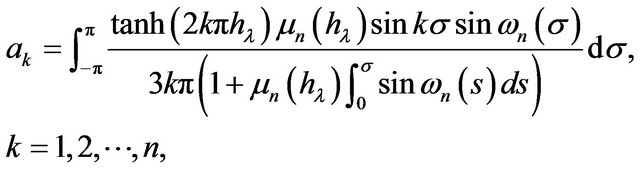 (1.3)
(1.3)
where the subscript 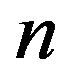 denotes that in Equation (1.1) a truncated kernel
denotes that in Equation (1.1) a truncated kernel  with
with 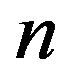 instead of
instead of  is used. Below the subscript
is used. Below the subscript  of any function will be omitted if
of any function will be omitted if
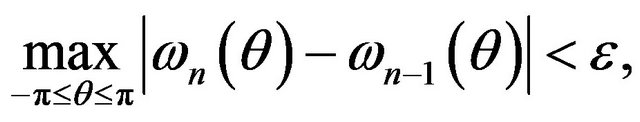 (1.4)
(1.4)
where 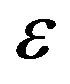 - is a suitable from accuracy point of view small number The system (1.3) containing
- is a suitable from accuracy point of view small number The system (1.3) containing 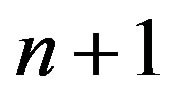 unknowns
unknowns 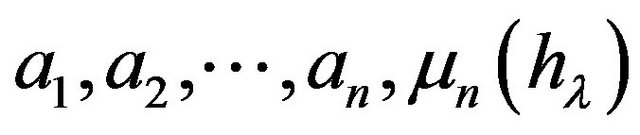 is underdetermined and has a set of solutions including the trivial.
is underdetermined and has a set of solutions including the trivial.
We assume that the first coefficient 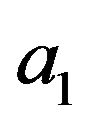 is independent of
is independent of 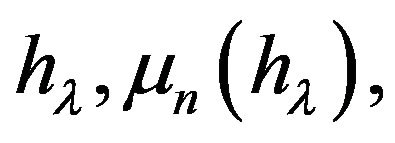 and
and 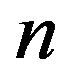 and can be calculated from the linearized on
and can be calculated from the linearized on 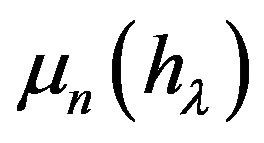 system (1.3) at
system (1.3) at 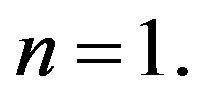 Thus
Thus
 satisfy the equation
satisfy the equation
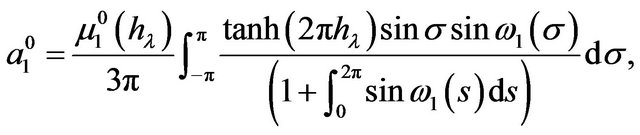 (1.5)
(1.5)
This equation has been solved by Kellogg’s method [5] in [1,2]. Let us remark that the Kellogg method was applied to a non-linear integral equation and the discovered solution is not spectral. In accordance with [2], Equation (1.5) has a unique solution (the motionless point) 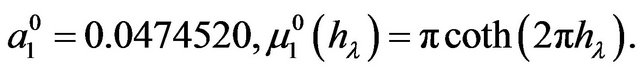
Now we fix in (1.3) 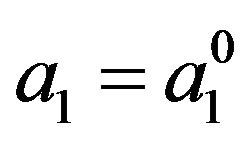 and consider
and consider 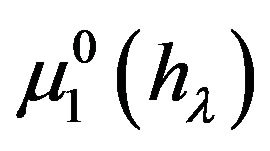 as the initial approximation of
as the initial approximation of 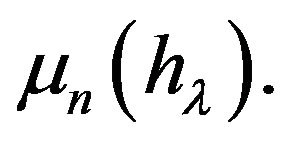 After that, the system of
After that, the system of 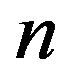 Equations (1.3) contains n unknowns
Equations (1.3) contains n unknowns  and has a solution that cannot be trivial.It has been shown [1,2] that coefficient
and has a solution that cannot be trivial.It has been shown [1,2] that coefficient 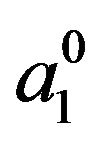 satisfy system (1.3) at
satisfy system (1.3) at 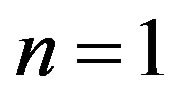 if
if
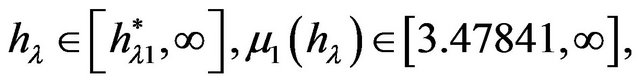 where
where
 (in [2]
(in [2] 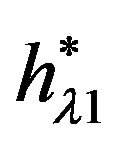 is designated as
is designated as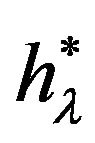 ).This means that the solution of the system (1.3) at
).This means that the solution of the system (1.3) at 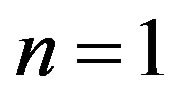 exists and is unique if the wavelength
exists and is unique if the wavelength 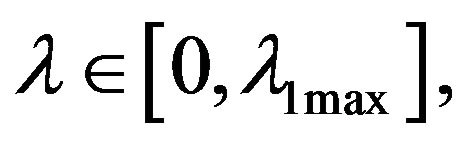
where 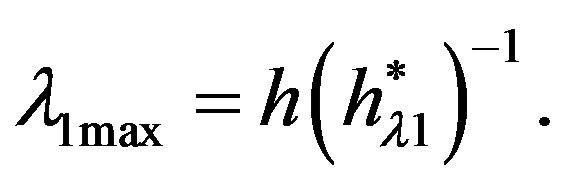
The lower boundary of the segment 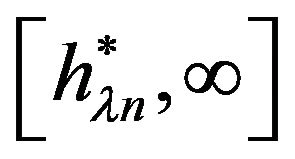 on which coefficient
on which coefficient 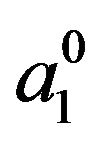 satisfy system (1.3) at
satisfy system (1.3) at 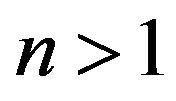 can be obtained by solving the system
can be obtained by solving the system
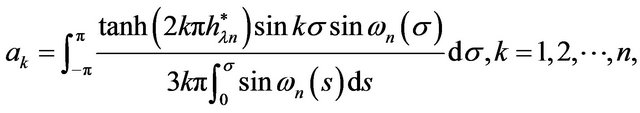
(1.6)
derived from (1.3) by substitution 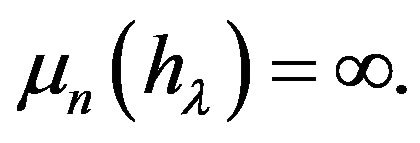 If requirements (1.4) are satisfied, then the function
If requirements (1.4) are satisfied, then the function 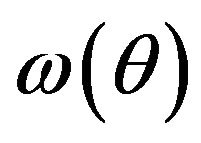 is defined on the segment
is defined on the segment 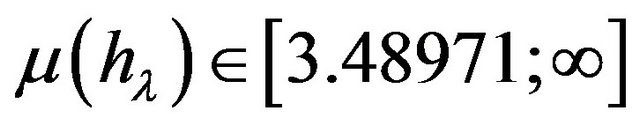 (see [1]), or on the equivalent segment
(see [1]), or on the equivalent segment
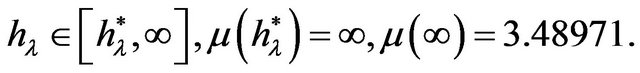 (1.7)
(1.7)
The system (1.6) containing n unknowns
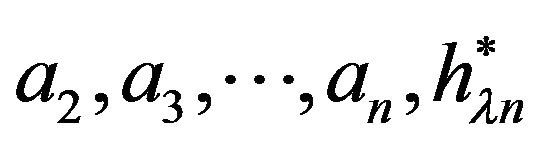 was solved by successive approximations method at
was solved by successive approximations method at 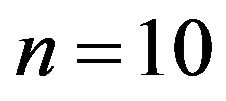 limited by computer’s throughput. The results of calculations of
limited by computer’s throughput. The results of calculations of 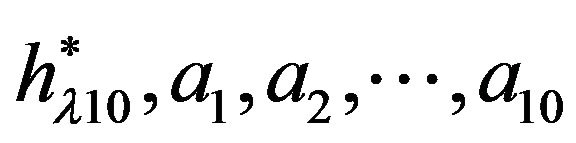 are given below:
are given below:

The function 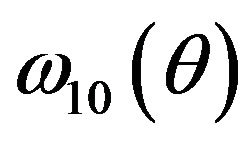 is of low accuracy at the point
is of low accuracy at the point 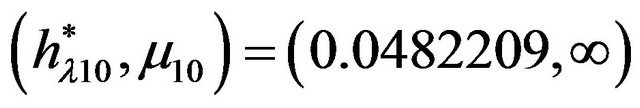 and allows only to write the inequalities
and allows only to write the inequalities 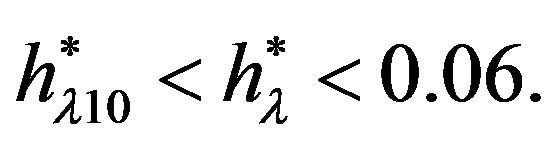 From these inequalities follows that the maximal wavelength
From these inequalities follows that the maximal wavelength  is bounded on both sides
is bounded on both sides 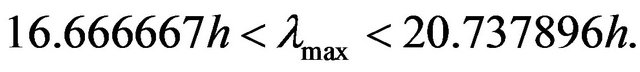
The results of calculations of 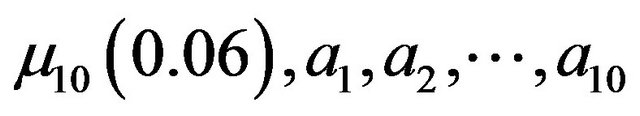 for
for 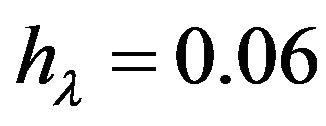 are given below:
are given below:

These numerical results assert that  will remain the solution of system (1.3) at
will remain the solution of system (1.3) at 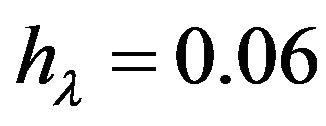 for any
for any 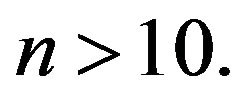
From the solution of the system (1.6) we obtain the coefficients of the function . This function achieves the maximum
. This function achieves the maximum 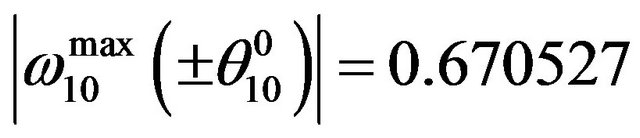 at the points
at the points
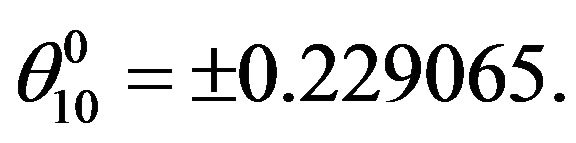 The maximum slope of the free surface in degrees
The maximum slope of the free surface in degrees 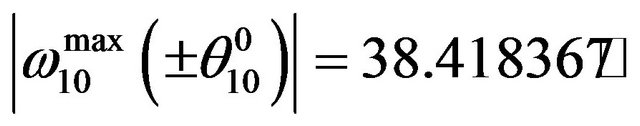 exceeds the value
exceeds the value  received in [6]. We suppose that
received in [6]. We suppose that 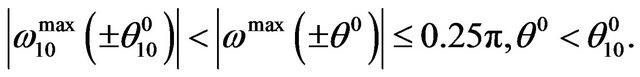
3. The Conservation Law of Full Mechanical Energy and Stability of a Wave
For evaluation of a wave square and full mechanical energy we need the parametric equations of wave’s surface coordinates
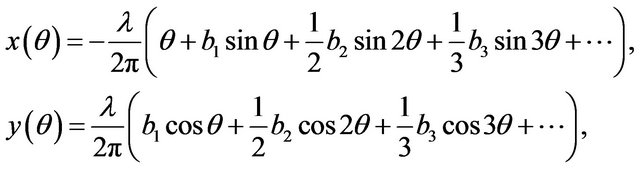
(2.1)
and the function
 (2.2)
(2.2)
Coefficients 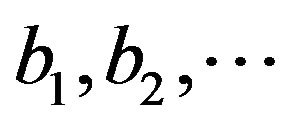 are determined as algebraic expressions of
are determined as algebraic expressions of 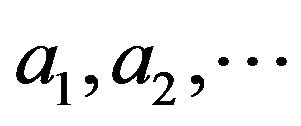 [2].
[2].
The coordinate origin O is on vertical line through the wave crest at distance h from the bottom,the 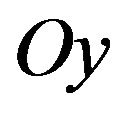 axis is directed upward, and the
axis is directed upward, and the 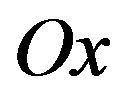 axis to the right. In a coordinate system attached to the wave the bottom moves from right to left at velocity [4]
axis to the right. In a coordinate system attached to the wave the bottom moves from right to left at velocity [4]
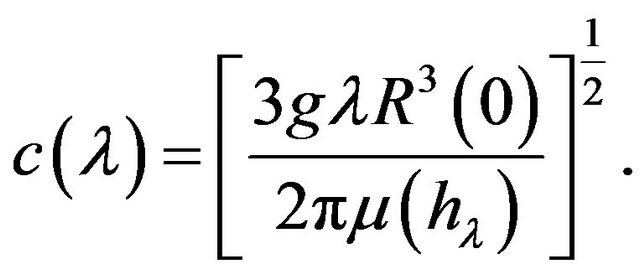 (2.3)
(2.3)
Using (2.1)-(2.3) at fixed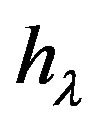 , we obtain the expressions for the properties of the wave: the surface area
, we obtain the expressions for the properties of the wave: the surface area
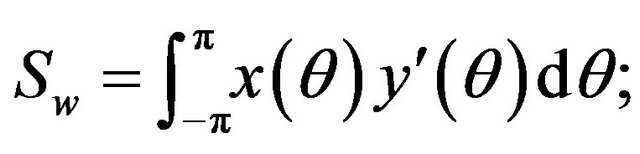 (2.4)
(2.4)
the coordinates of the center of mass
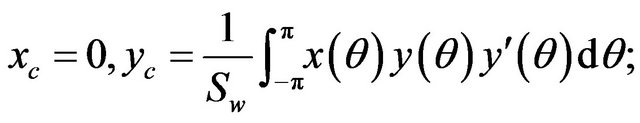 (2.5)
(2.5)
the kinetic energy
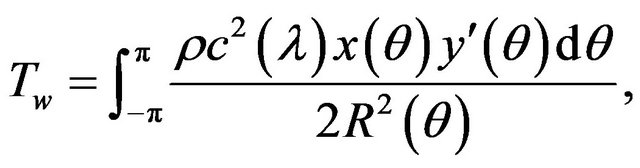 (2.6)
(2.6)
where constant  denotes the fluid density; the potential energy
denotes the fluid density; the potential energy
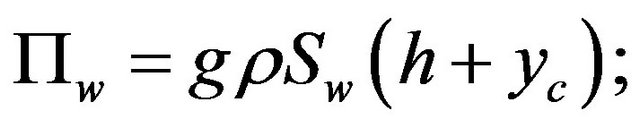 (2.7)
(2.7)
the full mechanical energy
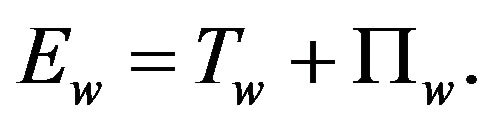 (2.8)
(2.8)
The full mechanical energy of an unperturbed fluid layer with depth h and wavelengths  is considered as
is considered as
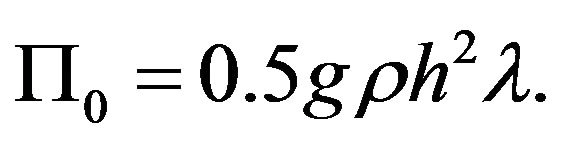 (2.9)
(2.9)
Substituting  for
for  in (2.4)-(2.9), we get
in (2.4)-(2.9), we get
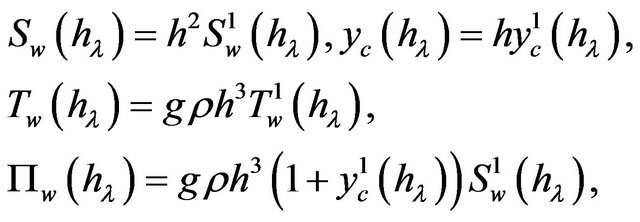
and 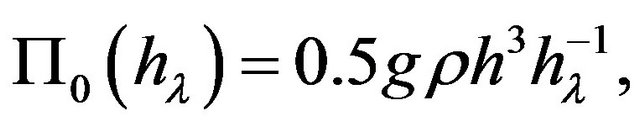 where
where
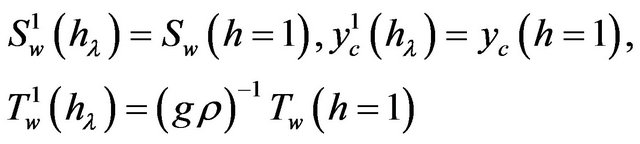
are integrals depending on parameter  Using these relationships we can write the Equations (2.6)-(2.8) in the form
Using these relationships we can write the Equations (2.6)-(2.8) in the form
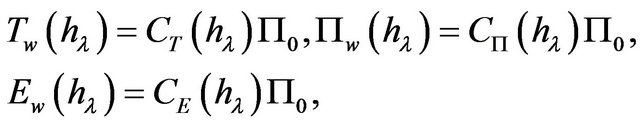 (2.10)
(2.10)
where
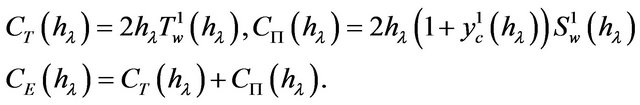
The solution of system (1.3) obtained in [2] allow us to calculate the function 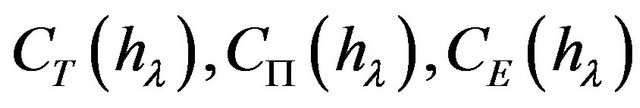 on the segment
on the segment 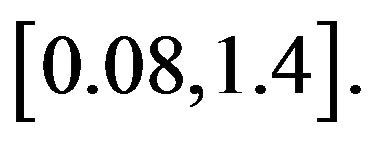 On the boundaries of this segment we have
On the boundaries of this segment we have
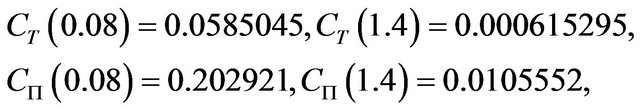
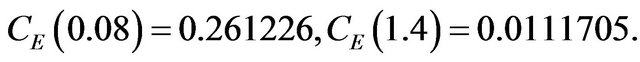
The functions 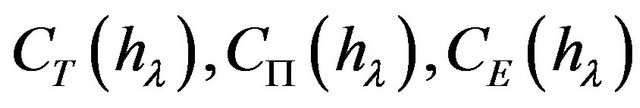 calculated on the segment
calculated on the segment 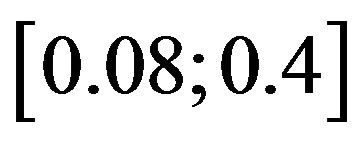 are shown on Figure 1. The outcomes of these calculations allow to assert that the wave potential energy
are shown on Figure 1. The outcomes of these calculations allow to assert that the wave potential energy 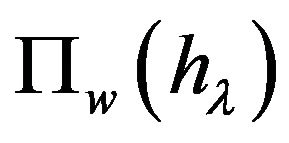 as function of parameter
as function of parameter
 monotonically diminishes on the segment
monotonically diminishes on the segment 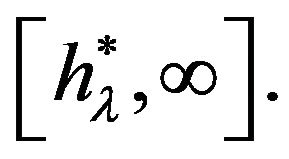
In order to calculate the greatest value of potential energy of a wave we need the 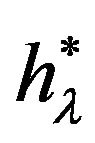 solution of system (1.6) instead of
solution of system (1.6) instead of 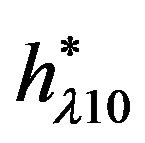 (see above).
(see above).
The law of the wave kinetic energy change is formulated in the form of a mathematical theorem in [3]. This theorem asserts that: 1) for any constant depth of fluid 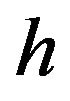 the wavelength
the wavelength 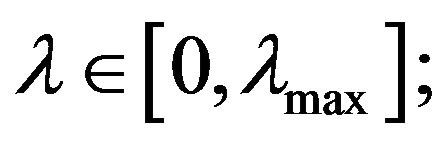 2) at the boundaries of the segment
2) at the boundaries of the segment 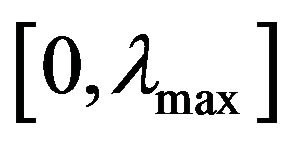 the wave velocity
the wave velocity
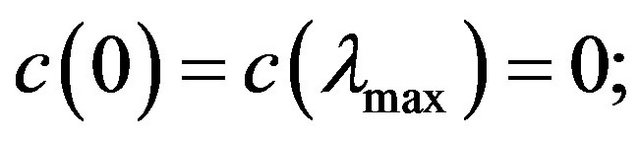 3) there is a value
3) there is a value 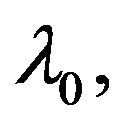 at which
at which
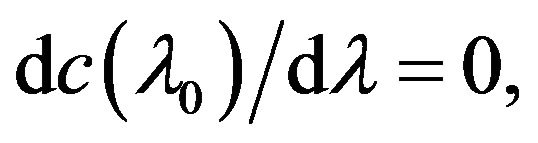 and the wave velocity is maximum, i.e.
and the wave velocity is maximum, i.e. 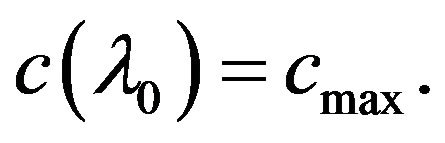
Let’s prove the points 1) - 3). 1) From (1.7) follows that 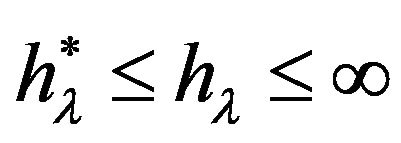 and therefore
and therefore 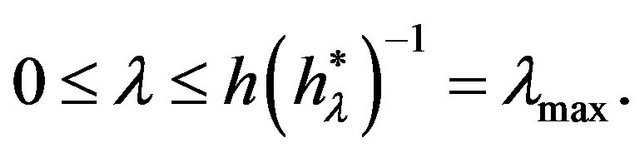
2) Suppose the solution 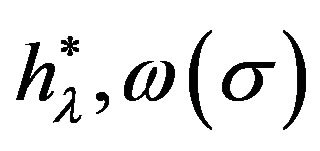 of (1.6) is known and satisfy (1.4). Using this solution we write the integrals
of (1.6) is known and satisfy (1.4). Using this solution we write the integrals
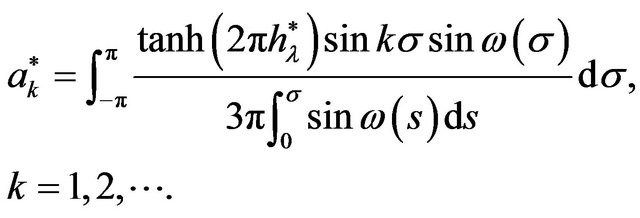 (2.11)
(2.11)
The first integral is known 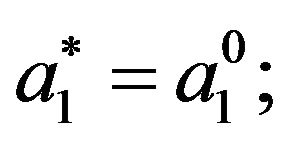 the integral
the integral  tends to zero as
tends to zero as  (see [7]). Now it is possible to express the coefficients
(see [7]). Now it is possible to express the coefficients 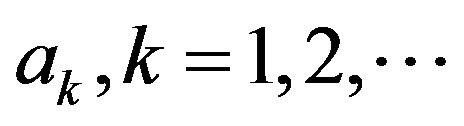 from the system (1.6) in terms of the integrals
from the system (1.6) in terms of the integrals 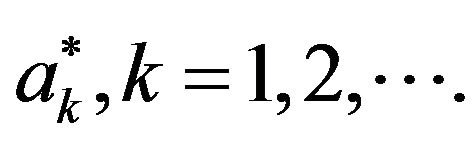 We have
We have
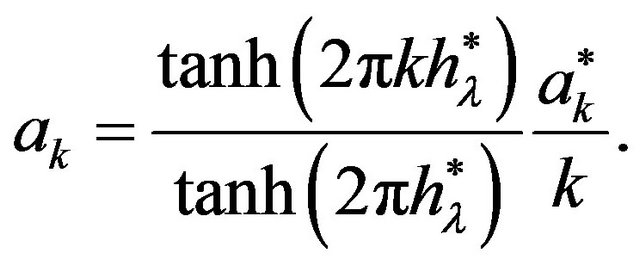 (2.12)
(2.12)
Taking into account 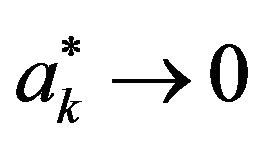 as
as 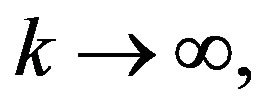 from (2.12) follows that series
from (2.12) follows that series 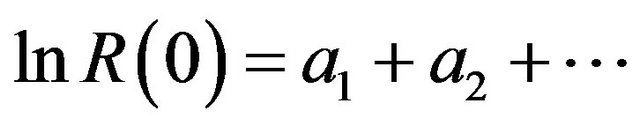 is converging independently of convergence or divergence of the series
is converging independently of convergence or divergence of the series 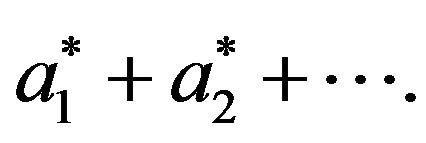 Now if we recall (1.7), (2.3), and (2.12), we get
Now if we recall (1.7), (2.3), and (2.12), we get 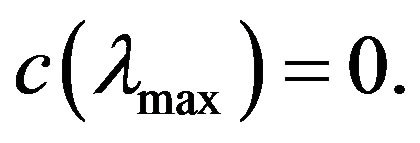
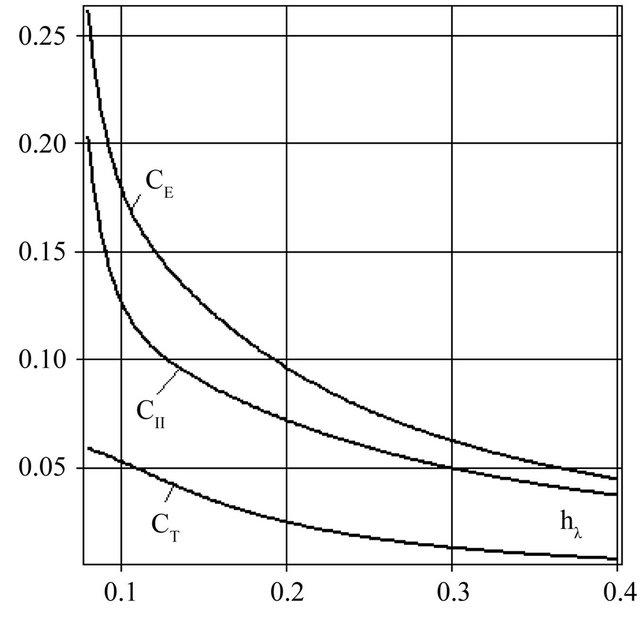
Figure 1. The functions CT(hλ), CII(hλ), CE(hλ) for 0.08 ≤ hλ ≤ 0.4.
Let as remark that the convergence of series 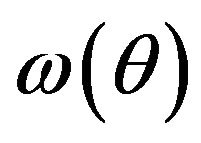 has been proved at a limited
has been proved at a limited  in [4] and for a
in [4] and for a 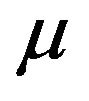 presented as a converging series in [8].
presented as a converging series in [8].
3) Since the function 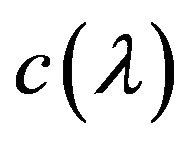 vanishes at the boundaries of the interval
vanishes at the boundaries of the interval  it follows from the Rolles theorem [7] that exists at least a point
it follows from the Rolles theorem [7] that exists at least a point
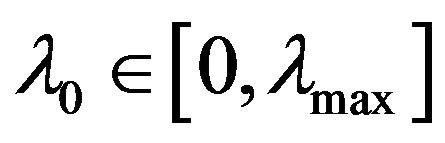 such that
such that  As the numerator and denominator of right side of Equation (2.3) are strictly increasing functions of
As the numerator and denominator of right side of Equation (2.3) are strictly increasing functions of  the point
the point 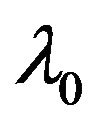 is single and
is single and 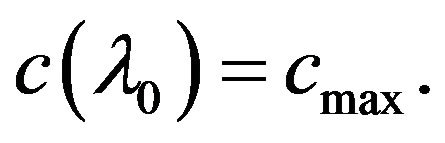 The values
The values
 have been calculated in [2].
have been calculated in [2].
The kinetic energy of the wave vanishes at boundaries of the segment 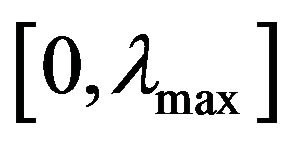 and reaches the maximum at a point
and reaches the maximum at a point 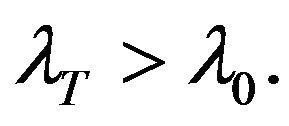 (This point is not presented on Figure 1). The full mechanical energy of a wave is a monotonically decreasing function on the segment
(This point is not presented on Figure 1). The full mechanical energy of a wave is a monotonically decreasing function on the segment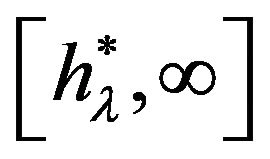 , but vanishes only at the point
, but vanishes only at the point  We note also that the profiles of the waves calculated on the segment
We note also that the profiles of the waves calculated on the segment 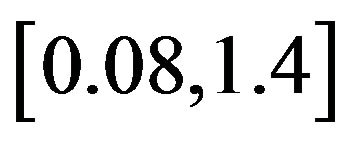 obey the law of mass conservation
obey the law of mass conservation 
The stability of Nekrasov’s waves has been considered in [9]. We proceed from the fact that a liquid maintaining the invariable shape without a vessel, does not suspect that is a fluid.We suppose that a steady state wave can be presented as a compound pendulum with a suspension center in the origin of coordinates. This wave is stable if
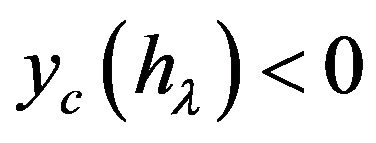 and unstable if
and unstable if  The function
The function
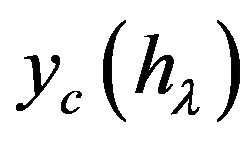 is presented on Figure 2. This function has the greatest value
is presented on Figure 2. This function has the greatest value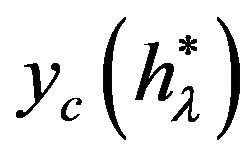 , is equal to zero
, is equal to zero
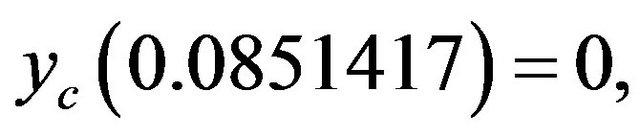 has the minimum
has the minimum
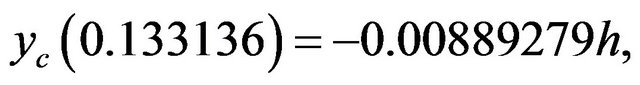 and monotonically increases on the interval
and monotonically increases on the interval  reaching the value
reaching the value 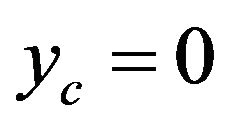 only at the point
only at the point 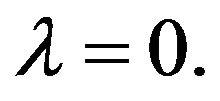 If we continue to con-
If we continue to con-
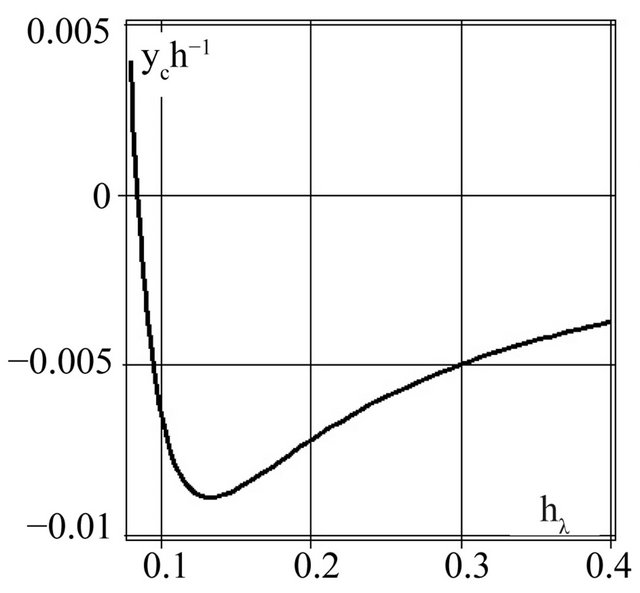
Figure 2. The dependence ych−1(hλ) for 0.08 ≤ hλ ≤ 0.4.
sider the wave behaving as a compound pendulum then it is stable at 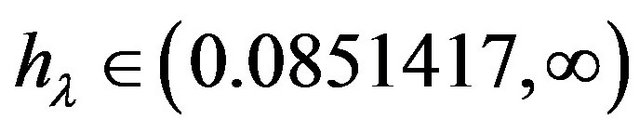 and unstable when
and unstable when
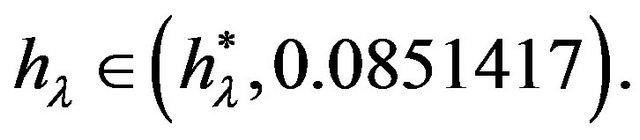
In particular let’s consider a wave of length λ = 1000 m on the surface of a fluid with depth 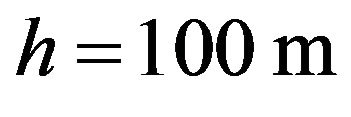 and density
and density 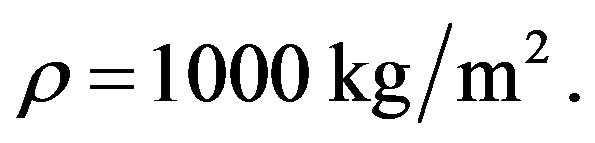 The solution of system (1.3) at the point
The solution of system (1.3) at the point  (see [2]) and the coefficients b1, b2, …, b7 are given below: μ7(0.1) = 7.449619; a1 = 0.047452; a2 = 0.0195554; a3 = 0.00606262; a4 = 0.00183319; a5 = 0.000565461; a6 = 0.000178475; a7 = 0.0000568810; b1 = 0.047452; b2 = 0.0206812; b3 = 0.00700837; b4 = 0.00233431; b5 = 0.000787255; b6 = 0.000268797; b7 = 0.0000921207. Using these outcomes in Formulas (2.1)-(2.10) we get the parameters of the wave: the crest coordinate
(see [2]) and the coefficients b1, b2, …, b7 are given below: μ7(0.1) = 7.449619; a1 = 0.047452; a2 = 0.0195554; a3 = 0.00606262; a4 = 0.00183319; a5 = 0.000565461; a6 = 0.000178475; a7 = 0.0000568810; b1 = 0.047452; b2 = 0.0206812; b3 = 0.00700837; b4 = 0.00233431; b5 = 0.000787255; b6 = 0.000268797; b7 = 0.0000921207. Using these outcomes in Formulas (2.1)-(2.10) we get the parameters of the wave: the crest coordinate 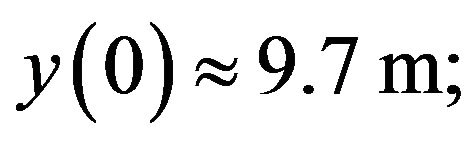 the trough coordinate
the trough coordinate 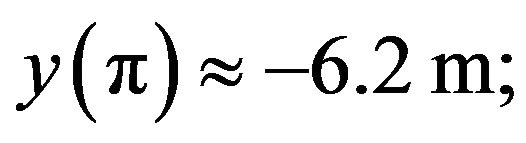 the amplitude
the amplitude 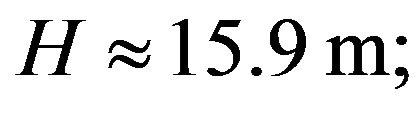 the coordinates of mass center
the coordinates of mass center 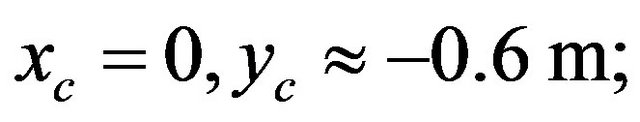 the kinetic energy
the kinetic energy 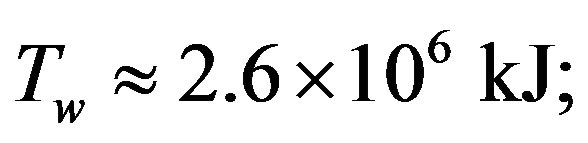 the potential energy
the potential energy  the velocity
the velocity  This wave similar to a tsunami is stable as
This wave similar to a tsunami is stable as 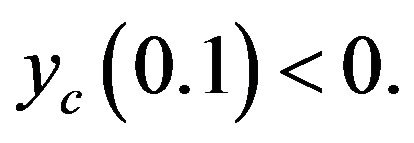
For 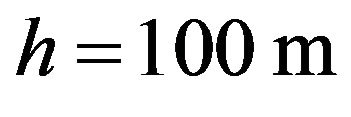 and
and 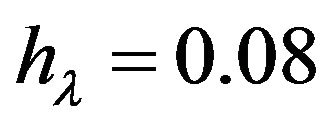 (The solution of the system (1.3) and coefficients
(The solution of the system (1.3) and coefficients 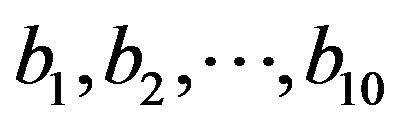 for
for 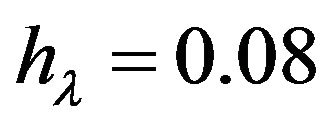 are given in [2]) the wave parameters will be:
are given in [2]) the wave parameters will be: 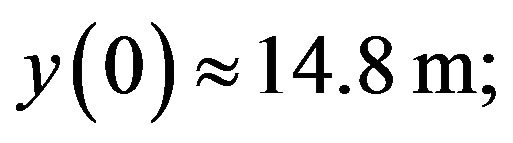
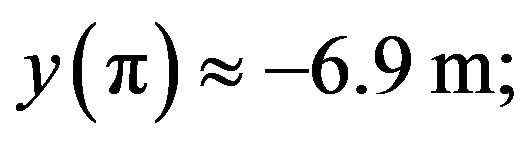
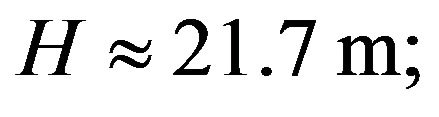
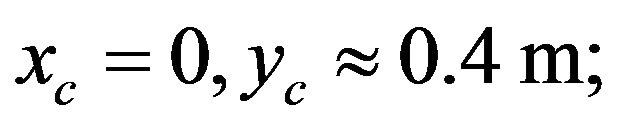
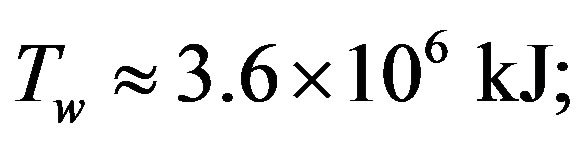
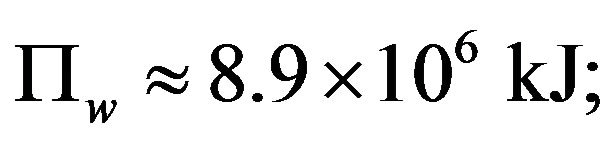
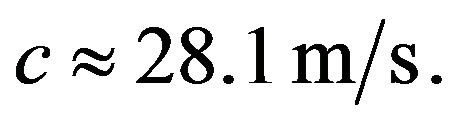 This wave is unstable as
This wave is unstable as 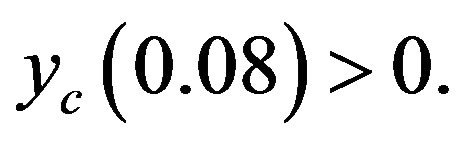
In summary it is necessary to note that in the accessible publications we have not discovered materials for comparison, except [6].
4. Conclusion
Solving the Nekrasov’s integral equation we avoided the “Liapunov-Schmidt” method and other methods of searching the solution in the neighbourhood of eigenvalues of Nekrasov’s linearized equation. We sought the solution of this equation in the neighbourhood of the motionless point of the nonlinear integral Equation (1.5). This is the point 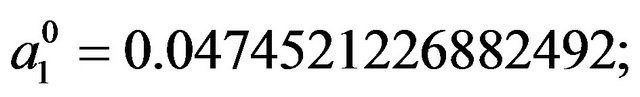
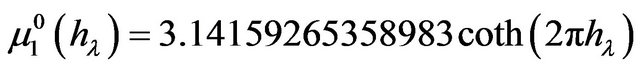 .
.
REFERENCES
- T. A. Bodnar’, “One Approximate Solution of the Nekrasov Problem,” Journal of Applied Mechanics and Technical Physics, Vol. 48, No. 6, 2007, pp. 818-823. doi:10.1007/s10808-007-0105-9
- T. A. Bodnar’, “On Steady Periodic Waves on the Surface of a Fluid of Finite Depth,” Journal of Applied Mechanics and Technical Physics, Vol. 52, No. 3, 2011, pp. 378-384. doi:10.1134/S0021894411030072
- T. A. Bodnar’, “On Steady Waves on the Surface of a Finite-Depth Fluid,” Free Boundary Problems: Theory, Experiment, and Applications, 3rd All-Russian Conference with International Participation, Biisk, 28 June-3 July 2008, pp. 25-26.
- A. I. Nekrasov, “Exact Theory of Steady Waves on the Surface of a Heavy Fluid,” Izd.Akad.Nauk SSSR, Moskow, 1951. (In Russian)
- R. Courant and D. Hilbert, “Methods of Mathematical Physics,” Interscience, New York, 1953.
- G. A. Chandler and I. G. Graham, “The Computation of Water Waves Modelled by Nekrasov’s Equation,” SIAM Journal on Numerical Analysis, Vol. 30, 1993, pp. 1041- 1065. doi:10.1137/0730054
- R. Courant, “Differential and Integral Calculus,” Interscience, New York, 1936.
- L. N. Sretenskii, “Theory of Fluid Wave Motion,” Nauka, Moscow, 1977. (In Russian)
- T. A. Bodnar’, “Conservation Law of the Full Mechanical Energy and Stability of the Steady-State Waves on the Surface of a Fluid of Finite Depth,” In: IV All-Russian Conference with foreign participation on Free Boundary Problems: Theory, Experiment, and Applications, Biisk, 5-10 July 2011, pp. 18-19.

