Received 30 October 2015; accepted 30 November 2015; published 3 December 2015

ABSTRACT

In previous modeling works, the waveguide radiation in the free space is modeled using an infinite flange in aperture plan. In this paper, we propose a new formulation to analyze the radiation of twin rectangular waveguides in free space. Our formulation consists firstly in simulating the free space as rectangular waveguide and seeking the appropriate dimensions that do not affect scattering parameters. In the second step, we use the symmetry principle to reduce the coupling problem to single guide radiating in the free space. Moreover, for more simplification, we consider a concentric discontinuity to solve this latter. This approach is based on moments method combined to the generalized equivalent circuit method (MoM-GEC) in order to reduce the number of unknown problems and alleviate their computation. Obtained numerical results are presented and discussed. A good agreement with literature is shown and the boundary conditions are verified.

Keywords:

Waveguide, Coupling, MoM-GEC, Propagation, Scattering, Electric Field

1. Introduction

Open-ended rectangular waveguide is the elementary component for the millimeter wave’s propagation. Several kinds of design have been proposed (single, coupled, networked...) to be used to achieve several applications such as satellite communication, radar, medical systems, and geophysical applications [1]-[3]. The variational method is the first approach used to solve the electromagnetic problem concerning these areas [4] [5]. The boundary conditions require a relationship between the tangential electromagnetic fields, which leads to an integral equation [6]. This integral equation is solved using the moment method to investigate the single or coupled waveguides behaviors.

In [7], the aperture field is modeled using the LSE modes and is based on point-matching approach. This formulation is extended in [8] by including the cross-polarized component and a dominant mode approximation is employed. Another approach consists in formulating the waveguide coupling with an iris [9] by employing orthogonal functions associated to the LSE modes. In fact, a general asymptotic formula to study the coupling between modes in separate waveguides has been applied to investigate the response of a uniform [10], and non- uniform rectangular waveguide array [11].

Some other authors have used the generalized network formulation, which is based essentially on the equivalence principle and MoM method. This formulation was applied to finite phased arrays [12] and reactively loaded waveguide arrays [13].

There are many other methods which have been applied to solve the flanged waveguide problem. The correlation matrix method which is based on the energy conservation low has been applied in [14], while Teodoridis et al. [15] have used the characteristic modes and the problem is solved by investigating the eigenvalues. In [16], Boudrant et al. have obtained an integral equation by using the operator transverse method. This integral equation was solved using the Galerkin’s method.

In [17], a rigorous formulation based on integral equation is proposed and solved by MoM method in order to analyse the radiation of rectangular waveguides array. Another approach consists to consider this last as a flanged parallel-plate waveguides array [18]. Serizawa et al. [19] used an analytical method based on Kobayashi potential method (KP) and derived the exact expressions of the fields to obtain reference data of physical quantities.

Mongiado et al. [20] proposed a singular integral equation approach including the edge condition on the apertures from the start, in order to analyze single and twin radiating waveguide

In this paper, a new rigorous approach is proposed to model two opened waveguides radiating in free space. The concept consists, firstly, in using the symmetry principle to reduce structure to half, and simulate properly the free space by another rectangular waveguide. Secondly, it consists in studying electromagnetic coupling between these waveguides. For validity purpose, obtained results are compared to those in previous works, and good agreement is shown.

2. MoM-GEC Formalism

Basically, in electromagnetic study, problems are well described by Maxwell’s equations. But in order to alleviate their complexity, we always need to choose the appropriate method that can approximate environment and boundary conditions to compute it within a reasonable time [11] [21] [22] . In our case, we combine two methods. The first method is the generalized equivalent circuits (GEC) [22] . It can present integral equations by equivalent circuit that describes faithfully all the problem. Electromagnetic phenomenon in discontinuity, which is the unknown problem, is presented by a virtual source. The environments are brought back to discontinuity plan as admittance or impedance operator. Excitation is illustrated as a localized or a modal source, in discontinuity too [23] . The second is the method of moment (MoM) [21] . It associates the known test functions to a virtual source, injects a modal basis in environment operator, and projects test function on a modal basis to resolve the problem. The good choices of test function lead to accelerate the convergence, and reduce greatly the computation time [24] .

3. Formulation

In this paper, we propose a modular formulation, in other terms, we simplify the problem step by step.

As shown in Figure 1, the study structure is composed by two opened rectangular waveguides, called network guides (NG), radiate in free space. This latter is considered as a third waveguide, called space guide (SG); with large dimensions.

In the second time, we use the symmetry principle to reduce the number of NGs to one. Then, odd and even input impedance are determined respectively when we apply structures presented in Figure 2(a) and Figure 2(b). Thus, the input impedance of initial structure is easily deduced as it will be shown later.

Finally, we propose to study the SG influence, and we will make sure that considered dimensions do not affect the results.

So, now the problem is reduced to study NG1 radiates in SG. For this, we present the new considered structure in Figure 3(a); and its relative equivalent circuit model in Figure 3(b).

The excitation is brought back to discontinuity plan as a modal source of current, its value is the current density of the fundamental mode, and its internal admittance is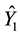. This latter represents the evanescent modes contribution of NG1; and its formal writing is:

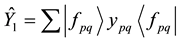(1)

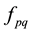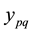where is the NG1 modal basis without the fundamental, and is the mode admittance of each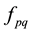. The voltage at terminals of this source is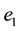, who is its dual greatness.

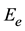is the virtual voltage source defined in discontinuity plan, and is the current flowing it. 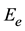is the problem unknown, and 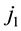is expressed as a serial of test functions 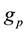weighted by unknown modal amplitudes:

(2)

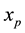The modes contribution of SG is expressed in the discontinuity plane by admittance operator .

(3)

where is the SG modal basis and is the relative mode admittance.

Based on the equivalent circuit shown in Figure 3(b), the integral equations associated with the problem can be easily derived by applying Kirchhoff laws generalized:

(4)

Using the equations system (4), we can write:

(5)

Then, we can deduce the current:

(6)

Taking into account the expression, we can find the relation between source variables and their duals:

(7)

Applying the Galerkin’s method:

(8)

This equations system can be rewritten as:

(9)

where A is the excitation vector, and [y] is the admittance matrix:

(10)

(11)

By separating the admittance operators, we can rewrite [y] matrix as:

(12)

where the first term presents the projection of test functions on the evanescent modes of the same guide, and the second term their projection on the load operator.

Finally, we deduce the unknown problem:

(13)

Taking into account relations (8) and (12), we deduce the input impedance z:

(14)

And scattering matrix is given by:

(15)

where is impedance of free space.

To pass to total structure, we must replace, in Equation (13), by or depending on boundary conditions described in Figure 2.

So, the total input impedance matrix is given by:

(16)

We note that z is the reduced matrix, and is the identity matrix.

(17)

The total scattering matrix is expressed as:

(18)

Here, the formulation gives us parameters between NG1 and NG2; and SG has no more influence.

4. Numerical Results

In this section, we propose first a quantitative discussion about the radiation of a real opened waveguide (WR90) in the free space, in order to determine the appropriate model that simulates the free space. Second, we study the electromagnetic coupling between NG1 and NG2.

To set certain parameters of the problem, a convergence study is strongly recurred. Figure 4 presents the S11 norm as a function of a mode number for different test function number.

As shown in Figure 4, the convergence is obtained for 15 test functions and 1600 mode functions. To ensure the convergence, in the following, we always use these last values.

First main target of this study is to determine the appropriate SG dimensions to simulate the free space. For this, we present in Figure 5 the S parameters as function of. Where D and d₁ are respectively dimensions of SG and NG1.

We can consider that from D = 7d₁ − 4.6λ there is no influence of SG on results. For a validation propose, we present in Table 1, the obtained reflection coefficient S11 and that obtained in previous works for operating frequency f = 9.33 GHz. It is found that a good agreement is shown.

The second main target of this study is to determine coupling effects between two NGs. For this we explore parameter, and validate it with previous work [7] (Figure 6). We notice that a good agreement is shown around the operating frequency f = 9.33 GHz.

We present in Figure 7, the parameter as function of the coupling distance d. And we can notice that for, the coupling coefficient reaches a value of. This coupling value corresponds to 2% of the total energy. So, from this distance, we can consider the two waveguides as isolated.

At, the scattering matrix worth:

(19)

We can notice that does not contain the entire energy.

(20)

If one considers the load operator which is in fact the free space, we can explain the difference by the radiating energy in the free space. This radiation is given by:

(21)

Moreover, we present the normalized electric field in the discontinuity plan, with, r = 10, and we can verify that it is maximum in the radiating apertures and vanishes on the metal surface. This shows (Figure 8) that the electric field verifies the boundary conditions.

5. Conclusion

In this study, we apply a rigorous formulation based on MoM-GEC method to explore a radiation of twin open ended waveguides in the free space. The main idea is composed by two steps. First, we consider two waveguides with a simple concentric discontinuity in the propagation axis. One represents the radiating waveguide, and the other is the load waveguide; and we seek how this latter can properly simulate the free space. So, we determine its appropriate dimensions so that it no longer affects the scattering parameters. Second, we studied the electro- magnetic coupling between these two open ended waveguides and we present the electric field in the discontinuity plan. For a validation purpose, obtained results are compared to previous published works and boundary conditions are verified and a good agreement is shown. From the numerical results discussion, we can deduce that the load waveguide emulates the free space and no mismatch problem is shown from r = 7 and that the electromagnetic coupling for twin waveguides can be considered neglected from a coupling distance.

Cite this paper

AbdessalemKaddouri,MouradAidi,TaoufikAguili, (2015) A New Approach Based on MoM-GEC Method to Mutual Coupling Analysis of Symmetric Twin Waveguides for Antenna Applications. Journal of Electromagnetic Analysis and Applications,07,283-290. doi: 10.4236/jemaa.2015.712030

References