Journal of Water Resource and Protection
Vol.4 No.9(2012), Article ID:22721,22 pages DOI:10.4236/jwarp.2012.49080
Hydrogeological Conceptual Model of Groundwater from Carbonate Aquifers Using Environmental Isotopes (18O, 2H) and Chemical Tracers: A Case Study in Southern Latium Region, Central Italy
1Dipartimento di Ingegneria Civile, Edile ed Ambientale, Sapienza Università di Roma, Rome, Italy
2Dipartimento Scienze della Terra, Sapienza Università di Roma, Roma, Italy
3Centro Reatino di Ricerche di Ingegneria per la Tutela e la Valorizzazione dell’Ambiente e del Territorio, Sapienza Università di Roma, Rieti, Italy
Email: *giuseppe.sappa@uniroma1.it
Received July 11, 2012; revised August 15, 2012; accepted August 27, 2012
Keywords: Carbonate Aquifers; Recharge Areas; Hydrogeochemical Modeling; Groundwater Flow; Stable Isotopes
ABSTRACT
The present work provides hydrochemical and stable isotope data and their interpretations for 54 springs and 20 wells, monitored from 2002 to 2006, in the Southern Latium region of Central Italy to identify flow paths, recharge areas and hydrochemical processes governing the evolution of groundwater in this region. The hydrogeological conceptual model of the carbonate aquifers of southern Latium was based on environmental isotopic and hydrochemical investigation techniques to characterize and model these aquifer systems with the aim of achieving proper management and protection of these important resources. Most of the spring samples, issuing from Lepini, Ausoni and Aurunci Mts., are characterized as Ca-Mg-HCO3 water type, however, some samples show a composition of Na-Cl and mixed Ca-Na-HCO3-Cl waters. Groundwater samples from Pontina Plain are mostly characterized by Na-Cl and Ca-Cl type waters. Geochemical modeling and saturation index computation of the Lepini, Ausoni Aurunci springs and Pontina Plain wells shows an interaction with carbonate rocks. Most of the spring and well water samples were saturated with respect to calcite and dolomite, however all sampled waters were undersaturated with respect to gypsum and halite. The relationship between δ18O and δ2H, for spring and well water samples, shows shifts of both the slope and the deuterium excess when compared to the world meteoric (WMWL) and central Italy meteoric (CIMWL) water lines. The deviation of data points from the meteoric lines can be attributed to evaporation both during the falling of the rain and by run-off on the ground surface before infiltration. Most springs and wells have a deuterium excess above 10‰ suggesting the precipitation in the groundwater comes from the Mediterranean sector. On the basis of local isotopic gradients, in combination with topographic and geologic criteria, four recharge areas were identified in the Aurunci Mountains. In Pontina Plain, the elevations of the recharging areas suggest that the Lepini carbonate aquifers are feeding them.
1. Introduction
Groundwater constitutes about two thirds of the freshwater resources of the world and plays an important role in human life and economic activity [1]. Current aquifer recharge rates are a fundamental consideration in the sustainability of groundwater resource development. Moreover, understanding aquifer recharge processes and their linkages with land-use is essential for integrated water resources management. The quantification of natural recharge, however, is affected by methodological difficulties, data deficiencies, and resultant uncertainties due to temporal variability of rainfall and variation in soil properties [2]. The traditional quantitative approaches in the characterization of carbonate aquifers provides important data. However, these data are not sufficient to construct a conceptual hydrogeological models. Recently, monitoring changes in stable isotopes provides a better understanding of aquifer recharge and spring discharge that are essential for defining groundwater hydrodynamics. Isotopic data characterization techniques have become well established in the hydrological society for groundwater resource assessment, development, and management, and are now an integral part of many water quality and environmental studies [3-5]. Isotope tracing methods usually rely on “tracing” either isotope species naturally occurring in water (environmental isotopes) or on isotope tracers intentionally introduced into water [6]. The isotopes commonly employed in groundwater investigations are the stable isotopes of the water molecule, 2H and 18O and the radioactive isotopes, tritium and carbon-14. The stable isotopes are excellent indicators of the circulation of water, while the radioactive isotopes are of special value in determining the residence time, assuming no contamination of the water has occurred [7]. If the stable isotope content does not change within the aquifer, it will reflect the origin of the water, particularly the location, season and processes of recharge. If the isotope content changes along groundwater flow paths, this reflects the history of the water, particularly the mixing and salinization. The 18O and 2H data from groundwater are widely used to obtain information the functioning of the karst systems [8], to determine important recharge areas of these aquifers, and to delineate protection areas for the main springs [9]. The isotopic compositions of O and H in water, modified by meteoric processes, are useful tracers for determining groundwater origin and movement because they generally do not change as a result of rockwater interactions at low temperatures [10]. When stable isotopes and geochemical data are examined for both surface water and groundwater systems, important information about processes related to water cycling and waterrock interaction that may affect water quality. Thus, environmental isotopic and hydrochemical investigation techniques provide much information for the identification of groundwater flow systems and the main hydrogeochemical processes affecting the composition of water within the karst aquifers.
Groundwater resources in the southern part of the Latium region play an important role in providing water for domestic, industrial, and agricultural uses (i.e. Mazzoccolo spring, in the Ausoni Mts., is an important source for drinking water supply). Until now quantitative approaches have been developed to study these carbonate aquifers. However, no studies have been undertaken to understand the detailed classifications of groundwater flow systems recharge areas and geochemical evolution of groundwater in this region. The distinct isotopic signature of precipitation has been used in hydrological studies in Italy, however, these studies do not address the spatial isotope pattern of spring and well water from the carbonate aquifers of Sothern Latium. In the present work, groundwater in the carbonate aquifers of the Southern Latium region was characterized employing isotopic and hydrochemical data to identify groundwater flow paths, recharge areas and the main processes control the evolution of water. This study was also designed to hydrochemically characterize and model these aquifer systems with the aim of achieving proper management and protection of these important resources.
2. Geological and Hydrogeological Setting
Lepini, Ausoni and Aurunci are three distinct groups of mountains belonging to the pre-Apennines of Latium and they occupy a well-defined geographic area, called “Volscian mountain range” (Figure 1). These mountains are separated from the Apennine ridge by the Latina valley. The topography of the Apennine mountain structures is broken by NE-SW-trending fluvial valleys formed by streams that were channeled along existing tectonic lineaments. The springs lie at the bottoms of these mountain structures, which form an important groundwater reservoir that must be protected.
2.1. Lepini Mts. and Pontina Plain
The Lepini Mts. are located in the northern part of Pontina Plain and they are oriented parallel to the Apennine range, adjacent to the Latium coastline. The Lepini calcareous massif, hosts an important karst aquifer and it may be regarded as an isolated hydrogeological unit, nevertheless there is still some uncertainty about its SE margins. The top of mountains are calcareous, the valley are igneous rocks, which are often covered with younger alluvial fan and colluvial deposits. The Pontina Plain, situated in the southwestern part of the Latium Region, is a coastal plain developed along an extensional marine boundary (Figure 1). This Plain is positioned between the Lepini-Ausoni mountains of the Central Appenines and the Tyrrhenian Sea. Although Pontina Plain is generally between 0 and 40 m asl, it has several areas where the land is below sea level [11]. Marine transgressions resulted in thick deposits of marine, fluvial and fluvial— coastal sediments. In the case of the Pontina Plain, much of the groundwater comes out in springs near the boundary between the Pontina Plain, and the carbonate massif, joining a series of streams and canals that drain to the Tyrrhenian Sea [12]. The aquifer in the Lepini massif may be classified as “unconfined with an undefined bottom surface”. The saturated zone of the aquifer lies more than 1000 m below the surface in the inner areas of the relief. However, near the margins of the ridge, it is shallow and may be easily investigated through boreholes [13]. To study groundwater circulation, the most important hydrogeological unit is the so-called “Carpineto line”. The recharge areas of the aquifer generally coincide with the outcropping of the limestones, where groundwater recharge takes place through karst fissures. Two aquifers are present in Pontina Plain: one is an unconfined aquifer lying under the Quaternary deposits covering the limestones at the south-western margin of the
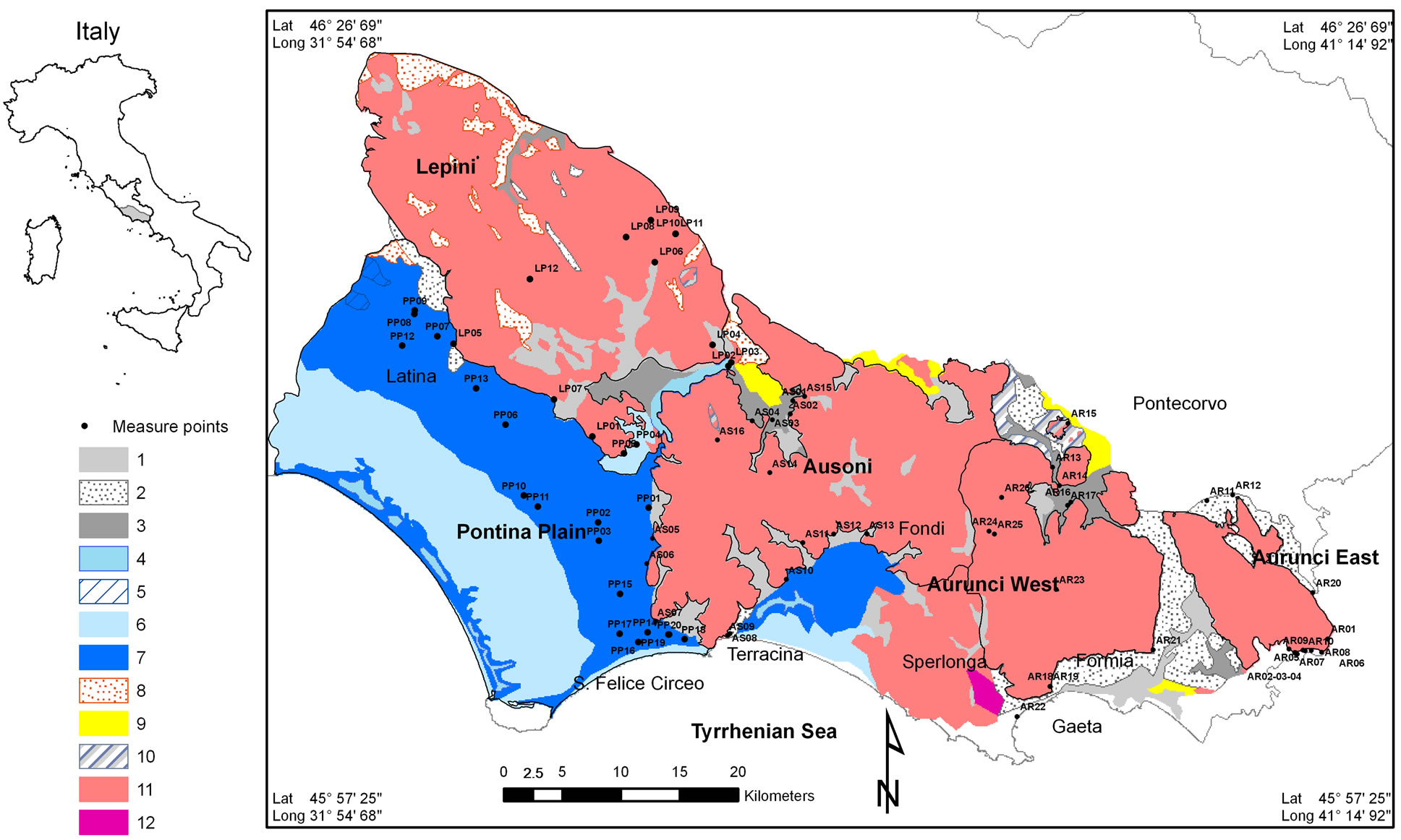
Figure 1. Simplified hydro-geological map of the study area: (1) Recent deposits (Holocene); (2) Detritic complex (PleistoceneHolocene); (3) Alluvial complex (Pleistocene-Holocene); (4) Alluvial deposits from perennial streams (Pleistocene-Holocene); (5) Travertine complex (Pleistocene-Holocene); (6) Sand dunes (Pleistocene-Holocene); (7) Fluvial lacustrine deposits (Holocene); (8) Pyroclastic complex (Pliocene-Pleistocene); (9) Heterogeneous clastic deposits (Pleistocene); (10) Clayey-marly Flysch complex with interbedded lithoids (Cretaceous-Miocene); (11) Carbonate platform complexes (Middle Lias-Upper Cretaceous); (12) Basal dolomite complex (Triassic-lower Lias). Spring and well sampling locations (LP: Lepini springs, AS: Ausoni springs, AR: Aurunci springs, PP: Pontina Plain groundwater).
Lepini complex, and the second one is a confined aquifer where the water is discharged from the calcareous aquifer of the Lepini massif and flows to the sea. The aquifer lies under the limestones and it is located at a depth of over 100 m, thus all of the wells sampled within the study area, penetrate the unconfined aquifer [14].
2.2. Ausoni Mts.
The Ausoni Mts. (Figure 1) rise in southern Latium and extend to close to the coastline, starting immediately after the middle Amaseno valley. The mountain structure consists mainly of Cretaceous limestones and includes both surficial and subsurface karst forms (Pastena caves). Groundwater flow takes place almost entirely in the subsurface, with the formation of high-discharge at the bottom of the slope. The Ausoni hydrogeological unit is mainly composed of limestones with interbedded dolomitic limestones. Depending on its fracturing and karst, the aquifer is very permeable; its hydrogeological properties permit flexible and sustainable exploitation of groundwater, where the circulation is very complex [15]. Major springs lie along all of its borders but with no sharp separations between their recharge areas. Groundwater flow paths and preferential discharge areas are controlled by important tectonic unconformities [16].
2.3. Aurunci Mts.
The Aurunci Mts. represent the southeastern part of the Volscian range and are oriented more or less parallel to the Apennine range (Figure 1). The Aurunci Mts. mostly consist of massive layers of dolomitic limestone and dolomites that were deposited on the carbonate platform that extended from Latium to Abruzzo from the Jurassic to the Palaeocene [17]. The Aurunci Mts. are composed of two distinct hydrogeological units: the western Aurunci, belonging to the Ausoni-Aurunci system, and the eastern Aurunci, which is separated from the western ones by a marly-arenaceous flysch complex [18]. The Western Aurunci hydrogeological unit consists of dolomitic limestones and dolomites of Jurassic and Cretaceous age. The springs are supplied by groundwater derived from these geological formations. The upper parts of the carbonate series contain relatively less-permeable Miocene calcareous formations. This carbonate unit is located on the east part of the Itri fault and within the Mt. Leucio and Mt. D’Oro blocks. The groundwater is directly discharged into the Liri river through the narrow alluvial belt separating the unit from the river. The carbonate unit holds multiple hydrogeological basins, whose boundaries match important tectonic lines that caused the outcropping of the calcareous-dolomitic Jura. The Eastern Aurunci hydrogeological carbonate structure is surrounded by relatively less-permeable sediments, including the Frosinone flysch, the Roccamonfina volcanites and the Garigliano plain alluvia [19,20]. Considering the structural setting of the massif, surrounded by the clay belt, groundwater runs from the carbonate structure towards the plain in the SE-NW direction. In the alluvial cover, groundwater flow is intercepted by the Liri paleo— river bed, and from west to east is not significantly mixed with the groundwater of the plain. The hydrogeological unit hosts two groundwater basins bounded by the Maio valley. The basin consists of the Mt. Maio monocline and the remaining part of the massif, which distributes main groundwater along the aquifer front, starting from the Mola Salomone spring and reaching Suio. Substantial water leakage occurs in the river bed and in the Garigliano alluvia. Hydrological data reported in the literature [19], suggest that this unit is well-demarcated structurally, morphologically and hydrogeologically. Therefore, no water inflow from other aquifers occur. Previous studies suggest that the potential groundwater discharge can be conservatively estimated at roughly 48 million m3/yr [20].
3. Methodology, Groundwater Sampling and Analytical Procedures
The main spring and well water sampling survey (Table 1) were carried out in Lepini, Ausoni, Aurunci Mts. and Pontina Plain, from 2002 to 2006. On the basis of the hydrogeological setting of study area, 54 spring and 20 well samples were characterized. Chemical and isotope analyses were performed on the collected water samples,
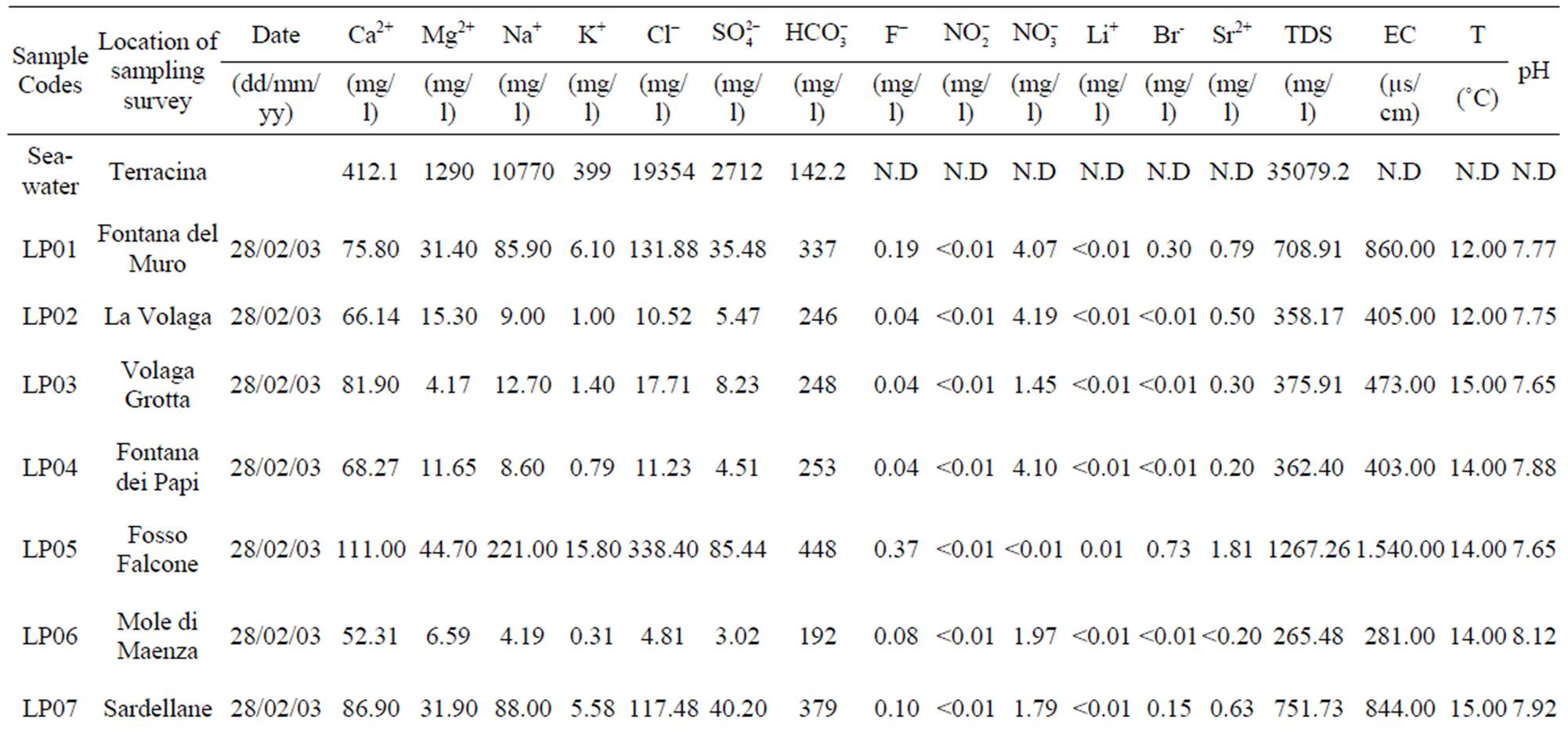
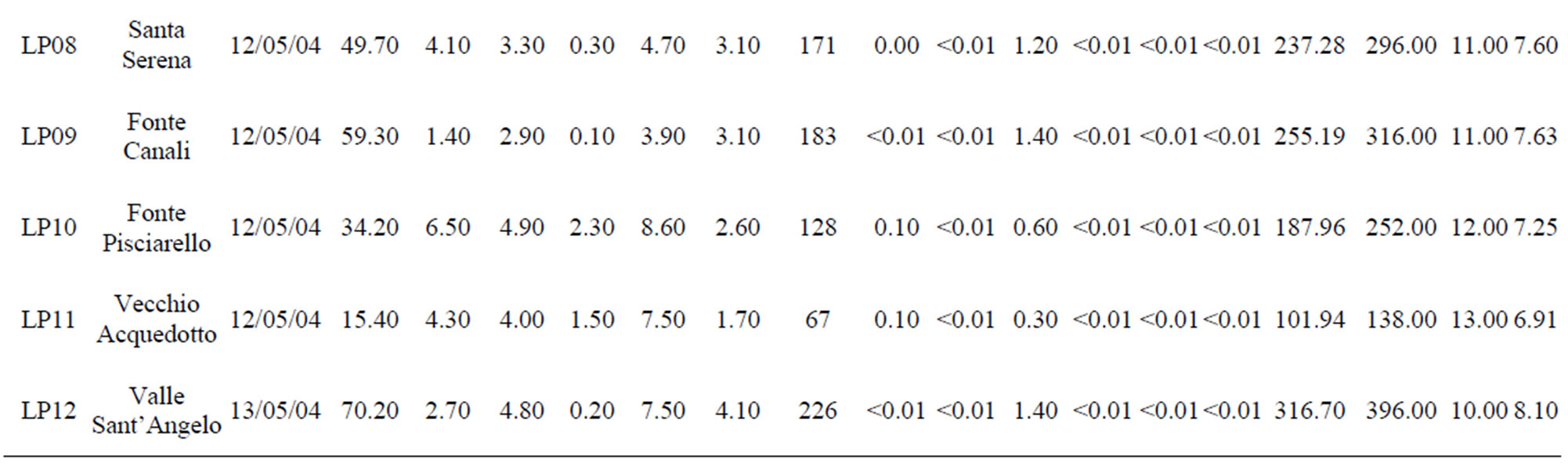
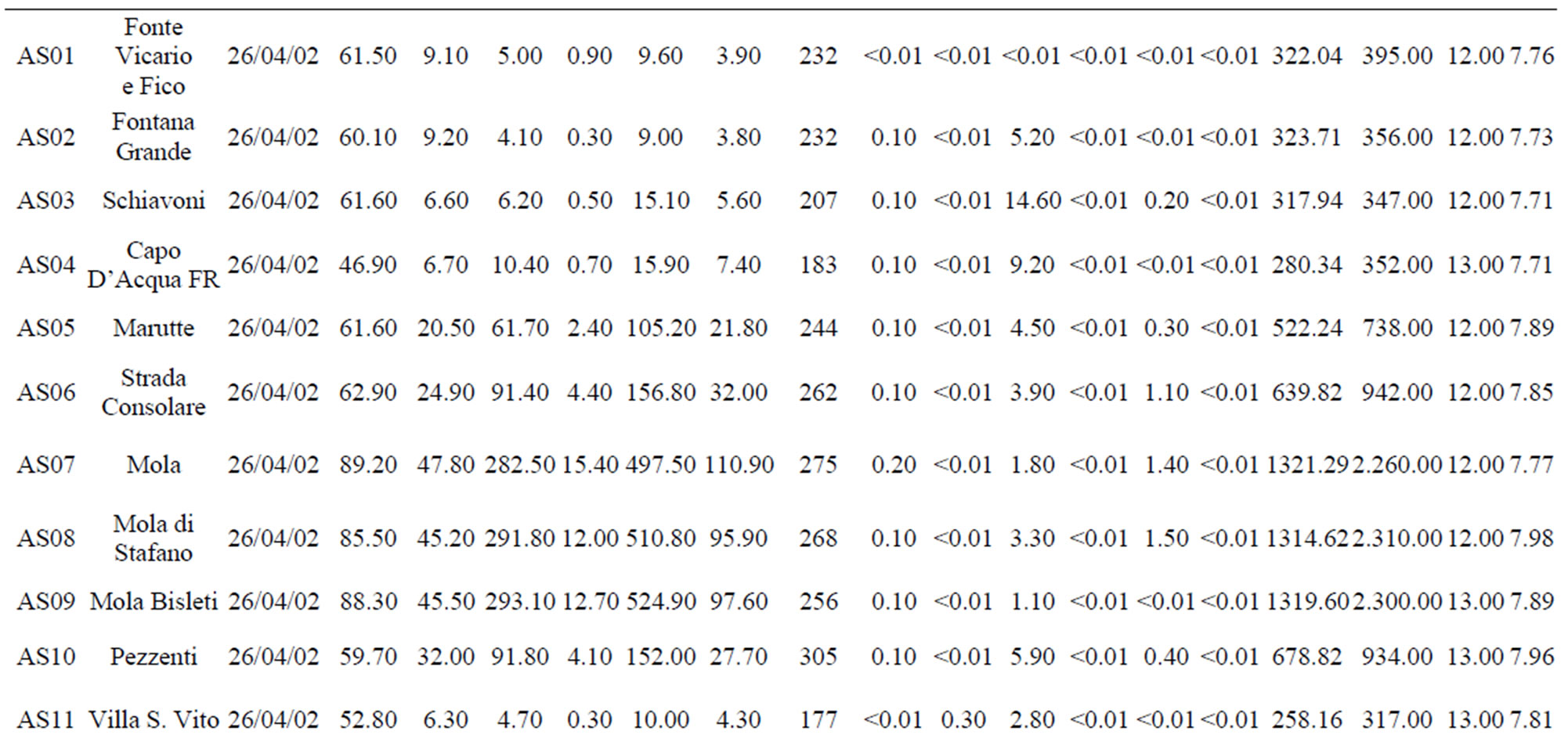
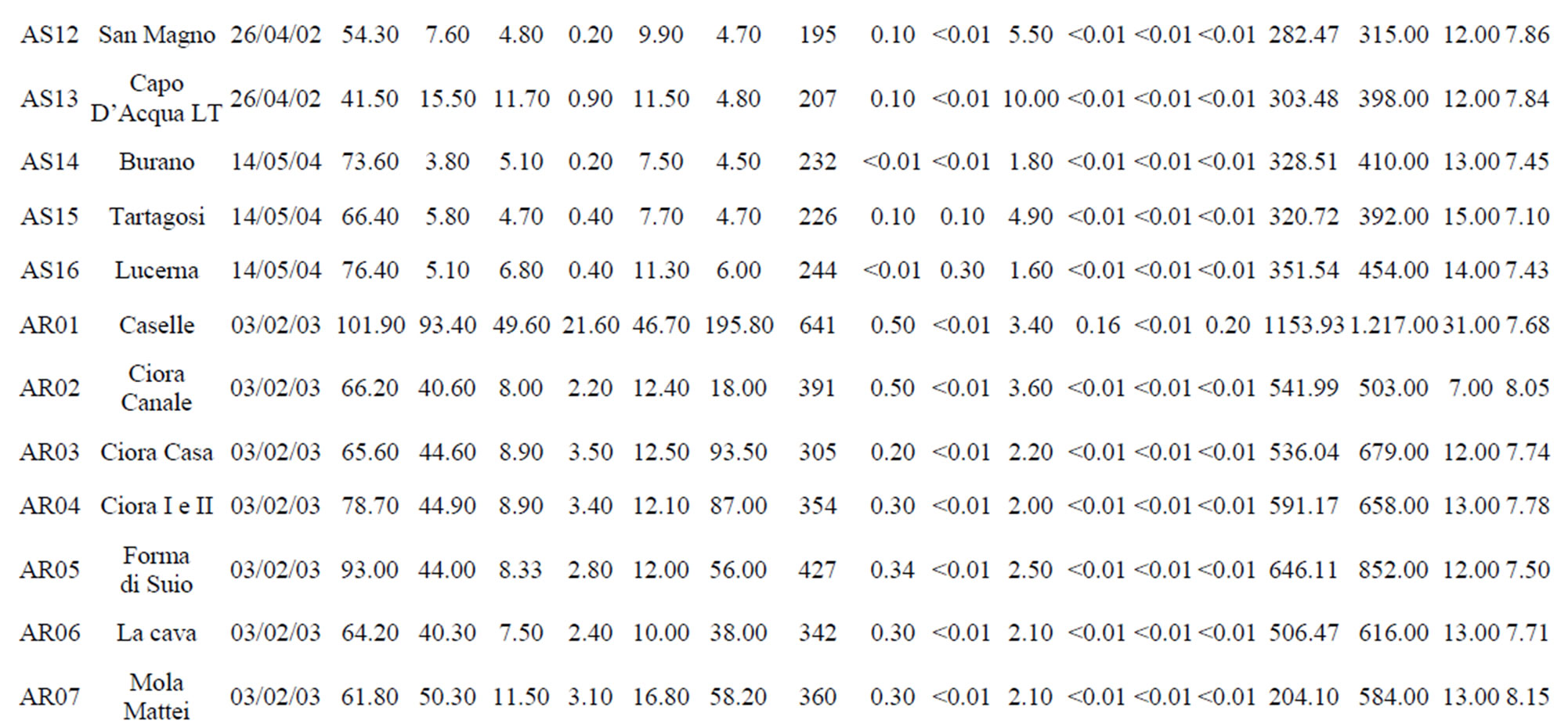
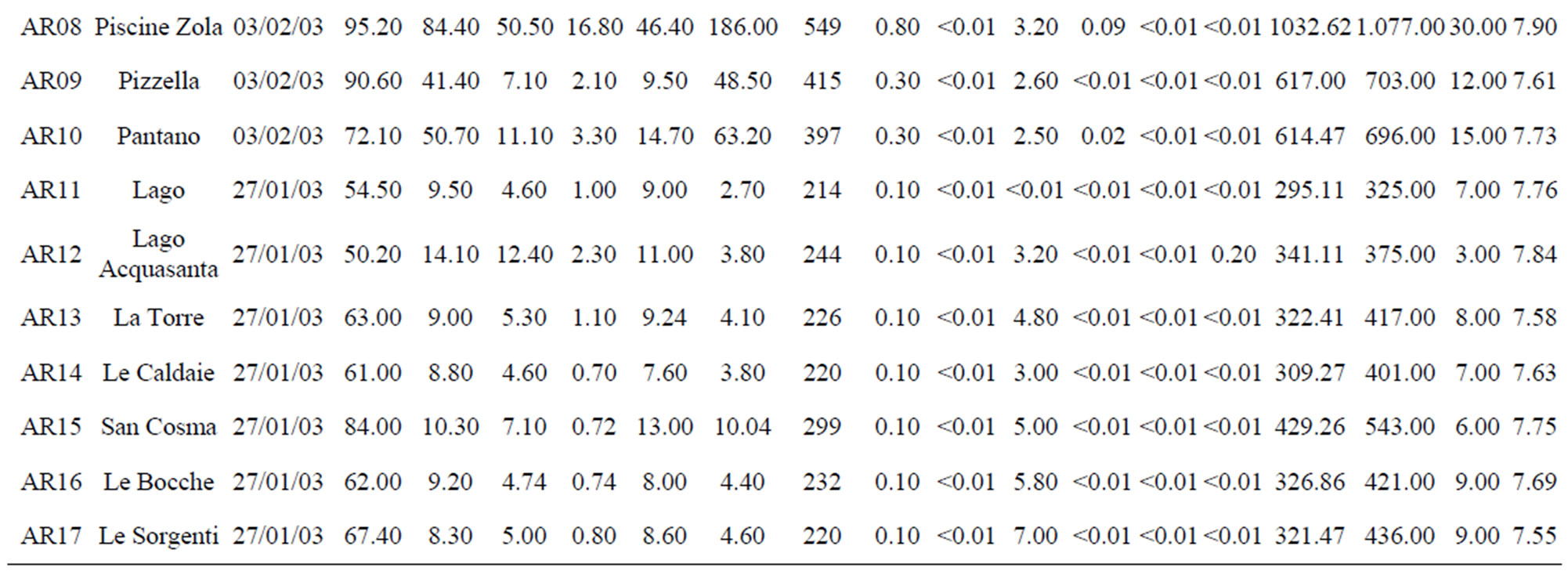
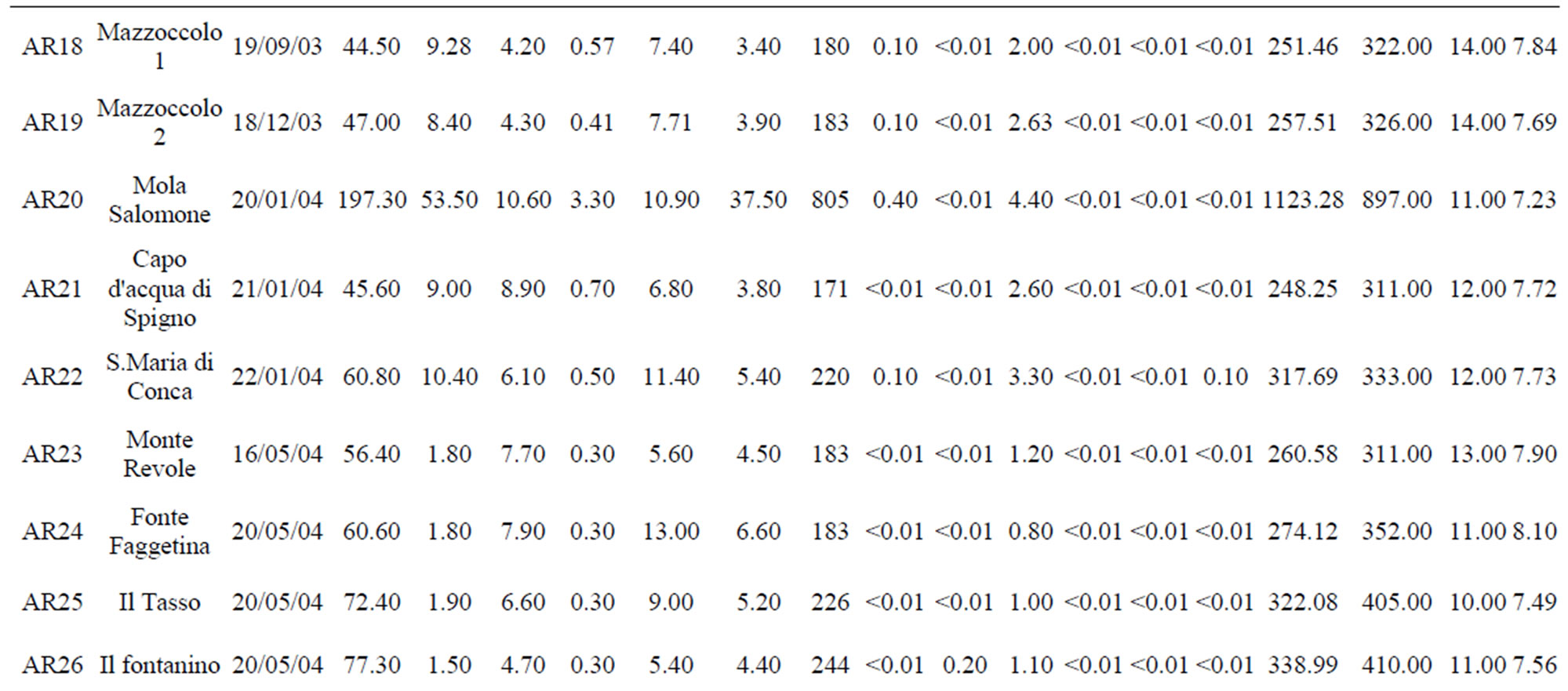
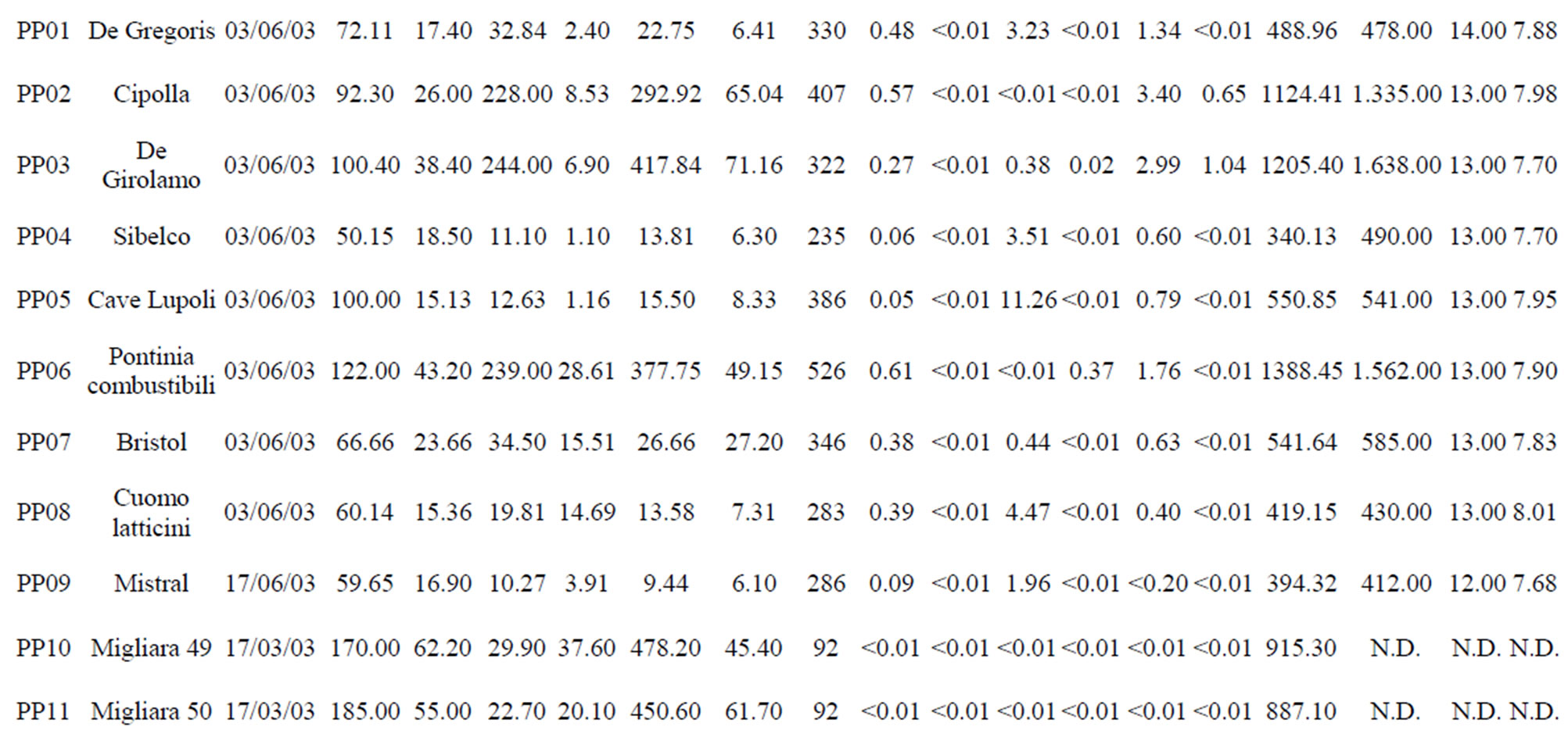
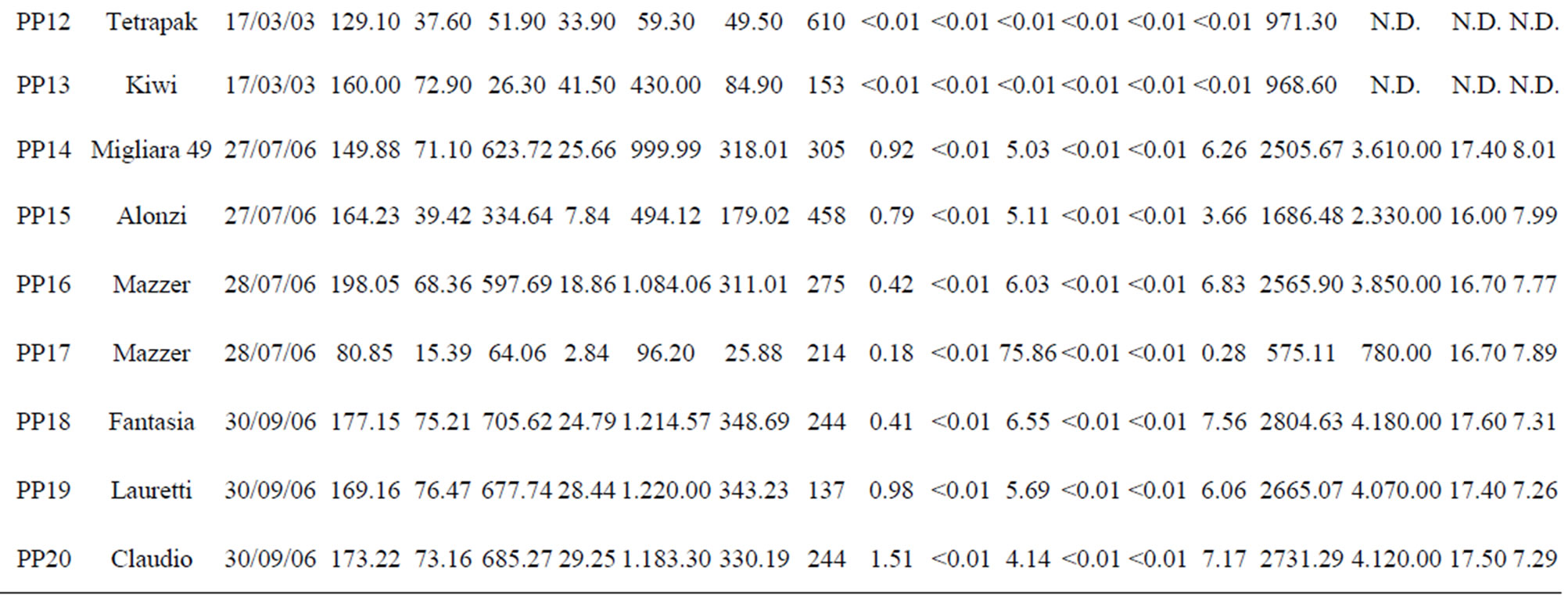
Table 1. The location of spring and well water sampling survey. Main physico-chemical characteristics and chemical composition of Lepini (LP), Ausoni (AS), Aurunci (AR) springs and Pontina Plain (PP) groundwater samples. Major ion composition of seawater near the study area (N.D. = not determined).
except for seven samples in Pontina Plain, collected in 2003-2006. Water temperature, electrical conductivity and pH values were determined in the field using PC 300 Waterproof Hand-held meter. Bicarbonate content was measured by titration with 0.1 N HCl. Water samples were filtered through cellulose filters (0.45 µm), and their major and minor constituents were determined by a Metrohm 761 Compact IC ion chromatograph (replicability ±2%). A Metropes C2-100 column was used to determine cations (Na+, K+, Mg2+, Ca2+, Li+, Sr2+), while a Metropes A Supp 4 - 250 column was used for anions (F–, Cl–,  ,
, 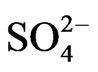 , Br–,
, Br–, 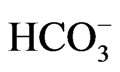 ,
,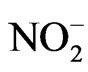 ). Chemical analyses were carried out at the Geochemical Laboratory of Sapienza, “University of Rome”. The analytical accuracy of these methods ranged from 2% to 5%. Isotope analyses were conducted at the isotope geochemistry laboratory of the University of Parma. 2H/H isotopes analyses were carried out the method of Kendall and [21] (reaction with Zn at 450˚C), while 18O/16O analyses based on the CO2-water equilibration technique [22]. Isotopic analysis was performed with a Finnigan Delta Plus mass spectrometer and the results are reported in ‰ units (permil deviation of the isotope ratio from the V-SMOW isotopic standard). δ is defined by the relationship:
). Chemical analyses were carried out at the Geochemical Laboratory of Sapienza, “University of Rome”. The analytical accuracy of these methods ranged from 2% to 5%. Isotope analyses were conducted at the isotope geochemistry laboratory of the University of Parma. 2H/H isotopes analyses were carried out the method of Kendall and [21] (reaction with Zn at 450˚C), while 18O/16O analyses based on the CO2-water equilibration technique [22]. Isotopic analysis was performed with a Finnigan Delta Plus mass spectrometer and the results are reported in ‰ units (permil deviation of the isotope ratio from the V-SMOW isotopic standard). δ is defined by the relationship:
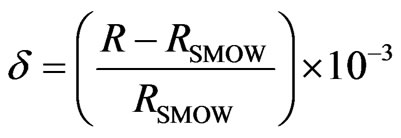 (1)
(1)
where R = D/H or 18O/16O [23]. The standard deviations of the measurements were equal to approximately ±1‰ for 2H/H and to ±0.2‰ for 18O/16O. The geochemical program PHREEQC software, version 2.10.0.0 [24], with the thermodynamic dataset wateq4f.dat, was employed to evaluate the saturation status of minerals in spring and well water samples. The SI indicates the potential for chemical equilibrium between water and minerals and the tendency for water-rock interaction [25]. If undersaturated (SI < 0), that phase, if present, feasibly could be dissolved by the groundwater and, thus, could be a potential source of constituents. Likewise, if supersaturated (SI > 0), that phase feasibly could precipitate, thus limiting the constituent concentrations. The characterization of spring and well water samples has been evaluated by means of 18O/16O and 2H/H as isotope tracers and by major ions, Ca2+, Mg2+, 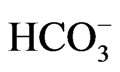 , Na2+, K+, Cl–,
, Na2+, K+, Cl–, 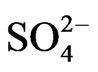 , as they are the best indicators of chemical evolution along groundwater flow paths. The chemical analysis data of the spring and well water samples have been plotted on the Piper diagram using Geochemistry Software AqQA.
, as they are the best indicators of chemical evolution along groundwater flow paths. The chemical analysis data of the spring and well water samples have been plotted on the Piper diagram using Geochemistry Software AqQA.
4. Results
Hydrochemical and stable isotope data (18O-2H) were employed to identify the factors (i.e. water types, recharge elevations and flow paths) that control the evolution of water systems of southern Latium region.
4.1. Hydrochemical Facies
Major ion concentrations and physico-chemical characteristics of the analyzed spring and well water samples are presented in Table 1. The hydrochemical facies of spring and well waters was studied by plotting the concentrations of major cations and anions in the Piper trilinear diagram. The diagram displays the relative concentrations of the major cations and anions on two separate trilinear plots, together with a central diamond plot where the points from the two trilinear plots are projected [26]. In Figure 2, major ion concentrations in meq/L for each spring (54) and well (20) water samples are reported as percentages of the total anion and cation cotent. Based on the dominance of major cationic and anionic species four hydrochemical facies have been identified in the study area: (1) Ca-Mg-HCO3; (2) Mixed Ca-Na-HCO3-Cl; (3) Ca-Cl; and (4) Na-Cl (Table 2). In all cases, CaMg-HCO3 facies predominate reflecting the main rock types in the area investigated, where limestone, dolomitic limestones and dolomites are the most dominant formations. On the contrary, Na-Cl water types dominate in the coastal aquifers where groundwater salinity is high. Most of the spring samples from Lepini Mts., labelled LPG1, belong to the Ca-HCO3 type, while the only one sample, LPG3, belongs to the Na-Cl water type. Besides, LPG2 samples show a tendency to Na-Cl-composition. In Ausoni Mts., most of the spring samples, labeled as ASG1, belong to the group of Ca-HCO3 waters. On the contrary ASG2 group, occur in the center of Piper diagram, show a tendency to the Na-Cl waters due to the proximity location of the samples to the Pontina Plain, which is affected by seawater intrusion. Spring samples grouped as ASG3, belong to the group of Na-Cl waters due to the proximity of the sampling locations to the coast. The piper diagram shows that the spring samples from Aurunci Mts. are characterized as Ca-HCO3 and Mg-HCO3 type. Groundwater samples from Pontina Plain can be split into four groups: the first one (PPG1) belongs to the Ca-HCO3 type, the second one (PPG2) fall in the field of mixed Ca-Na-HCO3-Cl type, the third one (PPG3) shows the characteristics of a typical Na-Cl water type and the fourth one (PPG4) shows the composition of Ca-Cl waters (Figure 2). Many studies have been performed on the chemical composition of saline groundwater suggesting four basic processes are associated with the hydrologic environmental characteristics of seawater intrusion.
These include: mixing of groundwater (including fluids associated with evaporates) and saltwater, carbonate precipitation and/or diagenesis (e.g. dolomitization), ion exchange and silicate (largely clay) diagenesis and redox reactions [27]. The different hydrochemical facies between four regions (Lepini, Ausoni, Aurunci Mts. and
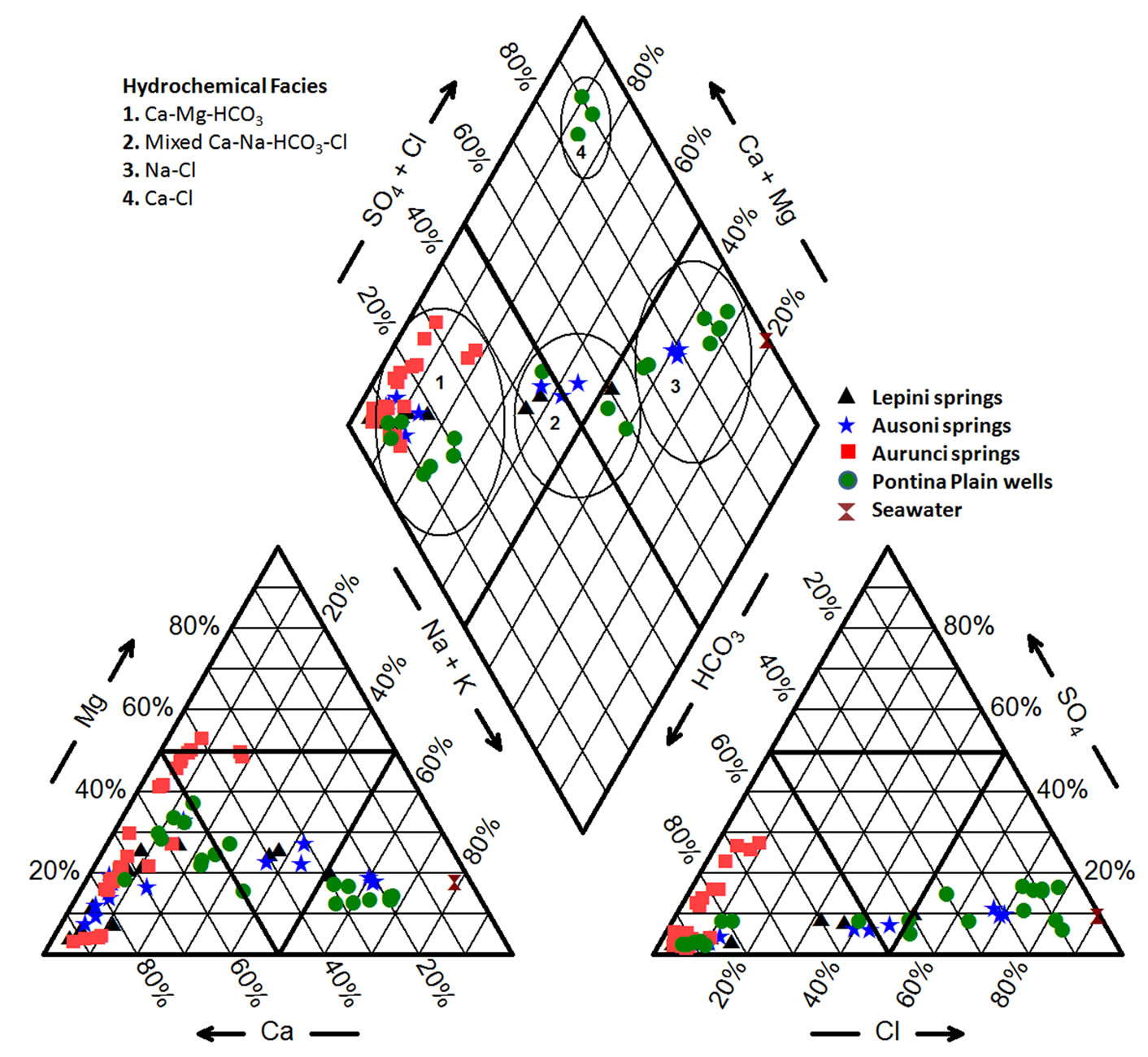
Figure 2. Piper trilinear plot for hydrochemical facies evolution and water classification.
Pontina Plain) reflects on the presence high concentrations of Na2+, Cl–, 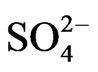 and Mg2+ in the coastal area due to seawater intrusion. Obviously, the water rock interactions still in progress. When seawater intrusion occurs, the seawater undergoes chemical changes due to the cation exchange reactions. Generally, the hydrochemical composition of fresh groundwater in coastal carbonate aquifers is typically dominated by Ca2+ and
and Mg2+ in the coastal area due to seawater intrusion. Obviously, the water rock interactions still in progress. When seawater intrusion occurs, the seawater undergoes chemical changes due to the cation exchange reactions. Generally, the hydrochemical composition of fresh groundwater in coastal carbonate aquifers is typically dominated by Ca2+ and 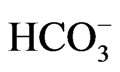 ions. Cation exchangers in the aquifer therefore have mostly Ca2+ on the exchange sites. In seawater, Na+ and Cl– are the dominant ions. Consequently, the adsorbed cation on the sediments in contact with seawater should consist dominantly of Na+. The exchange processes are relevant not only in coastal alluvial aquifers, but they also occur in karstic aquifers, where a minor presence of clays or other exchangers is sufficient to allow salinization to activate ion exchange [28]. When seawater intrudes in a coastal fresh groundwater aquifer, the cation exchange reaction takes place as follows:
ions. Cation exchangers in the aquifer therefore have mostly Ca2+ on the exchange sites. In seawater, Na+ and Cl– are the dominant ions. Consequently, the adsorbed cation on the sediments in contact with seawater should consist dominantly of Na+. The exchange processes are relevant not only in coastal alluvial aquifers, but they also occur in karstic aquifers, where a minor presence of clays or other exchangers is sufficient to allow salinization to activate ion exchange [28]. When seawater intrudes in a coastal fresh groundwater aquifer, the cation exchange reaction takes place as follows:
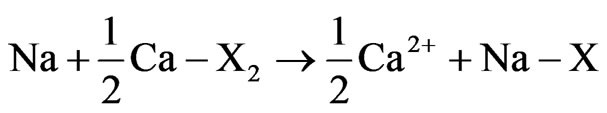 (2)
(2)
where X indicates the soil exchanger site. The equation shows that Na+ is taken up by the exchanger Ca2+, which is consequently released into the water allowing the formation of Ca-Cl water type. The reverse process can also takes place with refreshening of groundwater when fresh groundwater flushes a salt water aquifer [29]. In this case, the sediment adsorbs Ca2+ and releases Na+. This process would produce a Na-HCO3 type water. However, groundwater of the Na-HCO3 type does not exist in the study area implying that high salinity in the study area is mainly caused by recent seawater intrusion. The hydrochemical processes controlling the chemistry of spring water and groundwater will be discussed in detail later.
4.2. Physico-Chemical Characteristics of Springs and Wells
Lepini springs: The temperature of Lepini springs range from 10˚C to 15˚C. The pH of these springs ranges from 6.9 to 8.1. The Ca-HCO3 type Lepini springs (LPG1) show a total dissolved solids (TDS) content within the range 101 - 375 mg/l. Calcium and bicarbonate is the dominant constituents in spring samples, belong to the group of Ca-HCO3, followed by magnesium, sodium, sulphate and chloride. The electrical conductivity (EC) value of the springs, belong to the group LPG1, varies from 138 to 473 µS/cm. However, the springs belongs to or have a tendency to the group of Na-Cl type show an increase of total dissolved solids (751 - 1267 mg/l) and electrical conductivity (708 - 1540 µS/cm) values (Figure 3).
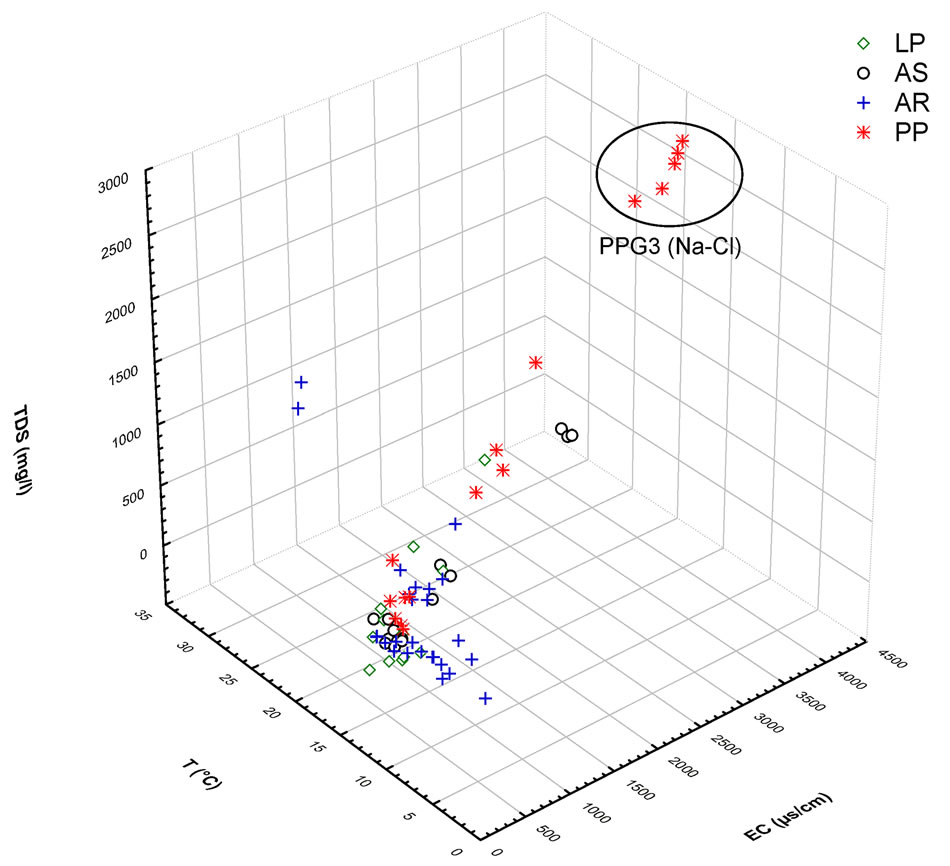
Figure 3. Electrical conductivity (EC), total dissolved solids (TDS) and temperature of spring and well water samples.
Ausoni springs: The temperature of springs ranges from 12˚C to 15˚C. The pH of the Ausoni springs ranges from 7.10 to 7.98 indicating alkaline nature of the water. The EC and TDS values of the springs, belong to the group ASG1, range from 315 to 454 µS/cm and 258 to 351 mg/l, respectively. On the contrary, spring samples of group ASG2 and ASG3 show higher EC (738 - 2310 µS/cm) and TDS (522 - 1321 mg/l) values (Figure 3). The large variation in total dissolved solids (TDS) thought to be mainly due to water-rock interaction along the flow paths and proximity of the sampling locations to the coast.
Aurunci springs: The temperature of Aurunci springs ranges from 3˚C to 15˚C except for two samples (AR01 and AR08). The high temperatures (31˚C) may be related to Roccamonfina volcanic system, which is quite close to these springs. The total dissolved solids (TDS) content ranges from 254 to 1153 mg/l. Aurunci springs are alkaline (pH: 7.23 - 8.15) with low to medium electrical conductivity. However, few springs with high total dissolved solids (TDS) and electrical conductivity (EC) is attributed to the more time for water to interact with the host rock (Figure 3).
Pontina Plain: The groundwater temperatures ranges between 12˚C and 20˚C and it increases towards the coast. The groundwater of Pontina Plain show alkaline character with pH values ranging from 7.26 to 8.0 corresponding to carbonate system waters.
As mentioned before, different hydrochemical groundwater types prevail in Pontina Plain and thus, TDS and EC values vary too much. The TDS and EC values of groundwater vary from 340 mg/l to 2804 mg/l and 490 µS/cm - 4180 µS/cm (Figure 3).
4.3. Geochemical Modeling
Geochemical modelling and saturation index computation of the Lepini, Ausoni Aurunci springs and Pontina Plain wells shows an interaction with carbonate rocks. It can be explained by means of the absorbed carbon dioxide coming from the soil, during recharge, which reacts with the carbonate rocks of the aquifer, dissolving calcite and dolomite according to the following main reactions:
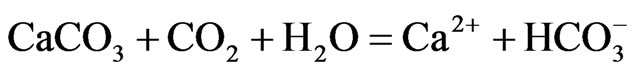 (3)
(3)
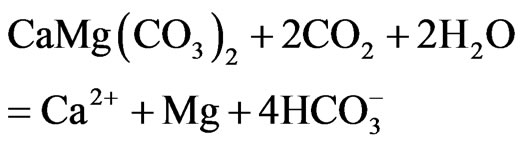 (4)
(4)
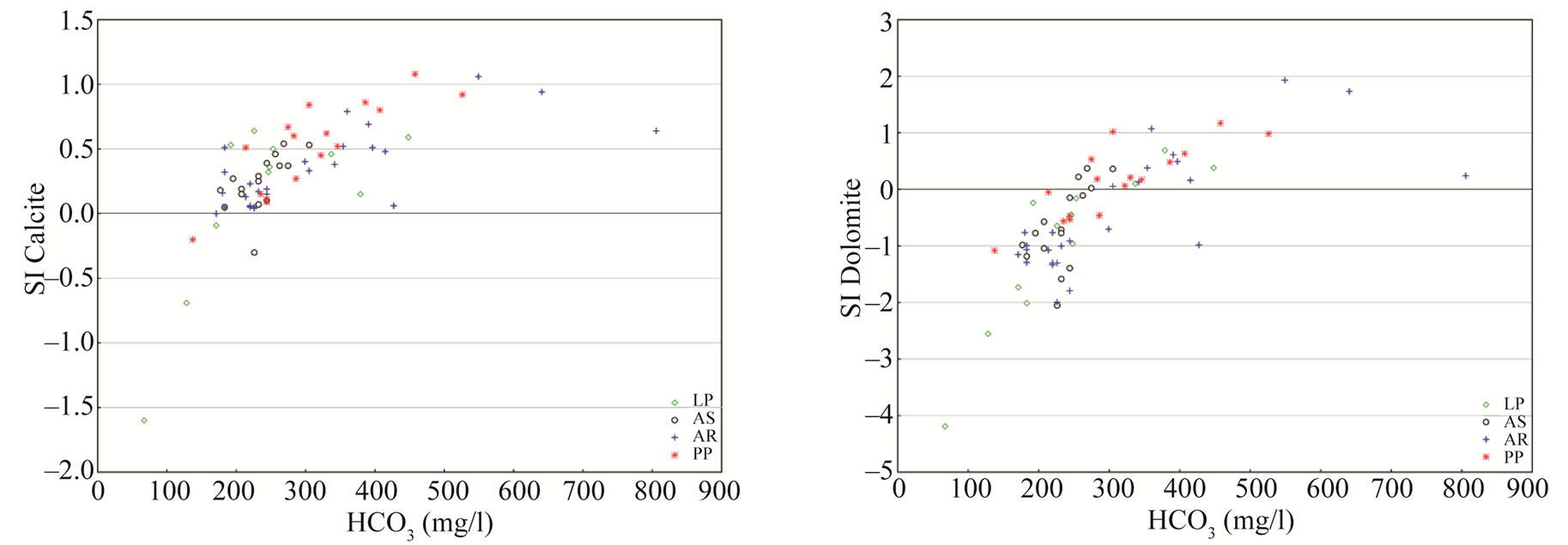
Calculated saturation indexes with respect to various mineral phases (i.e. calcite, dolomite, gypsum and halite) of the spring and groundwater samples are shown in Table 2. The positive and negative SI values represent the thermodynamic potential for precipitation and dissolution, respectively. The results of geochemical modeling suggest that most of the spring water samples from Lepini, Ausoni, Aurunci Mts. and well water samples from Pontina Plain are saturated with respect to calcite. Figures 4a and 4b show a comparison of calcite and dolomite saturation indexes of all water. samples as a function of  concentration. More than half of the samples are undersaturated with respect to dolomite, while all the sampled waters are undersaturated with respect to gypsum and halite (Figures 4(c) and (d)). However, some samples are saturated or oversaturated with respect to calcite and dolomite, which implies a great dissolution and strong mineralization along groundwater flow paths. The high dissolution rate for carbonatic rocks allows for waters close to saturation with respect to calcite and dolomite and evaporate minerals (gypsum and halite) to remain undersaturated, resulting in continued dissolution along flow paths. This indicates that the groundwater has capacity to dissolve gypsum and halite along the flow paths and hence, the concentrations of Ca2+,
concentration. More than half of the samples are undersaturated with respect to dolomite, while all the sampled waters are undersaturated with respect to gypsum and halite (Figures 4(c) and (d)). However, some samples are saturated or oversaturated with respect to calcite and dolomite, which implies a great dissolution and strong mineralization along groundwater flow paths. The high dissolution rate for carbonatic rocks allows for waters close to saturation with respect to calcite and dolomite and evaporate minerals (gypsum and halite) to remain undersaturated, resulting in continued dissolution along flow paths. This indicates that the groundwater has capacity to dissolve gypsum and halite along the flow paths and hence, the concentrations of Ca2+,  , Na+ and Cl– in the solution would increase [30]. In addition, the water samples undersaturated with respect to dolomite indicate that dolomite also can dissolve in this system adding Ca2+, Mg2+, and
, Na+ and Cl– in the solution would increase [30]. In addition, the water samples undersaturated with respect to dolomite indicate that dolomite also can dissolve in this system adding Ca2+, Mg2+, and 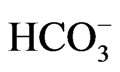 to the solution. The ionic composition may be caused by several factors during water-rock interaction, seawater intrusion and ionic exchange etc. Hence, it is necessary to use ionic ratios.
to the solution. The ionic composition may be caused by several factors during water-rock interaction, seawater intrusion and ionic exchange etc. Hence, it is necessary to use ionic ratios.
The degree of hydrochemical evolution is determined by series of ionic ratios of interest (Mg/Ca, Na/Cl, SO4/Cl, HCO3/Cl and Ca/Na) and presented in Table 2. The compositional changes in Mg and Ca concentrations mainly depend on the residence of water in karstic systems which is controlled by the volume and mechanism of recharge, the distance from the recharge area and dissolution of carbonate minerals [31-33]. Thus, this parameter can be used as a qualitative indicator of the resi-
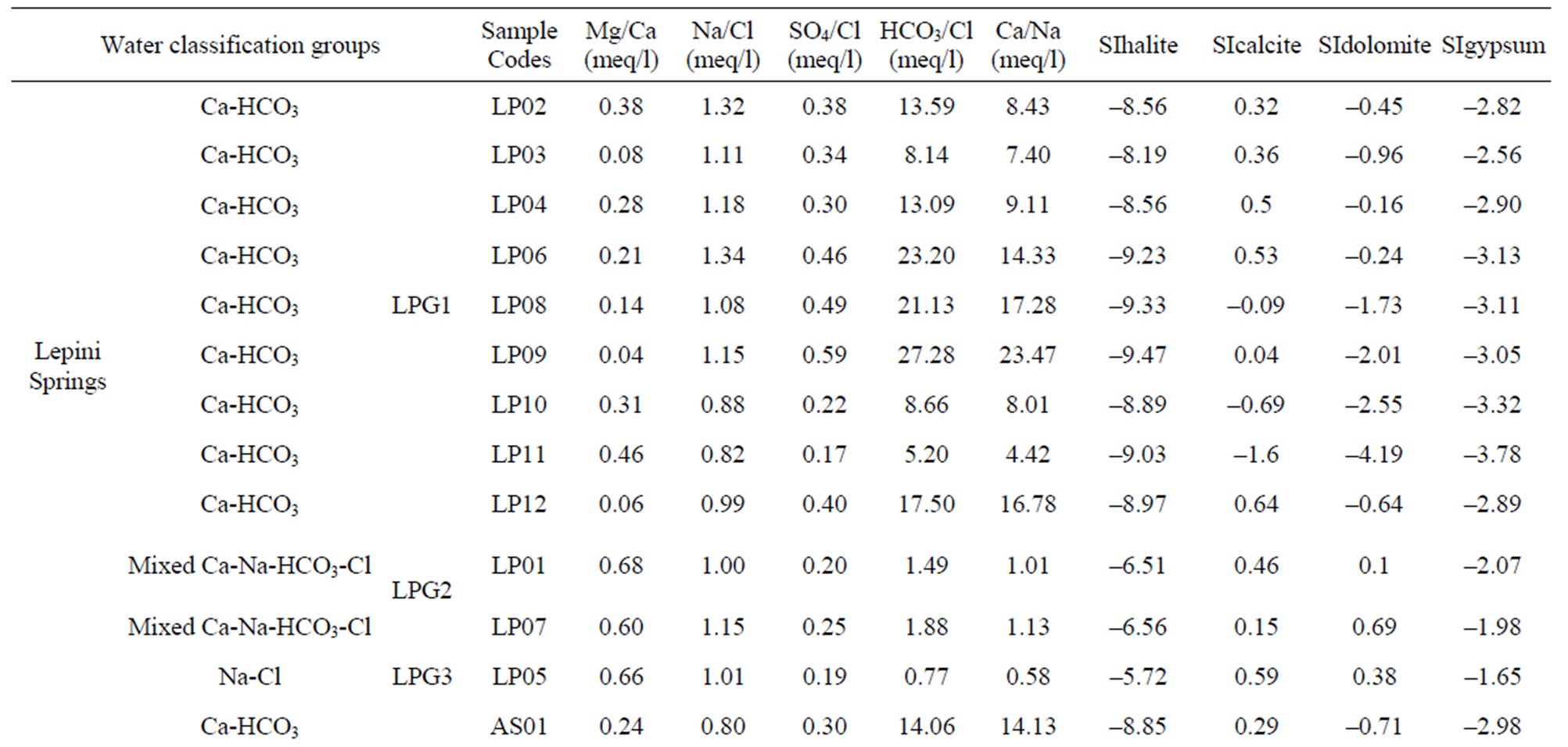
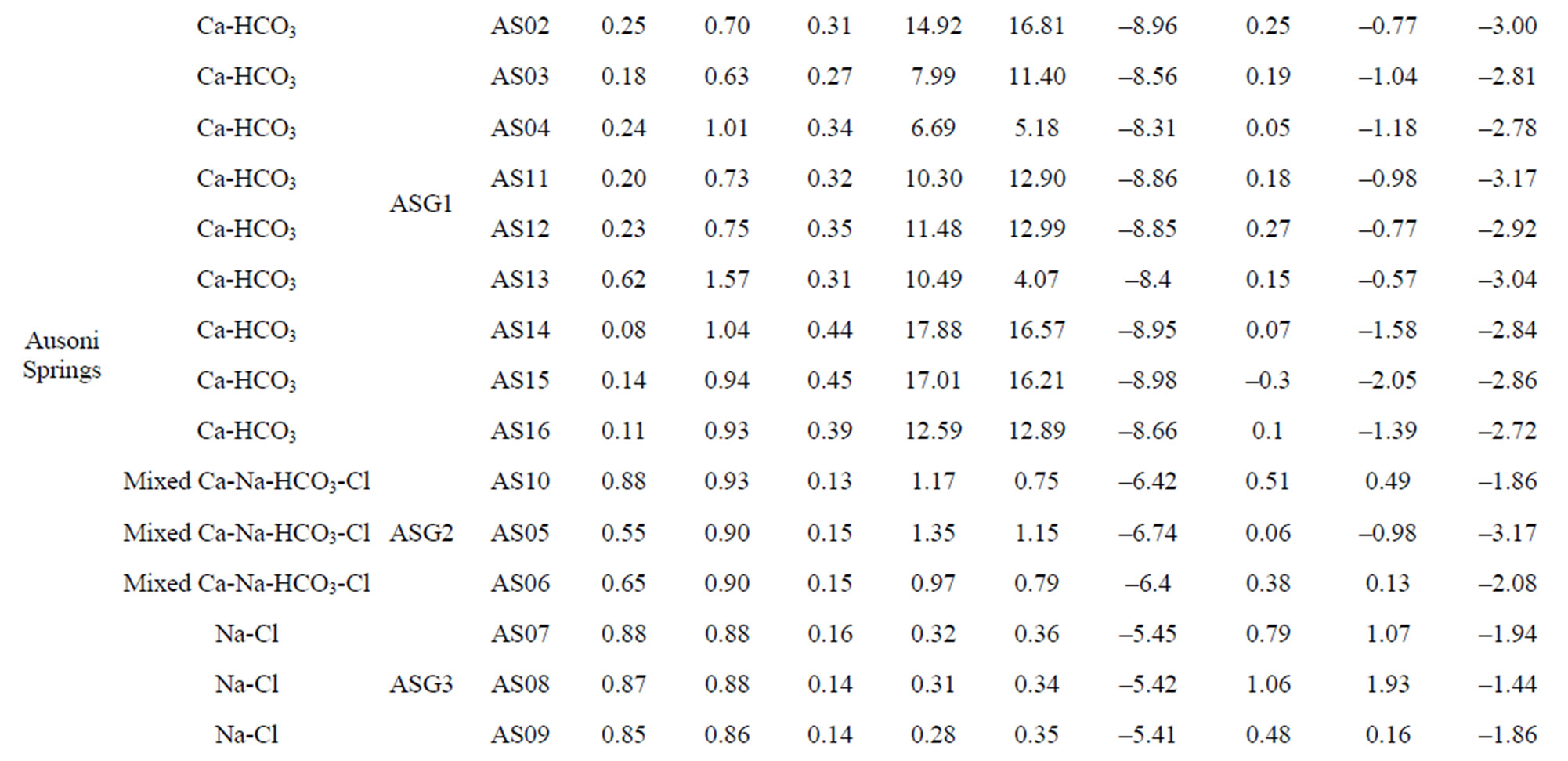
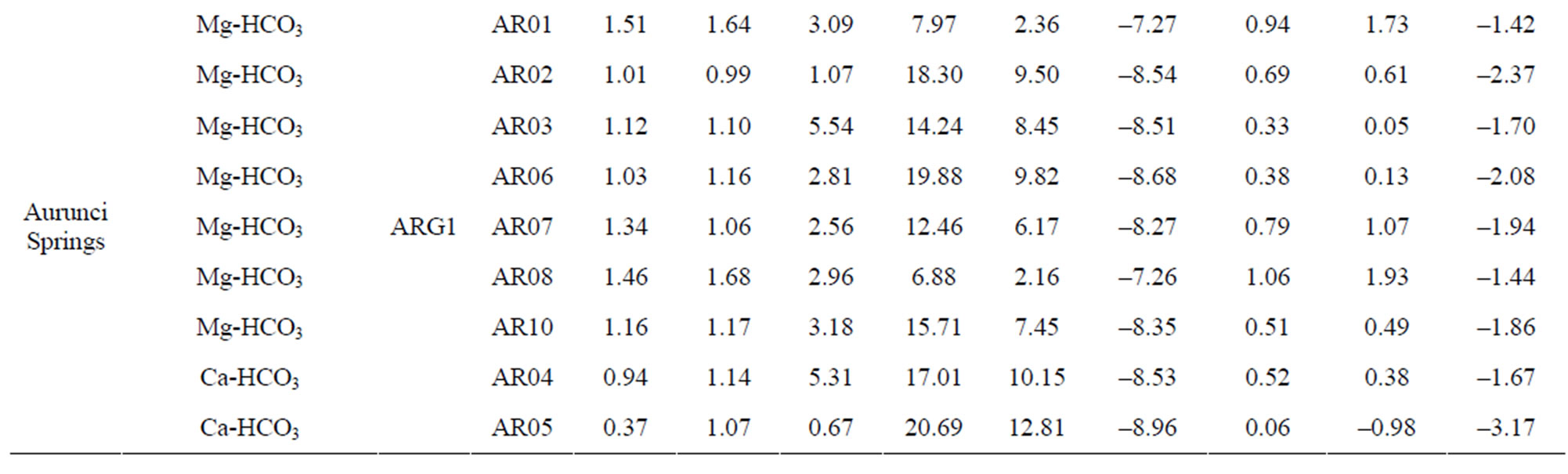

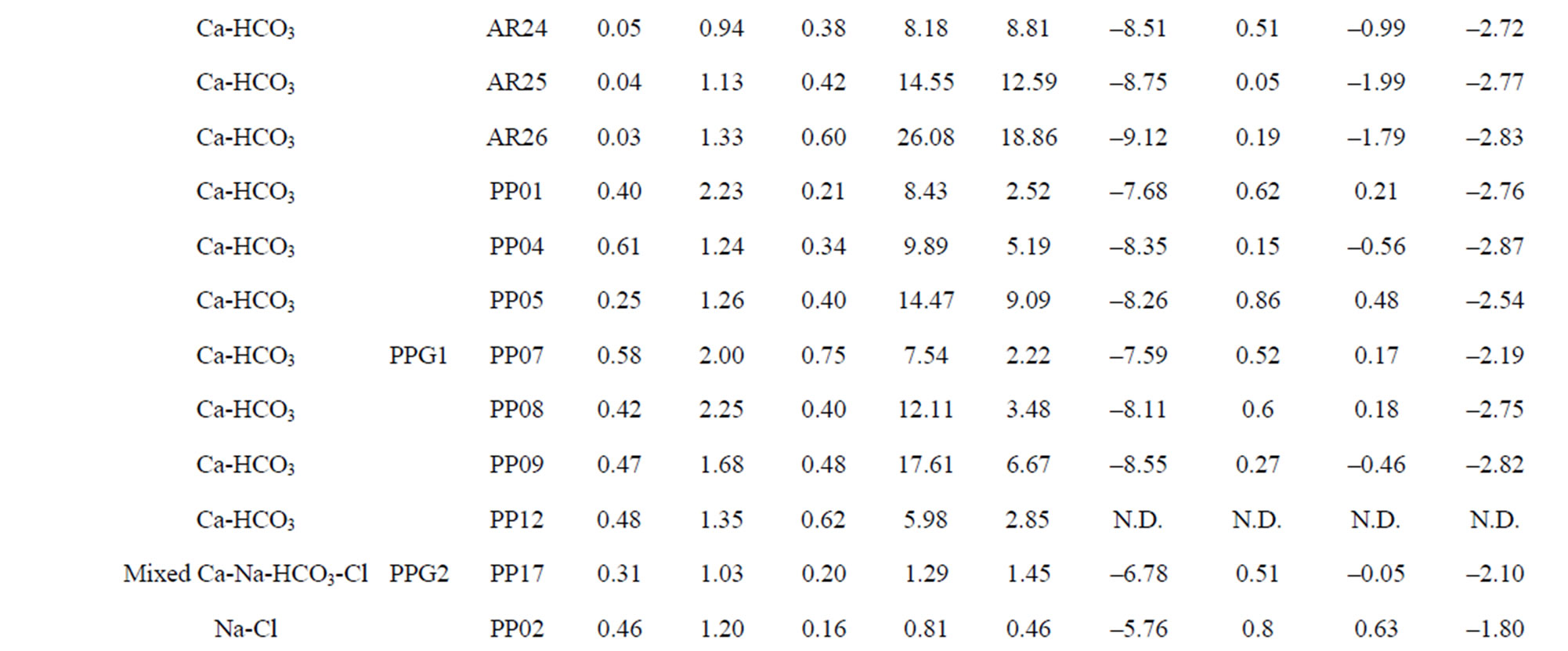

Table 2. Water classification groups. Saturation index values of calcite, dolomite, gypsum and halite for Lepini, Ausoni and Aurunci springs and Pontina Plain wells. Some ionic ratios (in meq·L−1 ) of interest from spring and well water samples in the study area.
 (a)
(a)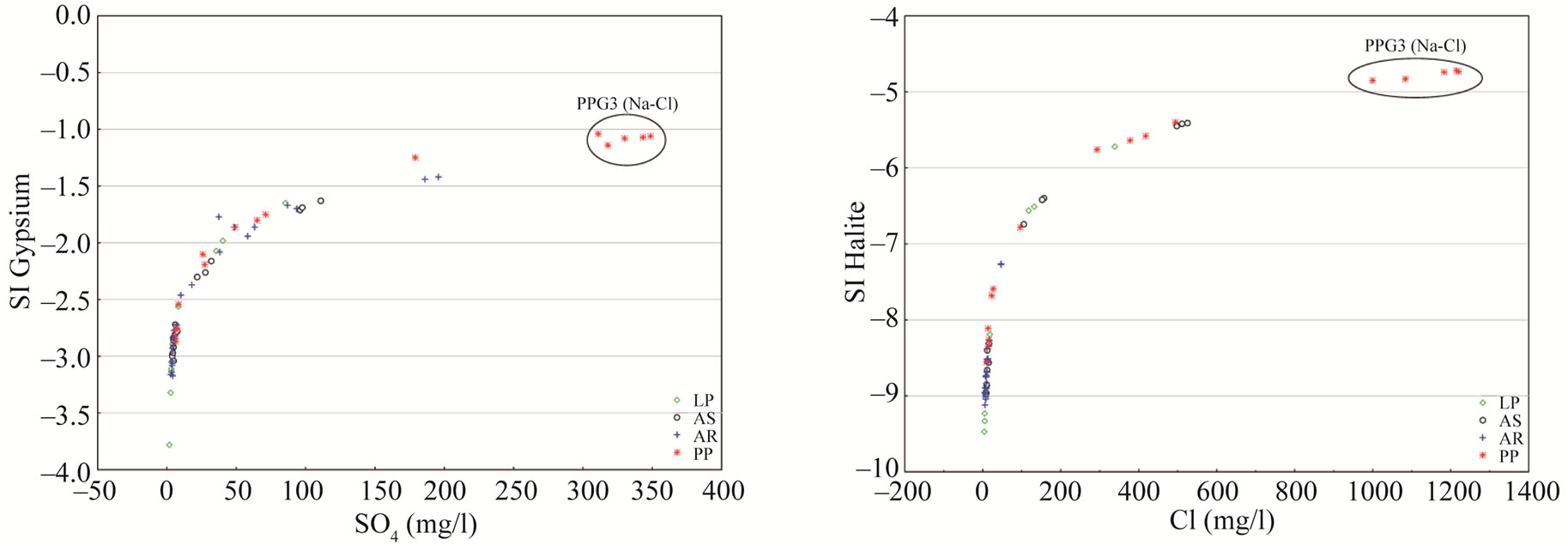 (b)
(b)
Figure 4. Saturation Index (SI) values for common carbonate and evaporate minerals of spring and well water samples. (a) Calcite saturation index versus ; (b) Dolomite saturation index versus
; (b) Dolomite saturation index versus ; (c) Gypsum saturation index versus
; (c) Gypsum saturation index versus ; (d) Halite saturation index versus Cl−.
; (d) Halite saturation index versus Cl−.
dence time of groundwater. The relationship between dolomite dissolution and calcite precipitation is thought to increase Mg/Ca ratios along flow paths (Figure 5). In fact, high elevation springs near the recharge area show the lowest Mg/Ca ratios (<0.1), while low-elevation springs farther from the recharge area show higher Mg/Ca ratios (up to 1.5) and  concentrations. Nevertheless, the increase in magnesium concentrations, and hence Mg/Ca ratio not only depends on the dissolution/precipitation reaction of calcite and dolomite, but also an increase in water temperature accelerates the kinetics of the dissolution of dolomite [34,35]. The highest Mg/Ca ratios (~1.5) were found in high temperature Aurunci springs, discharge at lower elevations, highlighting long residence time and enhanced weathering along the groundwater flow paths. These springs are oversaturated with respect to calcite and dolomite, however, they are much more oversaturated with respect to dolomite than calcite. The high Mg/Ca ratio may be due to the weathering of Mg-rich dolomite, where dolomitic limestones and dolomites are the most dominant formations in this area. Gypsum and halite may participate in water-rock interactions, probably as natural salts, which are more soluble than calcite and dolomite and will further affect the amount and type of dissolved solids in groundwater. Samples with the lowest sulfate concentration, and therefore gypsum saturation index, are found in high elevation springs near the recharge area. However, springs farther from the recharge areas have the highest sulfate concentrations. If Ca2+, Mg2+, SO42– and
concentrations. Nevertheless, the increase in magnesium concentrations, and hence Mg/Ca ratio not only depends on the dissolution/precipitation reaction of calcite and dolomite, but also an increase in water temperature accelerates the kinetics of the dissolution of dolomite [34,35]. The highest Mg/Ca ratios (~1.5) were found in high temperature Aurunci springs, discharge at lower elevations, highlighting long residence time and enhanced weathering along the groundwater flow paths. These springs are oversaturated with respect to calcite and dolomite, however, they are much more oversaturated with respect to dolomite than calcite. The high Mg/Ca ratio may be due to the weathering of Mg-rich dolomite, where dolomitic limestones and dolomites are the most dominant formations in this area. Gypsum and halite may participate in water-rock interactions, probably as natural salts, which are more soluble than calcite and dolomite and will further affect the amount and type of dissolved solids in groundwater. Samples with the lowest sulfate concentration, and therefore gypsum saturation index, are found in high elevation springs near the recharge area. However, springs farther from the recharge areas have the highest sulfate concentrations. If Ca2+, Mg2+, SO42– and  were only derived from the dissolution of carbonate (calcite and dolomite) and evaporate minerals (gypsum), ionic ratios of (Ca + Mg) to (SO4 + HCO3) or Na/Cl should be a constant value of one [36]. Thus, the plot of (Ca + Mg) versus (HCO3 + SO4) was prepared to identify the ion exchange and weathering processes (Figure 6). As can be seen in Figure 6, most of the spring and well water samples fall along the 1:1 equiline suggesting that these ions have resulted from weathering of carbonates. However, well water samples from Pontina Plain (groups PPG4 and PPG3) and spring samples from Ausoni Mts. (group ASG3) are clustered above the 1:1 line indicating ion exchange process. Generally, if halite dissolution is responsible for sodium and chloride, the Na/Cl molar ratio
were only derived from the dissolution of carbonate (calcite and dolomite) and evaporate minerals (gypsum), ionic ratios of (Ca + Mg) to (SO4 + HCO3) or Na/Cl should be a constant value of one [36]. Thus, the plot of (Ca + Mg) versus (HCO3 + SO4) was prepared to identify the ion exchange and weathering processes (Figure 6). As can be seen in Figure 6, most of the spring and well water samples fall along the 1:1 equiline suggesting that these ions have resulted from weathering of carbonates. However, well water samples from Pontina Plain (groups PPG4 and PPG3) and spring samples from Ausoni Mts. (group ASG3) are clustered above the 1:1 line indicating ion exchange process. Generally, if halite dissolution is responsible for sodium and chloride, the Na/Cl molar ratio
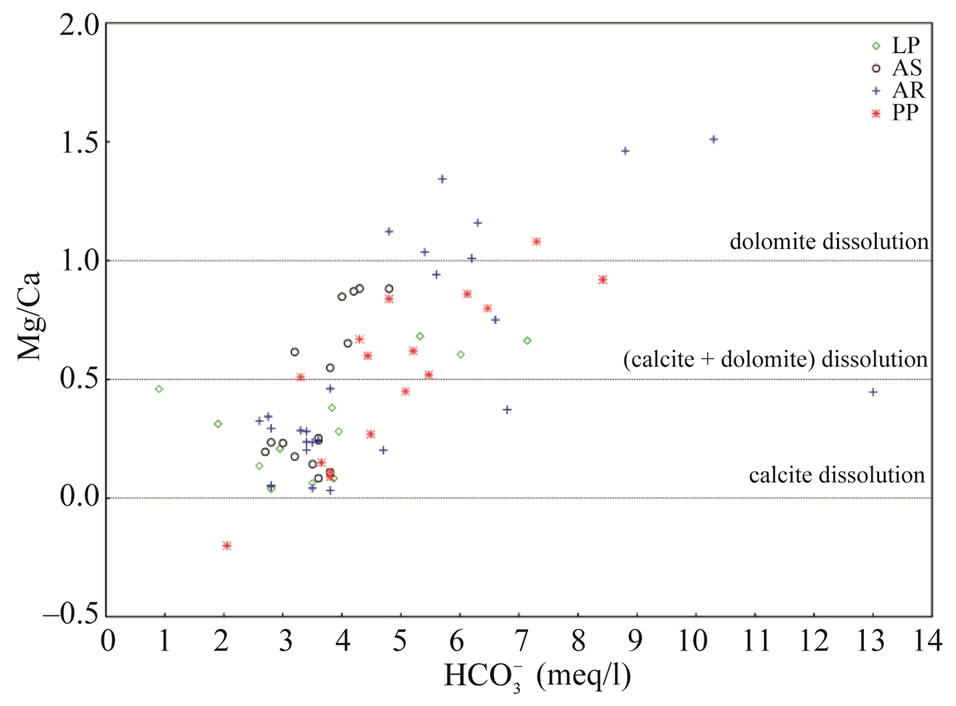
Figure 5. Mg/Ca vs.  in meq/l.
in meq/l.
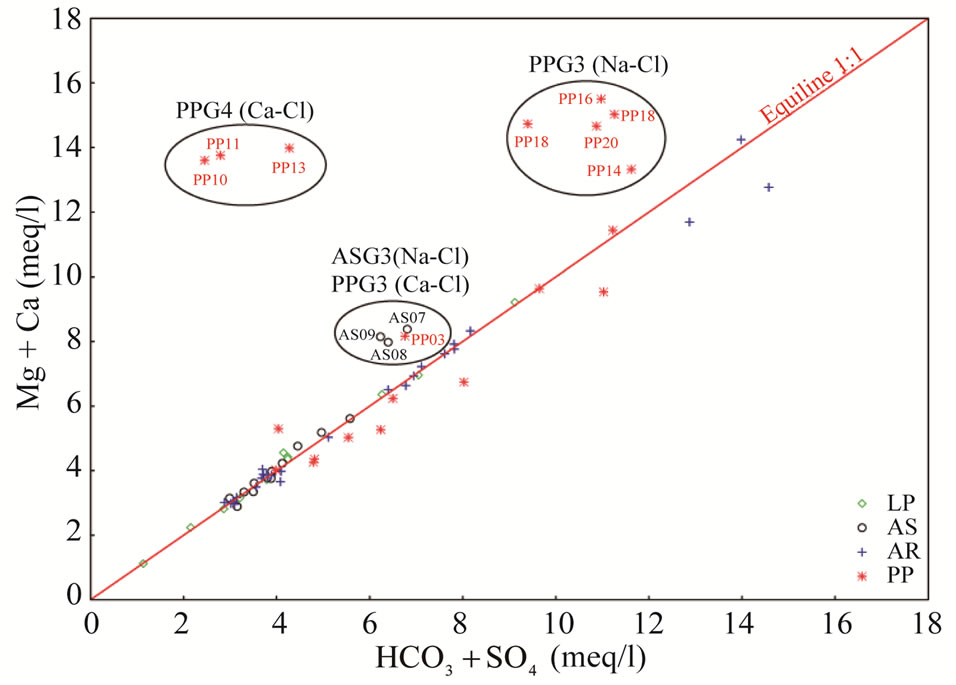
Figure 6. Plot of Ca + Mg vs. HCO3 + SO4 in meq/l.
should be approximately one [37].
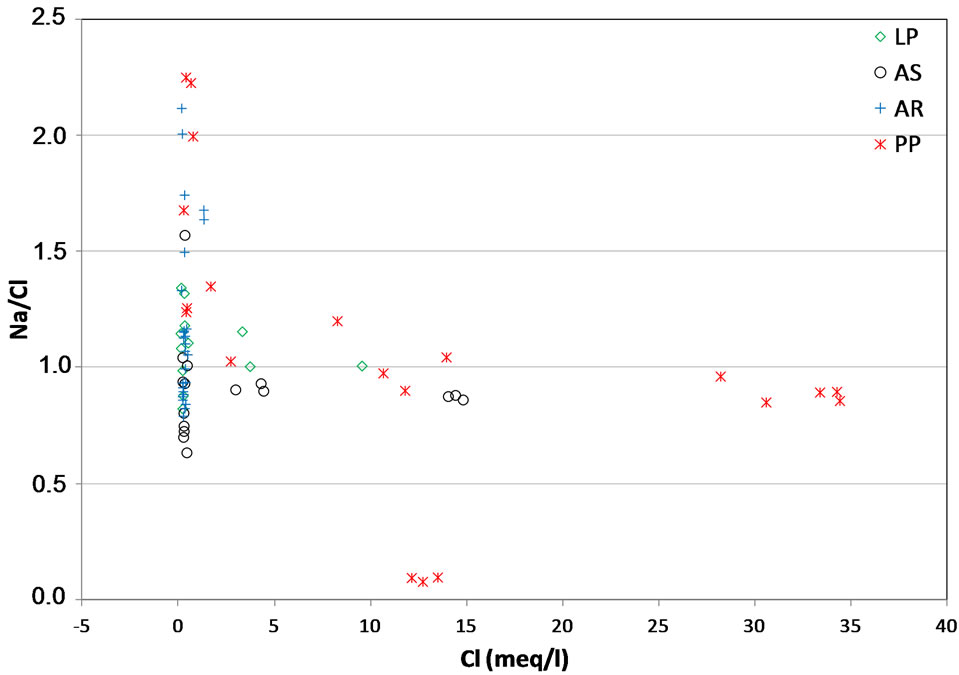
The Na/Cl ratios of spring and well water samples range from 0.08 to 2.25 (Table 2). Majority of the samples have molar ratio greater or equal to one, which indicates Na2+ may increase due to ion exchange and halite dissolution. However, some well water samples from Pontina Plain and spring samples from Ausoni Mts. show Na/Cl molar ratio close to and less than the value in seawater (0.86) indicating possible seawater intrusion, where groundwater becomes enriched in Cl– that leads to decrease the value of this ratio. The lower values of this ratio may be attributed to depletion of Na probably caused by cation exchange through clastics (mainly clays and marly limestone). The plot of Na/Cl and SO4/Cl ratios versus Cl– concentrations suggest that these ratios increase with reduced salinity and vary between water types (Figures 7(a) and (b)). The HCO3/Cl ratios give a clear picture of the relative concentration of chloride and bicarbonate. Thus, this ratio can be a good indicator for salinization. The ratios of HCO3/Cl, indicative of freshwater recharge, are all greater (>5), however groundwater from Pontina Plain, belong to groups PPG3 and PPG4, and Ausoni (ASG2 and ASG3 groups) and Lepini (LPG2 and LPG3 groups) springs have values of HCO3/Cl ratio close to the seawater ratio indicating possible mixing with seawater, where groundwater becomes enriched in Cl– that leads to decrease the value of this ratio (Figure 7(c)). These spring and well water samples show also low Ca/Na ratios close to the seawater ratio (0.04), however for the springs and groundwater belong to Ca-MgHCO3 type, Ca/Na molar ratios are much more than those of seawater, as the carbonate dissolution and/or depletion of Na become the prevailing processes in the aquifer matrix (Figure 7(d)). In addition, bromide ions are considered good indicators of seawater intrusion in the coastal aquifers due to their similar geochemical behavior and low chemical activity [38].
Br and Cl are relatively conservative in hydrological systems and the Br/Cl ratios are often associated to study the origin of chloride and the anomalies of salinity. In the study area, Ca-Mg-HCO3 type spring and well water samples show low Br values (<0.01 mg/l), however for Na-Cl type waters Br/Cl ratios similar to normal and modern seawater ratio of 0.0015 to 0.003, respectively [39,40].
4.4. Stable Isotope Analysis of Spring and Well Water
The isotopic composition (18O and 2H) and the calculated deuterium excess values of spring and well water samples, recorded in different periods, are presented in Table 3. For the isotopic characterization of the spring samples from Lepini, Ausoni and Aurunci Mountains and the well samples from Pontina Plain, we plot the isotope data of sampled waters in the binary diagram of δ2H-δ18O (Figure 8). The Mediterranean meteoric water line (MMWL), [41], the world meteoric water line (WMWL), [42], and the central Italy meteoric water line (CIMWL), [43], are also plotted on the same diagram. The equations describe the relationship between 18O and 2H are the following:
 (5)
(5)
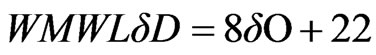 (6)
(6)
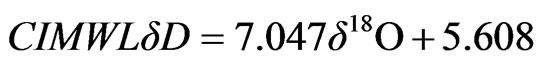 (7)
(7)
The comparison of δ18O and δ2H values of spring and well water samples with meteoric water lines (Figure 8) shows that most of the samples fall to the left of the world meteoric water line suggesting input to local rainfall that derives from weather fronts coming from the Mediterranean Sea.
The precipitation of Mediterranean origin should contribute to the recharge of the regional aquifers.
The deviation of data points from the meteoric lines
 (a)
(a)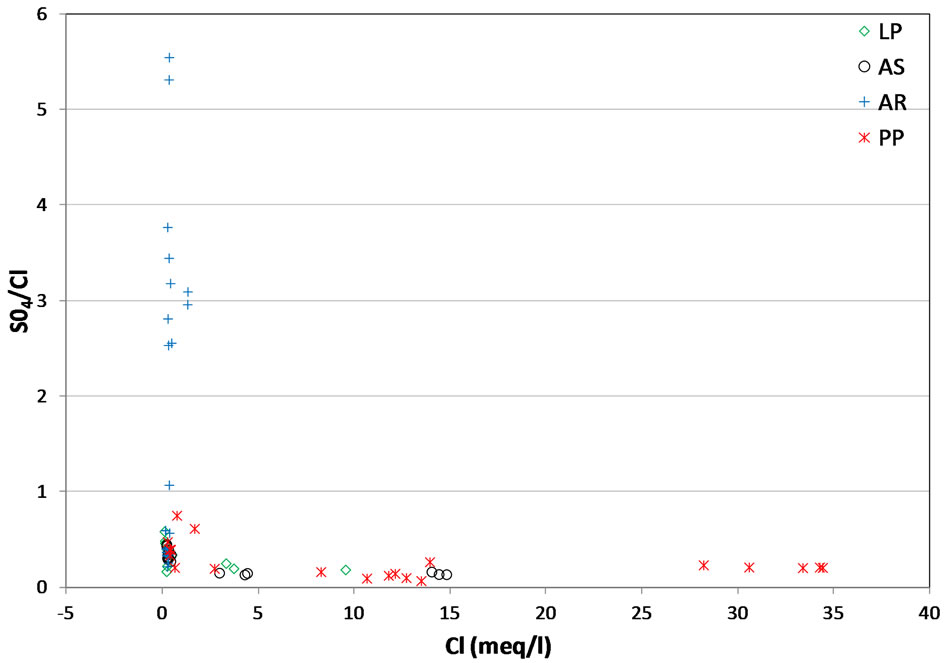 (b)
(b)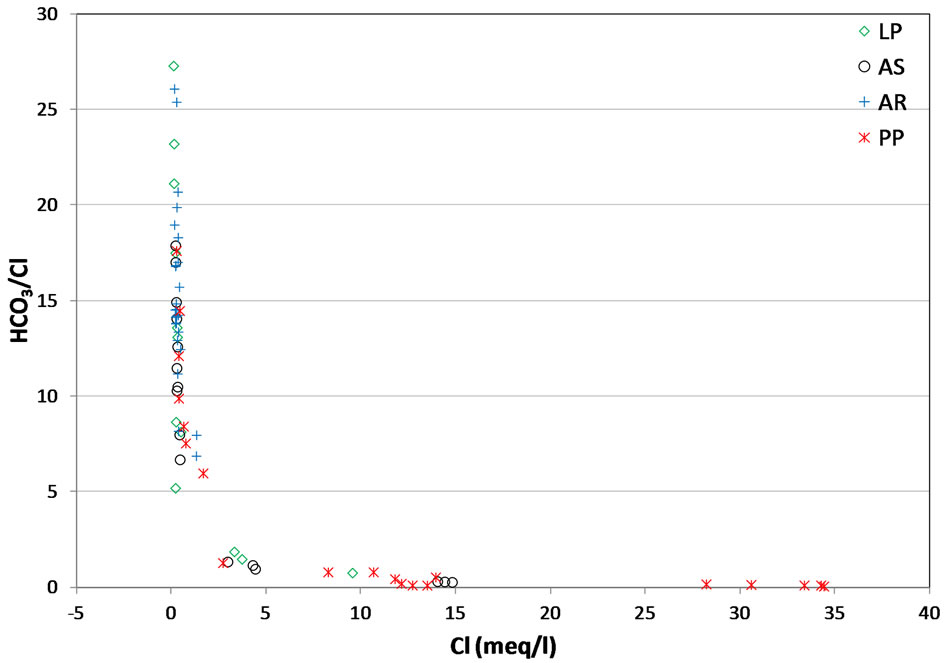 (c)
(c)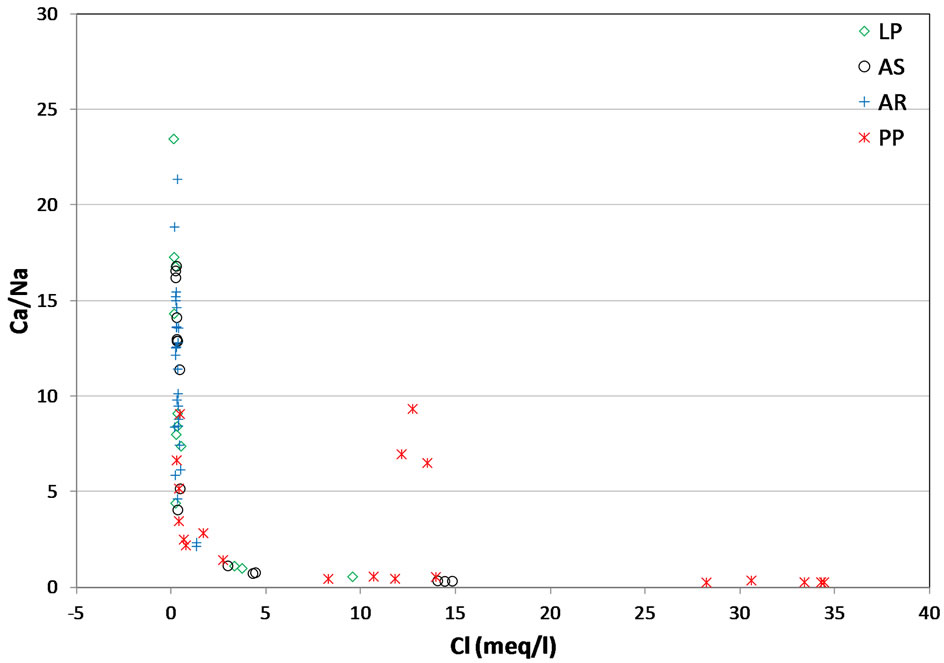 (d)
(d)
Figure 7. (a) Relationship between Cl vs. Na/Cl; (b) Relationship between Cl vs. SO4/Cl; (c) Relationship between Cl vs. HCO3/Cl; (d) Relationship between Cl vs. Ca/Na.
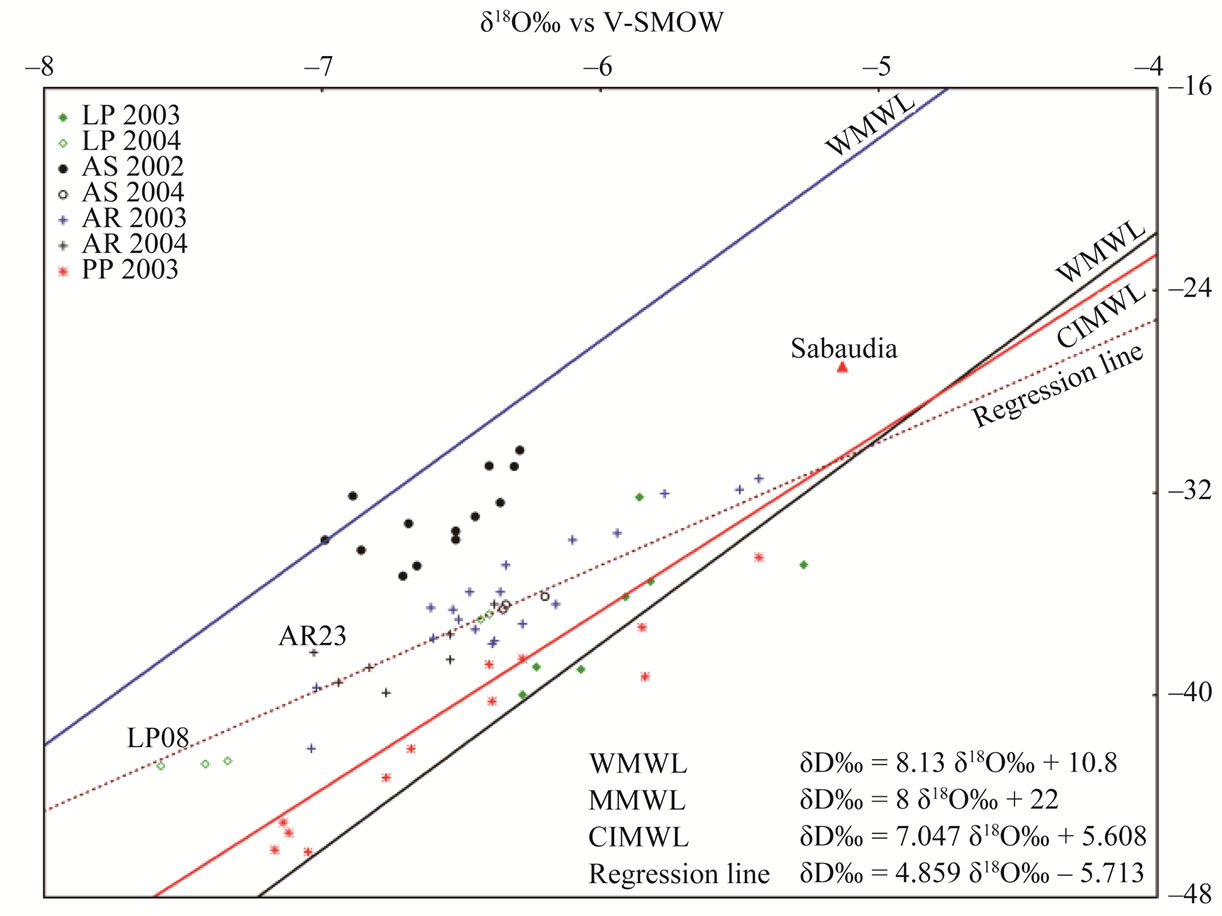
Figure 8. Isotope values (δ2H and δ18O) of spring water samples from Lepini, Asusoni and Auruci Mts. and well water samples from Pontina Plain. Samples plotted together with the Mediterranean Meteoric Water Line (MMWL) (Gat and Carmi, 1970), the World Meteoric Water Line (WMWL) (Rozanski et al., 1993) and the Central Italy Meteoric Water Line (CIMWL) (Longinelli and Selmo, 2003).
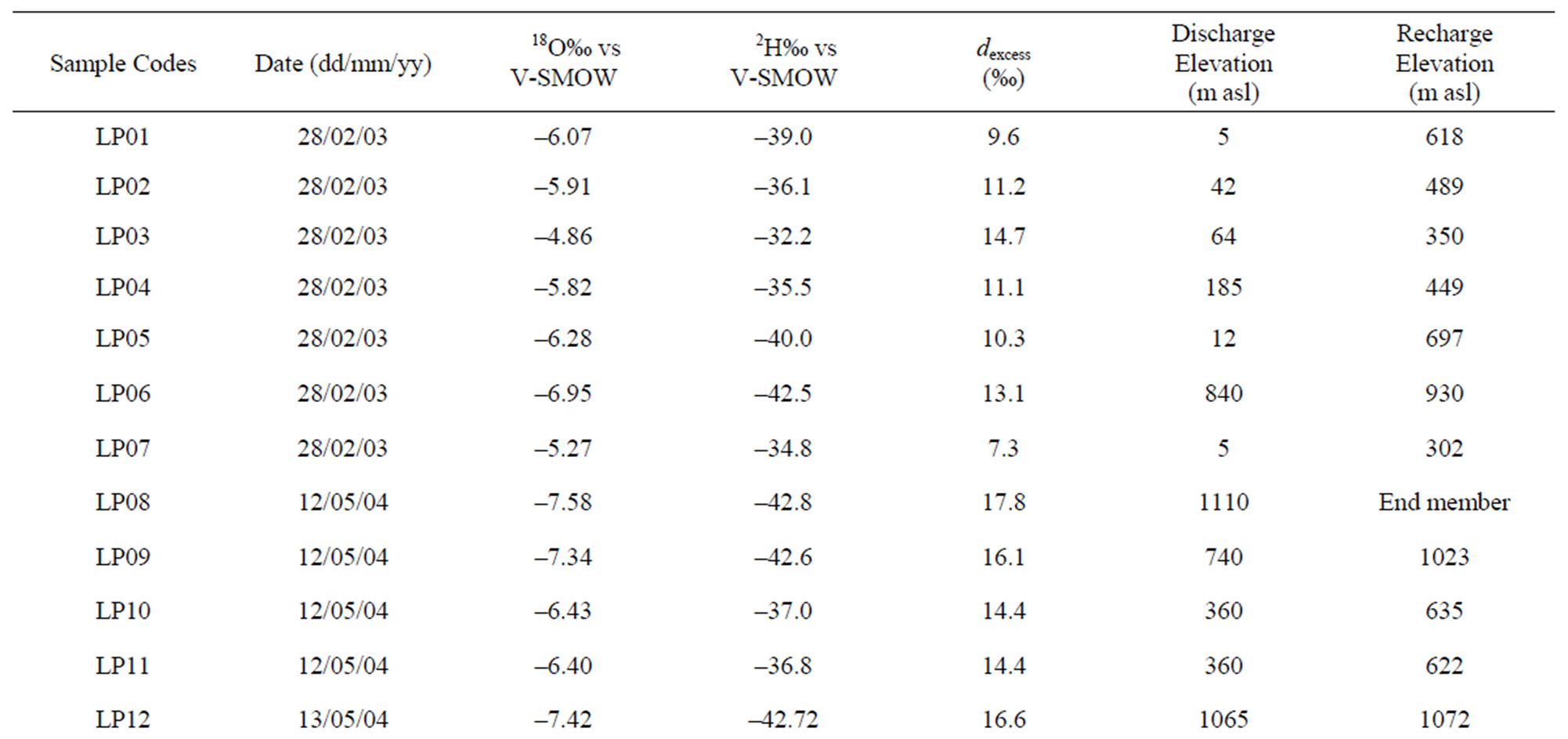
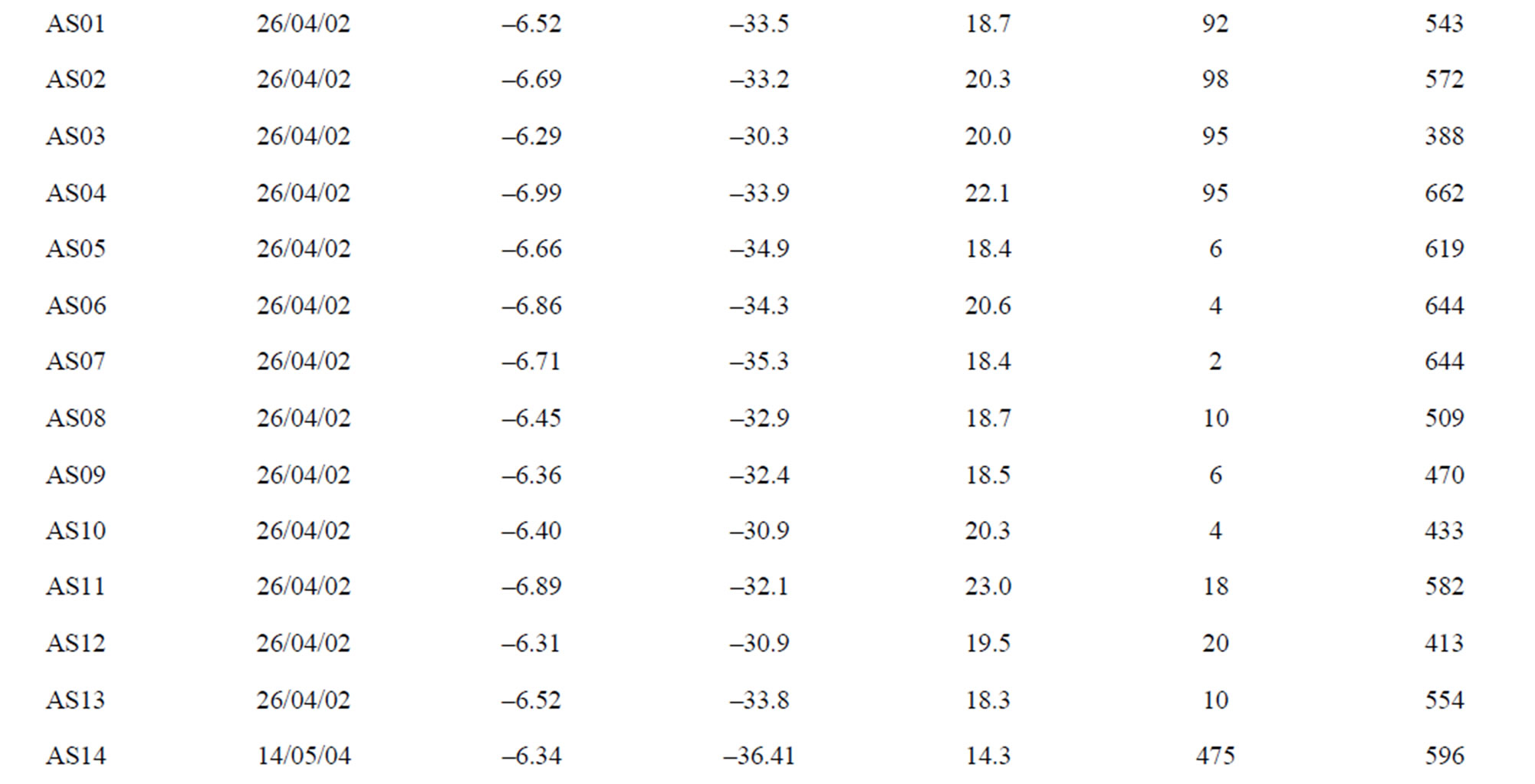
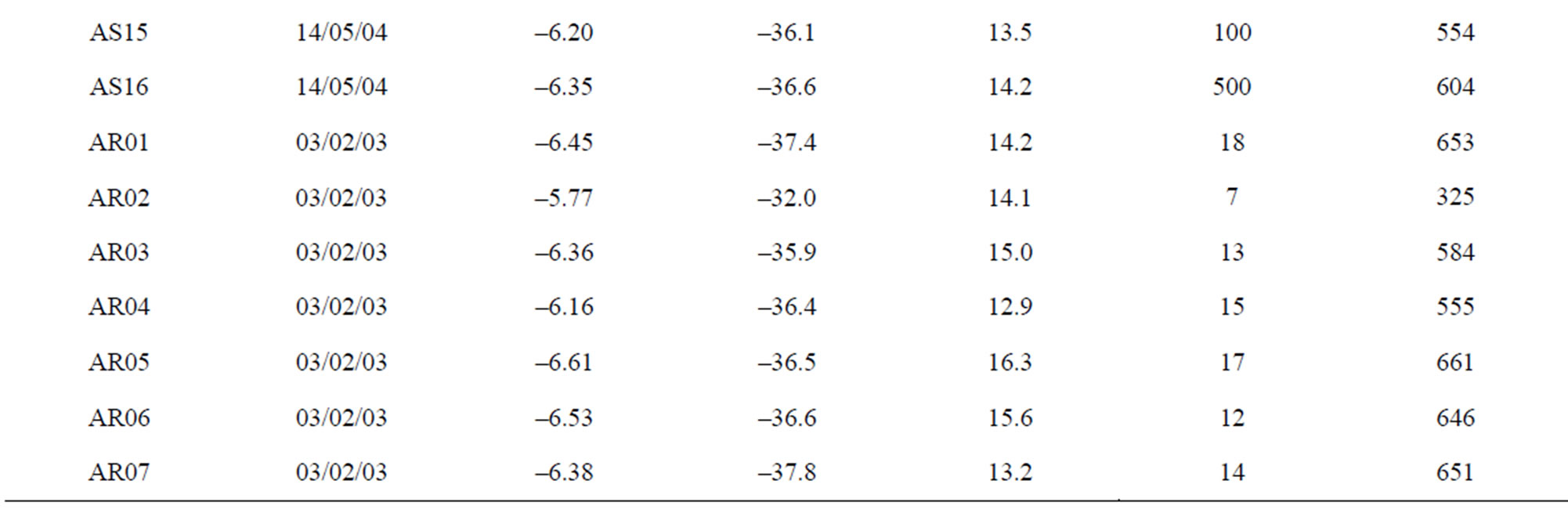
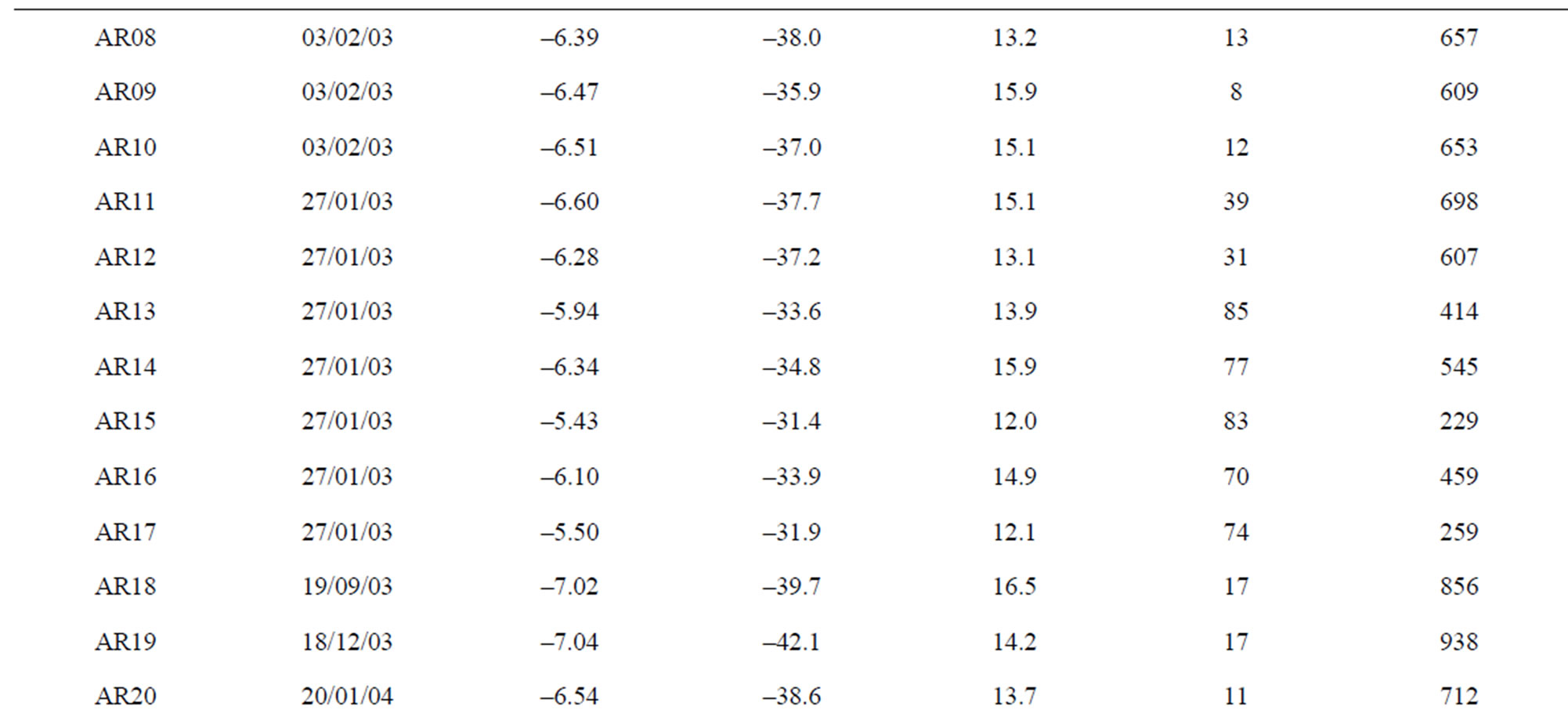
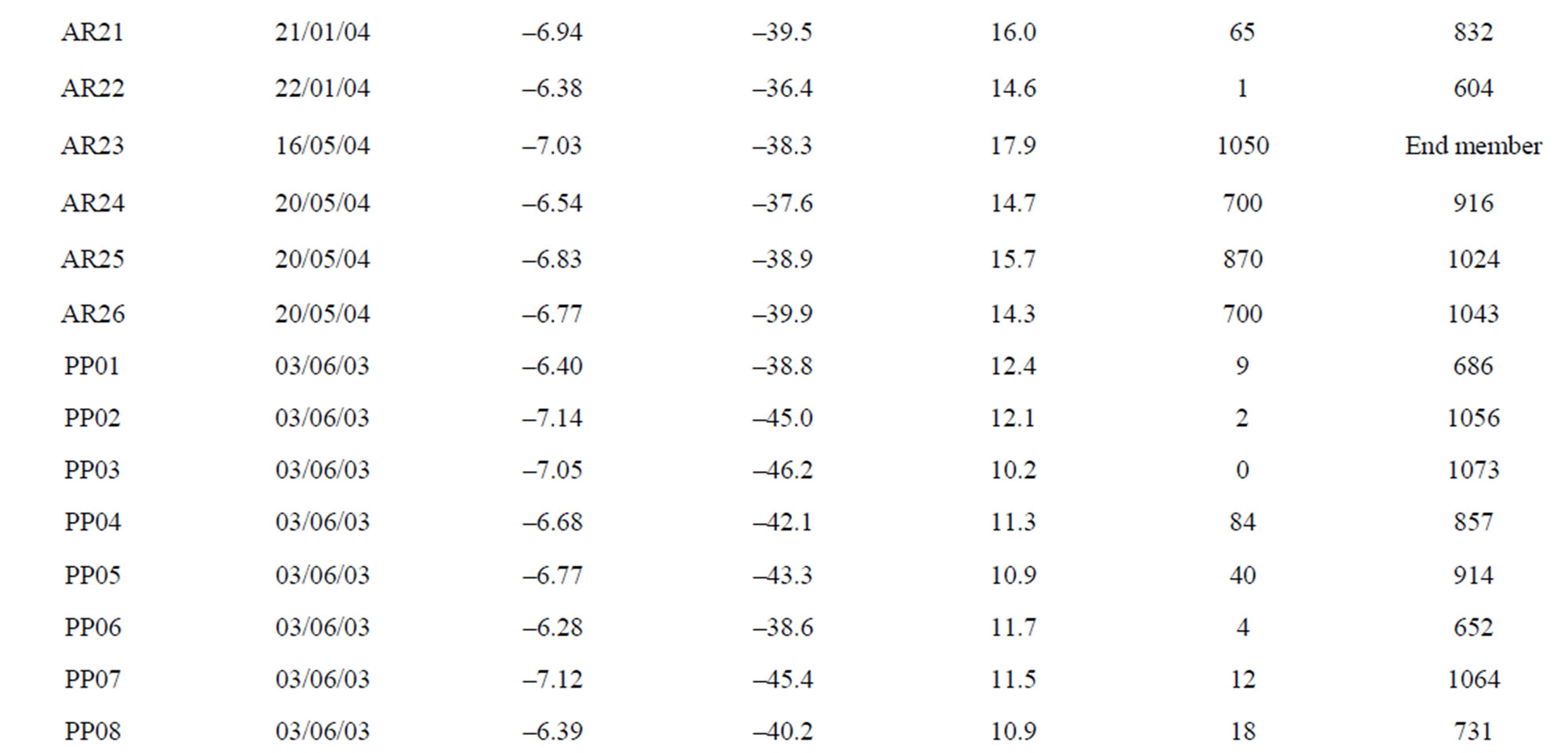
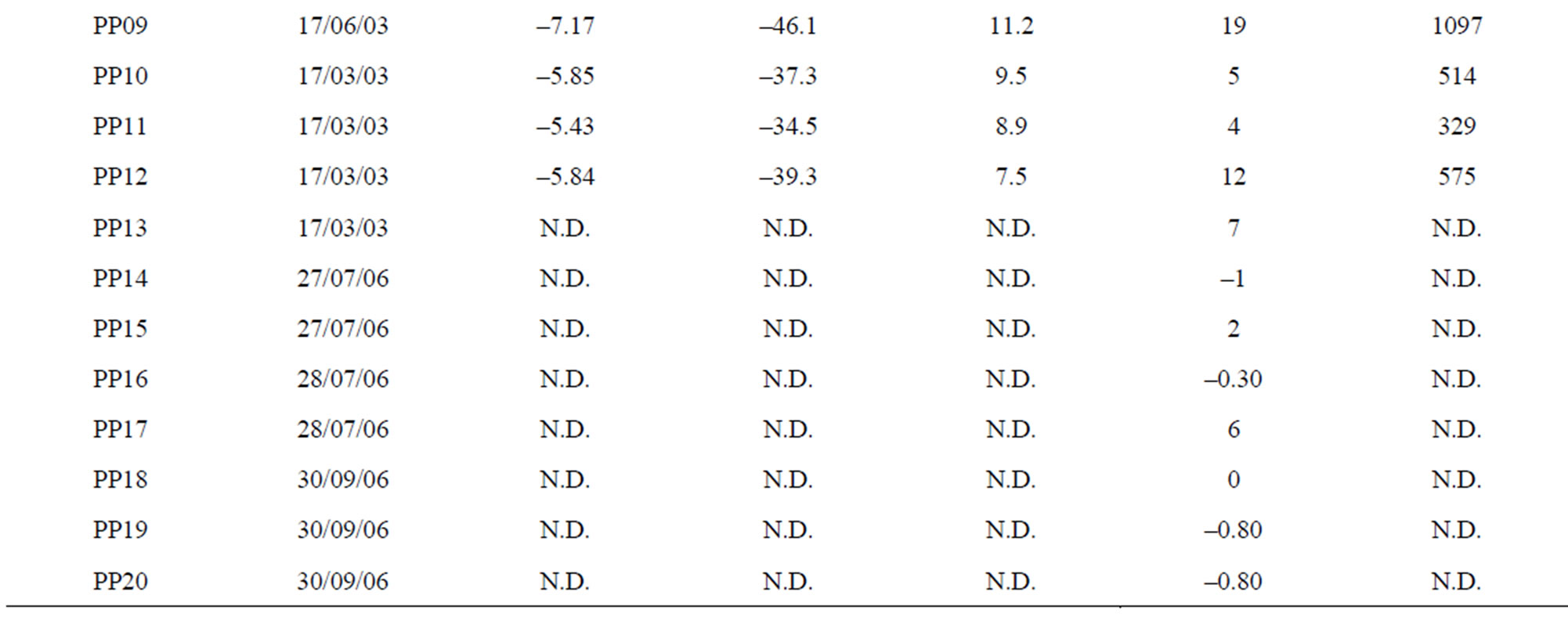
Table 3. Isotope composition, deuterium excess (dexcess) values and calculated recharge elevations of spring and well water samples.
can be attributed to evaporation both during the falling of the rain and by run-off on the ground surface before infiltration, i.e. the dotted line shows linear regression of all data. A slope of 4 to 6 is attributed to waters with a significant rate of evaporation relative to input [44,45]. The dotted line has a slope of 4.8 and is calculated from a linear regression on all data. Most of the samples from Lepini springs fall above and below the CIWML, however, spring samples LP01 and LP07 plot below the WMWL suggesting that the water has evaporated or has mixed with evaporated water. The plot of δ2H values versus δ18O for spring samples from Ausoni Mts., collected during April 2002, shows a distribution which tends to converge with the MMWL showing more δ18O enrichment at the expense of δ2H. Samples AS14, AS15 and AS16, collected during May 2004, fall above the CIWML. The measured isotope values of the spring samples from Aurunci Mts., are aligned near to and above the CIWML. In Pontina Plain, the isotope compositions of the groundwater samples plot below the CIWML and close to WMWL. However, PP10, PP11 and PP12, samples fall below the WMWL: it may be related to an evaporation process from falling rain or before infiltration through soils and sand surfaces in the recharge areas (Figure 8).
A deuterium excess of 10‰, the average value for global precipitation, is significantly lower than that of Eastern Mediterranean meteoric water line (EMMWL), where a significant part of the vapour mass has originnated in a closed basin. The low d-values of precipitation reflect slow evaporation at its source region due to high humidity, whereas the high d-values reflect fast evaporation at its source region due to low humidity [3]. Previous studies showed that the western part of the Mediterranean basin has a d-excess of 14‰, whereas the eastern part shows an excess of + 22‰ and this variation reflects a mixture between the Mediterranean and the Atlantic air masses [46]. These high values probably are related to a strong kinetic isotopic effect during evaporation in the summer above the Mediterranean Sea because of the low relative humidity of the atmosphere. The values of the deuterium excess (d) of spring and well water samples were calculated by the following equation [47]:
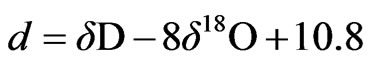 (8)
(8)
The relationship between δ18O and δ2H, for spring and well water samples, shows shifts of both the slope andthe deuterium excess when compared to the global and central Italy meteoric water lines.
The deuterium excess values has been found in the study area with a range of “d” values minimum +7.3‰ in February 2003 and maximum + 23‰ in April 2002 (Table 3). These values include the range from 10 for global precipitation to 22 for the eastern Mediterranean area indicating the significance of the Mediterranean as a moisture source for Italy [48]. Most of the spring samples with high deuterium excess values (above 14‰) suggest that the precipitation in the groundwater comes from the Mediterranean sector (Figure 9). On the contrary, groundwater samples from Pontina Plain show low d-excess values (ranges from 7.5‰ to 12.5‰).
A d-parameter value around 10 ‰ indicates that the water has not been significantly evaporated, and plots on the WMWL and close to the LMWL. The spring and well water samples plot below WMWL show low deuterium excess values (<10‰) indicating the groundwater is isotopically different from its original isotopic composition due to evaporation.
The altitude effect has been used to estimate the mean elevation of recharge of spring and well water samples. The mean isotopic gradient with altitude can be determined directly from the samples collected at a series of sites located at different altitudes, as well as from a series of rainwater samples obtained by different pluviometric stations. As no there is no precipitations isotope data were available for precipitation in the area under study, for the identification of mean isotope gradients, we use the isotope (18O-2H) and elevation values of precipitation, obtained from the four sampling pluviometers near study area (Sabaudia, Roccamonfina, Vairano 1 and Vairano 2).
This methodological process is used to confirm the hypothesis of the conceptual hydrogeological model about groundwater circulation. The altitude effect is found by the relation between precipitation isotope values and elevation in meters (h) highlighting a depletion of heavy stable isotopes of about –0.22‰ and –1.55‰ per 100 m elevation for δ18O and δ2H, respectively (Figure 10). Then, the calculated gradients (Δ18O/Δh = –0.22‰/100 m and Δ2H/Δh = –1.55‰/100 m) were used to obtain information about recharge elevations for each spring

Figure 9. Box plot of deuterium excess values of spring and well water samples.
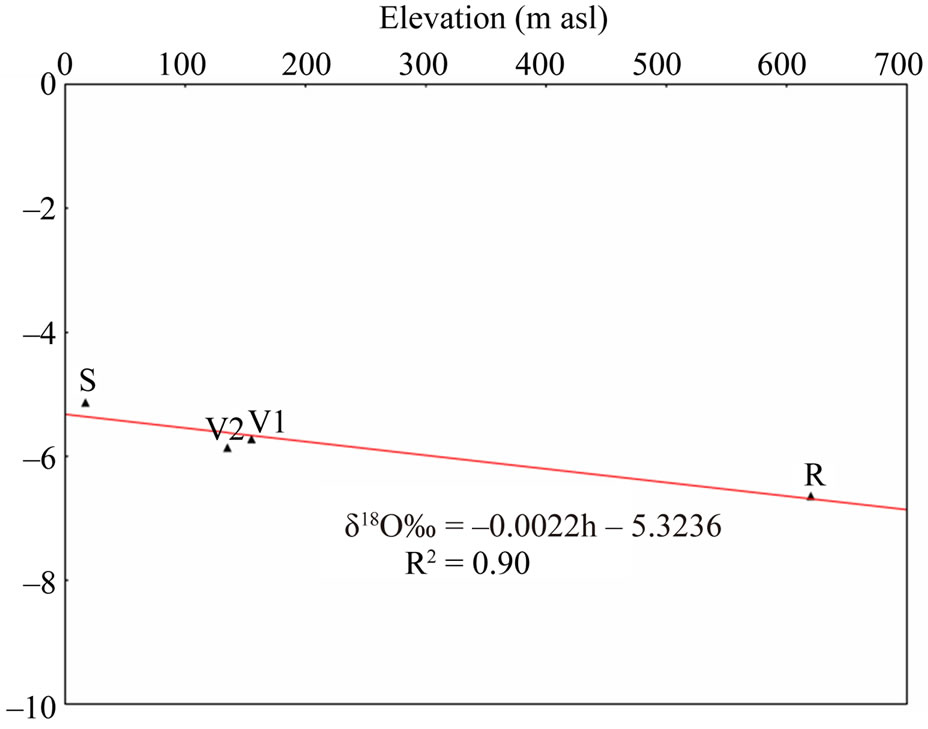
Figure 10. Relationship between δ18O isotope values and elevation (m asl) of precipitation samples from pluviometric stations. (S) Sabaudia (17 m asl) weighted mean δ18O (–5.13) and δ2H (–27.0); (V1) Variano 1 (155 m asl) weighted mean δ18O (–5.72) and δ2H (–32.7); (V2) Variano 2 (135 m asl) weighted mean δ18O (–5.86) and δ2H (–35.7); (R) Roccamonfina (620 m asl) weighted mean δ18O (–6.64) and δ2H (–37.8).
and well water samples comparing the elevation at which the measured isotopic content of the water samples match the isotopic content of precipitation. As a matter of fact, for the calculation of mean recharge elevation of spring and well water samples, we use:
i) an average weight value of δ18O (–5.13) and δ2H (–27.0) [43], which come from the rain gauge station at Sabaudia (17 m asl), for lower elevations in the Lepini, Ausoni, Aurunci Mts. and Pontina Plain;
ii) for higher elevations in Lepini Mts., the isotope value of δ18O (–7.58) and δ2H (–42.8) of LP08 (Santa Serena) sample as end-member ,whose recharge elevation is less than 1200 m asl.
iii) the isotope value of δ18O (–7.03) and δ2H (–38.3) from end member sample AR23 (Mt. Revole), whose recharge elevation topographically does not exceed 1200 m asl, in Aurunci Mts. for the higher elevations. Identified recharge elevations for each spring and well water samples are presented in Table 3.
The distribution of δ18O values of sampled waters show a gradual decrease with increasing recharge elevation (Figure 11).
The main directions of identified groundwater flow paths are shown in Figure 12. For Lepini springs, discharge at higher elevations, the estimated mean recharge elevations vary between 900 and 1100 m asl (LP06, LP08, LP09, LP12), while for LP10 and LP11 samples, discharge at 360 m asl, the calculated mean recharge elevation is about 630 m asl. These springs discharge near the recharge area and characterized by low TDS concentrations (<300 mg/l) indicating low mineralization/short
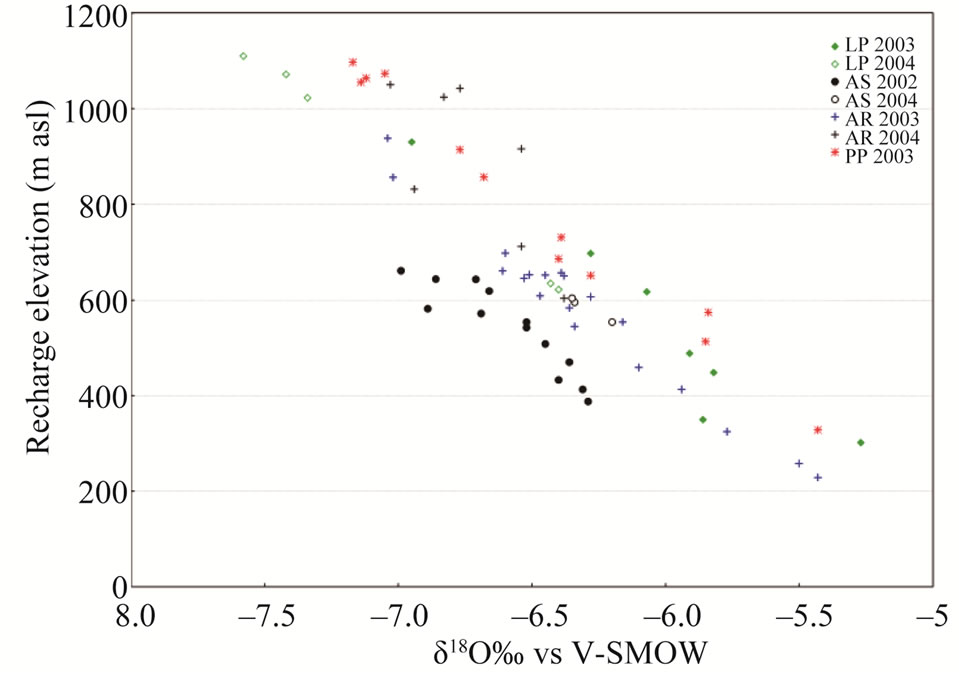
Figure 11. δ18O values of analysed spring and well water samples plotted versus recharge elevations. Data refer to Table 3.
residence time (Mg/Ca ~ 0.3) and short groundwater flow paths (Table 3). LP01 and LP05 spring samples, discharge at lower elevations, show a mean recharge elevation of 618 - 697 m asl, respectively. However, for LP07 spring, discharge at 5 m asl, the estimated mean recharge is about 302 m asl. These samples show average TDS concentration of 900 mg/l. LP07 spring shows a short groundwater flow path considering its recharge elevation, thus the high TDS value is attributed to seawater intrusion. Calculated mean recharge elevations for the springs LP02, LP03, LP04, located in the eastern part of Lepini Mts., range between 350 and 489 m asl. The average TDS concentration of these samples is 350 mg/l. This is attributed to the short residence time of water flowing along the flow paths (i.e. Mg/Ca ratio ~ 0.25). In Ausoni Mts., for the most elevated springs, AS14 and AS16 (500 m asl), the calculated mean recharge elevations are similar to the discharge elevation highlighting short groundwater flow paths. On the contrary, for the samples located at lower discharge elevations (<20 m asl), the mean recharge elevations range between 413 and 644 m asl.
Among these samples AS07, AS08 and AS09, located near the coast, show the highest TDS concentrations (~1300 mg/l) suggesting long groundwater flow path, long residence time (Mg/Ca ~ 0.87) and seawater intrusion (Tables 1 and 3). For the samples AS01, AS02, AS03, AS04, AS15, which are located at the north part of Ausoni Mts., the average discharge elevation is about 95 m asl. The mean recharge elevation for these springs varies between 388 and 662 m asl suggesting long groundwater flow paths, but short residence time (Mg/Ca ~ 0.2) and low TDS concentrations ( ~323 mg/l). Based on the isotopic gradients, three different recharge areas occur in the western Aurunci: two recharge areas are located in the north and the other one is in the south part of the massif. The first area in the north feeds the springs AR23,
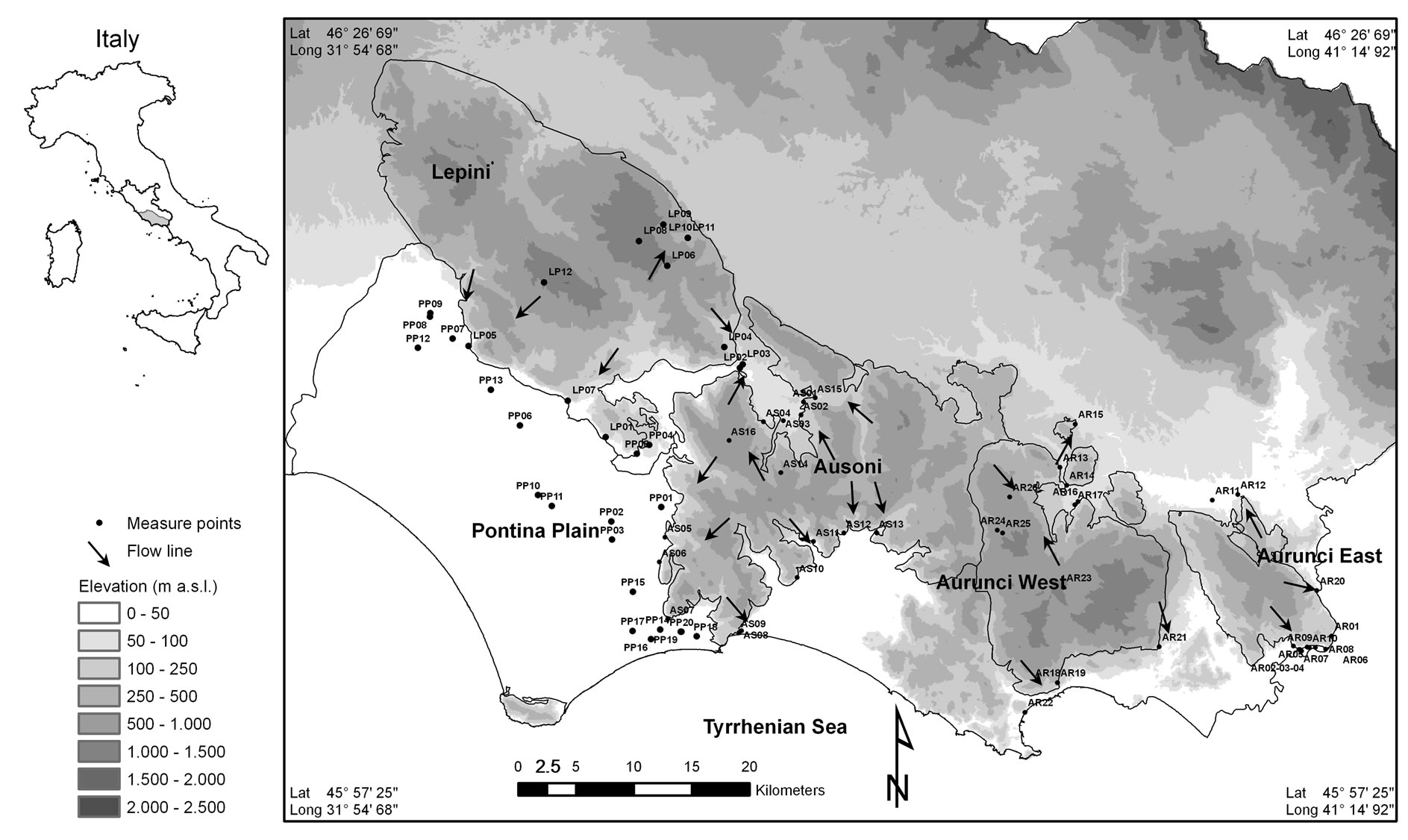
Figure 12. Site study area, location of sampled springs and wells and the main directions of groundwater flow paths (LP: Lepini springs, AS: Ausoni springs, AR: Aurunci springs, PP: Pontina Plain wells).
AR24, AR25 and AR26. For these springs, the mean recharge elevation range between 916 and 1050 m asl and the average TDS content is less than 300 mg/l indicating short groundwater flow paths and short residence time (Mg/Ca ~ 0.04). Considering the mean recharge elevation of these springs and their low mineralization, it can be assumed that they are recharged by a perched aquifer. The second area in the north recharges the springs AR13, AR14, AR15 and AR16. The mean recharge elevations varies from 229 to 545 m asl for these springs. These springs show average TDS concentrations of 347 mg/l. The recharge area occur in the south part recharges the springs AR18, AR19, AR21 and AR22. The discharge elevation of these spring samples occur at lower elevations, while the mean recharge elevation range between 604 and 938 m asl indicating long groundwater flow paths, short residence time (Mg/Ca ~ 0.3) and low TDS (<325 mg/l) concentrations. In the eastern part of Aurunci Mts., the identified recharge area feeds the springs located at lower elevations (AR01 to AR12 and AR20). For these samples the mean recharge elevations vary from 325 to 712 m asl suggesting long groundwater flow paths. The samples AR01, AR08 and AR20 show higher TDS contents (~1100 mg/l) than other sampled springs, which may be related to the enhanced water-rock interaction along the groundwater flow paths (Tables 1 and 3). Calculated mean recharge elevations, identified by the isotopic analysis, for well water samples from Pontina Plain, range between 652 and 1097 m asl, except for the samples PP10, PP11 and PP12 (329 - 575 m asl). The identified mean recharge elevations for these well water samples (PP01 to PP09) are higher than the recharge elevations of Ausoni springs (<650 m asl), which indicates that the aquifer feeding them is the carbonate aquifer of Lepini Mts. The mean recharge elevations correspond to approximately the topographic elevations of the study area. The samples PP02, PP03 and PP06 show high TDS concentrations (1200 mg·L–1) and medium residence times (Mg/Ca ~ 0.56), which indicates enhanced interaction with calcareous and calcareousdolomitic lithologies and/or seawater intrusion. However, samples PP14, PP16, PP18, PP19 and PP20 show the highest TDS concentrations (>2500 mg/l), according to Lepini, Ausoni and Aurunci springs, which is related to seawater intrusion in the coastal area. In Pontina Plain, the mean recharge elevations of some well water samples (PP13 to PP20) were not determined due to the lack of isotope data.
5. Conclusions
A combined geochemical and isotopic investigation techniques were applied to spring and well waters from the carbonate aquifers of Southern Latium region, Italy, which are the most important groundwater reservoirs in this area, in order to define in detail the hydrogeological conceptual model of these aquifer systems. The geochemical characterization of spring and well water samples lead to the definition of the aquifers, based on both hydrogeochemical facies and TDS concentrations. In fact, the results of chemical composition and identified recharge elevations of springs and wells allowed us to advance realistic assumptions about the type of aquifer rocks, formed mostly of carbonates, the length of groundwater flow paths and groundwater residence times. The geochemical characteristics of the spring and well water samples, identify different types of groundwater evolution consisting of modifications of chemical composition.
The most common hydrofacies for the spring samples in the Lepini, Ausoni and Aurunci Mts., Ca-Mg-HCO3- type, however some samples show a composition of Na-Cl-type. On the contrary, most of the well water samples from Pontina Plain show the characteristics of a typical composition of Ca-Cl and Na-Cl type waters. The identified hydrochemical facies suggest that water types undergo further geochemical evolution through water rock interaction and/or seawater intrusion in coastal area to reach a final stage of evolution represented by the Na-Cl water type.
The results of geochemical modeling suggest that most of the spring and well water samples are saturated with respect to calcite and dolomite, however all sampled waters were undersaturated with respect to gypsum and halite. The high dissolution rate for carbonate formations allows for waters close to saturation with respect to calcite and dolomite and evaporate minerals (gypsum and halite) to remain undersaturated, resulting in continued dissolution along flow paths. The hydrochemical processes controlling the chemistry of spring and well waters were identified using different ionic ratios (Mg/Ca, Na/Cl, SO4/Cl and HCO3/Cl, Ca/Na and Br/Cl). Most of the groundwater samples from Pontina Plain and low elevation Lepini and Ausoni Mts. springs have molar ratios of these ionic constituents nearly close to the seawater ratio indicating the contribution of seawater. The results of geochemical modelling and the selected ionic ratios shows that the chemical weathering along with the dissolution of rock-forming minerals and seawater intrusion, in the coastal area, which have contributed in the modification of the groundwater chemistry.
The evaluation of δ18O and δ2H values of spring and well water samples shows that most of the samples fall to the left of the WMWL suggesting input to local rainfall that derives from weather fronts coming from the Mediterranean Sea. This fact is also confirmed by the high deuterium excess values (above 14‰) of spring samples suggesting the precipitation in the groundwater comes from the Mediterranean sector. Groundwater samples from Pontina Plain show low d-excess values (ranges from 7.5‰ to 12.5‰) indicating that they were affected by evaporation. The relationship between δ18O and δ2H, for spring and well water samples, shows shifts of both the slope and the deuterium excess when compared to the world meteoric (WMWL) and central Italy meteoric (CIMWL) water lines. The deviation of data points from the meteoric lines can be attributed to evaporation both during the falling of the rain and by run-off on the ground surface before infiltration. Stable isotope values (δ18O and δ2H) of spring and groundwater samples and their relationship between meteoric water lines show that Lepini and Aurunci springs and groundwater samples from Pontina Plain follow what appear to be linear trends that suggest varying source elevations for different springs, while Ausoni springs does not seem to show this trend; suggesting a similar recharge elevation for waters discharging at all springs in the Ausoni Mts. system. Based on the isotopic characterization and geographic positions of the various springs, four different recharge areas identified in the Aurunci Mountains. In Pontina Plain, the elevations of the recharging areas, identified by the isotopic analysis, suggest that the Lepini carbonate aquifers are feeding them.
The primary contribution of this paper was the delineation of potential recharge areas and elevations in several mountain blocks in Central Italy using environmental isotopes, which are a common natural tracer, to predict recharge elevation and constrain recharge area. Geochemistry was also used to infer source region and hydrochemical processes control groundwater composition. The results demonstrate the importance of integrating knowledge of environmental isotopic and hydrochemical characterization for the better management of these aquifer systems in the region.
6. Acknowledgements
The authors gratefully acknowledge the financial support of the Regional Basins Authority of Latium (Department of Environment of Latium Region).
REFERENCES
- D. Chapman, “Water Quality Assessment; A Guide to the Use of Biota, Sediments and Water in Environmental Monitoring,” Chapman & Hall, University Press, Cambridge, 1992.
- S. S. D. Foster, A. R. Lawrence and B. L. Morris, “Groundwater in Urban Development: Assessing Management Needs and Formulating Policy Strategies,” World Bank Technical Paper, Washington DC, 1997.
- I. D. Clark and P. Fritz, “Environmental Isotopes in Hydrogeology,” CRC Press/Lewis Publishers, Boca Raton, 1997.
- P. G. Cook and A. L. Herczeg, “Environmental Tracers in Subsurface Hydrology,” Kluwer Academic Publishers, Boston, 2000. doi:10.1007/978-1-4615-4557-6
- P. Fritz and J. Ch. Fontes, “Handbook of Environmental Isotope Geochemistry,” The Terrestrial Environment A, Elsevier, Amsterdam, Vol. 1, 1980.
- W. G. Mook, “Environmental Isotopes in the Hydrological Cycle,” Principles and Applications, IHP-V, Technical Documents in Hydrology 1, Vol. IV, No. 39, UNESCO, Paris, 2000.
- J. L. Terwey, “Challenges in African Hydrology and Water Resources,” Proceedings of the Harare Symposium, July 1984, IAHS Publication No. 144.
- H. Azzaz, M. Cherchali, M. Meddi, B. Houha, J. M. Puig and A. Achachi, “The Use of Environmental Isotopic and Hydrochemical Tracers to Characterize the Functioning of Karst Systems in the Tlemcen Moutains, Northwest Algeria,” Hydrogeology Journal, Vol. 16, No. 3, 2008, pp. 531-546. doi:10.1007/s10040-007-0235-4
- G. M. Zuppi and E. Sacchi, “Dynamic Processes in the Venice Region Outlined by Environmental Isotopes,” In: P. Krumbergel, Ed., Isotopes in Environmental and Health Studies, Vol. 40, No. 1, 2004, pp. 35-44.
- W. C. Sidle, “Environmental Isotopes for Resolution of Hydrology Problems,” Environmental Monitoring and Assessment, Vol. 52, No. 3, 1998, pp. 389-410. doi:10.1023/A:1005922029958
- L. Serva and F. Brunamonte, “Subsidence in the Pontina Plain, Italy,” Bulletin of Engineering Geology and the Environment, Vol. 66, No. 2, 2007, pp. 125-134. doi:10.1007/s10064-006-0057-y
- R. Salvati and I. D. Sasowsky, “Development of Cover Collapse Sinkholes in Areas of Groundwater Discharge,” Journal of Hydrology, Vol. 264, No. 1, 2002, pp. 1-11. doi:10.1016/S0022-1694(02)00062-8
- C. Boni, P. Bono, G. Capelli, S. Lombardi and G. M. Zuppi, “Contributo all’Idrogeologia dell’Italia Centrale: Analisi Critica dei Metodi di Ricerca,” Memorie Società, Geologica Italiana, Vol. 35, 1986, pp. 947-956.
- R. Casa, M. Rossi, G. Sappa and A. Trotta, “Assessing Crop Water Demand by Remote Sensing and GIS for the Pontina Plain, Central Italy,” Water Resources Management, Vol. 23, No. 9, 2009, pp. 1685-1712. doi:10.1007/s11269-008-9347-4
- C. Boni, P. Bono and G. Capelli, “Schema Idrogeologico dell’Italia Centrale,” Memorie Società Geologica Italiana, Vol. 36, 1986, pp. 991-1012.
- M. Boccaletti, M. Coli and G. Napoleone, “Nuovi Lineamenti Strutturali da Immagini Landsat e Rapporti con l’Attività Sismica Negli Appennini,” Bollettino della Società Geologica Italiana, Vol. 96, 1977, pp. 679-694.
- C. Boni and P. Bono, “Carta Idrogeologica del Territorio della Regione Lazio—Scala 1:250.000,” Regione Lazio, Assessorato alla Programmazione, Ufficio Parchi e Riserve, Università degli Studi di Roma “La Sapienza”, Roma, 1988.
- C. Boni, “The Relationship between the Geology and Hydrology of the Latium-Abruzzi Apennines,” In: M. Parotto and A. Praturlon, Eds., Geological Summary of the central Apennines, Quaderni de “La ricerca scientifica”, Vol. 90, 1975, pp. 301-311.
- B. Accordi, A. Biasini, C. Caputo, G. Devoto, R. Funiciello, G. B. La Monica, E. Lupia Palmieri, R. Matteucci and U. Pieruccini, “Geologia e Dissesti del Territorio Montano della Regione Lazio,” In: Carta della Montagna, Vol. 2, Monografia Regionali No. 12 Lazio, Ministero di Agricoltura, Roma, 1976, pp. 55-101.
- P. Celico, “Schema Idrogeologico dell’Appennino Carbonatico Centro-Meridionale,” Memorie e Note dell’ Istituto di Geologia Applicata, Vol. 14, 1978, pp. 1-97.
- C. Kendall and T. B. Coplen, “Multisample Conversion of Water to Hydrogen by Zinc for Stable Isotope Determination,” Analytical Chemistry, Vol. 57, 1985, pp. 1437- 1440. doi:10.1021/ac00284a058
- S. Epstein and T. Mayeda, “Variation of 18O Content of Water from Natural Sources,” Geochimica et Cosmochimica Acta, Vol. 4, No. 5, 1953, pp. 213-224. doi:10.1016/0016-7037(53)90051-9
- H. Craig, “Isotopic Variation in Meteoric Water,” Science, Vol. 133, No. 3465, 1961, pp. 1702-1703. doi:10.1126/science.133.3465.1702
- D. L. Parkhurst and C. A. J. Appello, “User’s Guide to PHREEQC (Version 2)—A Computer Program for Speciation, Batch-Reaction, One-Dimensional Transport, and Inverse Geochemical Calculations,” US Geological Survey Water-Resources Investigations, Report 99-4259, 1999.
- X. H. Wen, Y. Q. Wu and J. Wu, “Hydrochemical Characteristics of Groundwater in the Zhangye Basin, Northwestern China,” Environmental Geology, Vol. 55, No. 8, 2008, pp. 1713-1724.
- A. M. Piper, “A Graphic Procedure in the Geochemical Interpretation of Water-Analyses,” Transactions of the American Geophysical Union, Vol. 25, 1944, pp. 914- 923.
- B. F. Jones, A. Vengosh, E. Rosenthal and Y. Yechieli, “Geochemical Investigations,” In: J. Bear, A. H. D. Cheng, S. Sorek, D. Ouazar and I. Herrera, Eds., Seawater Intrusion in Coastal Aquifers—Concepts, Methods and Practises, Kluwer Academic Publishers, Dordrecht, 1999, pp. 51-72.
- G. Ghiglieri, G. Oggiano, D. Fidelibus, G. Barbieri, A. Vernier and A. Tamiru, “Hydrogeology of the Nurra Region, Sardinia (Italy): Basement-Cover Influences on Groundwater Occurrence and Hydrogeochemistry,” Hydrogeology Journal, Vol. 17, No. 2, 2009, pp. 447-466. doi:10.1007/s10040-008-0369
- C. A. J. Appelo and D. Postma, “Geochemistry, Groundwater and Pollution,” 2nd Edition, Balkema, Rotterdam, 2005, pp. 241-309. doi:10.1201/9781439833544.ch6
- W. Stumm and J. J. Morgan, “Chemical Equilibria and Rates in Natural Waters,” John Wiley and Sons, New York, 1996.
- D. Langmuir, “Geochemistry of Some Carbonate Ground Waters in Central Pennsylvania,” Geochimica et Cosmochimica Acta, Vol. 35, No. 10, 1971, pp. 1023-1045. doi:10.1016/0016-7037(71)90019-6
- I. J. Fairchild, A. Borsato, A. F. Tooth, S. Frisia, C. J. Hawkesworth, Y. M. Huang, F. McDermott and B. Spiro, “Controls on Trace Element (Sr-Mg) Compositions of Carbonate Cave Waters: Implications for Speleothem Climatic Records,” Chemical Geology, Vol. 166, 2000, pp. 255-269. doi:10.1016/S0009-2541(99)00216-8
- W. B. White, “Geomorphology and Hydrology of Karst Terrains,” Oxford University Press, New York, 1988.
- C. D. Palmer and J. A. Cherry, “Geochemical Evolution of Groundwater in Sequences of Sedimentary Rocks,” Journal of Hydrology, Vol. 75, No. 2, 1984, pp. 27-65. doi:10.1016/0022-1694(84)90045-3
- J. S. Herman and W. B. White, “Dissolution Kinetics of Dolomite: Effects of Lithology and Fluid Flow Velocity,” Geochimica et Cosmochimica Acta, Vol. 49, 1985, pp. 2017-2026. doi:10.1016/0016-7037(85)90060-2
- W. McLean, J. Jankowski and N. Lavitt, “Groundwater Quality and Sustainability in an Alluvial Aquifer, Australia,” In: O. Sililom, et al., Eds., Groundwater past Achievements and Future Challenges, A Balkema, Rotterdam, 2000, pp. 567-573.
- M. Meybeck, “Global Chemical Weathering of Surficial Rocks Estimated from River Dissolved Loads,” American Journal of Science, Vol. 287, 1987, pp. 401-428. doi:10.2475/ajs.287.5.401
- Y. Fakir, M. El Mernissi, T. Kreuser and B. Berjami, “Natural Tracer Approach to Characterize Groundwater in the Coastal Sahel of Oualidia (Morocco),” Environmental Geology, Vol. 43, 2002, pp. 197-202. doi:10.1007/s00254-002-0644-6
- D. C. Andreasen and W. B. Fleck, “Use of Bromide: Chloride Ratios to Differentiate Potential Sources of Chloride in a Shallow, Unconfined Aquifer Affected by Brackish-Water Intrusion,” Hydrogeoly Journal, Vol. 5, No. 2, 1997, pp. 17-26. doi:10.1007/s100400050104
- E. Randolph McFarland and T. Scott Bruce, “Distribution, Origin, and Resource-Management Implications of Ground-Water Salinity along the Western Margin of the Chesapeake Bay Impact Structure in Eastern Virginia,” Studies of the Chesapeake Bay Impact Structure—The USGS-NASA Langley Corehole, Hampton, Virginia, and Related Coreholes and Geophysical Surveys, US Geological Survey Professional Paper 1688, Virginia, 2005.
- J. R. Gat and I. Carmi, “Evolution of the Isotopic Composition of Atmospheric Waters in the Mediterranean Sea Area,” Journal of Geophysical Research, Vol. 75, 1970, pp. 3039-3048. doi:10.1029/JC075i015p03039
- K. Rozanski, L. Araguas Araguas and R. Gonfiantini, “Isotopic Patterns in Modern Global Precipitation,” In: P. K. Swart, et al., Eds., Climate Change in Continental Isotopic Records, Geophysical Monograph Series, AGU, Washington DC, Vol. 78, 1993, pp. 1-36.
- A. Longinelli and E. Selmo, “Isotopic Composition of Precipitation in Italy: A First Overall Map,” Journal of Hydrology, Vol. 270, No. 1-2, 2003, pp. 75-88.
- H. Craig, L. I. Gordon and Y. Horibe, “Isotopic Exchange Effects in the Evaporation of Water,” Journal of Geophysical Research, Vol. 68, No. 17, 1963, pp. 5079-5087.
- J. Fontes and G. Zuppi, “Isotopes and Water Chemistry in Sulphide-Bearing Springs of Central Italy,” Proceedings of Advisory Group Meeting on Interpretation in Environmental Isotope and Hydrochemical Data in Groundwater Hydrology, IAEA, Vienna, 1976, pp. 143-158.
- P. Vreča, T. Kanduč, S. Žigon and Z. Trkov, “Isotopic Composition of Precipitation in Slovenia,” In: Isotopic Composition of Precipitation in the Mediterranean Basin in Relation to Air Circulation Patterns and Climate, IAEA, TECDOC-1453, Vienna, 2005, pp. 157-172.
- W. Dansgaard, “Stable Isotopes in Precipitation,” Tellus, Vol. 16, No. 4, 1964, pp. 436-468.
- A. Nir, “Development of Isotope Methods Applied to Groundwater Hydrology,” Proceeding of a Symposium on Isotope Techniques in the Hydrologic Cycle, American Geophysical Union, Monograph Series, Vol. 11, 1967, pp. 109-116.
NOTES
*Corresponding author.

