Journal of Modern Physics
Vol.06 No.01(2015), Article ID:53218,10 pages
10.4236/jmp.2015.61001
A Real Version of the Dirac Equation and Its Coupling to the Electromagnetic Field
Eckart Marsch
Institute for Experimental and Applied Physics, Christian Albrechts University at Kiel, Kiel, Germany
Email: marsch@physik.uni-kiel.de
Copyright © 2015 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 10 November 2014; accepted 23 November 2014; published 14 January 2015
ABSTRACT
A real version of the Dirac equation is derived and its coupling to the electromagnetic field considered. First the four-component real Majorana equation is briefly discussed. Then the complex Dirac equation including an electromagnetic field will be written as an eight-component real spinor equation by separating it into its real and imaginary parts. Through this decomposition, what becomes obvious is the way in which the electromagnetic field couples to charged fermions (electron and positron) when being described by real spinor fields. Thus, contrary to common expectation, charged fermions can also be described by a real Dirac equation if they are considered as a doublet related to the SO(2) symmetry group, which enables a matrix coupling to the electromagnetic field and corresponds to the usual U(1) gauge symmetry of the standard Dirac equation.
Keywords:
Dirac Equation, Coupling to Electromagnetic Field, Real Field Equation

1. Introduction
In modern elementary particle physics the complex Dirac equation [1] plays a fundamental role and is used in the standard model (SM) to describe the charged fermions (see, e.g., the textbooks by Lee [2] and Kaku [3] ), which are represented in terms of four-component complex spinor fields or two-component complex Weyl spinor fields [4] in the chiral representation for the massless case. The aim of the present work is to show that charged fermions can also be described by a purely real Dirac equation that does not involve any complex numbers at all but only real matrices and spinors. This approach is quite different from the abstract mathematical way of Hestenes [5] , who reformulated the Dirac theory completely in terms of a real Clifford algebra characterizing the geometrical properties of space-time. Our approach also differs substantially from the treatment by Ljolje [6] , who argued that the analogy with the classical electromagnetic field suggests a full description also of the Dirac field in the real domain. In this paper, the gauge symmetry considered thereafter for the coupling of fermions to an electromagnetic field is based on the SO(2) group. It is, while being its adjoint representation, closely related to the U(1) group which is commonly used in electrodynamic gauge theory of the standard com- plex Dirac equation.
It is well known that fermions described by the real four-component Majorana [7] - [9] equation can not be coupled to the electromagnetic field. Therefore that equation can only describe neutral fermions and has found no application in the SM. We will show, however, that for the real version of the Dirac equation the coupling of charged fermions to an electromagnetic field is possible, and can be retained if in that equation the real and imaginary part of a complex Dirac spinor field are assembled in a doublet associated with the SO(2) symmetry group. This arrangement enables a matrix coupling to the electromagnetic field. We will subsequently derive the eigenfunctions of this real version of the Dirac in some detail, and also discuss some problems arising with the evaluation of the related Lorentz-invariant bilinear form that appear in the mass term.
The outline of the paper is as follows: First, the four-component real Majorana equation is briefly discussed as the classical paradigm for a real spinor field equation. Second, the covariant derivative in electromagnetic gauge theory is reconsidered, and a way opened to introduce electromagnetism into a real spinor field equation. Third, the complex Dirac equation including an electromagnetic field will be transformed into a real Dirac equation for an eight-component real spinor field. Through this procedure it becomes obvious how the electromagnetic field couples to charged fermions (e.g., electrons and positrons) when being described by real spinor fields. The symmetries of the resulting real Dirac equation are also discussed. Fourth, the eigenfunctions of the real Dirac equation are derived. Fifth, the properties of bilinear forms are shortly addressed, and then we consider similarity transformations. Finally, the conclusions are presented.
2. The Majorana Equation
At the start we rederive the real four-component spinor Majorana equation, without recourse to the Dirac equation (thereby following the recent work of Aste and Marsch [10] - [12] ). The Majorana equation with a mass term provides a useful description of massive neutrinos. Its solutions include both kinetic helicities, as it is required for a massive relativistic particle.
In the subsequent algebra we make use of conventional symbols, notations and definitions, and use units of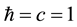 . The covariant four-momentum operator, defined as
. The covariant four-momentum operator, defined as , acts for example on the scalar field
, acts for example on the scalar field 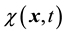 or the real four-component spinor field
or the real four-component spinor field . Insertion of the differential operator
. Insertion of the differential operator 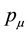 into the relativistic energy and momentum relation, written in manifestly covariant form as mass-shell con- dition
into the relativistic energy and momentum relation, written in manifestly covariant form as mass-shell con- dition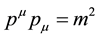 , readily yields the real Klein-Gordon equation [13] [14]
, readily yields the real Klein-Gordon equation [13] [14]
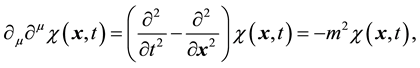 (1)
(1)
for the scalar field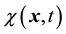 . This equation is the starting point of any quantum field theory and involves only the second-order differential d’Alembert operator which obviously is invariant under a Lorentz transformation. Equation (1) can formally be linearized to read
. This equation is the starting point of any quantum field theory and involves only the second-order differential d’Alembert operator which obviously is invariant under a Lorentz transformation. Equation (1) can formally be linearized to read
 (2)
(2)
with appropriately defined real spinor field , and by introduction of the non-commuting matrix operators
, and by introduction of the non-commuting matrix operators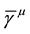 . Here we indicated the real gamma matrices as appearing in (2) by a top bar, in order to avoid confusion with the standard nomenclature of the Dirac [1] equation. By squaring now equation (2) one finds that, for reproducing (1), the gammas have to obey the algebra
. Here we indicated the real gamma matrices as appearing in (2) by a top bar, in order to avoid confusion with the standard nomenclature of the Dirac [1] equation. By squaring now equation (2) one finds that, for reproducing (1), the gammas have to obey the algebra
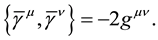 (3)
(3)
Here  is the Minkowskian metric tensor, and the brackets denote the anticommutator. We shall derive in the course of this paper various forms of the gamma matrix operators.
is the Minkowskian metric tensor, and the brackets denote the anticommutator. We shall derive in the course of this paper various forms of the gamma matrix operators.
Apparently, the condition (3) can be fulfilled by the real  gamma matrices derived from the repre- sentation first found by Majorana [7] , which then makes (2) a real spinor field equation. It is easily transformed into the standard Dirac equation by use of the purely imaginary gamma matrices
gamma matrices derived from the repre- sentation first found by Majorana [7] , which then makes (2) a real spinor field equation. It is easily transformed into the standard Dirac equation by use of the purely imaginary gamma matrices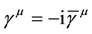 , in which case the spinor field
, in which case the spinor field  would also have to be complex and then is conventionally denoted by
would also have to be complex and then is conventionally denoted by


Obviously, all three sigma matrices mutually anti-commute with each other and, when being squared, are equal to the 



In terms of the above lambda matrices the Majorana gamma matrices (see, e.g. Kaku [3] ) can be written as:

whereby 




transposed matrix. The standard representation of the Dirac matrices [3] is obtained from (5) by means of a similarity transformation. For subsequent use we also introduce another name for the real gamma matrix, writing it as


We emphasize that the Majorana equation, while being real, has two degrees of freedom (

3. Covariant Derivative in Electromagnetic Gauge Theory
As is well known from the classical work of Klein, Pauli and Weisskopf [3] [14] [15] , the real scalar Klein- Gordon field can also be used for charged particles, namely by considering two independent scalar fields which both obey the Klein-Gordon equation and are associated with the positively and negatively charged bosons having the same mass. They can be arranged in a complex composite scalar field, such that




This equation obeys U(1) symmetry, meaning that the spinor field 








However, apparently this procedure does not work for the real Majorana Equation (2) or (6), without destroy- ing their real nature. So, the real four-component Majorana equation can not be coupled to an electromagnetic field (and also not to other gauge fields when being associated with complex phase factors) by means of the minimal coupling in the above form.
Yet, there is another way to introduce electromagnetism in a real field equation, if we just assemble the real and imaginary parts of a complex spinor 





which is a generator of the SO(2) group. Here use has been made of



As shown below, when the Dirac equation is brought into its real form the SO(2) symmetry is obeyed instead of U(1), and thus a Dirac fermion can be charged if it is described in terms of a real eight-component spinor field.
The transformation of the spinor field for a spatio-temporal varying phase 


if the gauge field is transformed as
4. The Real Dirac Equation
Following the reasoning in the previous sections, we now want to derive a real version of the Dirac equation. The complex standard Dirac equation for 

The Dirac matrices in their standard form can be written concisely as

Using the above lambda matrices, we obtain

Note that the three components of the vector 


We can now decompose the complex Dirac Equation (12) into its real and imaginary parts. Thereby we assemble again the real and imaginary parts of a complex four-component Dirac spinor, 




The gamma matrices have a 



These four matrices commute with

Let us discuss the general symmetries of the real Dirac equation. Concerning its space-time symmetries, we define conventionally the time and space coordinate inversion operations as 













whereby we find for the product of Dirac matrices the result

The charge conjugation operation 




which is found to obey



















Finally, let us briefly discuss chiral symmetry. The real chiral matrix is defined appropriately as 

which yields




jection operators by
left-chiral components, i.e.

and are coupled by the mass term which obviously breaks chiral symmetry.
5. Eigenfunctions of the Real Dirac Equation
We return to the real Dirac Equation (16) with the aim to derive its eigenfunctions for




It resembles very much the Majorana Equation (6), with the difference being in the dimension of the gamma matrix representation and the associated spinors. Since Equation (24) is real, and as each component of 

with free real amplitude 




By insertion of the first into the second equation, or vice versa, the relativistic dispersion relation is obtained from:

which yields the two eigenvalues:

The negative root in Equation (29) is related to antiparticles, the positive one to particles. By solving (26) for 


The second solution is obtained from first one if the free phase is chosen as 










where the polarization vector is defined by






Returning now to the standard Dirac Equation (7), we can chose for it the standard basis

corresponding to particles 





How many physical degrees of freedom does the real Dirac equation have? Of course it should not be more than the complex one, which has four, as the complex spinor fields 




Let us now determine the basis spinors of the real Dirac equation. Obviously, in the above general solutions (32) and (33) the polarization spinors can be chosen freely, and the question then arises how to chose them adequately for the real Dirac equation. For that purpose we make use of the standard basis vectors (34), and with their help construct the new extended fundamental basis as follows

corresponding to particle and antiparticle states arranged in doublets of four-component spinors. Note that these basis spinors are eight-dimensional, corresponding to the dimension of the phase space of the complex standard Dirac equation, and form an orthonormal basis of the vector space of the real Dirac equation. Its solution is the doublet spinor field









Let us also discuss briefly how the charge conjugation and the chiral operator act on the basis spinors. We recall the definition of the charge conjugation, which is given by


showing the tight connection between particle and antiparticle spinors. As the charge conjugation operator 



which is in consistent with the fact that 
6. Bilinear Forms Associated with the Real Dirac Equation
Like for the complex Dirac equation, bilinear forms involving the spinor field and its adjoint play an important role in a relativistic field theory. Therefore, we must consider such Lorentz-invariant bilinear forms (see, e.g., [3] ) like


where 


means 
is intimately connected with the negative sign in front of the metric (3), which requires
have








Using these mathematical properties one finds after some algebra by means of (32) and (33) that for any pair of solution field spinors we have
Let us consider a general polarization spinor of the real Dirac equation



which vanishes identically, in particular for any pair of the basis spinors in Equation (34), since beta is sym- metric:





since
The transition to quantization is readily achieved, if we rewrite (omitting the index P here) the real spinor field (32) by replacing the trigonometric by exponential functions. We then obtain

with a new abbreviation that is the operator
properties

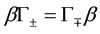
is


To render (42) becoming a quantum field, we just have to promote the amplitudes to fermion annihilation and creation operators that obey the canonical anti-commutation rule

With these comments and conclusions we shall close the discussion of the bilinear forms which determine the mass term in the Lagrangian density.
7. Similarity Transformations
Finally, we will return to the Majorana equation and present its eight-component version which can be obtained by a similarity transformation from the real Dirac Equation (16). The similarity transformation matrix associated with the standard Dirac equation can be written as

By definition it is unitary and has the property

In a similar way we can now subject the real Dirac Equation (16) to a corresponding similarity transformation defined as

where use is made of the y-component of the gamma matrix four vector as given in Equation (17). Note that again the matrix 



Remember that 



which includes the coupling to the electromagnetic field via the matrix

and the Majorana spinor field is connected to the Dirac spinor field by the transformation


Without an electromagnetic field for 


8. Conclusions
In this paper we have shown that the standard complex Dirac equation can be transformed into a real Dirac equation, which still permits the electromagnetic field to be introduced by minimal coupling, and which enables the real nature of that equation to be preserved in an eight-component spinor representation. This coupling to the electromagnetic field is established via the SO(2) symmetry group, which is equivalent to the U(1) symmetry of the complex Dirac equation. Thus the real Dirac equation also describes massive charged fermions coupled to an electromagnetic field. Coupling to other non-abelian gauge symmetry groups may also be possible, while pre- serving the real nature of that equation, if for the involved group use is made of its adjoint representation, which is purely imaginary and thus yields a real covariant derivative.
The real Dirac equation has a beta matrix that is antisymmetric, a property which causes problems for the fundamental Lorentz-invariant bilinear forms, such as 



The real Dirac equation as here derived has essentially the same physical content as its complex ancestor, but looks somewhat cumbersome due to the larger gamma matrices involved. This is the mathematical price to be paid if complex numbers are fully avoided. The related Majorana version appears to be more transparent and seems easier to handle. The electromagnetic gauge field coupling in this equation is of matrix nature, as the imaginary unit is effectively replaced by the matrix
Acknowledgements
The author gratefully acknowledges the financial and institutional support provided for his work by the Extrater- restrial Physics Division headed by Robert Wimmer-Schweingruber at the Institute for Experimental and Ap- plied Physics of Kiel University in Germany.
References
- Dirac, P.A.M. (1928) Proceedings of the Royal Society of London. Series A, Containing Papers of a Mathematical and Physical Character, A117, 610-624.
- Lee, T.D. (1981) Particle Physics and Introduction to Field Theory. Harwood Academic Publishers, New York.
- Kaku, M. (1993) Quantum Field Theory, A Modern Introduction. Oxford University Press, New York.
- Weyl, H. (1929) Zeitschrift für Physik, 56, 330-352. http://dx.doi.org/10.1007/BF01339504
- Hestenes, D. (1967) Journal of Mathematical Physics, 8, 798-808. http://dx.doi.org/10.1063/1.1705279
- Ljolje, K. (2004) The Dirac Field in Real Domain. 1-15, arXiv quant-ph/0401068.
- Majorana, E. (1937) Il Nuovo Cimento, 14, 171-184. http://dx.doi.org/10.1007/BF02961314
- Case, K.M. (1957) Physical Review, 107, 307-316. http://dx.doi.org/10.1103/PhysRev.107.307
- Mannheim, P.D. (1984) International Journal of Theoretical Physics, 23, 643-674. http://dx.doi.org/10.1007/BF02214135
- Aste, A.A. (2010) Symmetry, 2, 1776-1809. http://dx.doi.org/10.3390/sym2041776
- Marsch, E. (2012) International Scholarly Research Notices: Mathematical Physics, 2012, 1-17. http://dx.doi.org/10.5402/2012/760239
- Marsch, E. (2012) Symmetry, 5, 271-286.
- Pauli, W. (1927) Zeitschrift für Physik, 43, 601-623. http://dx.doi.org/10.1007/BF01397326
- Klein, O. (1927) Zeitschrift für Physik, 41, 407-422. http://dx.doi.org/10.1007/BF01400205
- Pauli, W. and Weisskopf, V. (1934) Helvetica Physica Acta, 7, 709-731.
- Yang, C.N. and Mills, F. (1954) Physical Review, 96, 191-195. http://dx.doi.org/10.1103/PhysRev.96.191
- Pal, P.B. (2011) American Journal of Physics, 79, 485-498. http://dx.doi.org/10.1119/1.3549729

