Modern Economy
Vol.06 No.12(2015), Article ID:61737,8 pages
10.4236/me.2015.612112
Market Share Restrictions and Efficiency
Caglar Yurtseven
Department of Economics, Bahcesehir University, Istanbul, Turkey

Copyright © 2015 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Accepted 3 December 2015; published 7 December 2015
ABSTRACT
This paper studies the effects of market share restrictions on research and development activities of firms. Market share of firms is closely followed by regulatory authorities and restrictions are applied in many cases around the world. This essay investigates if these restrictions affect the cost reduction based R&D efforts of the firms in a market. The question asked is important in the sense that countries with different regulations on market share, end up having different innovative structures, which in turn affect their economic growth and well-being. The paper uses a Cournot model, which shows that under the no exit assumption, market share restrictions lower the level of competition and possible rewards from R&D efforts, therefore causing smaller levels of R&D efforts both for big and small firms in the market.
Keywords:
Market Share, Cost Reduction, R&D, Cournot

1. Introduction
In the industrial organization literature, market share restrictions have been a controversial question. It is important because it has been an important evidence of market power, if not the most. In some cases it is seen that market shares as low as 30% are even enough for antitrust authorities to act to regulate the market [1] .
Market share restrictions are basically designed to protect smaller firms in the industry. By this protection, regulators want to keep the competition, by preventing the monopolization of the market. However, as in every policy, trade-offs are important to consider. In this paper, we’ll try to examine a particular one. The effects of market share restrictions on the research and development efforts of big and small firms of a market. This question is important in the sense that countries using different regulations on market share, end up having different innovative structures which in turn affect their economic growth and well-being.
In the literature we see many studies on the total quantity implications of market share restrictions. For example, in a study by Donald W. Kloth and Leo V. Blakley [2] , it is found that total quantity in milk industry can be increased by market share restrictions. On the other hand, in some other industries market share restrictions can restrict the market output. A recent case is shown by Foros, Kind, and Shaffer [3] in which they consider a model of resale price maintenance.
In addition, there is a big literature on the determinants of research and development and its results. As shown by Spence [4] in many cases R&D has the same ultimate effect as direct cost reduction. In this paper, we use this approach. In another paper by Creane and Konishi [5] effects of cost reductions via technology transfers are studied. They study the effects of joint production between rival firms using a basic Cournot model. A similar Cournot model is also used in this paper to investigate the question of this paper. In this paper, however, we only investigate the effects of market share restrictions on the optimal levels of research and development investments of big and small firms of a market.
In some industries like the washing machine and dishwasher industries, especially in Europe, certain producers like Siemens, Bosch and Miele introduce many of the innovations to the market. Most of these innovations are patented for limited times. So these high share firms are nearly sure that their innovations are not going to be matched by the smaller competitors at least in very short term. New technologies as dishwashers with turbidity sensors (2010), steam generator for a household appliance (2010), system comprising a vacuum cleaner and dust bag (2012) are all patented by the producer Miele and not copied by any competitor in Europe for at least one year time period [6] .
We start by covering the possibility, in which big firms are nearly sure that the competitors will not match their efforts. However, they may be under the pressure of market share regulations. We try to see how these regulations will affect the optimal levels of R&D investments of the big firms of the market.
We proceed with a more common case in which each firm has equal access to R&D efforts and can reduce their production costs at the same time period. This possibility is true for many industries. Some examples are food, beverage and furniture industries. For example, in Turkey, after 2010 traditional fast food “doner” started to be marketed as a higher-end food with carefully designed impressive outlets and with menus which incorporated marketing strategies from western fast food chains, this innovation by an entrepreneur succeeded and it is followed easily by the competitors in a very short time. Kasap Doner, Bay Doner, Doner Stop, and Bereket Doner are only a few examples to the firms using these innovations in doner marketing [7] -[9] .
The case we consider will be a market with a small number of firms, similar cost structures, and small possible gains from R&D in terms of cost reduction. We’ll see how the market share restrictions affect both the cost efficient/high market share firms and higher cost/lower share firms of the market. We find that generally market share restrictions lower the level of competition and possible rewards from R&D efforts, therefore causing smaller levels of R&D efforts both for big and small firms of the market.
2. Theoretical Foundation and Conceptual Framework
Since we are assuming a highly homogenous good, basic Cournot model will be our reference point. The demand curve is described by a twice continuously differentiable monotonically decreasing function for :
:
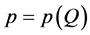
where p(0) = 1, p(Q) ≥ 0 if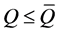 , and p(Q) = 0 otherwise. Thus, p′(Q) < 0 for all
, and p(Q) = 0 otherwise. Thus, p′(Q) < 0 for all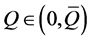 .
.
For the market we assume N ≥ 2 firms where N shows the number of firms in the market. Firms are indexed as . Here, the firm which is indexed with one is the most cost efficient firm and so the one which is most likely to face market share restrictions. We will represent the set of
. Here, the firm which is indexed with one is the most cost efficient firm and so the one which is most likely to face market share restrictions. We will represent the set of 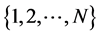 with the letter N. We assume each firm has a marginal cost of
with the letter N. We assume each firm has a marginal cost of 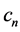 and they all face the same operational fixed cost F. Each firm’s production level is denoted by
and they all face the same operational fixed cost F. Each firm’s production level is denoted by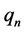 .
.
We assume each firm can reduce their costs by Δ by their research and development efforts. However it is known that cost reduction efforts are not always successful. Considering this fact, we assume that as the firms increase their R&D efforts they are more likely to decrease their cost levels by Δ. Hence we assume each firm is able to choose their success probability level for cost reduction “r”. Of course higher levels of success probability is more costly, so we assume these effort are costly and the cost of R&D effort which brings success proba-
bility of r is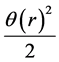 .
.
In this Cournot model each firm is producing the quantity level 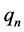 based on their marginal costs.
based on their marginal costs.
Lemma 1: For marginal cost profile 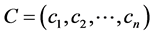 there exists a unique equilibrium, which is characte-
there exists a unique equilibrium, which is characte-
rized by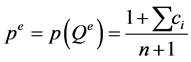 ,
, 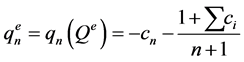 ,
, 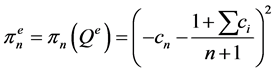
and
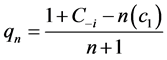
where 
Assuming no share restriction is applied, if a firm is able to decrease its costs by Δ then it will produce the quantity level
3. Analysis of the Effects of Market Share Restrictions on the R&D Efforts of Different Market Structures
3.1. R&D Done Only by the Dominant Firm
In industries where the new technologies are protected at least in the short term by patent laws, the dominant firms are nearly sure that their innovations are not going to be matched by competitors in the short run. As an example to this we cited the durable goods industry in Europe in the introduction.
When the number of firms is not big, cost structures are not very different and possible gains from R&D in terms of cost reduction are not very high, exit threat is not very big for the existing firms. In the following case a market under such conditions will be examined.
We assume a market with 2 firms. Firm 1 knows that Firm 2 is not going to be successful in its cost reduction efforts in the short run. Hence it will try to maximize its profit level by choosing the optimal probability of success “r₁”.
For simplicity from now on 

When Firm 1 is not under any market share restriction threat, the optimal success probability level for cost reduction that is chosen by the leader firm can be written as:
If market share restriction is effective then Firm 1 cannot increase its production levels, even it is successful in its cost reduction efforts. So it will be still producing the same quantity A. However, due to decreasing marginal costs it will get an extra profit equal to the quantity it produces times the reduction in its marginal cost, AΔ1.
In this case the profit function to be maximized will be:
and the optimal level of success probability can be written as:
Since 2n < n + 1 and the second term is positive when share restrictions are in effect, we can conclude that under this patent laws and leader-follower assumptions, market share restrictions cause big firm to lower its R&D efforts.
Lemma 2: When exit is not a big threat for the firms in the market, and assuming short term patent laws with the existence of leader-follower firms; market share restrictions lower the rewards from innovation and so lower the incentives for R&D efforts for the cost efficient firm of the market.
3.2. R&D Done by the Dominant and Small Firms
After this restricted case we’ll examine a more common case in which both the dominant and small firms can do cost reduction by their R&D efforts. We will assume again that exit is not a big threat for the firms of the market as we did in the above part.
When we consider a market with 2 firms, Firm 1 will know that Firm 2 is going to be successful in its cost reduction efforts with the probability r2. Hence, it will try to maximize its profit level by choosing the optimal probability of success “r1”.

The profit function to be maximized will be symmetric for the Firm 2 (
The first order conditions for r1 and r2 can be written as:
and the equilibrium levels of r1 and r2 can be written as:
Now let’s consider the case where the market share restrictions are applied and they are effective for Firm 1. Using the simplified notations we assumed before; Firm 1 is trying to maximize the following profit function.

In this function, note that, Firm 1 cannot freely increase its production levels even it is successful in its cost reduction efforts. If Firm 1 is successful and Firm 2 is not successful in their R&D efforts, then the Firm 1 won’t be able to increase its production level at all due to market share restrictions. The only gain will be the increase in its profits due to smaller marginal cost. So it will resume the same quantity A, but will get an extra profit equal to the quantity it produces times the reduction in its marginal cost, AΔ4.
And Firm 2 will be maximizing the function;
Here, note the third term which shows the fact that even the small firm is not successful and bigger firm is successful in its cost reduction efforts; small firm is still able to keep its pre-cost reduction profit level. This is due the fact that big firm is not able to increase its quantity as a result of the market share restrictions.
The first order conditions for r₁ and r₂ when there are effective market share restrictions can be written as:
and the equilibrium levels of r1 and r2 can be written as:


Lemma 3: When exit is not a big threat for the firms in the market, market share restrictions lower the level of competition and cause small firm(s) to choose smaller levels of R&D investment to reduce their marginal costs.
Lemma 4: When exit is not a big threat for the firms in the market, market share restrictions make it more difficult to get the rewards of its cost decreasing efforts and cause cost efficient firm to choose smaller levels of R&D investment to reduce its marginal costs.
For the proof, see the Appendix.
4. Conclusion
In this paper we show that, under certain assumptions as no exit, market share restrictions lower the level of competition and possible rewards from R&D efforts, therefore causing smaller levels of R&D efforts both for big and small firms of the market. However, there are many cases in the real world which need an opposite explanation. Obviously there are many industries where exit is a real threat. This possibility may be a feasible topic for future research.
Cite this paper
CaglarYurtseven, (2015) Market Share Restrictions and Efficiency. Modern Economy,06,1181-1188. doi: 10.4236/me.2015.612112
References
- 1. Foros, O., Kind, H. and Shaffer, G. (2007) Resale Price Maintenance and Restrictions on Dominant Firm and Industry-Wide Adoption. CESifo Working Paper Series No. 2032
- 2. Spence, M. (1984) Cost Reduction, Competition, and Industry Performance. Econometrica, 52, 101-122. http://dx.doi.org/10.2307/1911463
- 3. Creane, A. and Konishi H. (2009) The Unilateral Incentives for Technology Transfers: Predation (and Deterrence) by Proxy. International Journal of Industrial Organization, 27, 379-389.
http://dx.doi.org/10.1016/j.ijindorg.2008.10.007 - 4. Ekelund, R., Ford, G. and Koutsky, T. (2000) Market Power in Radio Markets: An Empirical Analysis of Local and National Concentration. Journal of Law and Economics, 43, 157-184.
http://dx.doi.org/10.1086/467451 - 5. Levin, R. and Reiss, P. (1988) Cost-Reducing and Demand-Creating R&D with Spillovers. Rand Journal of Economics, 19, 538-556. http://dx.doi.org/10.2307/2555456
- 6. Patentdocs (2015) Patent and Technology Website. http://www.faqs.org/patents/assignee/miele-cie-kg/
- 7. Beef and Fish (2015) Sectorial Website. http://beefandfish.com/genel/turkiyede-doner-pazari.html
- 8. Landes, W. and Posner, R. (1981) Market Power in Antitrust Cases. Harvard Law Review, 94, 937-996. http://dx.doi.org/10.2307/1340687
- 9. Shapiro, C. (2010) A Tribute To Oliver Williamson: Antitrust Economics. California Management Review Winter, 52, Reprint Series.
Appendix
The steps that take us from the profit functions for the dominant and small firms to equilibrium levels of R&D probabilities when there is not a big exit consideration are shown in this part of the appendix.
When we get the first derivative of the profit function to be maximized by the dominant firm when there is no market share restriction

When we solve for the equilibrium value of r1
so we get

When we get the first derivative of the profit function to be maximized by the dominant firm when there is effective market share restriction
and for the smaller firm it will be
If we try to solve for the equilibrium value of r1

and when we solve for the equilibrium value of r

We’ll compare the r1’s and r2’s of without restriction case and with restriction cases. In the denominators one can realize a negative expression is subtracted from θ in the restriction cases which makes the denominators bigger and so causes the equilibrium levels of investment to be lower in the restriction cases. For the numerators, the numerator of small firm in the restriction case is clearly smaller, but the comparison is not that easy for the big firm. However, it is still clear that as long as number of firms is not unrealistically big and the cost reduction is not extremely small the numerator is smaller in the restriction case. Observing all these evidence we proved the Lemma 3 and Lemma 4 sated in the text.
NOTES
1Since 
2Here, n can be replaced with 2. We keep n in this form just to give an idea about the effect of the number of firms.
3Here, n can be replaced with 2. We keep n in this form just to give an idea about the effect of the number of firms.
4Since 
5
6
7We call the part in parenthesis as “D”.
8We call the part in parenthesis as “E”.



























