Received 11 July 2015; accepted 12 January 2016; published 15 January 2016

ABSTRACT

English mathematics Professor, Sir Andrew John Wiles of the University of Cambridge finally and conclusively proved in 1995 Fermat’s Last Theorem which had for 358 years notoriously resisted all gallant and spirited efforts to prove it even by three of the greatest mathematicians of all time―such as Euler, Laplace and Gauss. Sir Professor Andrew Wiles’s proof employed very advanced mathematical tools and methods that were not at all available in the known World during Fermat’s days. Given that Fermat claimed to have had the “truly marvellous” proof, this fact that the proof only came after 358 years of repeated failures by many notable mathematicians and that the proof came from mathematical tools and methods which are far ahead of Fermat’s time, has led many to doubt that Fermat actually did possess the “truly marvellous” proof which he claimed to have had. In this short reading, via elementary arithmetic methods, we demonstrate conclusively that Fermat’s Last Theorem actually yields to our efforts to prove it.

Keywords:

Diophantine Equations, Fermat’s Last Theorem, Fundamental Theorem of Arithmetic

“The world which we have to build from the crude material is the world of perception, and the process of building must depend on the nature of the percipient.”

Sir Arthur S. Eddington (1882-1944)

1. Introduction

The pre-eminent French lawyer and amateur¹ mathematician, Advocate―Pierre de Fermat (1607-1665) in 1637, famous in the margin of a copy of the famous book Arithmetica which was written by Diophantus of Alexandria (~201 - 215 AD), wrote:

“It is impossible to separate a cube into two cubes, or a fourth power into two fourth powers, or in general, any power higher than the second, into two like powers. I have discovered a truly marvellous proof of this, which this margin is too narrow to contain.”

In the parlance of mathematical symbolism, this can be written succinctly as:

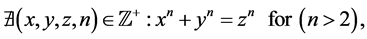(1)

where the triple, is piecewise coprime, and 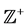is the set of all positive integer numbers. This theorem is classified among the most famous theorems in all History of Mathematics and prior to 1995, proving it was―and is ranked in the Guinness Book of World Records as one of the “most difficult mathematical problems” known to humanity. Fermat’s Last Theorem is now a true theorem since it has been proved, but prior to 1995 it was only a conjecture. Before it was proved in 1995, it is only for historic reasons that it was known by the title “Fermat’s Last Theorem”.

Rather “notoriously”, it stood as an unsolved riddle in mathematics for well over three and half centuries. Many amateur and great mathematicians tried but failed to prove the conjecture in the intervening years 1637- 1995; including three of the World’s greatest mathematicians such as Italy’s Leonhard Euler (1707-1783), France’s Pierre-Simon, marquis de Laplace (1749-1827), and the celebrated mathematical prodigy, genius and Crown Prince of Mathematics, Germany’s Johann Carl Friedrich Gauss (1777-1855), amongst many other notable and historic figures of mathematics.

Without any doubt, the conjecture or Fermat’s Last Theorem is in-itself―as it stands as a bare statement, deceptively simple mathematical statement whose any agile 10-year-old mathematical prodigy can fathom with relative ease. As already said, Fermat famous―via his bare marginal note stated he had solved the riddle around 1637. His claim was discovered some 30 years later after his death in 1665, as an overly simple statement in the margin of the famous copy Arithmetica. As it is well known from the history of mathematics, Fermat wrote many notes in the margins and most of these notes were “theorems” that he claimed to have solved himself. Some of the proofs of his assertions were found. For those that were not found, all the proofs save for one resisted all intellectually spirited efforts to prove it and this was the marginal note pertaining the so-called Fermat’s Last Theorem.

This marginal note dubbed Fermat’s Last Theorem, was the last of the assertions made by Fermat whose proof was needed, and for this reason that it was the last of Fermat’s statement that stood unproven, it naturally found itself under the title “Fermat’s Last Theorem”. Because all of the many of Fermat’s assertions were eventually proved, most people believed that this last assertion must―too, be correct as Fermat had claimed. Few― if any, doubted the assertion may be false, hence the confidence to call it a theorem. Simple, the proof Fermat claimed to have had, had to be found! Alas, reality could prove otherwise that the proof was not a mere summer walk in the park.

So the question is: Did Fermat actually posses the so-called “truly marvelous” proof which he claimed to have had? This is the question many have justly and rightly asked over the years and this reading makes the temerarious endeavour to vindicate Fermat that he very well might have had the “truly marvellous” proof he claimed to have had and we accomplish by providing a proof that employs elementary arithmetic methods that were available in Fermat’s day.

Surely, there are just reasons to doubt Fermat actually had the proof and this is so given the great many notable mathematicians that tried and monumentally failed and as-well, gave the number of years it took to find the first correct proof. The first correct proof was supplied only 358 years later by the English Professor of mathematics at the University of Cambridge―Sir Professor Andrew John Wiles, in 1995 [1] .

To add salt to injury i.e. add onto the doubts on whether or not Fermat actually had his so-called “truly marvellous” proof is that Sir Professor Andrew Wiles’s proof² employs highly advanced mathematical tools and methods that were not at all available in the known World during Fermat’s days. Actually, these tools and methods were invented (discovered) in the relentless effort to solve this very problem. Herein, we supply a very simple proof of Fermat’s Last Theorem.

That said, we must hasten to say that, as a difficult mathematical problem that so far yielded only to the difficult, esoteric and advanced mathematical tools and methods of Sir Professor Andrew Wiles―Fermat’s Last Theorem, as any other difficult mathematical problem in the History of Mathematics, it has had a record number of incorrect proofs of which the present may very well be an addition to this long list of incorrect proofs. In the words of historian of mathematics―Howard Eves [2] :

“Fermat’s Last Theorem has the peculiar distinction of being the mathematical problem for which the greatest number of incorrect proofs has been published.”

With that in mind, it allows us to say, we are confident that the proof we supply herein is water-tight and most certainly correct and that, it will stand the test of time and experience.

As stated in the ante-penultimate above, in this rather short reading, we make the temerarious endeavour to answer this question―of whether or not Fermat actually possessed the proof he claimed to have had. We accomplish by supplying a simple and elementary proof that does not require any advanced mathematics but mathematics that was available in the days of Fermat. Sir Professor Andrew Wiles’s acclaimed proof, is at best very difficult and to the chagrin of they that seek a simpler understanding―the proof is nothing but highly esoteric. The question thus “forever” hangs in there to the searching and inquisitive mind: “Did Fermat really possess the proof he claimed to have had?” The proof that we supply herein leads us to strongly believe that Fermat might have had the proof and this proof most certainly employed elementary methods of arithmetics!

2. Proofs for Specific Indices

Before we go into the main business of the day, we shall give a short history of some notable efforts in finding a proof to Fermat’s Last Theorem. As is well known, the case for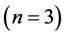, for all non-zero 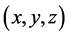and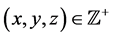, the equation admits no solutions. This was first proved by the great Italian mathematician Leonhard Euler in 1770 [3] , that is, 133 years after Fermat set into motion Fermat’s Last Theorem. Euler used the technique of infinite descent. Euler’s proof is not the only proof possible as other authors have published their independent proofs (cf. [4] -[8] , amongst many others).

Fermat was the first to provide a proof for the case 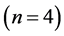which stated that for all non-zero piecewise coprime triple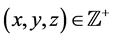, the equation 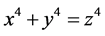admits no solutions. This proof by Fermat is the only surviving proof of Fermat’s Last Theorem and as is the case with Euler’s proof for the case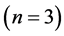, Fermat’s proof makes use of the technique of infinite descent. One wonders whether or not Fermat conducted this proof as part of a more general proof for all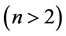. As is the case with Euler’s proof for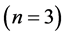, Fermat’s proof is not the only proof possible as other authors have published their independent proofs (see e.g. Refs. [5] [6] [9] -[11] , amongst many others). Even after Sir Professor Andrew Wiles’s 1995 breakthrough [1] , researchers are still publishing variants of the proof for the case (cf. [12] -[14] ).

The case 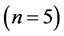was first proved independently by the French mathematician Adrien-Marie Legendre (1752-1833) and the German mathematician Johann Peter Gustav Lejeune Dirichlet (1805-1859) around 1825 and alternative and independent proofs were developed in the later years by others (cf. [5] [15] -[22] , amongst many others).

3. Lemma (I)

If where and is one of the prime factors of a, then, the following will hold true always:

(2)

The above statement is clearly evident and needs no proof. However, below we demonstrate that this statement is true. This demonstration does not constitute a proof.

What this statement really means is that the number [for any and], can always be

written as a sum or difference of two numbers p and q where are not co-prime, i.e.:

(3)

since one can always find some such that a will always be a common factor of, that is to say:

(4)

in which case we will have and where such that and. To see this, we know that if is the set of all the prime factors of a, then

where. Substituting all this into (4), we will have:

(5)

Setting and, (5) leads us to (2). As we did with Beal’s Conjecture in [23] , equipped with this simple fact, we will demonstrate that as we did with that Fermat’s Last Theorem yields to a proof in the simplest imaginable manner.

Proof.

If, then, according to the fundamental theorem of arithmetic, we can decompose the number a into its prime factors i.e.:

(6)

where and the’s are the prime factors of a and these are such that . We will have if and only if a is prime.

Now, and since and, it is clear that. Clearly, if, we can write where. From the foregoing, it follows from and, that. From (6), it follows that:

(7)

where and where. Hence result is proven. Now we proceed to the main task of the present reading.

4. Proof of Fermat’s Last Theorem (I)

Now, the proof that we are going to provide of FTL is a proof by contradiction and this proof makes use of Lemma §(3) whereby we demonstrate that the triple is such that it will always have a common factor if the equation, for all; is to hold true. We begin by assuming the statement:

(8)

to be true for some piecewise co-prime triple, the meaning of which is that the greatest common divisor of this triple or any pair of the triple is unity i.e., .

1) We must realise that if just one of the members of the triple is equal to unity for any, then, the other two members of this triple cannot be integers, hence, from this it follows that if a solution exists,

then, all the members of this triple will be greater than unity i.e..

2) By way of contradiction, we assert that there exists a set of positive integers that satisfy the simple relation for some piecewise co-prime triple. Having made this assumption, if we can show that, then, by way of contradiction FTL holds true.

3) If the statement (8) holds true, then―clearly; there must exist some such that , such that, and can be decomposed as follows:

(9)

4) According to the Lemma §(3), the equation; for any and for any, this equation, can always be written such that and for some and is any of the prime factors of z. Putting everything together, we will have. Substituting and into (9), we will have:

(10)

5) From (10), it is clear that since there exists a common divisor of the triple which is, that is to say, is a common divisor of the triple. If

, consequently, and this is in complete violation of the critical, crucial and sacrosanct assumption that. Q.E.D.

Alternatively, according to the Lemma §(3), the equation for any and for any, this equation, can always be written such that and for some and

is any of the prime factors of x; putting everything together, we will have. Now, substituting and into (9), we will have:

(11)

Again, from (11), it is clear that since the, that is to say, is a common divisor of triple. From the foregoing, it follows that the prime factors of are common divisors of the triple, the meaning of which is that.

Therefore, by way of contradiction, Fermat’s Last Theorem is true since we arrive at a contradictory result that. What this effectively means is that the equation for has a solution and this solution is such that the triple always has a common factor as is the case with all those values of that satisfy Fermat’s Last Theorem.

5. General Discussion

If the proof we have provided herein stands the test of time and experience, then, it is without a doubt that Fermat’s claim to have had a ‘truly marvellous’ proof may very well resonate with truth. The proof provided herein is not only simple, but surprisingly simple, so simple that one wonders how great mathematicians would have missed this. All this simplicity is embodied in Lemma (3). As we anxiously await the World to judge our proof, effort and work, we must―if this be permitted at this point of closing, say that, we are confident that―simple as it is or may appear, this proof is flawless, it will stand the test of time and experience. It strongly appears that the great physicist and philosopher―Albeit Einstein (1879-1955), was probably right in saying that “Subtle is the Lord. Malicious He is not”. Because in Lemma §(3), there exists deeply embedded therein, a subtlety that resolves and does away with the malice and notoriety associated with Fermat’s Last Theorem in a simpler and truly marvellous and general manner.

6. Conclusion

Given that the method used here to prove Fermat’s Last Theorem is so elementary, it is very much possible that Fermat actually processed the correct proof.

Cite this paper

Golden GadzirayiNyambuya, (2016) On a Simpler, Much More General and Truly Marvellous Proof of Fermat’s Last Theorem (I). Advances in Pure Mathematics,06,1-6. doi: 10.4236/apm.2016.61001

References

NOTES

¹While Fermat is ranked as one of the greatest mathematicians of the World, he modestly considered himself an amateur in the field.

²The proof by Sir Professor Wiles is well over 100 pages long and consumed about seven years of his research time. For this notable achievement of solving Fermat’s Last Theorem, he was Knighted Commander of the Order of the British Empire in 2000 by Her Majesty Queen Elizabeth (II), and received many other honours around the world.