ABSTRACT

We demonstrate the functional inverse of a Winter map, which is an analog of the exponential map, for Lie algebras over fields of prime characteristic.

Keywords:Prime-Characteristic Lie Algebras, Prime-Characteristic Lie Groups

“Historically,” note Strade and Farnsteiner in [1] , “Lie algebras emerged from the study of Lie groups.” In Section 1.1 of [1] , they give a simple example of the close connection between Lie algebras and Lie groups. In prime characteristic, David Winter [2] has defined maps which mimic the zero-characteristic exponential maps. See also Lemma 1.2 of [3] . In this paper, we focus on the following “Winter maps”: if is an element of a characteristic- Lie algebra such that 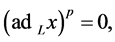we set

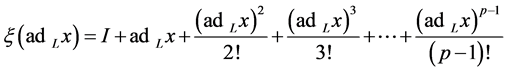
where is the identity transformation of. Such ad-nilpotent elements of degree less than do exist in some graded Lie algebras, as can be seen from Lemma 2.3 and Proposition 2.7 of Chapter 4 of [1] , as well as from Lemma 1 of [4] ; of course, it is well known that non-zero-root vectors of simple classical-type Lie algebras are ad-nilpotent of degree less than or equal to four.

We will show here that for 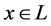such that 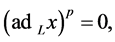the inverse of 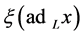as a linear transformation of is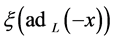, so that such transformations generate a group 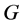of linear transformations of. We will also show that 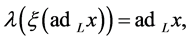where, for a linear transformation of, and as above, we define

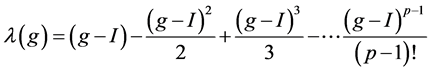(1)

Thus, like 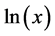and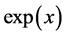, is, in a sense, the functional inverse of.

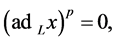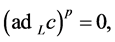Lemma 1 If and are elements of such that and then

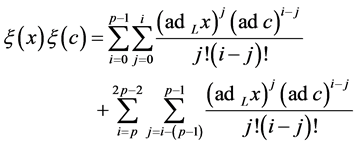
Proof. We group terms with respect to total degree in 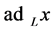and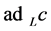

Lemma 2 Let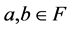, and suppose that is an element of such that 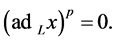then

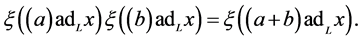
Proof. We have by Lemma 1 that 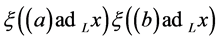equals

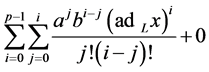
which we can write in terms of binomial coefficients as

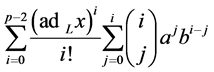
By the Binomial Theorem, the above expression is equal to

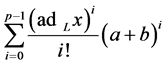
which we can rewrite as

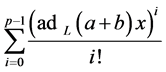
and recognize as.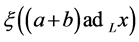

Lemma 3 For any integer 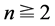and any integer, , 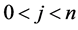we have

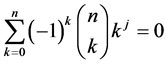
Proof. We proceed by induction on and. When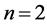, we must have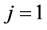, and we have 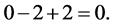For any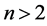, when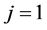, we have

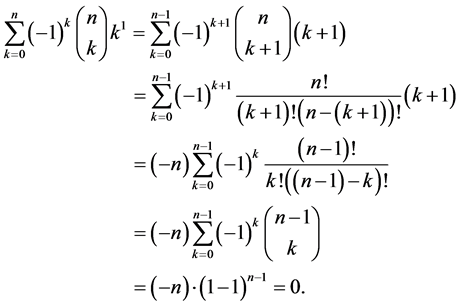
Now, for any 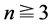and any positive integer less than, suppose that 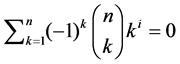for all positive 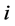less than Then we have

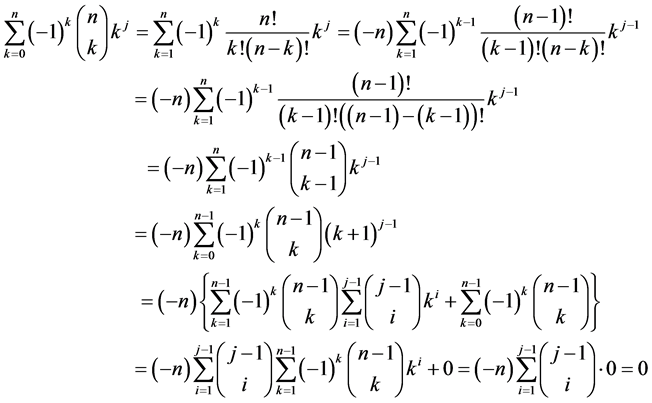
by induction, and the fact that 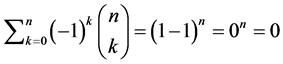(the “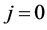case”).

Lemma 4 Let be an element of such that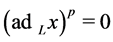. Define

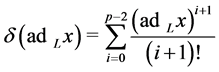(2)

Then for any positive integer less than,

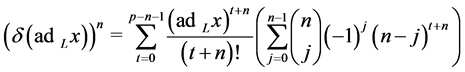(3)

Proof. We proceed by induction on. Since when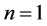, (3) is just (2), the initial step of the induction proof is established. Suppose (3) is true for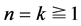. Then 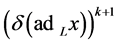equals

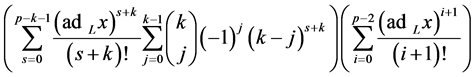
We group terms with respect to total degree (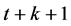, in this case) in 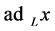and get that

.

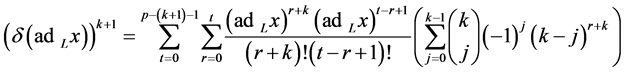Rewriting the above expression using another binomial coefficient, we get that 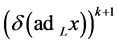equals

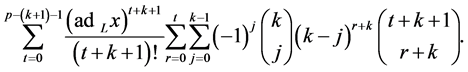
We change the order of summation to get

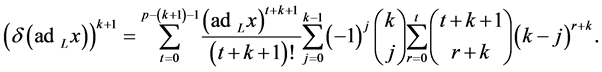
We replace the index of summation by 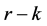to get

.

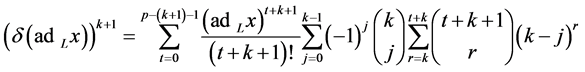Adding and subtracting terms, we get

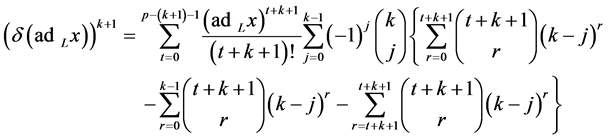
Setting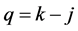, we see, as in the proof of Lemma 3, that when r ≥ 1,

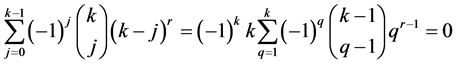
by that same Lemma 3. Thus,

so from the Binomial Theorem, we get that 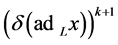equals

.

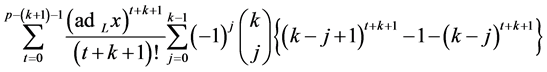We now distribute to get that 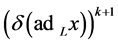equals

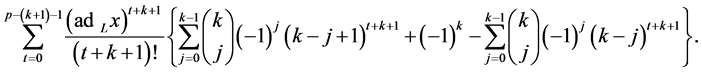
We replace the latter index of summation by 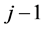to get that 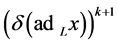equals

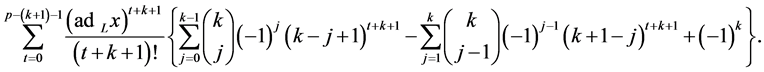
We change the order of summation and factor to get that 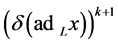equals

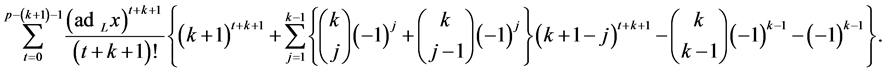
By binomial arithmetic 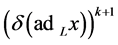equals

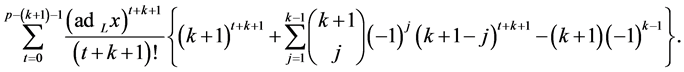
The above displayed formula is just (3) for; i.e., 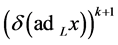equals

.

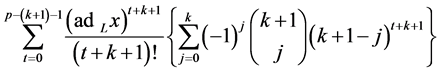Thus, the induction step is complete.

Theorem The linear transformation 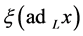of has 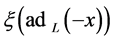as its inverse, whereas the map of 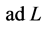to the group of non-singular linear transformations of has as its inverse, in the sense that

(a).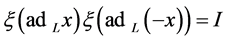, and

(b)..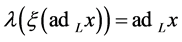

Proof. (a) If, in Lemma 2, we let 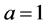and, we see that (a) is true.

(b) Since 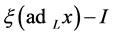equals the 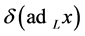of Lemma 4, we have that 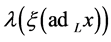equals

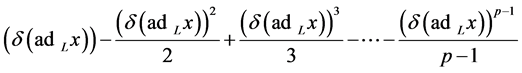
which, by Lemma 4 equals

We replace the index by to get that

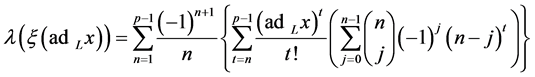
We change the order of summation to get that

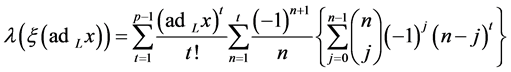
We replace the index by 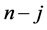to get that

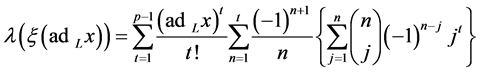
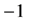We cancel an and a and combine the factors to get that

We replace the index by 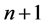and we replace the index by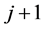, and we get that

We change the order of summation to get that

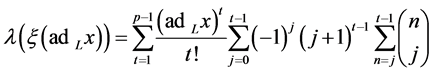
We now appeal to a little more binomial arithmetic to observe that since 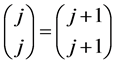and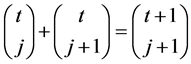, it follows by induction that

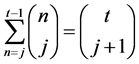
from which we obtain that

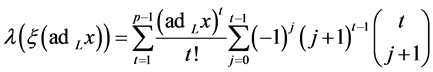
We replace the index by 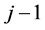to get that

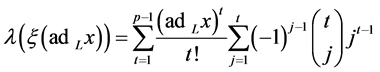
Finally, we use Lemma 3 to see that we are left with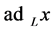

References