Advances in Pure Mathematics
Vol.4 No.8(2014), Article
ID:48785,11
pages
DOI:10.4236/apm.2014.48046
On the Stability of Solutions of Nonlinear Functional Differential Equation of the Fifth-Order
A. M. A. Abou-El-Ela1, A. I. Sadek1, A. M. Mahmoud2, R. O. A. Taie1
1Department of Mathematics, Faculty of Science, Assiut University, Assiut, Egypt
2Department of Mathematics, Faculty of Science, New Valley Branch, Assiut University, New Valley, El-Khargah, Egypt
Email: a.el-ela@aun.edu.eg, Sadeka1961@hotmail.com, math_ayman27@yahoo.com, Rasha_omath@yahoo.com
Copyright © 2014 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 17 June 2014; revised 15 July 2014; accepted 21 July 2014
ABSTRACT
The main purpose of this paper is to investigate global asymptotic stability of the zero solution of the fifth-order nonlinear delay differential equation on the following form
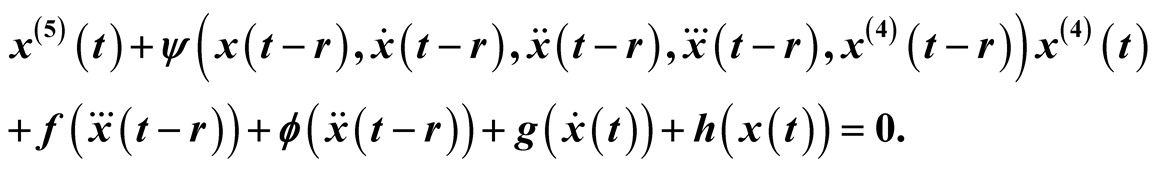 By constructing a Lyapunov functional, sufficient conditions for the stability of the zero solution of this equation are established.
By constructing a Lyapunov functional, sufficient conditions for the stability of the zero solution of this equation are established.
Keywords:Global Asymptotic Stability, Lyapunov Functional, Fifth-Order Delay Differential Equations

1. Introduction
As is well-known, the area of differential equations is an old but durable subject, that remains alive and useful to a wide variety of engineers, scientists and mathematicians. Now the subject of differential equations represents a huge body of knowledge including many subfields and a vast array of applications in many disciplines. It should be noted that principles of differential equations are largely related to the qualitative theory of ordinary differential equations. Qualitative theory refers to the study of behaviour of solutions, for example, the investigation of stability, instability, boundedness of solutions and etc., without determining explicit formulas for the solutions. In particular one can refer that many authors have dealt with delay differential equations and its problems, and many excellent results have been obtained on the behaviour of solutions for various higher-order: second-, third-, fourth-, and fifth-order nonlinear differential equations with delay, for example, [1] -[27] , and references quoted therein, which contain the differential equations without delay or with delay. In many of these references, the authors dealt with the problems by using Lyapunov’s second method [28] . By considering Lyapunov functionals we obtained the conditions which ensured the stability of the problem. It is worth-mentioning that construction of these Lyapunov functionals remains a general problem. We know that a similar problem exists for ordinary differential equations for higher-order [12] . Clearly, it is even more difficult to construct Lyapunov functionals for delay differential equations of higher-order. Up to this moment the investigations concerning the stability of solutions of nonlinear equations of fifth-order with delay have not been fully developed.
In particular in 2010 Tunç [29] obtained sufficient conditions, which ensure the stability of the zero solution of a nonlinear delay differential equation of fifth-order
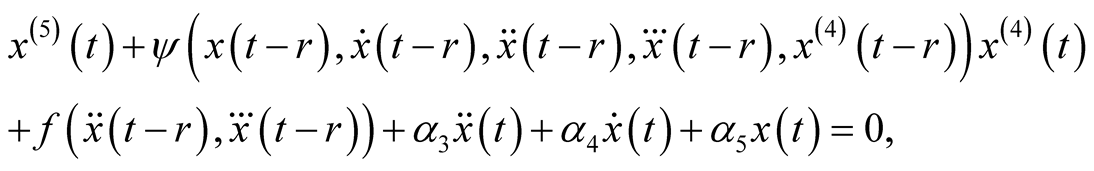
where
 and f are continuous functions;
and f are continuous functions; ,
,
 and
and
 are positive constants, r is a bounded delay and positive constant; the derivatives
are positive constants, r is a bounded delay and positive constant; the derivatives
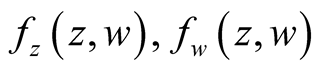 exist and are continuous for all z, w and
exist and are continuous for all z, w and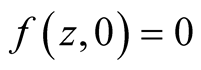 .
.
Later in 2011 Abou-El-Ela, Sadek and Mahmoud [30] obtained the sufficient conditions for the uniform stability of the zero solution of a nonlinear fifth-order delay differential equation of the following form
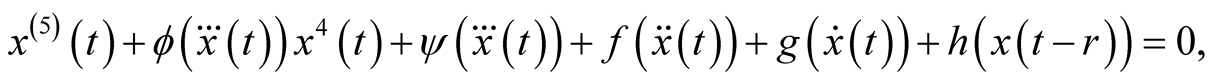
where
 is a positive constant;
is a positive constant;
 and
and
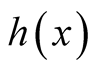 are continuous functions and
are continuous functions and
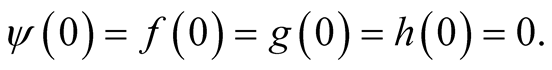
In the present paper, we are concerned with the stability of the zero solution of the fifth-order nonlinear delay differential equation on the form
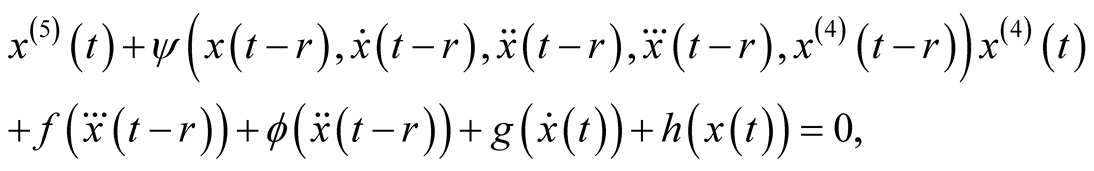 (1.1)
(1.1)
or its equivalent system form
 (1.2)
(1.2)
where ,
,
 ,
,
 ,
,
 and
and
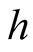 are continuous functions for the arguments displayed explicitly in (1.1) with
are continuous functions for the arguments displayed explicitly in (1.1) with
 r is a bounded delay and positive constant; the derivatives
r is a bounded delay and positive constant; the derivatives
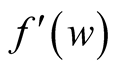 and
and
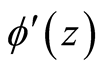 exist and are continuous for all
exist and are continuous for all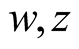 .
.
2. Preliminaries and Stability Results
In order to reach the main result of this paper, we will give some basic information to the stability criteria for the general autonomous delay differential system. We consider
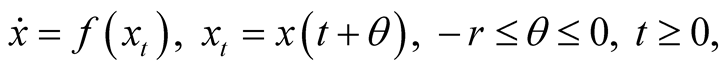 (2.1)
(2.1)
where
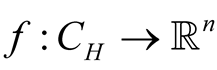 is a continuous mapping,
is a continuous mapping,
 and for
and for , there exists
, there exists
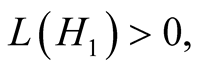 with
with
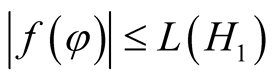 when
when
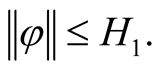
The following are the classical theorems on uniform stability and global asymptotic stability for the solution of (2.1).
Theorem 2.1. [31] . Let
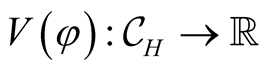 be a continuous functional satisfying a local Lipschitz condition
be a continuous functional satisfying a local Lipschitz condition
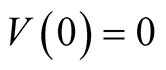 and the functions
and the functions
 are wedges such that i)
are wedges such that i)
 and ii)
and ii) .
.
Then the zero solution of (2.1) is uniformly stable.
Theorem 2.2. [32] . Suppose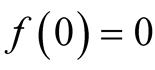 , let V be a continuous functional defined on
, let V be a continuous functional defined on
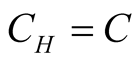 with
with , and let
, and let
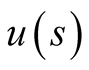 be non-negative and continuous function for
be non-negative and continuous function for ,
,
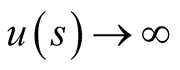 as
as
 such that for all
such that for all
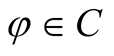
i)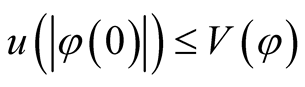 ,
,
 and ii)
and ii)
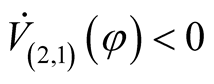 for
for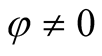 .
.
Then all solutions of (2.1) approach zero as
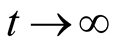 and the origin is globally asymptotically stable.
and the origin is globally asymptotically stable.
The following will be our main stability result for (1.1).
Theorem 2.3. In addition to the basic assumptions imposed on the functions
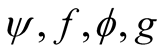 and h. Suppose that the following conditions are satisfied, where
and h. Suppose that the following conditions are satisfied, where
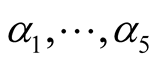 are arbitrary positive constants and
are arbitrary positive constants and
 and L are sufficiently small positive constants i)
and L are sufficiently small positive constants i)
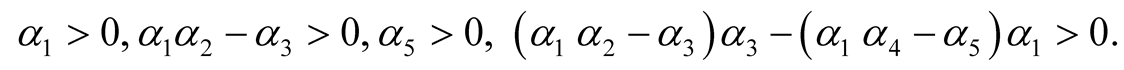
 (2.2)
(2.2)
and the following two inequalities
 (2.3)
(2.3)
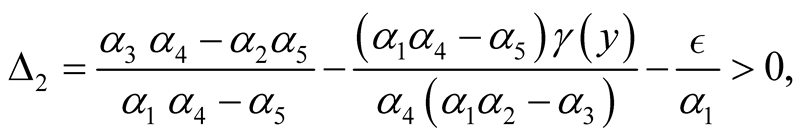 (2.4)
(2.4)
for all
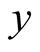 and all
and all
 where
where
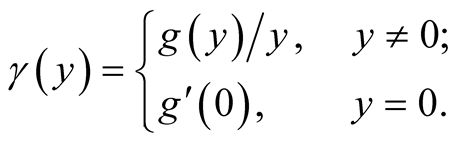
ii)
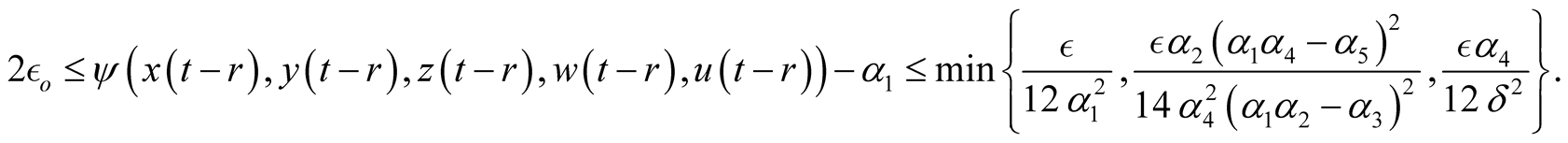
iii)
 and
and
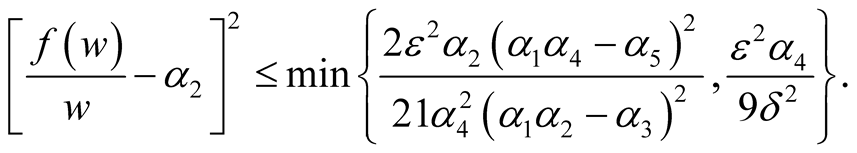
iv)
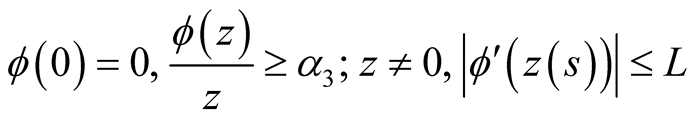 and
and
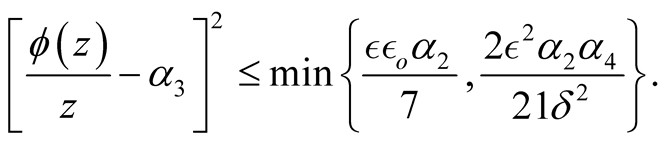
v)
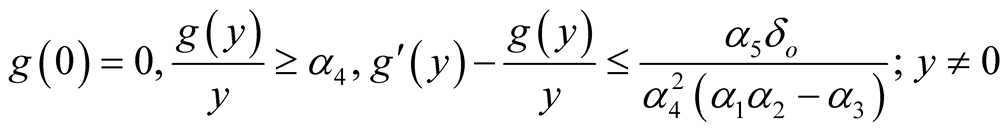 and
and
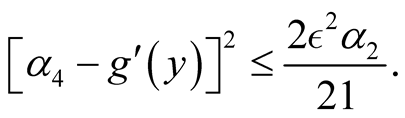
vi)
 and
and
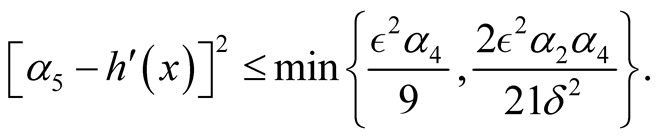
Then the zero solution of (1.1) is globally asymptotically stable, provided that
 .
.
Proof. We define the Lyapunov functional
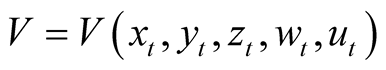 as:
as:
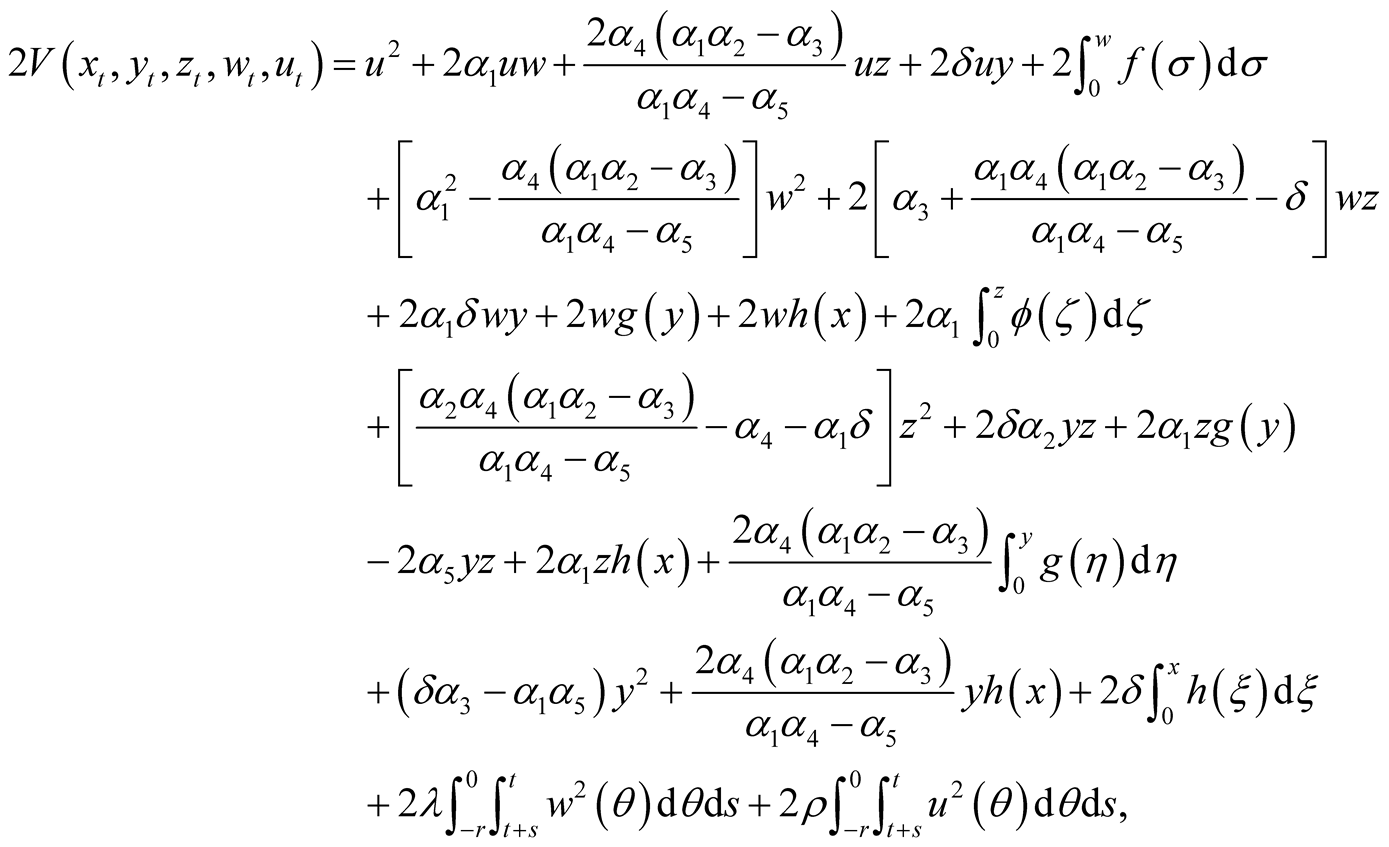 (2.5)
(2.5)
where
 and
and
 are two positive constants, which will be determined later and
are two positive constants, which will be determined later and
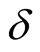 is a positive constant defined by
is a positive constant defined by
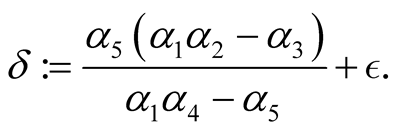 (2.6)
(2.6)
Then it is convenient to rewrite the expression for the Lyapunov functional defined in (2.5) in the following form
 (2.7)
(2.7)
where

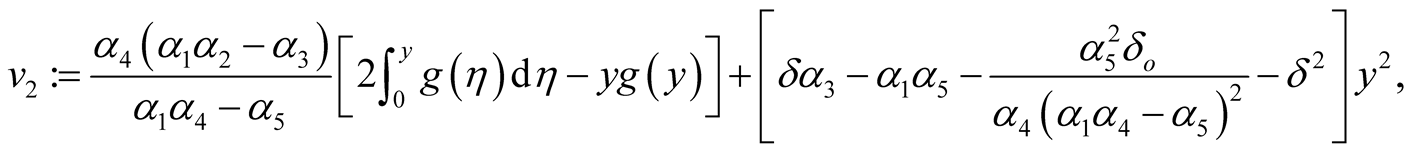

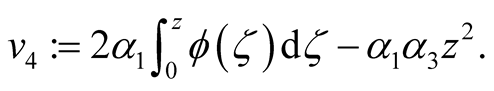
For the component , by using (2.6) and the definition of
, by using (2.6) and the definition of


since
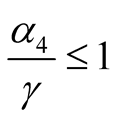 by v), thus we obtain
by v), thus we obtain

This is due to the fact that the integral on the right-hand side is non-negative by vi), therefore we get

From the identity
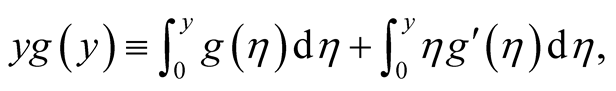
therefore

and by using v) we find
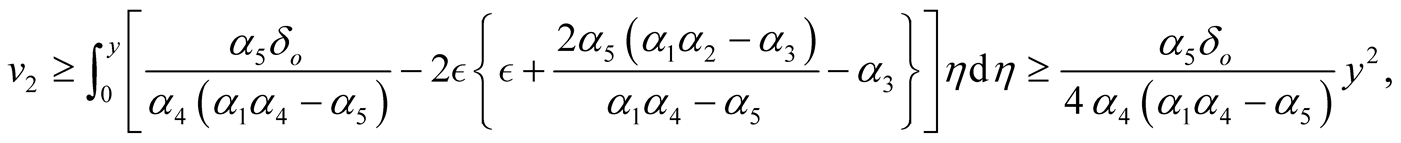
provided that
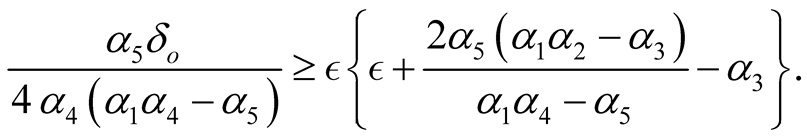

From iv) we find
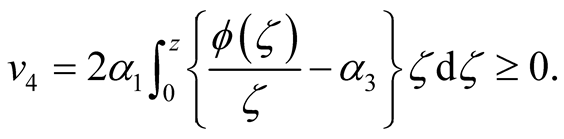
Summing up the four inequalities obtained from
 into (2.7), we have
into (2.7), we have
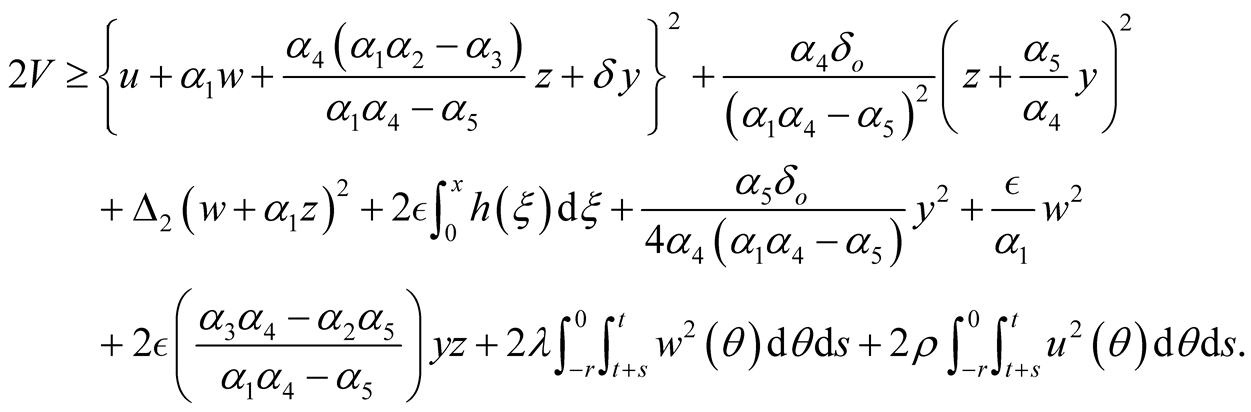 (2.8)
(2.8)
Clearly, it follows from the first six terms included in (2.8) that there exist sufficiently small positive constants
 such that
such that
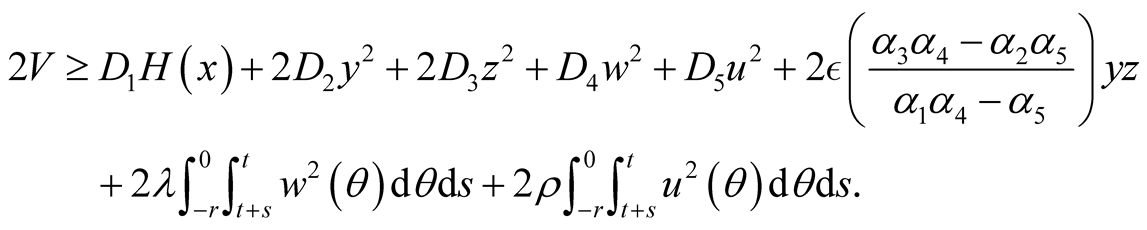 (2.9)
(2.9)
Now we consider the terms

which are contained in (2.9) and by using the inequality , we obtain
, we obtain
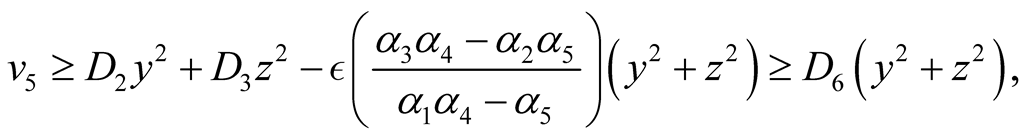
for some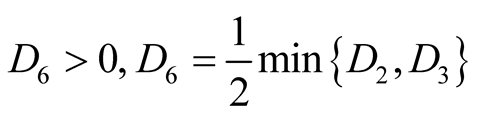 , if
, if

By using the previous inequality, we get from (2.9) that
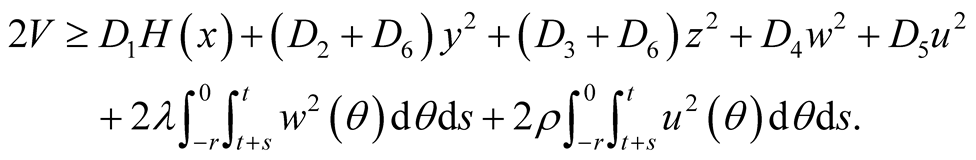 (2.10)
(2.10)
As a result, since the integrals
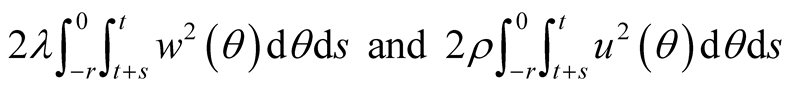
are non-negative, it is obvious that there exists a positive constant
 which satisfies the following inequality
which satisfies the following inequality
 (2.11)
(2.11)
where
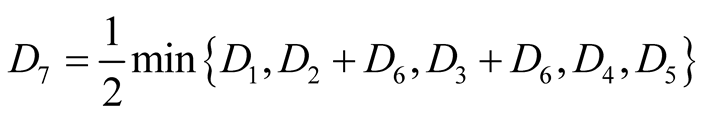 .
.
Now by a direct calculation from (1.2) and (2.5) one finds
 (2.12)
(2.12)
Making use of the assumptions ii)-vi), (2.3) and (2.6), we get
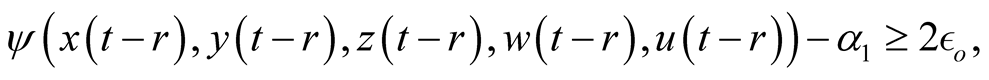


and

By v), vi) and (2.6).
By using the assumptions
 and
and
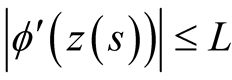 of the theorem and inequality
of the theorem and inequality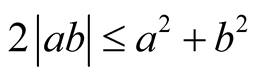 , we obtain the following inequalities
, we obtain the following inequalities
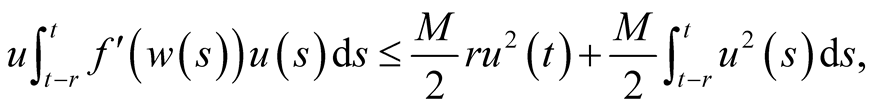
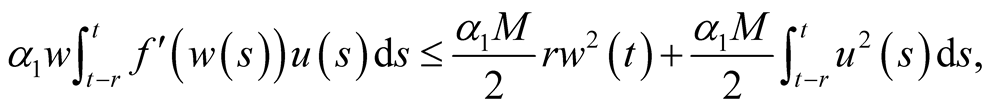
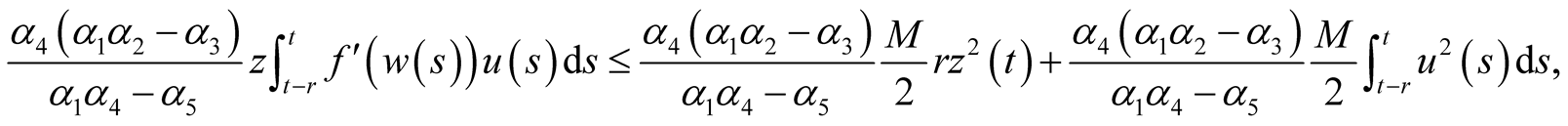


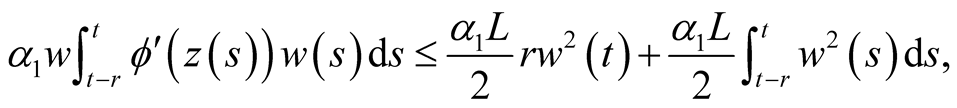
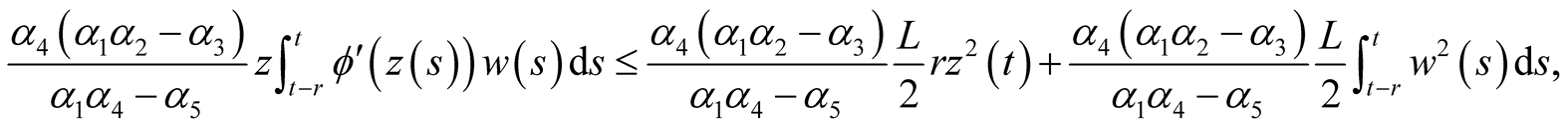
and
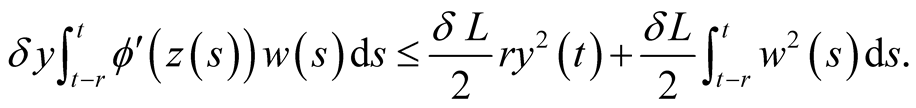
Replacing the last equality and the preceding inequalities into (2.12), we obtain
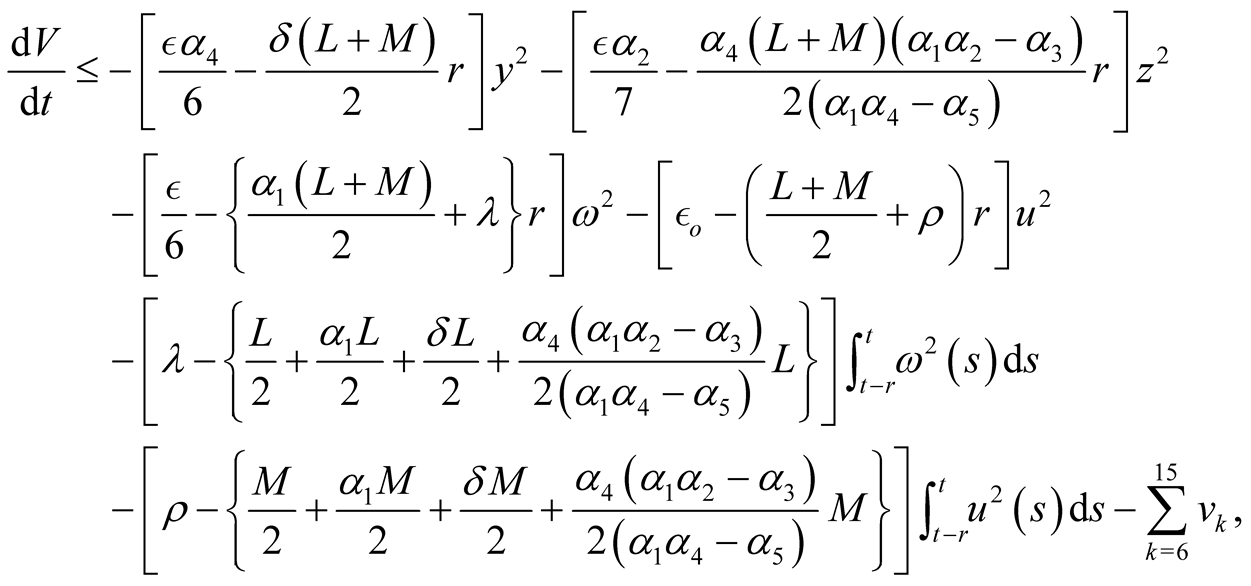 (2.13)
(2.13)
where

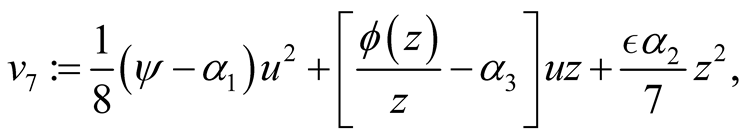

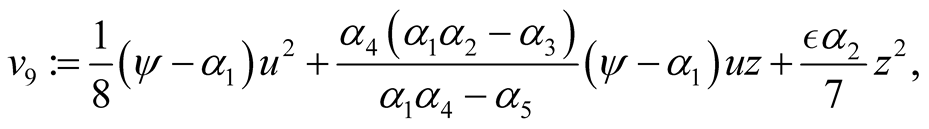
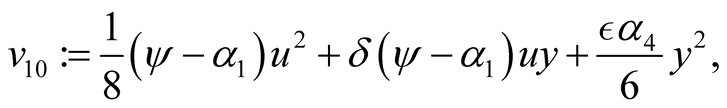
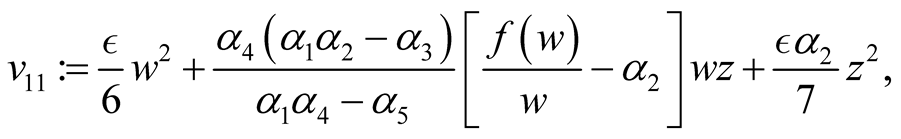


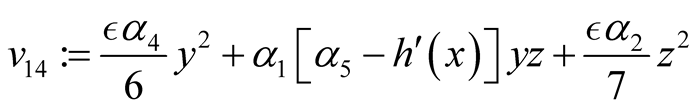
and

It is clear that the expressions given by
 and
and
 represent certain specific quadratic forms, respectively.
represent certain specific quadratic forms, respectively.
Making use of the basic information on the positive semi-definite of a quadratic form, one can easily conclude that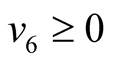 ,
,
 ,
,
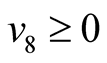 ,
,
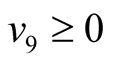 ,
,
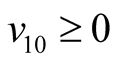 ,
,
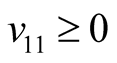 ,
,
 ,
,
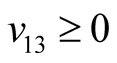 ,
,
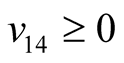 and
and
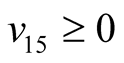 provided that
provided that

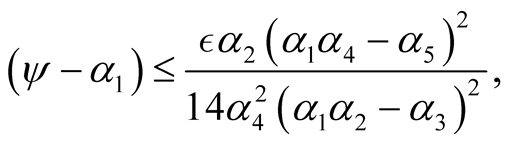
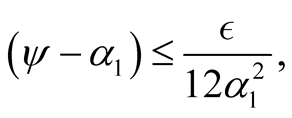
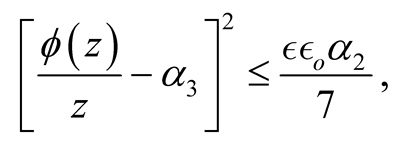

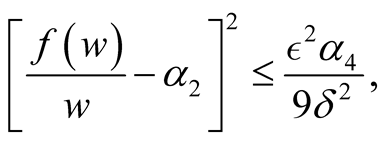
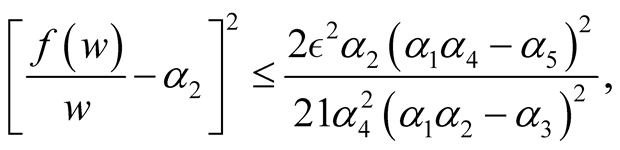

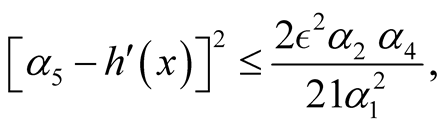
and

respectively.
Thus in view of the above discussion and inequality (2.13), it follows that
 (2.14)
(2.14)
So we can choose the constants
 and
and
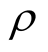 as the following
as the following
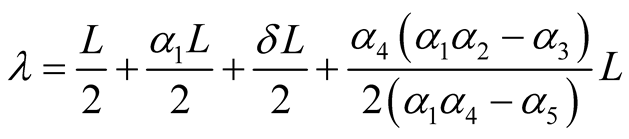
and
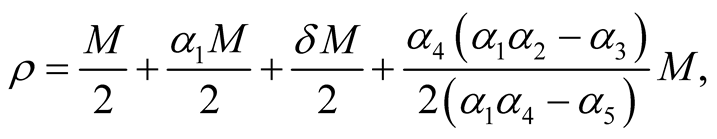
then the inequality in (2.14) implies that
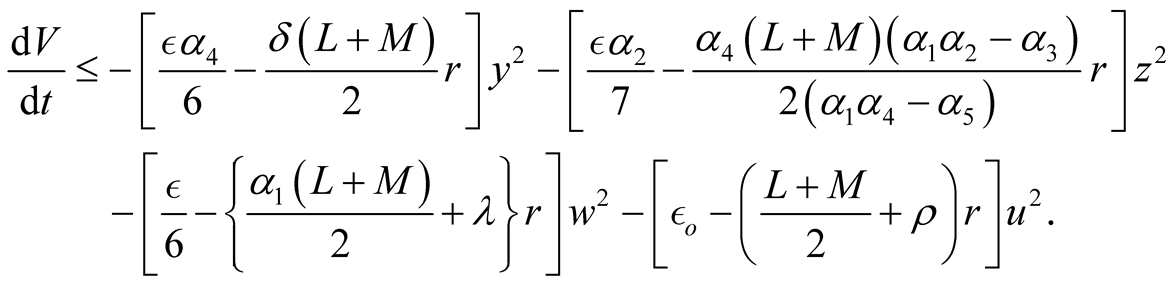 (2.15)
(2.15)
Hence one can easily get from (2.15) that
 (2.16)
(2.16)
for some positive constants , provided that
, provided that
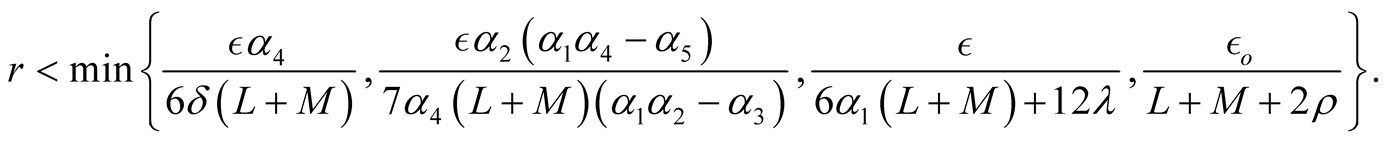
Finally, it follows that
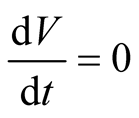 if and only if
if and only if
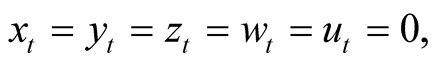
 for
for
 and
and
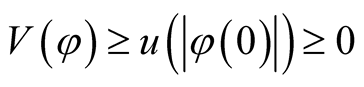 .
.
Thus all the conditions of Theorem 2.2 are satisfied. This shows that the zero solution of (1.1) is globally asymptotically stable.
Then the proof of Theorem 2.3 is completed.
References
- Adesina, O.A. (2007) Convergence Results for Solutions of a Certain Fifth-Order Nonlinear Differential Equation. Nonlinear Studies, 14, 289-300.
- Bereketoglu, H. (1998) Asymptotic Stability of a Fourth-Order Delay Differential Equation. Dynamic Systems an Applications, 7, 105-115.
- Burton, T.A. (1983) Volterra Integral and Differential Equations. Academic Press, New York.
- Burton, T.A. (1985) Stability and Periodic Solutions of Ordinary and Functional Differential Equations. Academic Press, New York.
- Chukwu, E.N. (1978) On the Boundedness and the Existence of a Periodic Solution of Some Nonlinear Third-Order Delay Differential Equation. Atti Accad. Naz. Lincei Rend. Cl. Sci. Fis. Mat. Nat., 64, 440-447.
- Harrow, M. (1967) A Stability Result for Solutions of Certain Fourth-Order Homogeneous Differential Equations. Journal London Mathematical Society, 42, 51-56. http://dx.doi.org/10.1112/jlms/s1-42.1.51
- Harrow, M. (1970) On the Boundedness and the Stability of Solutions of Some Differential Equations of the Fourth-Order. SIAM Journal on Mathematical Analysis, 1, 27-32. http://dx.doi.org/10.1137/0501002
- Hale, J.K. (1977) Theory of Functional Differential Equations. Springer-Verlag, New York. http://dx.doi.org/10.1007/978-1-4612-9892-2
- Kolmanovskii, V. and Myshkis, A. (1999) Introduction to the Theory and Applications of Functional Differential Equations. Kluwer Academic Publishers, Dordrecht. http://dx.doi.org/10.1007/978-94-017-1965-0
- Makay, G. (1994) On the Asymptotic Stability of the Solutions of Functional Differential Equations with Infinite Delay. Journal of Differential Equations, 108, 139-151. http://dx.doi.org/10.1006/jdeq.1994.1029
- Okoronkwo, E.O. (1989) On Stability and Boundedness of Solutions of a Certain Fourth-Order Delay Differential Equation. International Journal of Mathematics and Mathematical Sciences, 12, 589-602. http://dx.doi.org/10.1155/S0161171289000724
- Reissing, R., Sansone, E. and Conti, R. (1974) Non-Linear Differential Equations of Higher-Order. Noordhoof, Toom.
- Sadek, A.I. (2003) Stability and Boundedness of a Kind of Third-Order Delay Differential System. Applied Mathematics Letters, 16, 657-662. http://dx.doi.org/10.1016/S0893-9659(03)00063-6
- Sadek, A.I. (2004) On the Stability of Solutions of Certain Fourth-Order Delay Differential Equations. Applied Mathe-matics and Computation, 148, 587-597. http://dx.doi.org/10.1016/S0096-3003(02)00925-6
- Sadek, A.I. (2005) On the Stability of Solutions of Some Non-Autonomous Delay Differential Equations of the Third-Order. Asymptotic Analysis, 43, 1-7.
- Li, S.L. and Wen, L.Z. (1987) Functional Differential Equations. Hunan Science and Technology Press, Changsha.
- Sinha, A.S.C. (1973) On Stability of Solutions of Some Third and Fourth-Order Delay Differential Equations. Information and Control, 23, 165-172. http://dx.doi.org/10.1016/S0019-9958(73)90651-7
- Tejumola, H.O. and Tchegnani, B. (2000) Stability, Boundedness and Existence of Periodic Solutions of Some Third and Fourth-Order Nonlinear Delay Differential Equations. Journal of the Nigerian Mathematics Society, 19, 9-19.
- Tunc, C. (2002) A Study of the Stability and Boundedness of the Solutions of Nonlinear Differential Equations of the Fifth-Order. Indian Journal of Pure and Applied Mathematics, 33, 519-529.
- Tunc, C. (2005) Some Stability Results for the Solutions of Certain Fourth-Order Delay Differential Equations. Differential Equations & Applications, 4, 165-174.
- Tunc, C. (2006) On the Stability of Solutions of Certain Fourth-Order Delay Differential Equations. Applied Mathematics and Mechanics, 27, 1141-1148.
- Tunc, C. (2008) On the Stability of Solutions to a Certain Fourth-Order Delay Differential Equation. Nonlinear Dynamics, 51, 71-81. http://dx.doi.org/10.1007/s11071-006-9192-z
- Tunc, C. (2010) On Asymptotic Stability of Solutions of Fifth-Order Nonlinear Differential Equations with Delay. Functional Differential Equations, 17, 355-370.
- Tunc, C. (2013) New Results on the Stability and Boundedness of Nonlinear Differential Equations of Fifth-Order with Multiple Deviating Arguments. Bulletin of the Malaysian Mathematical Sciences Society, 36, 671-682.
- Yoshizawa, T. (1966) Stability Theory by Lyapunov’s Second Method. The Mathematical Society of Japan.
- Qin, Y., Liu, Y. and Wang, L. (1966) Stability of Motion for Dynamic Systems with Delay. Academic Press, Beijing.
- Zhu, Y. (1992) On Stability, Boundedness and Existence of Periodic Solution of a Kind of Third-Order Nonlinear Delay Differential System. Annals of Differential Equations, 8, 249-259.
- Lyapunov, A.M. (1966) Stability of Motion. Academic Press, London.
- Tunc, C. (2010) On the Stability of Solutions of Nonlinear Differential Equations of Fifth-Order with Delay. Mathematical Communications, 15, 261-272.
- Abou-El-Ela, A.M.A., Sadek, A.I. and Mahmoud, A.M. (2011) On the Stability of Solutions of a Certain Nonlinear Delay Differential Equation of the Fifth-Order. Proceedings of the 3rd Scientific Conference for Young Researchers, Assiut, 19-20 April 2011.
- Burton, T.A. and Hering, G. (1994) Lyapunov Theory for Functional Differential Equations. Rocky Mountain Journal of Mathematics, 24, 3-17. http://dx.doi.org/10.1216/rmjm/1181072449
- Hale, J.K. (1965) Sufficient Conditions for Stability and Instability of Autonomous Functional-Differential Equations. Journal of Differential Equations, 1, 452-482. http://dx.doi.org/10.1016/0022-0396(65)90005-7

