Advances in Pure Mathematics
Vol.2 No.6(2012), Article ID:24363,4 pages DOI:10.4236/apm.2012.26053
Some Symmetry Results for the A-Laplacian Equation via the Moving Planes Method*
School of Mathematical Sciences, Ocean University of China, Qingdao, China
Email: fangzb7777@hotmail.com
Received July 3, 2012; revised September 1, 2012; accepted September 8, 2012
Keywords: Symmetry; A-Laplacian; Moving Planes Method; Overdetermined Boundary Value Problem
ABSTRACT
In this paper, we are concerned with a positive solution of the non-homogeneous A-Laplacian equation in an open bounded connected domain. We use moving planes method to prove that the domain is a ball and the solution is radially symmetric.
1. Introduction
In this paper, we are going to study the symmetry results for the overdetermined problem
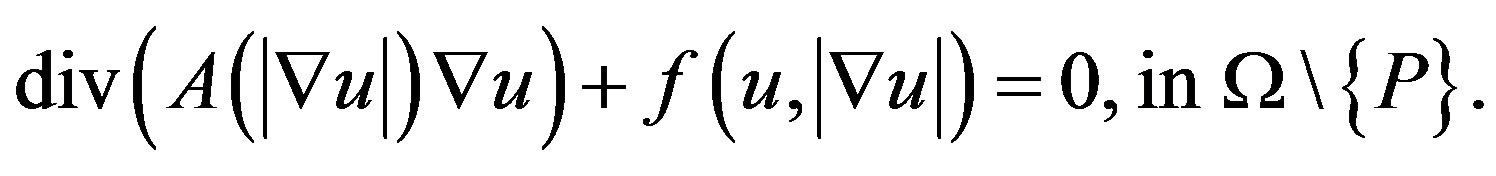 (1.1)
(1.1)
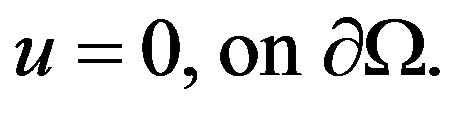 (1.2)
(1.2)
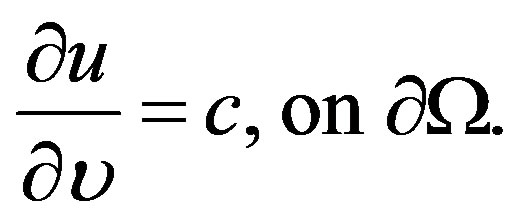 (1.3)
(1.3)
Here  is a bounded connected open subset of
is a bounded connected open subset of 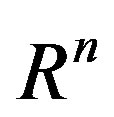 with
with 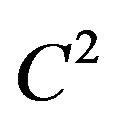 boundary and
boundary and 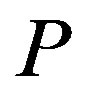 is a point in
is a point in . The function
. The function 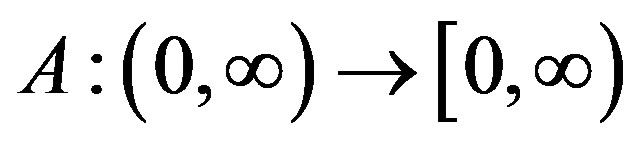 satisfies the regularity requirement
satisfies the regularity requirement
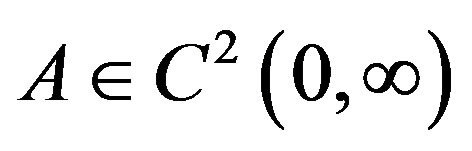 (1.4)
(1.4)
and the (possibly degenerate) elliptic condition
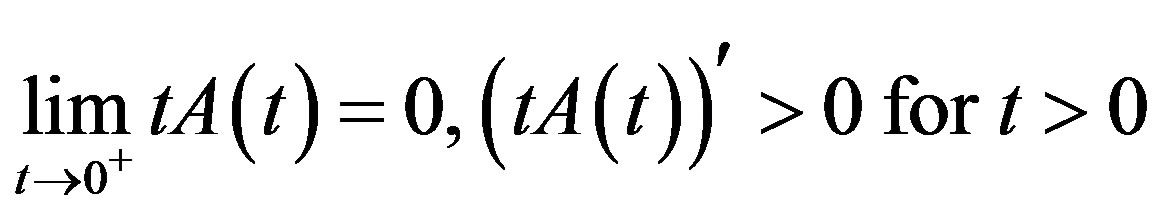 (1.5)
(1.5)
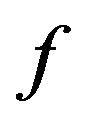 is a continuously differentiable function.
is a continuously differentiable function.  is a constant and
is a constant and 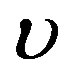 denotes the inner normal to
denotes the inner normal to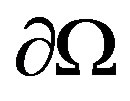 .
.
J. Serrin proved the radial symmetry for positive solutions of the equation 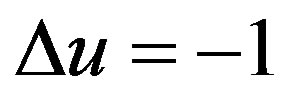 in
in  with the same overdetermined boundary conditions as the above problem, see [1]. N. Garofalo and J. Lewis extended Serrin’s result to a larger class of elliptic equations possibly degenerate, including the following p-Laplacian equation
with the same overdetermined boundary conditions as the above problem, see [1]. N. Garofalo and J. Lewis extended Serrin’s result to a larger class of elliptic equations possibly degenerate, including the following p-Laplacian equation
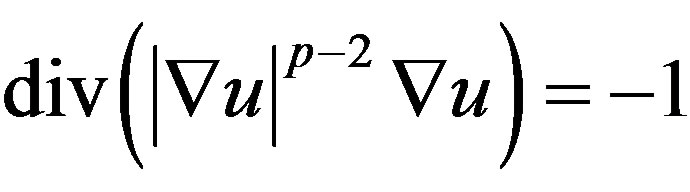 with the same boundary conditionssee [2]. For the overdetermined elliptic boundary value problem
with the same boundary conditionssee [2]. For the overdetermined elliptic boundary value problem 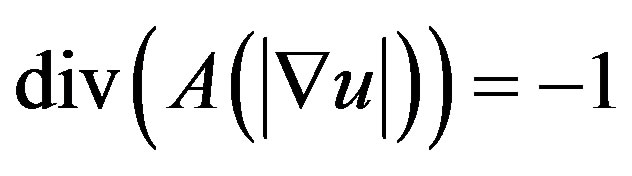 in
in  with the same overdetermined boundary conditions as above, I. Fragala, I. F. Gazzaola and B. Kawohl used the geometric approach which relies on a maximum principle for a suitable Pfunction, combined with some geometric arguments involving the mean curvature of
with the same overdetermined boundary conditions as above, I. Fragala, I. F. Gazzaola and B. Kawohl used the geometric approach which relies on a maximum principle for a suitable Pfunction, combined with some geometric arguments involving the mean curvature of  to prove that if the above problem admits a solution in a suitable weak sense, then
to prove that if the above problem admits a solution in a suitable weak sense, then  is a ball, see [3]. A. Farina and B. Kawohl obtained the same result under removing the strong ellipticity assumption in [4] and a growth assumption in [2] on the diffusion coefficient A, as well as a starshapedness assumption on
is a ball, see [3]. A. Farina and B. Kawohl obtained the same result under removing the strong ellipticity assumption in [4] and a growth assumption in [2] on the diffusion coefficient A, as well as a starshapedness assumption on  in [3], see [5]. A. Firenze considered the positive solution of problem (1.1)-(1.3) when it is a p-Laplacian equation in an open bounded connected subset
in [3], see [5]. A. Firenze considered the positive solution of problem (1.1)-(1.3) when it is a p-Laplacian equation in an open bounded connected subset  of
of 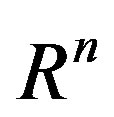 with
with 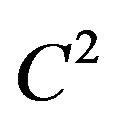 boundary, see [6]. All of the above motivated us to extend the symmetry result to the non-homogeneous A-Laplacian equation.
boundary, see [6]. All of the above motivated us to extend the symmetry result to the non-homogeneous A-Laplacian equation.
Our main result is that for the problem (1.1)-(1.3), if u has only one critical point in , then
, then  is a ball and u is radially symmetric.
is a ball and u is radially symmetric.
Section 2 of this paper is devoted to the main result and a more general version of this theorem. In Section 3, we will present the proof of the main theorem.
Some components, such as multi-leveled equations, graphics, and tables are not prescribed, although the various table text styles are provided. The formatter will need to create these components, incorporating the applicable criteria that follow.
2. Preliminaries and Statement of Results
In this section we give some lemma that we shall use and present our main result.
Lemma 2.1. (The boundary lemma at corner) (Lemma 2 in [1]) Let  be a domain with C2 boundary and
be a domain with C2 boundary and 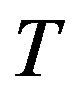 be a hyperplane containing the normal to
be a hyperplane containing the normal to 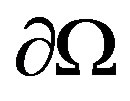 at some point
at some point . Let
. Let  denote the portion of
denote the portion of  lying on some particular side of
lying on some particular side of .
.
Suppose that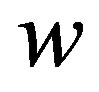 is of class
is of class 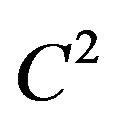 in the closure of
in the closure of  and satisfies the elliptic inequality
and satisfies the elliptic inequality
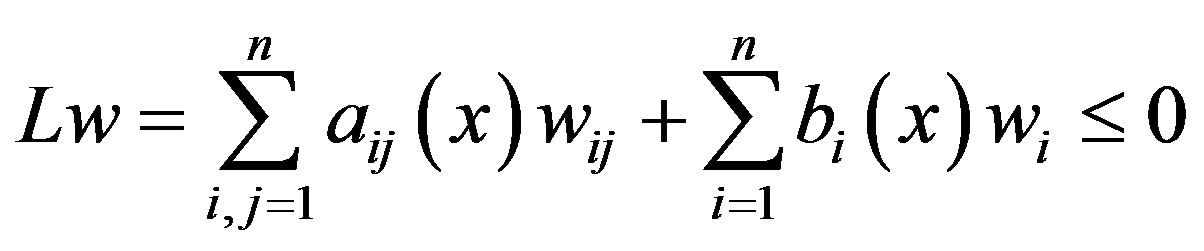 ,
, 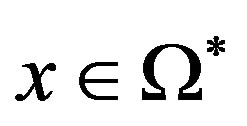
where the coefficients are uniformly bounded. We assume that the matrix 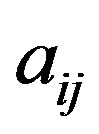 is uniformly definite
is uniformly definite
 ,
, 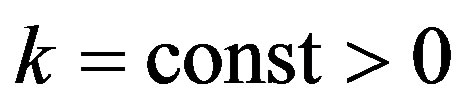 and that
and that
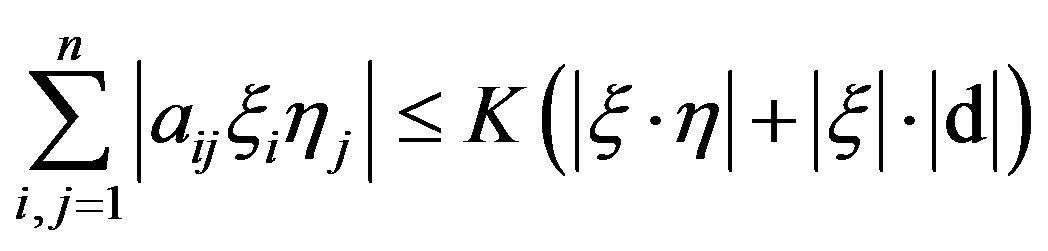 ,
, 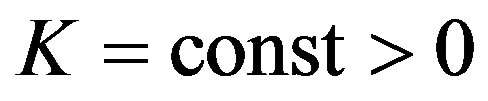 where
where  is an arbitrary real vector,
is an arbitrary real vector, 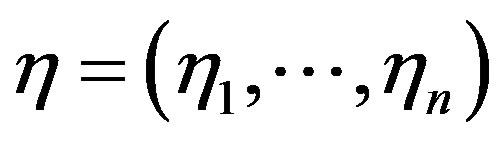 is the unit normal to the plane
is the unit normal to the plane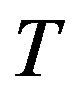 , and
, and 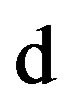 is the distance from
is the distance from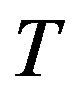 . Suppose also
. Suppose also  in
in  and
and 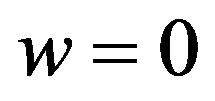 at
at . Let
. Let 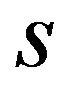 be any direction at
be any direction at  which enters
which enters  nontangentially. Then
nontangentially. Then
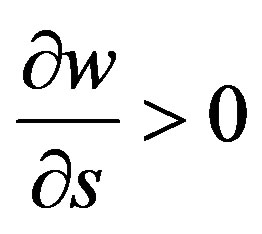 or
or 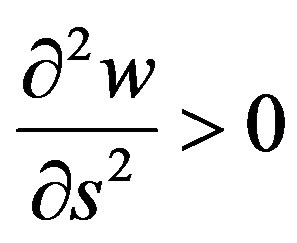 at
at unless
unless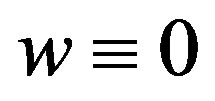 .
.
Our main results are as follows:
Theorem 2.2. Let  be a bounded connected open subset of
be a bounded connected open subset of 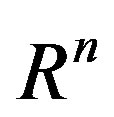 with
with 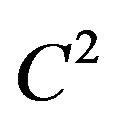 boundary and let
boundary and let 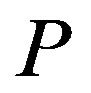 be a point in
be a point in
 . Let
. Let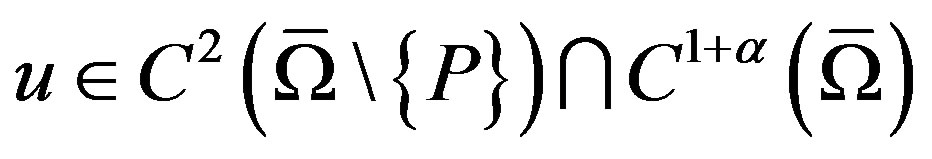 ,
, 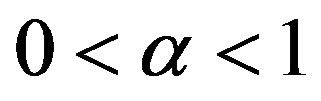 , be a strictly positive solution of the following overdetermined boundary value problem
, be a strictly positive solution of the following overdetermined boundary value problem
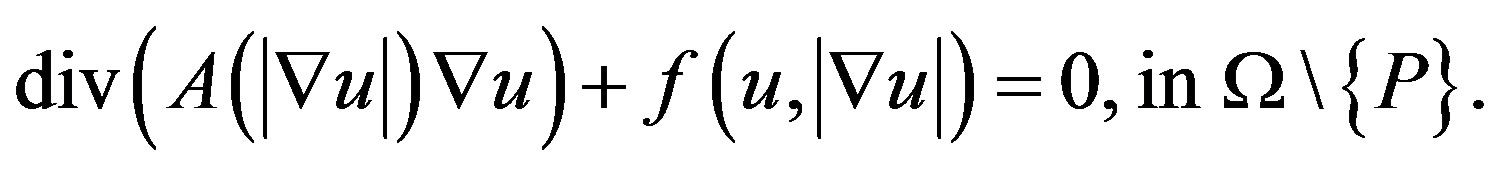 (2.1)
(2.1)
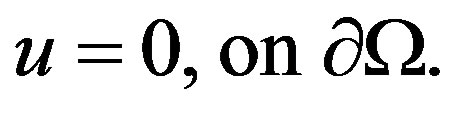 (2.2)
(2.2)
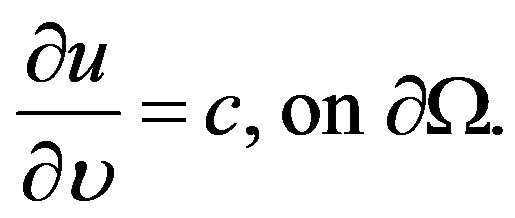 (2.3)
(2.3)
Here 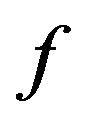 is a continuously differentiable function,
is a continuously differentiable function, 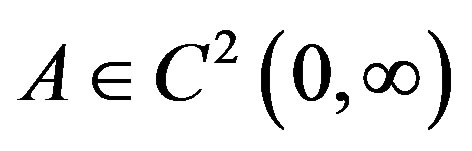 and
and
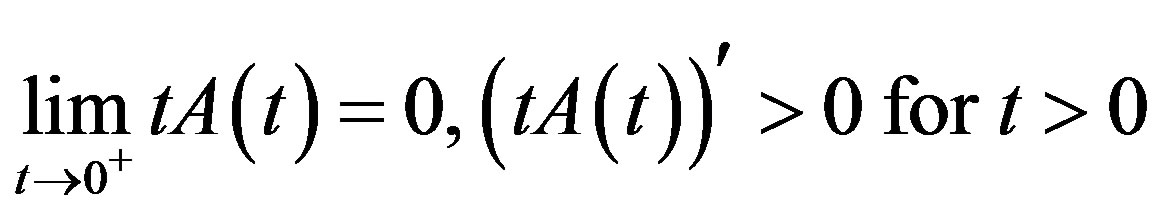 . (2.4)
. (2.4)
c is a constant and 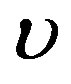 denotes the inner normal to
denotes the inner normal to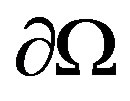 . Assume
. Assume
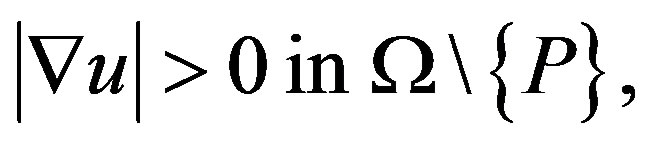 (2.5)
(2.5)
then  is a ball and
is a ball and  is radially symmetric.
is radially symmetric.
The following remark is a general version of the theorem. It can be viewed as an extension result of p-Laplacian too. As the proof is similar to Theorem 2.2, we omit it.
Remark 2.3. Let  be as in Theorem 2.2 and D be a subset of
be as in Theorem 2.2 and D be a subset of . Let
. Let 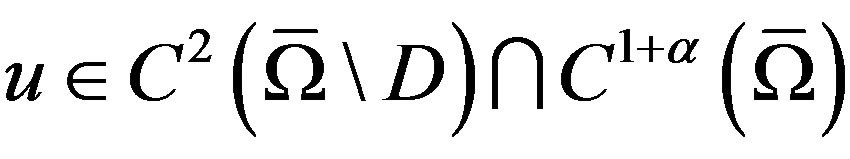 be a strictly positive solution of Equation (2.1) in
be a strictly positive solution of Equation (2.1) in 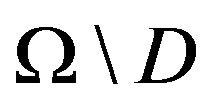 and verify the boundary conditions; Assume that
and verify the boundary conditions; Assume that 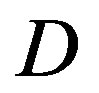 is the critical set of
is the critical set of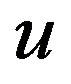 , then if
, then if 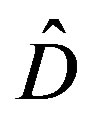 denotes the convex hull of
denotes the convex hull of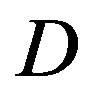 1) the normal line to
1) the normal line to 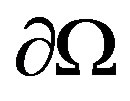 at an arbitrary point of
at an arbitrary point of  intersects
intersects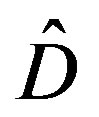 ;
;
2) if  is a support plane to
is a support plane to 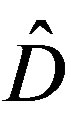 through
through 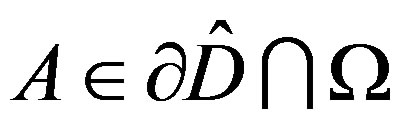 and
and 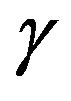 is a ray from A orthogonal to
is a ray from A orthogonal to  which lies in the half-space determined by
which lies in the half-space determined by  not containing
not containing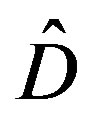 , then
, then 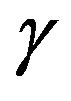 intersects
intersects 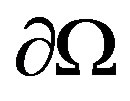 exactly in one point.
exactly in one point.
In what follows we assume that the origin  of the coordinates system is an interior point of
of the coordinates system is an interior point of , and we denote with
, and we denote with 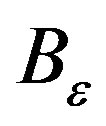 the closure of the ball centered in
the closure of the ball centered in  with radius
with radius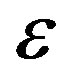 .
.
Theorem 2.4. Assume that the hypotheses of Theorem 2.2 hold and furthermore assume that
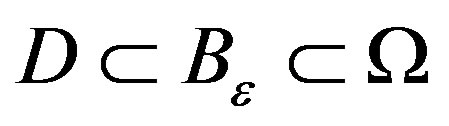
for some positive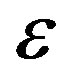 . Then 1)
. Then 1)  is starshaped with respect to
is starshaped with respect to ;
;
2) if
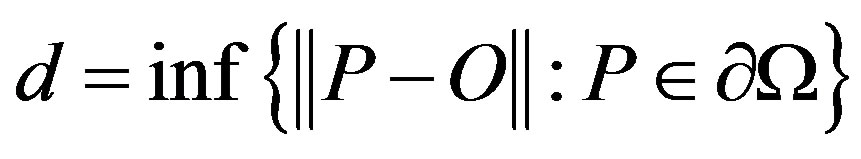 ;
;
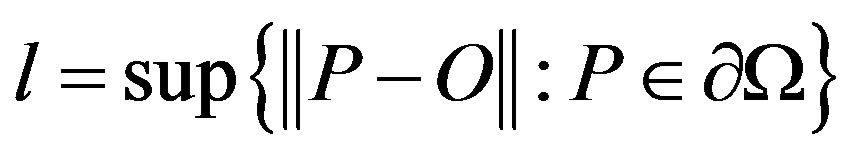 ;
;
then
 .
.
3. Proof of Theorem 2.1
The technique we are going to use is the moving planes method. For the detailed description about moving planes method, see [1].
Proof. Step 1: To prove  is a ball.
is a ball.
If we can demonstrate that for any point Q on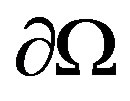 , P lies on the normal line to
, P lies on the normal line to 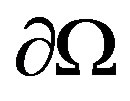 at Q, then
at Q, then  is a ball with centre P. To do this, we argue by contradiction.
is a ball with centre P. To do this, we argue by contradiction.
Assume that there exists a point 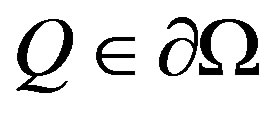 such that the normal line
such that the normal line 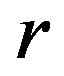 to
to 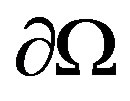 at Q does not contain P. We choose a coordinate system in
at Q does not contain P. We choose a coordinate system in 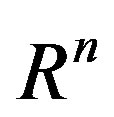 such that
such that 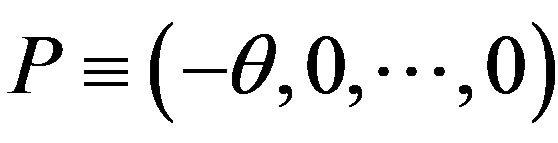 ,
, 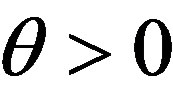 , and the xn axis coincides with r.
, and the xn axis coincides with r.
When we use the moving planes method, we choose a family of hyperplanes normal to the 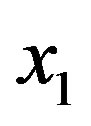 axis. Define hyperplan
axis. Define hyperplan 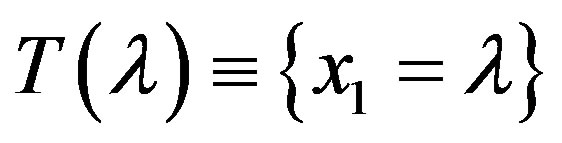 for any positive
for any positive ; Let
; Let 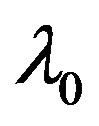 be the infimum of
be the infimum of 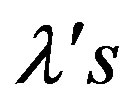 such that
such that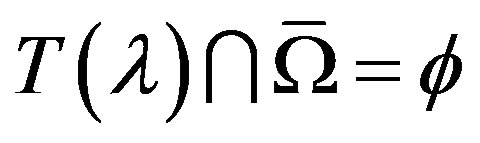 ; Define
; Define 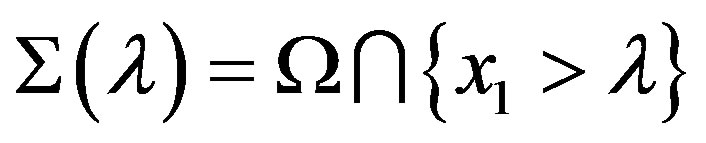 for
for 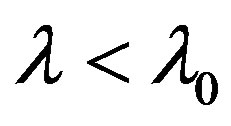 and we denote by
and we denote by 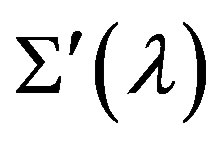 the reflection
the reflection 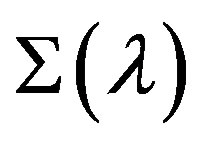 in
in . Since
. Since 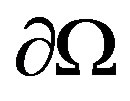 is
is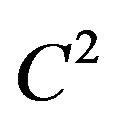 , for some
, for some  close to
close to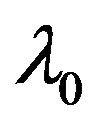 , v, we have
, v, we have
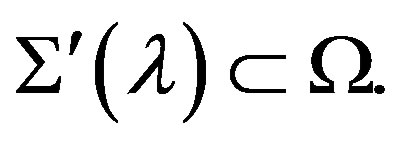 (3.1)
(3.1)
As  decreases, condition (3.1) holds until one of the following facts happens:
decreases, condition (3.1) holds until one of the following facts happens:
1) 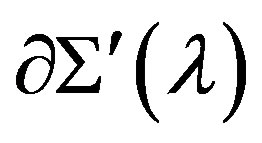 is internally tangent to
is internally tangent to 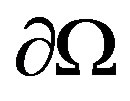 at some point of
at some point of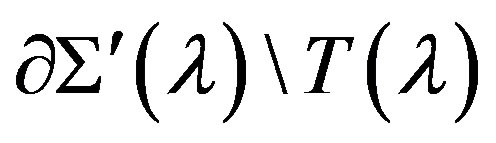 ;
;
2)  intersects
intersects 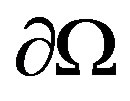 at some point of
at some point of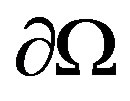 .
.
Let 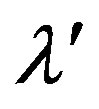 be the greatest value of
be the greatest value of ,
, 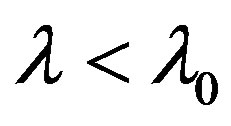 , such that either condition a) or b) is true. Since
, such that either condition a) or b) is true. Since 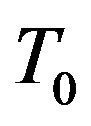 is orthogonal to
is orthogonal to 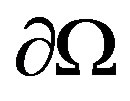 at
at , we have
, we have 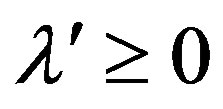 and then
and then 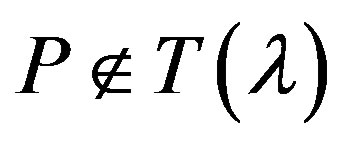 for any
for any  in
in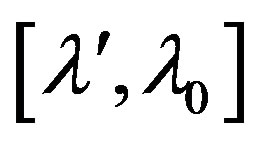 . This is the crucial point of our proof. We have found a direction such that as the moving plane
. This is the crucial point of our proof. We have found a direction such that as the moving plane 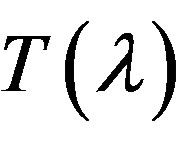 moves from
moves from 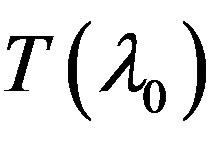 to the critical position
to the critical position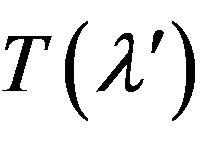 , it never intersects
, it never intersects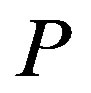 , so that the moving planes method may be applied.
, so that the moving planes method may be applied.
Let 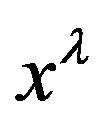 be the reflected point of x in
be the reflected point of x in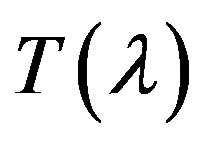 . We defined
. We defined
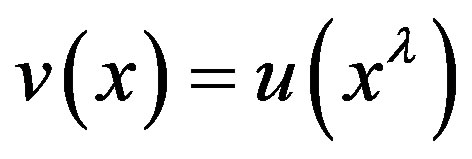 for
for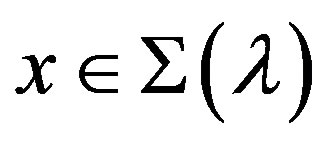 ,
, 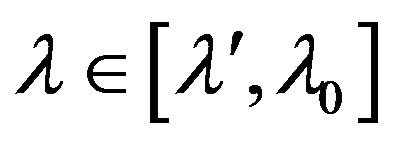 ,
,
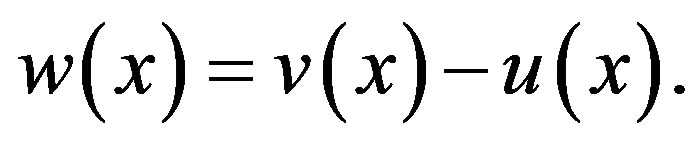
From Equation (2.1) we have for ,
,
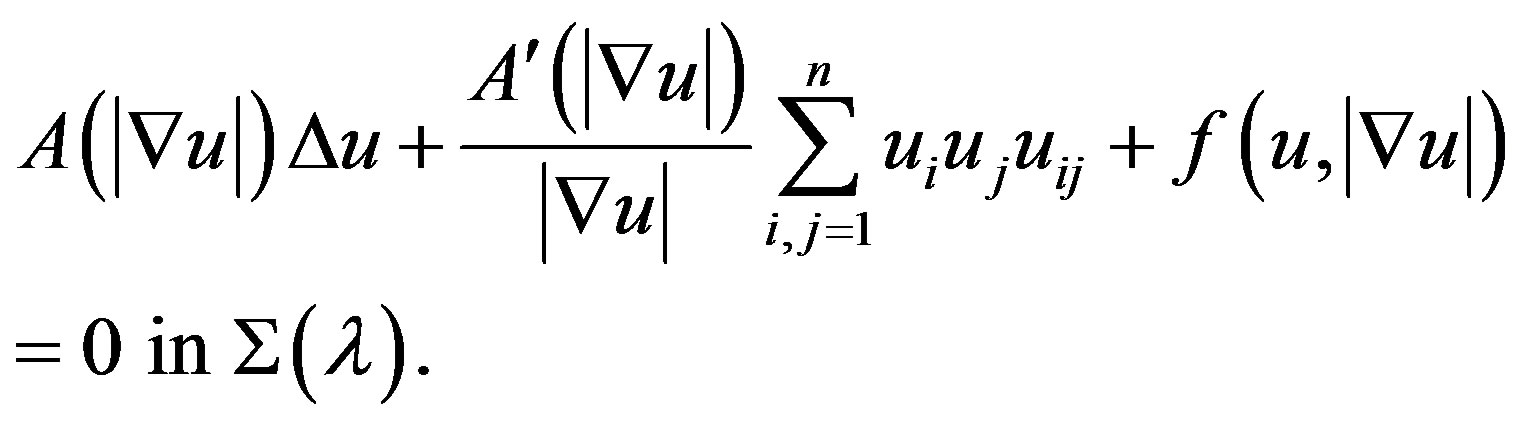 (3.2)
(3.2)
By the definition of v, we obtain
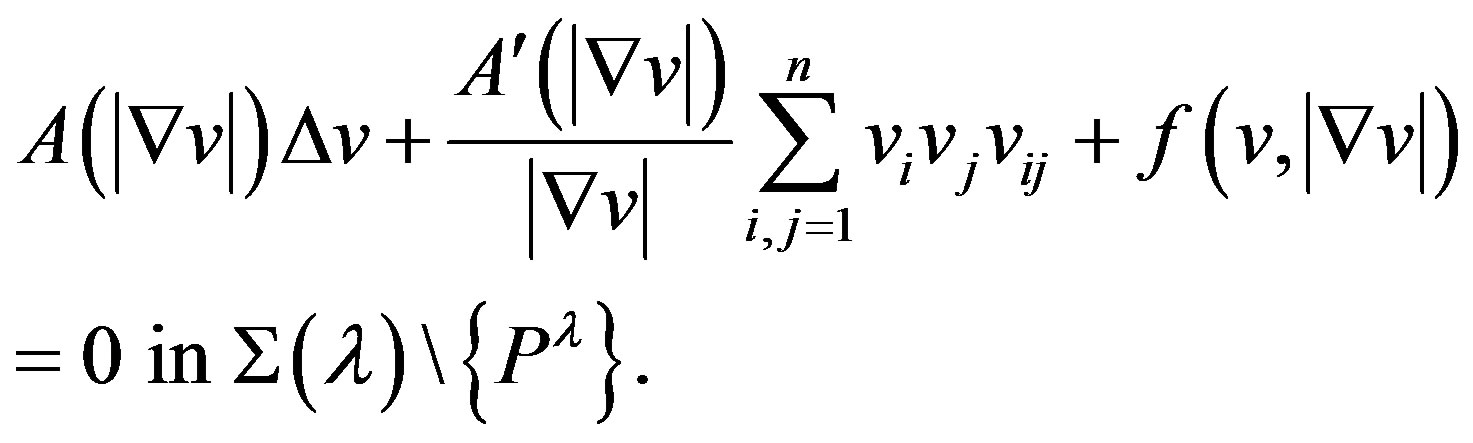 (3.3)
(3.3)
Differencing Equations (3.2) and (3.3) yields
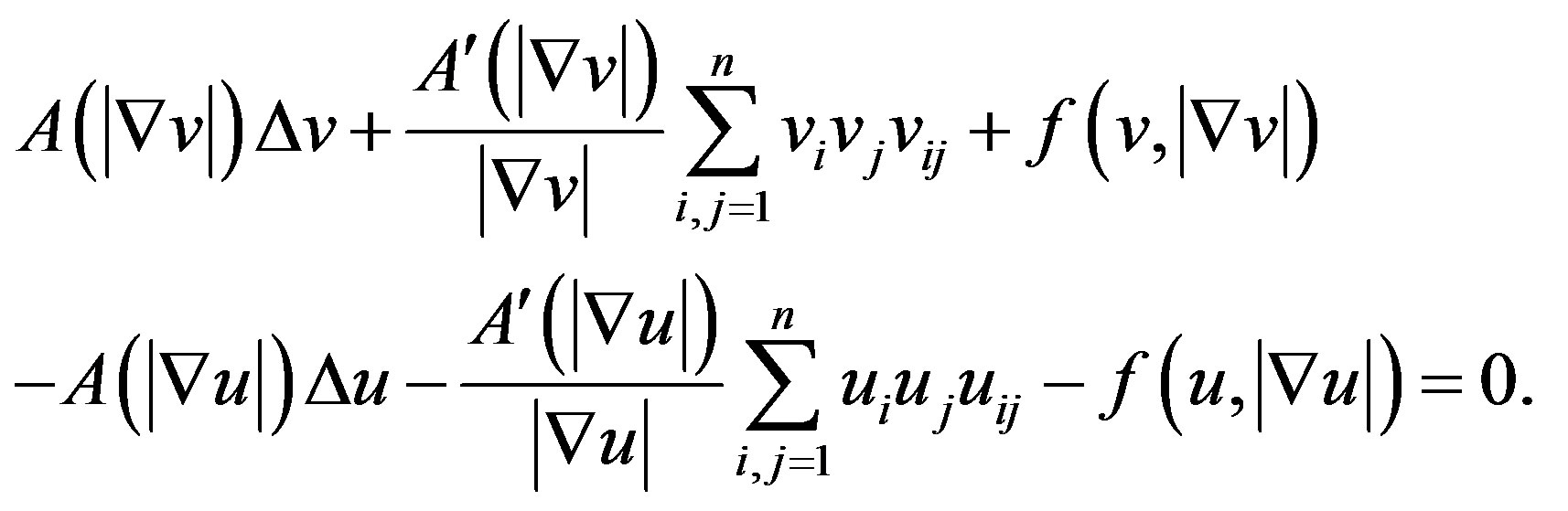 (3.4)
(3.4)
Meanwhile, (3.4) can also be rewritten into
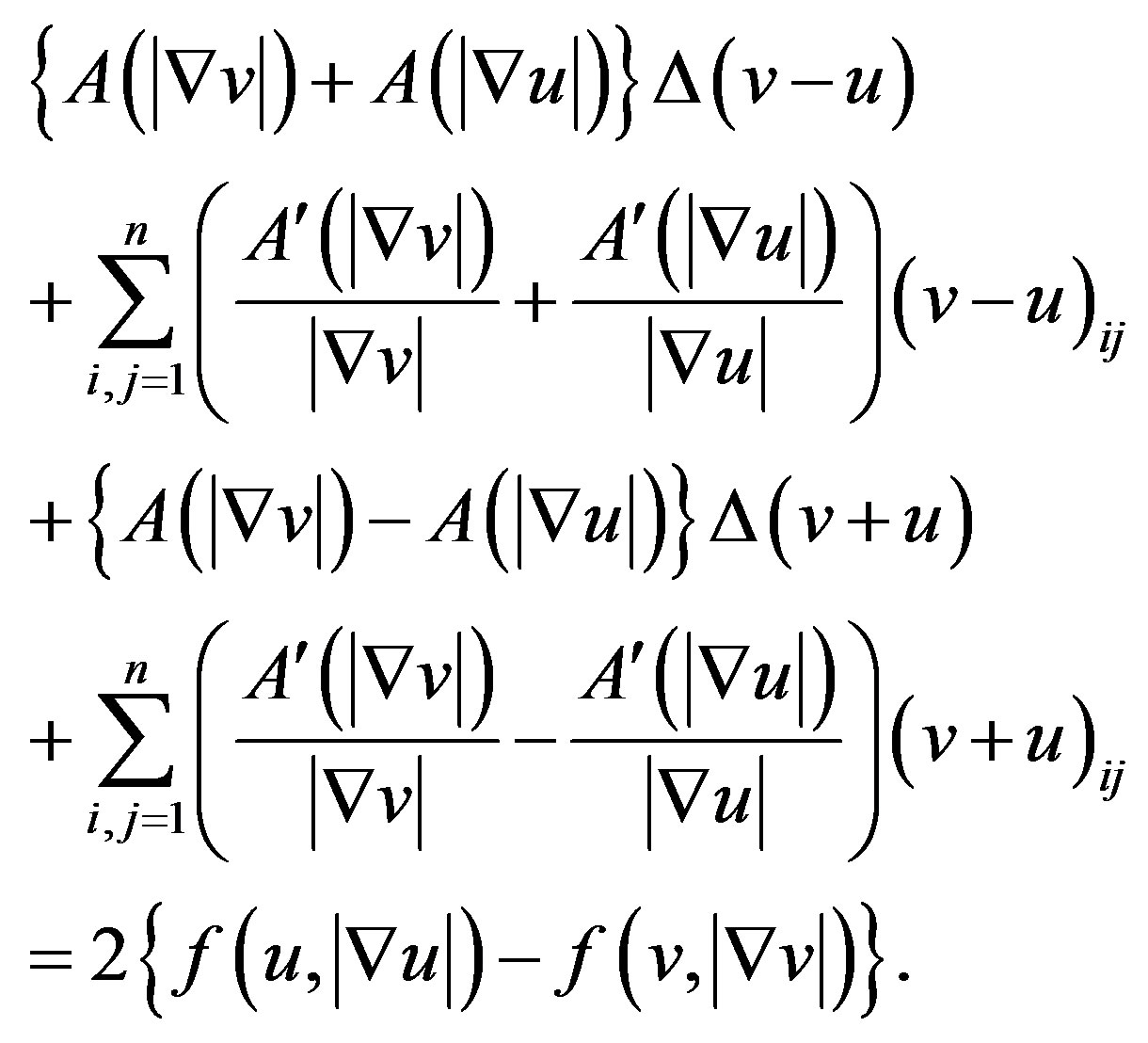 (3.5)
(3.5)
Denote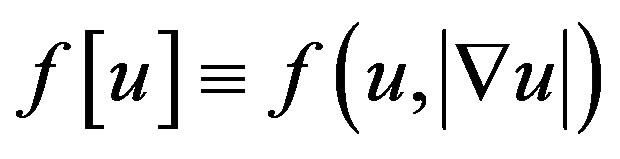 ,
, 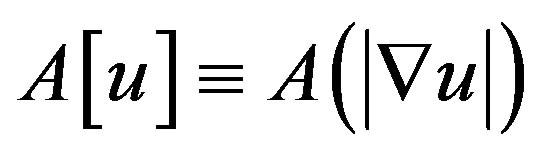 ,
,
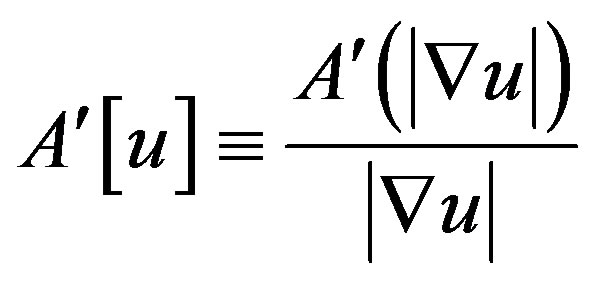 .
.
Let

By the mean value theorem, it follows from (3.5) that
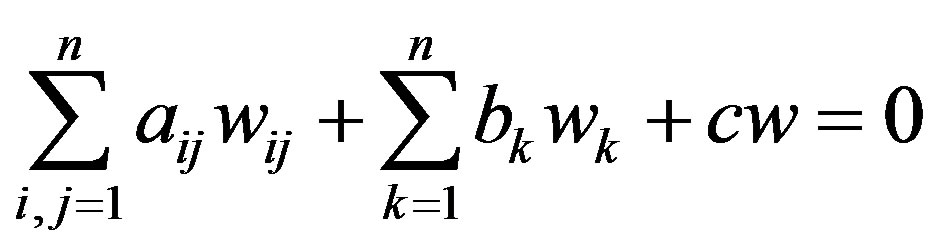 (3.6)
(3.6)
where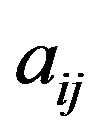 ,
, 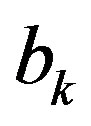 and c are certain functions depending on u and f. Here the matrix
and c are certain functions depending on u and f. Here the matrix 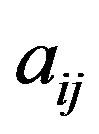 is uniformly positive definite, since both expressions
is uniformly positive definite, since both expressions 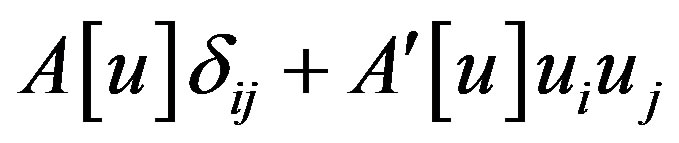 and
and 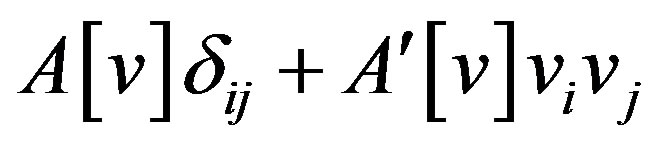 have this property (recall that Equation (2.1) is elliptic). So (3.6) is uniformly elliptic with bounded coefficients far from
have this property (recall that Equation (2.1) is elliptic). So (3.6) is uniformly elliptic with bounded coefficients far from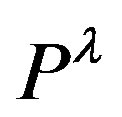 , i.e. in
, i.e. in 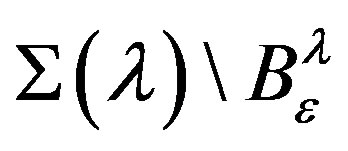 where
where 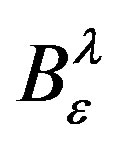 is a ball centered in with radius
is a ball centered in with radius , for any positive
, for any positive .
.
From the boundary condition (2.3) on the normal derivative of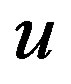 , it follows that
, it follows that
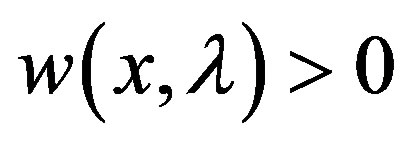 in
in 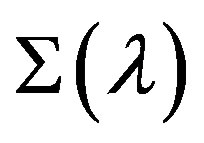 (3.7)
(3.7)
for some 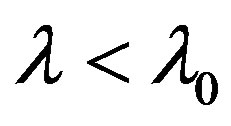 sufficiently close to
sufficiently close to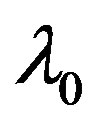 . Let
. Let
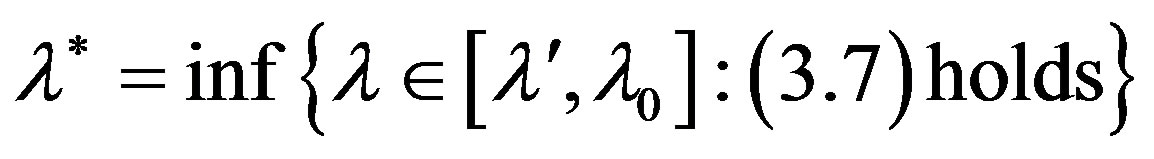 . We prove
. We prove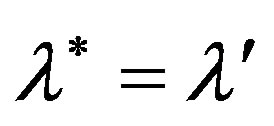 .
.
Assume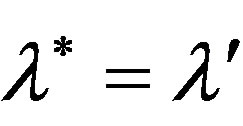 , by continuity,
, by continuity, 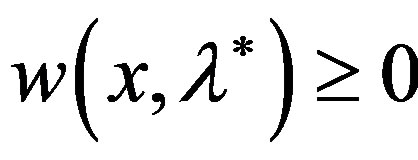 in
in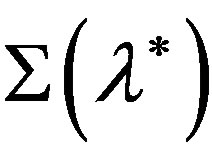 . On the other hand, since
. On the other hand, since  is not symmetric with respect to
is not symmetric with respect to ,
, 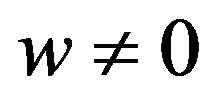 in
in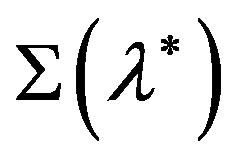 . By the strong version of the maximum principle, we obtain
. By the strong version of the maximum principle, we obtain 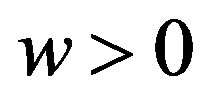 in
in
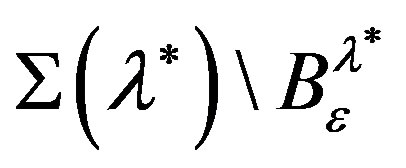 . Next we observe that
. Next we observe that 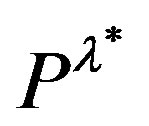 can not be a critical point for w since
can not be a critical point for w since 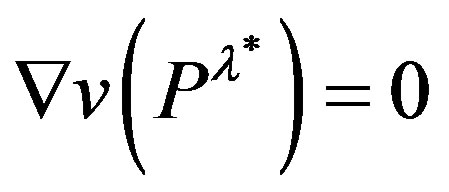 while
while
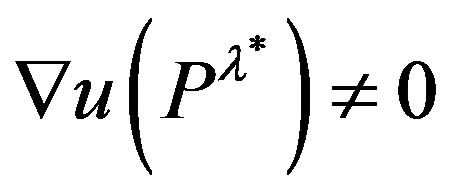 . So as
. So as 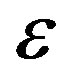 is arbitrarily small, it is
is arbitrarily small, it is 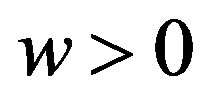
in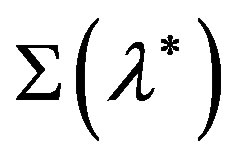 . Since
. Since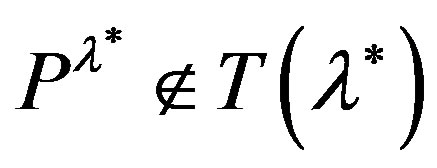 , we may apply the Hopf lemma to
, we may apply the Hopf lemma to 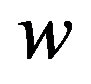 at each point of
at each point of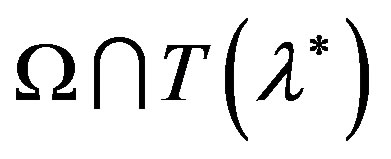 , we get
, we get
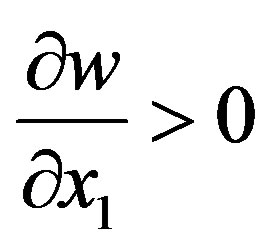 on
on 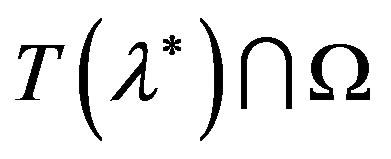 (3.8)
(3.8)
The plane 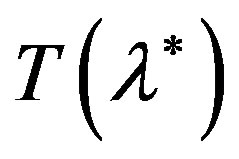 is not normal to
is not normal to 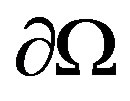 at any point, then from inequality (3.8) and the boundary condition (2.3) on the normal derivative of
at any point, then from inequality (3.8) and the boundary condition (2.3) on the normal derivative of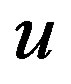 , we get
, we get
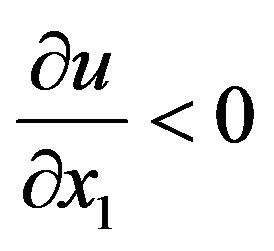 (3.9)
(3.9)
By the definition of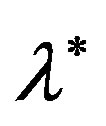 , there exists a sequence
, there exists a sequence 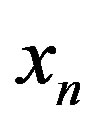 such that
such that 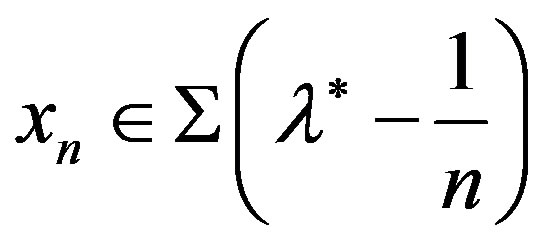 and
and
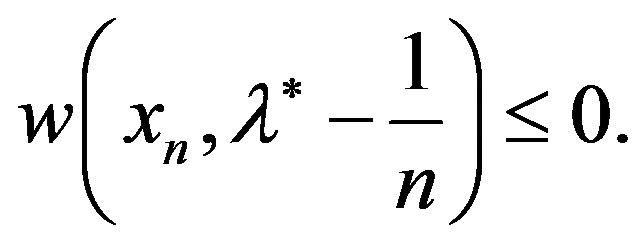 (3.10)
(3.10)
Let 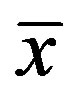 be a limit point for xn in the closure of
be a limit point for xn in the closure of by continuity
by continuity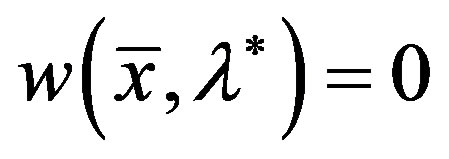 , thus
, thus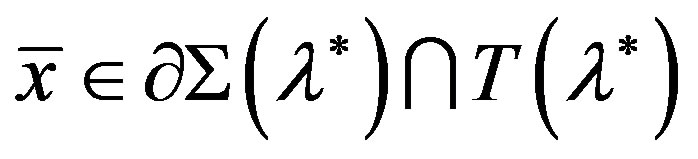 . But from inequality (3.10) and the mean value theorem we get
. But from inequality (3.10) and the mean value theorem we get 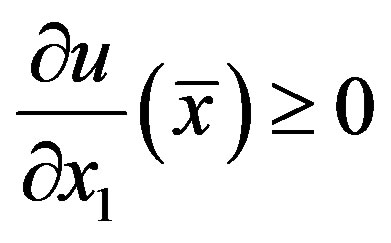 and this contradicts condition (3.9).
and this contradicts condition (3.9).
So 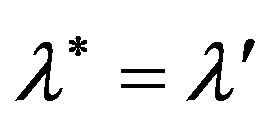 is proved.
is proved.
Now we will prove that u must be symmetric with respect to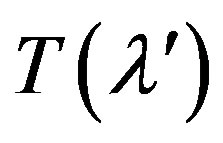 . Assume
. Assume 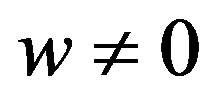 in
in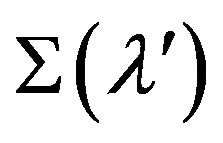 , so as we did for
, so as we did for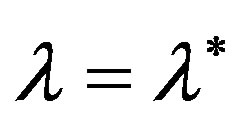 , we infer
, we infer 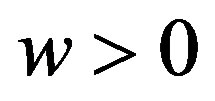 in
in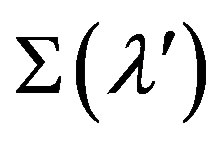 .
.
Assume next that condition a) holds, then 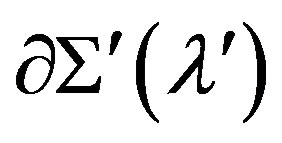 is internally tangent to
is internally tangent to 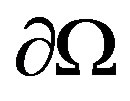 at some point
at some point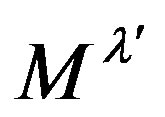 , where
, where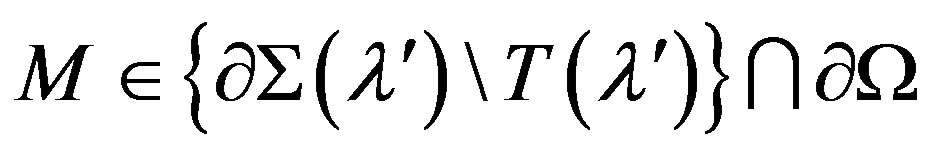 . Since P is an interior point of
. Since P is an interior point of ,
, 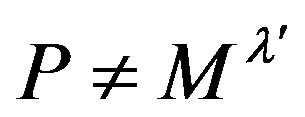 , so that we can apply the Hopf lemma to w at M and we obtain
, so that we can apply the Hopf lemma to w at M and we obtain
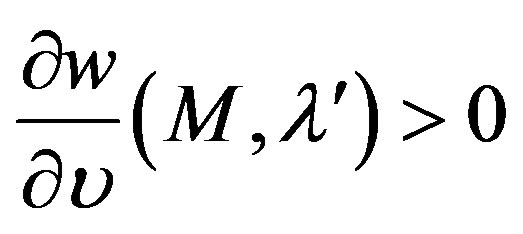 ;
;
where 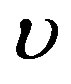 is the inner normal to
is the inner normal to 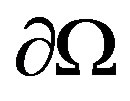 at M. For
at M. For
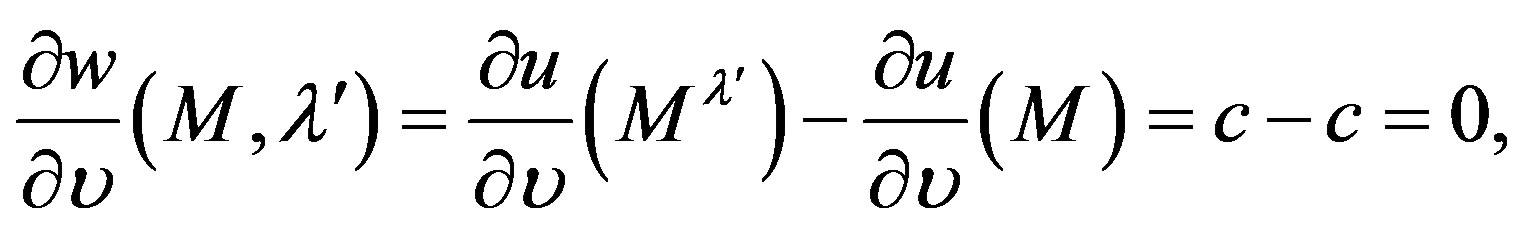
we get the contradiction. Hence condition 2) must be true, i.e. 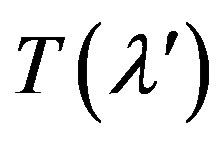 is orthogonal to
is orthogonal to 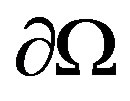 at some point B. From the boundary condition (2.3) and the definition of w it follows that all the first and second derivatives of w vanish at B. On the other hand, as
at some point B. From the boundary condition (2.3) and the definition of w it follows that all the first and second derivatives of w vanish at B. On the other hand, as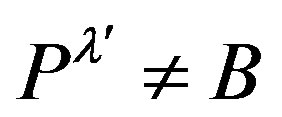 , Equation (3.6) is uniformly elliptic with bounded coefficents in a neighborhood of B, so that the boundary lemma at corner in [1] lemma 2, may be applied to w. Let s be a direction which enters
, Equation (3.6) is uniformly elliptic with bounded coefficents in a neighborhood of B, so that the boundary lemma at corner in [1] lemma 2, may be applied to w. Let s be a direction which enters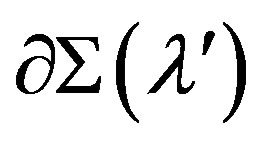 nontangentially at B, then by the Serrin’s lemma
nontangentially at B, then by the Serrin’s lemma
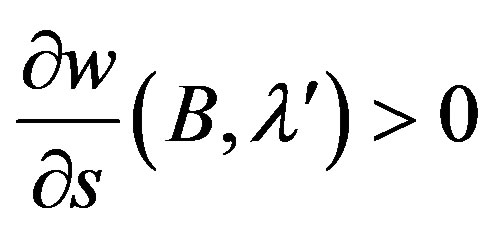 or
or 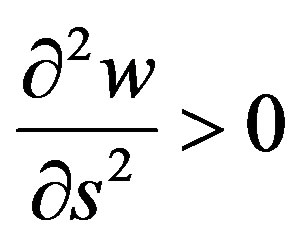
Then we have again a contradiction with the derivatives of w at B, so 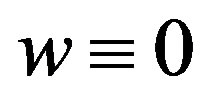 in
in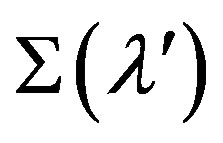 . But this last inequality can not be true since otherwise w would be a function symmetric in
. But this last inequality can not be true since otherwise w would be a function symmetric in 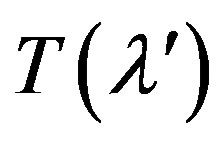 whose only critical point is not on
whose only critical point is not on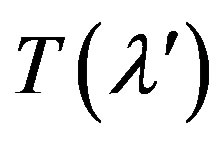 .
.
This completes the proof of Theorem 2.1.
REFERENCES
- J. Serrin, “A Symmetry Problem in Potential Theory,” Archive for Rational Mechanics and Analysis, Vol. 43, No. 4, 1971, pp. 304-318. doi:10.1007/BF00250468
- N. Garofalo and J. Lewis, “A Symmetry Result Related to Some Overdetermined Boundary Value Problems,” American Journal of Mathematics, Vol. 111, No. 1, 1989, pp. 9-33. doi:10.2307/2374477
- I. Fragala, I. F. Gazzaola and B. Kawohl, “Overdetemined Boundary Value Problems with Possibly Degenerate Ellipticity: A Geometry Approach,” Mathematische Zeitschrift, Vol. 254, No. 1, 2006, pp. 117-132. doi:10.1007/s00209-006-0937-7
- G. A. Philippin, “Application of the Maximum Principle to a Variety of Problems Involving Elliptic Differential Equations,” In: P. W. Schaefer, Ed., Maximum Principles and Eigenvalue Problems in Partial Differential Equations, Pitman Research Notes in Mathematics Series, Longman SciTech., Harlow, 1988, pp. 34-48.
- A. Farina and B. Kawohl, “Remarks on an Overdetermined Boundary Value Problem,” Calculus of Variations and Partial Differential Equations, Vol. 31, No. 3, 2008, pp. 351-357.
- A. Firenze, “A Symmetry Result for the p-Laplacian Equation via the Moving Planes Method,” Applocable. Analysis, Vol. 55, No. 3-4, 1994, pp. 207-213.
NOTES
*This work is supported by the Natural Science Foundation of Shandong Province of China (ZR2012AM018).

