World Journal of Mechanics
Vol.4 No.5(2014), Article ID:46259,11 pages
DOI:10.4236/wjm.2014.45014
A Series Solution for Three-Dimensional Navier-Stokes Equations of Flow near an Infinite Rotating Disk
Hamed Shahmohamadi*, Mahdi Mohammadpour
Wolfson School of Mechanical and Manufacturing Engineering, Loughborough University, Loughborough, UK
Email: *h.shahmohamadi@lboro.ac.uk
Copyright © 2014 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


Received 22 January 2014; revised 25 February 2014; accepted 21 March 2014
ABSTRACT
In this paper, homotopy analysis method (HAM) and Padé approximant will be considered for finding analytical solution of three-dimensional viscous flow near an infinite rotating disk which is a well-known classical problem in fluid mechanics. The solution is compared to the numerical (fourth-order Runge-Kutta) solution and the convergence of the obtained series solution is carefully analyzed. The results illustrate that HAM-Padé is an appropriate method in solving the systems of nonlinear equations.
Keywords:Homotopy Analysis Method, Padé Approximant, Navier-Stokes Equations, Rotating Disk

1. Introduction
Von Karman swirling viscous flow [1] is a well-known classical problem in fluid mechanics. The original problem raised by Von Karman is about the viscous flow induced by an infinite rotating disk where the fluid, far from the disk, is at rest. Then the problem is generalized in considering the case where the fluid itself is rotating as a solid body far from the disk with suction or injection at the disk surface [2] . This generates a parameter, i.e. the ratio of the angular velocity of the fluid at infinity to the angular velocity of the disk. Another generalization is to consider the viscous flow between two infinite coaxial rotating disks with suction or injection at both disks and this reveals another parameter, i.e. the Reynolds number determined by the distance of the two disks.
Nonlinear phenomena play a crucial role in applied mathematics and physics. It is
known that most of engineering problems are nonlinear and solving them analytically
is very difficult. Various powerful mathematical methods such as inverse scattering
method [3] , tanh method [4] , sine-cosine method [5] , homogeneous balance method
[6] , Exp-function method [7] and variational methods [8] -[12] have been proposed
for obtaining exact and approximate analytic solutions. Recently, two analytical
techniques have drawn specific attention, namely homotopy analysis method (HAM)
and homotopy perturbation method (HPM). The essential idea in these methods is introducing
a homotopy parameter, like , which takes the
value from 0 to 1. For
, which takes the
value from 0 to 1. For , the system of equations takes
a simplified form, which readily admits a particularly simple solution. When
, the system of equations takes
a simplified form, which readily admits a particularly simple solution. When
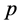 is gradually being increased to 1, the system goes through a sequence of “deformations”,
the solution of each of which is “close” to that at the previous stage of “deformation”.
Eventually at
is gradually being increased to 1, the system goes through a sequence of “deformations”,
the solution of each of which is “close” to that at the previous stage of “deformation”.
Eventually at
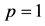 the system takes the original form of equation and the final stage of “deformation”
gives the desired solution. In 1992, Liao [13] [14] has been the leading exponent
of homotopy analysis method (HAM). In HAM, Liao keeps sufficient room for experimenting
the convergence of approximations by introducing auxiliary parameter and also, an
auxiliary non-zero function. Another way of viewing homotopy approach is to think
of
the system takes the original form of equation and the final stage of “deformation”
gives the desired solution. In 1992, Liao [13] [14] has been the leading exponent
of homotopy analysis method (HAM). In HAM, Liao keeps sufficient room for experimenting
the convergence of approximations by introducing auxiliary parameter and also, an
auxiliary non-zero function. Another way of viewing homotopy approach is to think
of
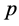 as a perturbation parameter. In 1998, He [15] has transformed this idea into homotopy
perturbation method (HPM). The approximate solution given by HAM is the same as
the approximate solution given by HPM, when auxiliary parameter and auxiliary non-zero
function are taken −1 and 1, respectively [16] -[19] . So, the approximate
analytical solution obtained by HAM contains the solution obtained by HPM. However,
the implementation of these methods shows that HAM is more effective than HPM. The
convergence of HAM solution series depends on four factors, i.e. initial guess,
auxiliary linear operator, auxiliary non-zero function and auxiliary parameter
as a perturbation parameter. In 1998, He [15] has transformed this idea into homotopy
perturbation method (HPM). The approximate solution given by HAM is the same as
the approximate solution given by HPM, when auxiliary parameter and auxiliary non-zero
function are taken −1 and 1, respectively [16] -[19] . So, the approximate
analytical solution obtained by HAM contains the solution obtained by HPM. However,
the implementation of these methods shows that HAM is more effective than HPM. The
convergence of HAM solution series depends on four factors, i.e. initial guess,
auxiliary linear operator, auxiliary non-zero function and auxiliary parameter
 However, as a special case of homotopy analysis method by having
However, as a special case of homotopy analysis method by having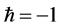 , the convergence
of HPM solution series only depends on two factors: the auxiliary linear operator
and the initial guess. Therefore, having the initial guess and the auxiliary linear
operator, HPM cannot provide other ways to ensure that the solution is convergent.
Note that the HAM has already been applied to the analytical solution of several
other problems [20] -[22] .
, the convergence
of HPM solution series only depends on two factors: the auxiliary linear operator
and the initial guess. Therefore, having the initial guess and the auxiliary linear
operator, HPM cannot provide other ways to ensure that the solution is convergent.
Note that the HAM has already been applied to the analytical solution of several
other problems [20] -[22] .
Ismail and Rabboh [23] presented a restrictive Padé approximation for the generalized Fisher and Burger-Fisher equations. The Padé approximants that often show superior performance over series approximations provide a successful tool and promising scheme for identical applications.
The purpose of this paper is to extend homotopy analysis method and Padé approximant to solve three-dimensional Navier-Stokes equations for the viscous flow near an infinite rotating disk.
2. Flow Analysis and Mathematical Formulation
The Navier-Stokes equations for cylindrical coordinate are as follows:
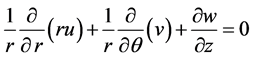 (1)
(1)
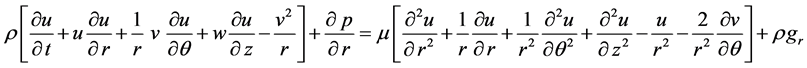 (2)
(2)
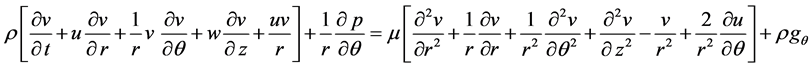 (3)
(3)
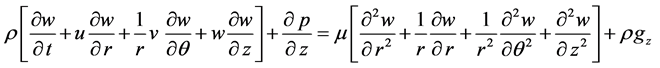 (4)
(4)
where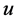 ,
,
 and
and
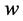 are velocities in the
are velocities in the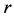 ,
,
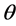 and
and
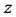 directions; respectively.
directions; respectively.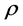 ,
,
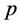 and
and
 are density, pressure and viscosity. Consider the steady flow which results if the
infinite plane
are density, pressure and viscosity. Consider the steady flow which results if the
infinite plane
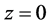 rotates at constant angular velocity
rotates at constant angular velocity
 about the axis
about the axis
 beneath a Newtonian viscous fluid which otherwise be at rest. The viscous drag of
the rotating surface would set up a swirling flow toward the disk. All three velocity
components
beneath a Newtonian viscous fluid which otherwise be at rest. The viscous drag of
the rotating surface would set up a swirling flow toward the disk. All three velocity
components ,
,
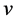 and
and
 would be involved in a genuine three-dimensional motion, but because of radial symmetry
they will be independent of
would be involved in a genuine three-dimensional motion, but because of radial symmetry
they will be independent of
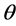 as would the pressure
as would the pressure , so it is required
to solve the equation for these four variables as functions of
, so it is required
to solve the equation for these four variables as functions of
 and
and
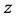 from the continuity equation and the momentum equations in the
from the continuity equation and the momentum equations in the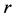 ,
,
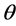 and
and
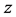 directions we obtain
directions we obtain
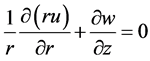 (5)
(5)
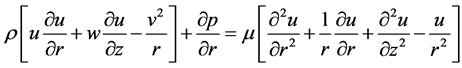 (6)
(6)
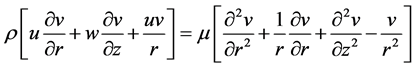 (7)
(7)
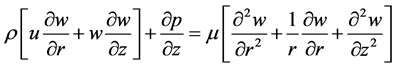 (8)
(8)
The boundary conditions are no-slip at the disk wall and inviscid flow far from the disk (except an axial inflow):
 (9)
(9)
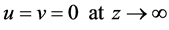 (10)
(10)
Note that we refrain from imposing any conditions on
 as
as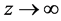 , because we expect (by hindsight, to be sure)
that the disk will have a centrifugal pumping action which must be balanced by a
uniform axial inflow
, because we expect (by hindsight, to be sure)
that the disk will have a centrifugal pumping action which must be balanced by a
uniform axial inflow far from the disk. Also the condition
far from the disk. Also the condition
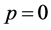 (a convenient constant) at the wall is by hindsight. It has been deduced that
(a convenient constant) at the wall is by hindsight. It has been deduced that
 and
and
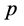 are all functions of
are all functions of . Since the only
two parameters in the problem are
. Since the only
two parameters in the problem are
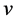 and
and , it is easy to see that the proper dimensionless
variable must be
, it is easy to see that the proper dimensionless
variable must be
 Regarding Karman (1921), we propose the new dimensionless variables
Regarding Karman (1921), we propose the new dimensionless variables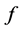 ,
,
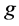 ,
,
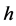 and
and
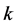 as
as
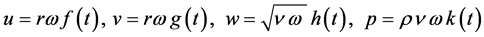 (11)
(11)
These variables are substituted into Equations (5)-(8) and the following set of nonlinear ordinary coupled differential equations is obtained:
 (12)
(12)
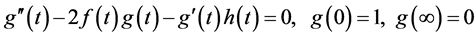 (13)
(13)
 (14)
(14)
 (15)
(15)
3. Basic Concepts of HAM
Consider the following differential equation
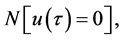 (16)
(16)
where
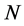 is a nonlinear operator,
is a nonlinear operator,
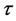 denotes an independent variable,
denotes an independent variable,
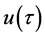 is an unknown function which is the solution
for the equation. The function is defined as
is an unknown function which is the solution
for the equation. The function is defined as
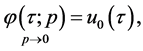 (17)
(17)
and
 (18)
(18)
where,
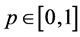 and
and
 are the initial guesses which satisfy the initial or boundary condition.
are the initial guesses which satisfy the initial or boundary condition.
By means of generalizing the traditional homotopy method, Liao [13] forms the so-called zero-order deformation equation as follows
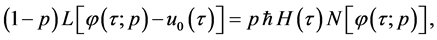 (19)
(19)
where
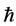 is the auxiliary parameter which increases the convergence of result ,
is the auxiliary parameter which increases the convergence of result ,
 is an auxiliary function and
is an auxiliary function and
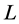 is an auxiliary linear operator,
is an auxiliary linear operator,
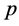 increases from 0 to 1, the solution
increases from 0 to 1, the solution
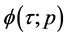 changes between the initial guess
changes between the initial guess
 and solution
and solution . Expanding
. Expanding
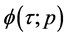 in Taylor series with respect to
in Taylor series with respect to , we obtain
, we obtain
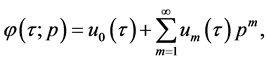 (20)
(20)
where
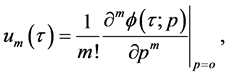 (21)
(21)
if the auxiliary linear operator, the initial guess, the auxiliary parameter
 and the auxiliary function are so properly chosen, the series (20) converges at
and the auxiliary function are so properly chosen, the series (20) converges at , so we have
, so we have
 (22)
(22)
which must be one of the solutions of the original nonlinear equation, as proved
by Liao [13] . It is clear that if the auxiliary parameter is , and auxiliary function
is determined to be
, and auxiliary function
is determined to be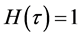 , Equation (19) would
be
, Equation (19) would
be
 (23)
(23)
This statement is commonly used in HPM procedure. Indeed, in HPM, the nonlinear
differential equation is solved by separating every Taylor expansion term. Now,
the vector of
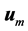 is defined as
is defined as
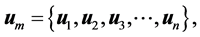 (24)
(24)
according to the definition Equation (21), the governing equation and the corresponding
initial condition of
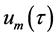 can be deduced from zero-order deformation Equation (19). Differentiating Equation
(19), m times with respect to the embedding parameter
can be deduced from zero-order deformation Equation (19). Differentiating Equation
(19), m times with respect to the embedding parameter
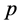 and setting
and setting
 and finally dividing by
and finally dividing by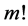 , we will have the socalled
mth order deformation equation in the following from
, we will have the socalled
mth order deformation equation in the following from
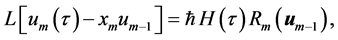 (25)
(25)
where
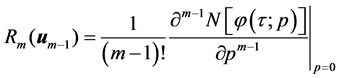 (26)
(26)
and
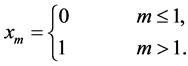 (27)
(27)
Therefore by applying inverse linear operator to both sides of the linear equation, Equation (25), we can easily solve the equation and compute the generation constant by applying the initial or boundary conditions.
4. HAM-Padé Solution
In this section the HAM is used to find approximate solutions of the Equations (12)-(15). We choose the initial approximation as
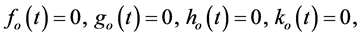 (28)
(28)
and the linear operator for Equations (12) to (15)
 (29)
(29)
 (30)
(30)
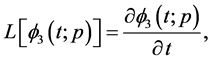 (31)
(31)
 (32)
(32)
We convert Equations (12) through (15) to nonlinear form:
 (33)
(33)
 (34)
(34)
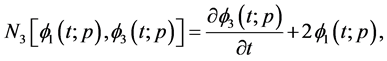 (35)
(35)
 (36)
(36)
Assuming
 we use the above definitions to form the zero-order deformation equations
we use the above definitions to form the zero-order deformation equations
 (37)
(37)
 (38)
(38)
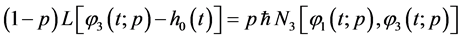 (39)
(39)
 (40)
(40)
Obviously, when
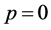 and
and ,
,
 (41)
(41)
 (42)
(42)
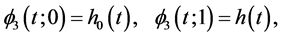 (43)
(43)
 (44)
(44)
Differentiating the zero-order deformation Equations (37)-(40) m times respecting
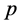 and finally dividing by
and finally dividing by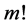 , we have the mth-order deformation
equations as follows
, we have the mth-order deformation
equations as follows
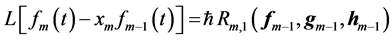 (45)
(45)
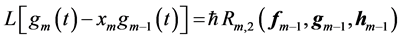 (46)
(46)
 (47)
(47)
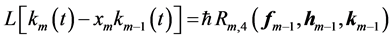 (48)
(48)
where
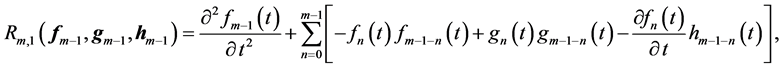 (49)
(49)
 (50)
(50)
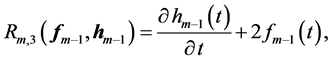 (51)
(51)
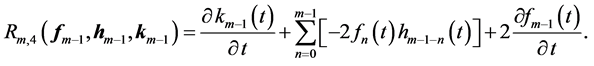 (52)
(52)
Using 10 terms in evaluating the approximate solution, and applying the padé approximation, we obtain
 (53)
(53)
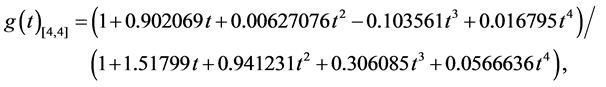 (54)
(54)
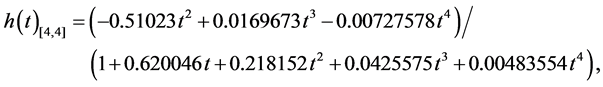 (55)
(55)
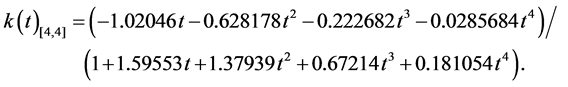 (56)
(56)
Noting that, the different from all other analytical techniques such as the perturbation
method, Adomian decomposition method, expansion method, and etc., the solutions
given by the HAM contain auxiliary parameters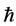 which could be used to control and adjust the convergence region and rate of the
HAM series.
which could be used to control and adjust the convergence region and rate of the
HAM series.
5. Convergence of HAM
It has been proven that, as long as a series solution given by the homotopy analysis
method is converging, it is certainly one of the exact solutions. The solution series
contains the auxiliary parameters
 and which provides us with a simple way to adjust and control the convergence of
the solution series. In general, by means of the so-called
and which provides us with a simple way to adjust and control the convergence of
the solution series. In general, by means of the so-called
 -curve, i.e., a curve of versus
-curve, i.e., a curve of versus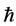 . As pointed
by Liao [13] , the valid region of
. As pointed
by Liao [13] , the valid region of
 is a horizontal line segment. To see the range of admissible values of these parameters,
the curves of
is a horizontal line segment. To see the range of admissible values of these parameters,
the curves of
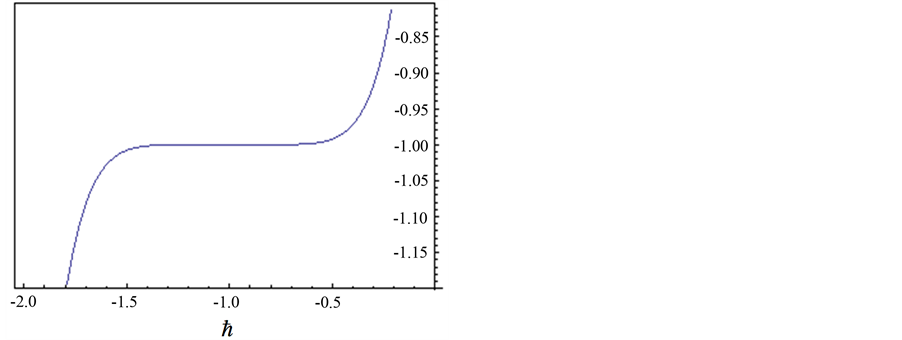
 are plotted in Figure 1 for
are plotted in Figure 1 for
 and
and
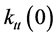 given by 10th-order approximation. For better presentation, these valid regions
are listed in Table 1. A wide valid zone is evident
in these figures ensuring convergence of the series. To choose optimal value of
auxiliary parameter, the averaged residual errors [24] are defined as
given by 10th-order approximation. For better presentation, these valid regions
are listed in Table 1. A wide valid zone is evident
in these figures ensuring convergence of the series. To choose optimal value of
auxiliary parameter, the averaged residual errors [24] are defined as
 (57)
(57)
 (58)
(58)
Table 1. The admissible
values of
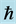 for each
for each .
.
 (a)
(a) (b)
(b)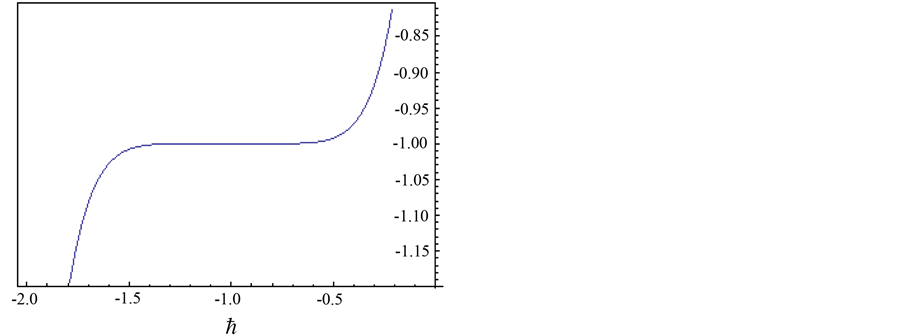 (c)
(c)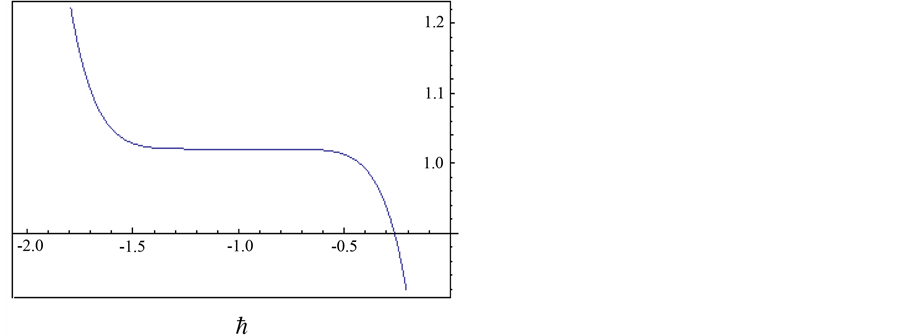 (d)
(d)
Figure 1.
 of (a)
of (a) ; (b)
; (b) ; (c)
; (c)
 and (d)
and (d)
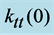 given by 10th order approximate solution.
given by 10th order approximate solution.
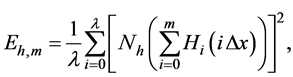 (59)
(59)
 (60)
(60)
where ∆x = 10/λ and λ = 20. For a given order of approximation m, the optimal
values of
 is given by the minimum of Em, corresponding to nonlinear algebraic equations
is given by the minimum of Em, corresponding to nonlinear algebraic equations
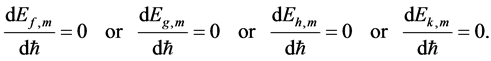 (61)
(61)
It is noticed that the optimal value of
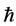 is replaced into the equations. Table 1 shows optimal
values obtained for the auxiliary parameters
is replaced into the equations. Table 1 shows optimal
values obtained for the auxiliary parameters
 . To see the accuracy of the solutions, the
residual errors for the system are defined as
. To see the accuracy of the solutions, the
residual errors for the system are defined as
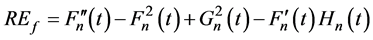 (62)
(62)
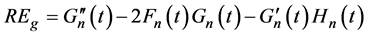 (63)
(63)
 (64)
(64)
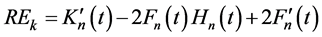 (65)
(65)
where Fn(t), Gn(t), Hn(t) and Kn(t) are the HAM solutions for f (t), g(t), h(t) and k(t).
6. Results and Discussion
HAM-Padé, in this paper was used to find approximate solutions for nonlinear equations
obtained by the steady, laminar, axially symmetric viscous flow near an infinite
rotating disk. The accuracy of the method is appropriate and obtained results are
close to the numerical solution. It is proper mentioning that the numerical results
have been obtained using the fourth-order Runge-Kutta method along shooting method
for satisfying boundary conditions at infinity. Figures
2-4 show the velocities in the ,
,
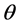 and
and
 directions respectively. Figure 5 demonstrates
distribution of pressure in the
directions respectively. Figure 5 demonstrates
distribution of pressure in the
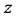 direction. In Table 1
direction. In Table 1
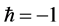 is not a good approximation in every case. The results show that the HPM-Padé isonly
valid for small values of independent variable.
is not a good approximation in every case. The results show that the HPM-Padé isonly
valid for small values of independent variable.
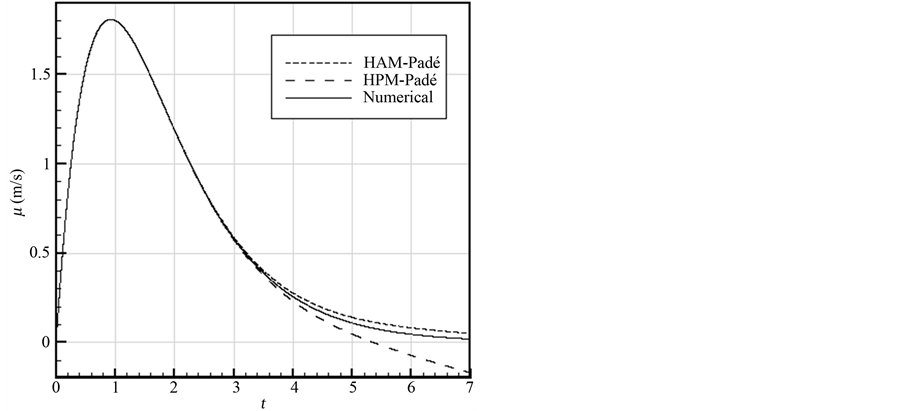
Figure 2. The velocity
in
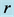 direction for water with
direction for water with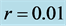 ,
,
 ,
,
 ,
,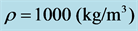 .
.

Figure 3. The velocity
in
 direction for water with
direction for water with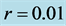 ,
,
 ,
,
 ,
,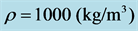 .
.

Figure 4. The velocity
in
 direction for water with
direction for water with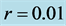 ,
,
 ,
,
 ,
, .
.
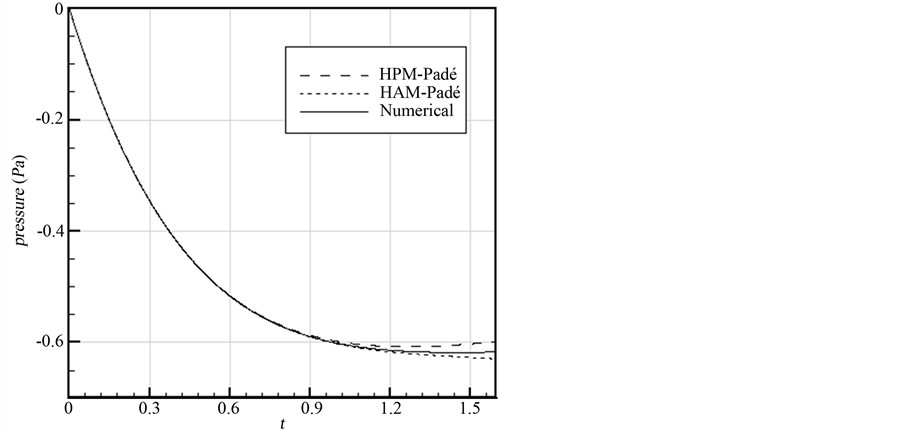
Figure 5. The pressure
distribution in the
 direction for water with
direction for water with ,
,
 ,
,
 ,
,
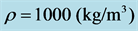 .
.
7. Conclusions
In this paper, homotopy analysis method (HAM) and Padé approximant were considered for finding analytical solution of three-dimensional viscous flow near an infinite rotating disk which is a well-known classical problem in fluid mechanics. A comparison of HAM-Padé and HPM-Padé is made. Unlike perturbation method, the HAM does not depend on any small physical parameters. Thus, it is valid for both weak and strong nonlinear problems. Besides, the HAM provides us with a convenient way of controlling the convergence of approximation series, by means of auxiliary parameter, which is a fundamental qualitative difference in analysis between the HAM and other methods. Also the figures show that the HAM-Padé is a powerful mathematical tool for solving the system of non-linear partial differential equations having wide applications in engineering.
Finally, the recent appearance of nonlinear differential equations as models in some fields of applied mathematics makes it necessary to investigate innovative methods of solution for such equations.
References
- Von Karman, T. (1921) Uberlaminare und turbulence reibung. ZAMM, 1, 52-233.
- Rashidi, M.M. and Shahmohamadi, H. (2009) Analytical Solution of Three-Dimensional Navier-Stokes Equations for the Flow near an Infinite Rotating Disk. Communications in Nonlinear Science and Numerical Simulation, 14, 2999- 3006. http://dx.doi.org/10.1016/j.cnsns.2008.10.030
- Ablowitz, M.J. and Clarkson, P.A. (1990) Solitons, Nonlinear Evolution Equations and Inverse Scattering Transform. Cambridge University Press, Cambridge.
- Fan, E. (2000) Extentedtanh-Function Method and Its Applications to Nonlinear Equations. Physics Letters A, 277, 212-218. http://dx.doi.org/10.1016/S0375-9601(00)00725-8
- Wazwaz, A.M. (2006) The Tanh and the Sine-Cosine Methods for a Reliable Treatment of the Modified Equal Width Equation and Its Variants. Communications in Nonlinear Science and Numerical Simulation, 11, 148-160. http://dx.doi.org/10.1016/j.cnsns.2004.07.001
- Fan, E. and Zhang, H. (1998) A Note on the Homogeneous Balance Method. Physical Letters A, 246, 403-406. http://dx.doi.org/10.1016/S0375-9601(98)00547-7
- He, J.H. and Wu, X.H. (2006) Exp-Function Method for Nonlinear Wave Equations. Chaos Solitons Fractals, 30, 700- 708. http://dx.doi.org/10.1016/j.chaos.2006.03.020
- Rashidi, M.M., Shahmohamadi, H. and Domairry, G. (2011) Variational Iteration Method for Solving Three-Dimensional Navier-Stokes Equations of Flow between Two Stretchable Disks. Numerical Methods for Partial Differential Equations, 27, 292-301. http://onlinelibrary.wiley.com/doi/10.1002/num.20522/pdf http://dx.doi.org/10.1002/num.20522
- Rashidi, M.M., Ganji, D.D. and Shahmohamadi, H. (2011) Variational Iteration Method for Two-Dimensional Steady Slip Flow in Micro-Channels. Archive of Applied Mechanics, 81, 1597-1605. http://link.springer.com/article/10.1007%2Fs00419-010-0504-x http://dx.doi.org/10.1007/s00419-010-0504-x
- Shahmohamadi, H., Rashidi, M.M. and Anwar Bég, O. (2012) A New Technique for Solving Steady Flow and Heat Transfer from a Rotating Disk in High Permeability Media. International Journal of Applied Mathematics and Mechanics, 8, 1-17. http://ijamm.bc.cityu.edu.hk/ijamm/outbox/Y2012V8N7P1C37192935.pdf
- Shahmohamadi, H. and Rashidi, M.M. (2010) A Novel Solution for the Glauert-Jet Problem by Variational Iteration Method-Padé Approximant. Mathematical Problems in Engineering, 2010, Article ID: 501476. http://dx.doi.org/10.1155/2010/501476
- Shahmohamadi, H. and Rashidi, M.M. (2011) Explicit Solutions for Steady Three-Dimensional Problem of Condensation Film on Inclined Rotating Disk. International Journal of Fluid Mechanics Research, 38, 353-365. http://www.dl.begellhouse.com/journals/71cb29ca5b40f8f8,3b02a11311f01f8d,714ff56d76778e5a.html http://dx.doi.org/10.1615/InterJFluidMechRes.v38.i4.50
- Liao, S.J. (1992) The Proposed Homotopy Analysis Technique for the Solution of Nonlinear Problems. PhD Thesis, Shanghai Jiao Tong University, Shanghai.
- Liao, S.J. (2003) Beyond Perturbation: Introduction to Homotopy Analysis Method. Chapman & Hall/CRC, Boca Raton. http://dx.doi.org/10.1201/9780203491164
- He, J.H. (2000) A Coupling Method for Homotopy Technique and Perturbation Technique for Nonlinear Problem. International Journal of Non-Linear Mechanics, 35, 37-43. http://dx.doi.org/10.1016/S0020-7462(98)00085-7
- Liao, S.J. (2005) Comparison between the Homotopy Analysis Method and Homotopy Perturbation Method. Applied Mathematics and Computation, 169, 1186-1194. http://dx.doi.org/10.1016/j.amc.2004.10.058
- Sajid, M., Hayat, T. and Asghar, S. (2007) Comparison between the HAM and HPM Solutions of Tin Film Flows of Non-Newtonian Fluids on a Moving Belt. Nonlinear Dynamics, 50, 27-35. http://dx.doi.org/10.1007/s11071-006-9140-y
- Abbasbandy, S. (2007) The Application of Homotopy Analysis Method to Solve a Generalized Hirota-Satsuma Coupled KdV Equation. Physics Letters A, 361, 478-483. http://dx.doi.org/10.1016/j.physleta.2006.09.105
- Sajid, M. and Hayat, T. (2008) Comparison of HAM and HPM Methods in Nonlinear Heat Conduction and Convection Equations. Nonlinear Analysis: Real World Applications, 9, 2296-2301. http://dx.doi.org/10.1016/j.nonrwa.2007.08.007
- Shahmohamadi, H. (2012) Analytic Study on Non-Newtonian Natural Convection Boundary Layer Flow with Variable Wall Temperature on a Horizontal Plate. Meccanica, 47, 1313-1323. http://link.springer.com/article/10.1007%2Fs11012-011-9515-0 http://dx.doi.org/10.1007/s11012-011-9515-0
- Shahmohamadi, H. (2011) Reliable Treatment of a New Analytical Method for Solving MHD Boundary-Layer Equations. Meccanica, 46, 921-933. http://link.springer.com/article/10.1007%2Fs11012-010-9350-8 http://dx.doi.org/10.1007/s11012-010-9350-8
- Rashidi, M.M., Shahmohamadi, H. and Dinarvand, S. (2008) Analytic Approximate Solutions for Unsteady Two-Dimensional and Axisymmetric Squeezing Flows between Parallel Plates. Mathematical Problems in Engineering, 2008, Article ID: 935095. http://dx.doi.org/10.1155/2008/935095
- Ismail, H.N.A. and Abde Rabboh, A.A. (2004) A Restrictive Padé Approximation for the Solution of the Generalized Fisher and Burger-Fisher Equations. Applied Mathematics and Computation, 154, 203-213. http://dx.doi.org/10.1016/S0096-3003(03)00703-3
- Liao, S.J. (2010) An Optimal Homotopy-Analysis Approach for Strongly Nonlinear Differential Equations. Communications in Nonlinear Science and Numerical Simulation, 15, 2003-2016. http://dx.doi.org/10.1016/j.cnsns.2009.09.002
NOTES

*Corresponding author.


