International Journal of Astronomy and Astrophysics
Vol.06 No.02(2016), Article ID:65395,10 pages
10.4236/ijaa.2016.62011
On a Fractal Version of Witten’s M-Theory
Mohamed S. El Naschie
Department of Physics, Faculty of Science, University of Alexandria, Alexandria, Egypt

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 15 March 2016; accepted 5 April 2016; published 8 April 2016
ABSTRACT
Starting from Witten’s eleven dimensional M-theory, the present work develops in an analogous way a corresponding 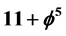 dimensional fractal version where
dimensional fractal version where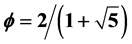 . Subsequently, the new fractal formalism is utilized to determine the measured ordinary energy density of the cos- mos which turns out to be intimately linked to the new theory’s fractal dimension via non-integer irrational Lorentzian-like factor:
. Subsequently, the new fractal formalism is utilized to determine the measured ordinary energy density of the cos- mos which turns out to be intimately linked to the new theory’s fractal dimension via non-integer irrational Lorentzian-like factor:  where
where 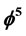 is Hardy’s probability of quan- tum entanglement. Consequently, the energy density is found from a limiting classical kinetic en- ergy to be
is Hardy’s probability of quan- tum entanglement. Consequently, the energy density is found from a limiting classical kinetic en- ergy to be 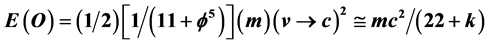 Here,
Here,  is ‘tHooft’s renormalon of dimensional regularization. The immediate logical, mathematical and physical im- plication of this result is that the dark energy density of the cosmos must be
is ‘tHooft’s renormalon of dimensional regularization. The immediate logical, mathematical and physical im- plication of this result is that the dark energy density of the cosmos must be 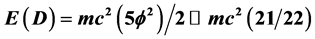 in astounding agreement with cosmic measurements and ob- servations.
in astounding agreement with cosmic measurements and ob- servations.
Keywords:
M-Theory, E-Infinity Theory, Hardy’s Quantum Entanglement, Transfinite Turing Computer, Dark Energy, Accelerated Cosmic Expansion, Noncommutative Geometry, Superstring Theory, Scale Relativity, Cantorian-Fractal Spacetime, Witten’s Theory, ‘tHooft Renormalon, Pure Gravity, Penrose Tiling

1. Introduction
Quantum mechanics implies a fundamentally discrete micro universe which could not be more different from both the continuous and smooth 3D space of classical mechanics nor the equally smooth and continuous 4D spacetime of relativity whether flat or curved as in the special and the general theory respectively [1] - [11] . This situation is not resolved by noting that orthodox quantum mechanics is in general not formulated in spacetime and the dilemma is noted long ago by no one less than Albert Einstein himself [1] - [30] . There are of course many theories which are developed especially to overcome the above difficulties, notably superstrings [21] - [23] , noncommutative geometry [24] and related theories [20] - [27] . In particular, Witten’s M-theory had the special merit of unifying the various known string theories [22] [23] . Apart from this mainstream cutting edge research activity, there is at least one direction which may appear at first glance as too radical but we hope to show in the present work that even though it looks radical, it is actually simpler to use in a complex situation like the one at hand when both the large scale and the micro scale structure of space, time and energy are all relevant [13] - [20] .
In the present work, we introduce E-infinity theory in which we can, in a manner of speech, “differentiate” and “integrate” the non-differentiable and non-integrible, namely geometrical structures made up entirely from transfinite point set of higher dimensional Cantor sets [12] - [16] . At a minimum, this is shockingly unconventional at least in the first few moments. However noting the use of non-standard analysis in the context of Nottale’s theory of scale relativity as well as similar tools used in the work of the great French mathematical physicist A. Connes, the inventor of noncommutative geometry [24] [28] , the situation may start appearing slowly but surely in a different light. On this optimistic note, we will give in the present paper some more detail and explanation of the essential tools of the E-infinity Cantorian spacetime trade [20] - [34] before embarking upon applying our theory to the major problem of the discrepancy between the measured energy density of the cosmos as compared to the theoretical expectations. We are of course not divulging prematurely any secret when we say that one of our main tools in reaching our exact result is the fractal version of Witten’s eleven dimensional theory as it is clear from the title of the present work and it goes without saying that this fractal M-theory [18] [19] is as much the central piece of the work as it is the accurate determination of the ordinary and the dark energy density of this cosmos which turns out to be quite a surprise connected to what is probably the most famous equation in the history of theoretical physics, namely Einstein’s E = mc2 where E is the energy, m is the mass and c is the speed of light [30] - [33] . The basic idea is that the fundamentally classical relativity theory E = mc2 consists of two fundamentally non-classical quantum components that when added together, produce Eins
tein’s beauty in the following manner: 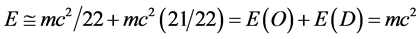 where E(O) is the ordinary energy and E(D) is the dark energy density of the cosmos [30] - [33] .
where E(O) is the ordinary energy and E(D) is the dark energy density of the cosmos [30] - [33] .
Readers who are interested in the foundation of the theory and not only the results may find consulting refs. [30] - [49] quite useful.
2. The Semi Calculus of Cantorian Geometry
The following example is the simplest way to illustrate our E-infinity semi calculus. Let us take 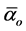 of the electromagnetic field. This is the inverse Sommerfeld fine structure constant. The integer part is the famous prime number 137 [12] - [20] . In addition we have
of the electromagnetic field. This is the inverse Sommerfeld fine structure constant. The integer part is the famous prime number 137 [12] - [20] . In addition we have  leading to the experimentally well founded value
leading to the experimentally well founded value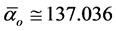 . Now in E-infinity theory the theoretical value is an exact transfinite number, which we give here without justification which will be given a posteriori [14] - [16] [40] - [46]
. Now in E-infinity theory the theoretical value is an exact transfinite number, which we give here without justification which will be given a posteriori [14] - [16] [40] - [46]
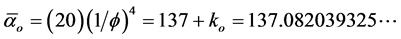 (1)
(1)
Here 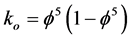 and
and 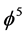 is Hardy’s quantum entanglement probability for two particles. Now we scale
is Hardy’s quantum entanglement probability for two particles. Now we scale 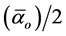 using
using 

where 


differentiation. Consequently scaling up, being the opposite operation is the counterpart of integration [14] - [20] [36] - [39] . Of course a claim is no proof but our present aim is to introduce the reader to the method and familiarize him with it and let it sink in first before showing it using more than plausible arguments.
Let us next ponder the meaning of our down scaling-differentiation [36] . First 42 + k is the exact inverse coupling constant of non-super symmetric, i.e. grand unification of all fundamental forces except gravity. The 26 + k is on the other hand the same as above but for super symmetric theory. Alternatively it is the exact dimension of the bosonic sector of string theory or Heterotic strings. Then we have 16 + k which is the exact fractal number of the extra 16 dimensions of Heterotic strings running in the opposite direction to the 26 + k. Subsequently we have the ten integer dimensions of superstrings which is a neat subtraction of 16 + k from 26 + k giving exactly 10 as it should be. Our Heterotic strings now go on to the next 6 + k compactified spacetime dimension of our basic D = 10 and that leads to a ‘tHooft-Veltman-Wilson dimensional regularization fractal space-time with ‘tHooft’s dimension 

of D = 4 topological dimensionality of spacetime. In this case we have 


Let us examine the last value of the above. This could actually be rewritten as [32] [33]

where E8E8 is the 496 dimensional Lie symmetry group of superstring theory and 

of the basic symmetry group of our spacetime symmetry after subtracting the topological dimensionality of Einstein’s spacetime, then times only to find this dimensionality again. That means [37]

or

How could this intricate web of delicately balanced interrelations exist without a firm “belief” in a single basic assumption namely that E-infinity and the associated golden mean transfinite computer is what nature has chosen to base itself upon [40] - [46] . The universe is indeed a gigantic transfinite Turing machine but we have to show much more to be truly convincing and there is indeed far more to come when we consider the transfinite
version of superstring

the degrees of freedom of |SL(2,7)| = 336 of the holographic boundary of Susskind and ‘tHooft which means (24)(336) = 8064. On the other hand, we know from E-infinity theory that 24 should be 26 + k and 336 should be 336 + 16k when transfinitely corrected to present perfect simplistic tiling of the compactified holographic boundary modelled by the compactified Klein modular curve [35] - [40] . Now, we examine the down scaling of the exact value of No, namely

Remarkably this is 

This means after 16 “steps” we found the exact dimension found for the first time by Ambjorn, Loll and their team for the emerging quantum gravity of their causal triangulation theory [47] .
3. Further Evidence for Grand Connectivity between Number Theory and Physics
The reader is asked to permit the Author to close the circle of interrelations for the time being in order to continue the main task of the present paper. We need to point out three facts behind the preceding demonstration and the belief that behind nature as a whole there is an average Lie symmetry group made up of all symmetry groups known in our present presumably exhaustive classification [37] [41] giving rise to what we observe in high energy physics experiments as well as cosmic measurement and observation. For this purpose we need the following [37] :
a) The fundamental equation of conserving the dimensions of the symmetry groups [37]

where 


b) Furthermore and remarkably so, E8E8 emerges as follows [37]

which is a well known value in Heterotic string theory. Then adding |SU(5)| we find the value for Witten’s 5 Brane in 11 dimensions theory, namely [37]

where 528 is the number of killing vector fields given otherwise by the well known formula [19] [23] [32]

c) Finally when noting that adding the standard model and 8 extra messenger particles leads to 528 + 20 = 548 gives us exactly



With that we think the reader can see that there are sufficient reasons to suspect that allegations of numerology is just a colossal misunderstanding of the depth of the role that transfinite number theory plays in physics and
that the universe may be indeed likened to a gigantic transfinite Turing computer. In fact our basic 





where 






and this quasi dimensionality in turn could be portioned into D = 4 for Einstein’s spacetime, D = 22 for the compactified dimensions of Bosonic D = 26 string theory leaving D = 74 being related to pure dark energy [48] . Thus the dark section of our 100 would be 22 + 74 = 96 or 96% of the total leaving only 4% for ordinary energy. This is almost exactly what we find from applying Dvoretzky’s theorem to our universe and more profoundly this is almost the result of the extensive cosmological measurements and observations which were found via COBE, WMAP and type 1a supernova [41] [48] . Consequently this is physics and cannot be dismissed using cheaply using words like numerology [36] [39] [49] . The Author notes what no one less than G. ‘tHooft told him, namely that normally 

4. The Fractal Witten Spacetime M-Theory
The exceptional mathematical physicist E. Witten started his derivation of M-theory [32] [49] with the crucial point, namely its super gravity-like eleven dimensionality by correctly noting that one needs a minimum of seven dimensions [32] to embed the standard Model SU(3) SU(2) U(1). Similarly we start by noting that in E- infinity theory the corresponding space needs a transfinitely corrected value equal to [33]

Next Witten added to these 7 dimensions the four dimensionalities of Einstein’s relativity and that gave him then his 7 + 4 = 11 dimensionality [32] [49] . Again we proceed in an analogous way and add to our 


which has a remarkable self-similar continued fraction expansion [33]

In the following section, we will see how this subtle 
5. Dark Energy Density via Fractal Cantorian Witten’s M-Theory
Let us start with a well established fact, namely that unlike a completely smooth isotropic space, a Penrose fractal tiling, i.e. Cantorian universe [28] [34] has an isomorphic length given by half the Hausdorff dimension of E- infinity spacetime, i.e. 

where 






corresponding space is easily found from adding to 





which is nothing else but the dimensionality of our fractal Cantorian M-theory. Noting all the preceding results it becomes obvious that Einstein’s maximal energy “density”

only needs to be scaled by the ratio of the isomorphic length of Einstein space, i.e. 1/2 and the isomorphic length of the super symmetric fractal M-theory, namely 

and the ordinary energy density

as found using other methods and in full agreement with cosmic measurements and observations [30] [48] . The physical meaning of the analysis, although relatively obvious, would benefit and become clearer from the following elucidation: Unlike classical particles a quantum particle is both a particle and a wave. In E-infinity, the
particle aspect is modelled by the zero set 

or \the equivalent bijection formula [14] - [17]

which we used earlier on.
The quantum wave on the other hand, is modelled by the empty set 


parable from its cobordism, namely the quantum wave and visa versa. Now, energy and volume at this level is also two sides of the same coin and that is why we aim at determining the volume of the particle and the area of its surface. Of course we know that for a hyper volume the hyper surface could be a volume rather than an area but we will keep the distinction for a very good reason. The reason is that volume is a multiplicative product while surface is an additive process. Thus noting that a maximal volume is given for n = 5 dimensions and that





Now the ratio between any of the two values to the sum would give us the corresponding percentage of the energy. Since the sum is [16] [18] [19]

it follows then that for the particle we have the ordinary energy density [18] - [20]

while for the wave we have the dark energy density [18] - [20]

The corresponding energy in terms of Einstein’s famous formula is thus [30] [33]

and

which confirms our previous derivation using Witten’s Cantorian-fractal M-theory.
Some minimalistic derivations of dark energy and ordinary energy of the cosmos based on Witten-Duff 5-D Brane in eleven dimensional models, the degree of freedom of the vacuum and ‘tHooft-Veltman-Wilson regularization space.
In the following, we give in a nutshell a few minimalistic derivations of E(D) and E(O) confirming the preceding result. The analysis is tailored mainly to the connoisseurs of the subject and goes as follows:
a) The vital five indices term in the total of 528 states in Witten-Duff theory is the dive dimensional 462 super charges. A little thinking makes it immediately clear that the dark energy density must be the ratio of the 462 to the total 528 minus 44 degrees of freedom of the D-11 vacuum or pure gravity [23] [32] . Consequently

exactly as we found earlier on.
b) We could argue in a similar way but using a pure gravity vacuum. For Einstein’s D = 4 pure gravity degrees of freedom is 


and thus

also as before.
c) Next, we look at the fact that Einstein’s smooth space is D = 4. However, ‘tHooft-Veltman-Wilson fractal spacetime is 



while 
d) Finally, for whatever its worth, the Author feels that the fractal Kaluza-Klein [3] theory with 

6. Conclusion
Using the E-infinity methodology, we transferred in the present work the essence of Witten’s M-theory to a fractal-Cantorian setting. Subsequently, the theory is used to give an exact solution to the problem of the missing dark energy and on route we give a deeper insight into how E-infinity theory is a concerted method which utilizes topology as well as number theory reinforced by traditional theoretical and experimental results to give a simple analysis for complex problems. Our theory is basically a simplistic theory for complex quantum cosmology and high energy physics.
Acknowledgements
As usual, the author is truly and genuinely indebted to all the authors of the cited papers, not withstanding his own.
Cite this paper
Mohamed S. El Naschie, (2016) On a Fractal Version of Witten’s M-Theory. International Journal of Astronomy and Astrophysics,06,135-144. doi: 10.4236/ijaa.2016.62011
References
- 1. W. Rindler: Relativity (Special, General and Cosmological). Second Edition, Oxford University Press, Oxford, 2006.
- 2. A. Zee: Einstein’s Universe. Oxford University Press, Oxford, UK, 1989.
- 3. P.S. Wesson: Five Dimensional Physics, World Scientific, Singapore, 2006.
http://dx.doi.org/10.1142/6029 - 4. S. Hawking and R. Penrose: The Nature of Space and Time. Princeton University Press, Princeton, USA, 1996.
- 5. S. Weinberg: Cosmology. Oxford University Press, Oxford, UK. 2008.
- 6. E.F. Tayler and J.A. Wheeler: Spacetime Physics. W.H. Freeman Company, New York, USA, 1966.
- 7. J. Moffat: A Physicist Goes Beyond Einstein. Smithsonian Books, New York, USA, 2008.
- 8. M. Carmeli: Cosmological Relativity. World Scientific, Singapore, 2006.
http://dx.doi.org/10.1142/6275 - 9. Ø. Grøn and S. Hervik: Einstein’s General Theory of Relativity—With ModernApplications in Cosmology. Springer, New York, USA, 2007.
http://dx.doi.org/10.1007/978-0-387-69200-5 - 10. Y. Baryshev and P. Teerikorpe: Discovery of Cosmic Fractals. World Scientific, New Jersey, USA, 2002.
- 11. G. Ellis and P. Williams: Flat and curved space-time. Second Edition. Oxford University Press, Oxford, UK, 2000.
- 12. M.S. El Naschie: Einstein’s dream and fractal gravity. Chaos, Solitons & Fractals, 24(1), 2005, pp. 1-5.
http://dx.doi.org/10.1016/j.chaos.2004.09.001 - 13. M.S. El Naschie: A resolution of cosmic dark energy via a quantum entanglement relativity theory. Journal of Quantum Information Science, 3(1), 2013, pp. 23-26.
http://dx.doi.org/10.4236/jqis.2013.31006 - 14. M.S. El Naschie: A review of E-infinity and the mass spectrum of high energy particle physics. Chaos, Solitons & Fractals, 19, 2004, pp. 209-236.
http://dx.doi.org/10.1016/S0960-0779(03)00278-9 - 15. M.S. El Naschie: The theory of Cantorian spacetime and high energy particle physics (an informal review). Chaos, Solitons & Fractals, 41, 2009, pp. 2635-2646.
http://dx.doi.org/10.1016/j.chaos.2008.09.059 - 16. L. Marek-Crnjac, et al.: Chaotic fractals at the root of relativistic quantum physics and cosmology. International Journal of Modern Nonlinear Theory & Application, 2(1A), 2013, pp. 78-88.
http://dx.doi.org/10.4236/ijmnta.2013.21A010 - 17. M.S. El Naschie: The concepts of E-infinity: An elementary introduction to the Cantorian-fractal theory of quantum physics. Chaos, Solitons & Fractals, 22, 2004, pp. 495-511.
http://dx.doi.org/10.1016/j.chaos.2004.02.028 - 18. M.S. El Naschie: A unified newtonian-relativistic quantum resolution of supposedly missing dark energy of the cosmos and the constancy of the speed of light. International Journal of Modern Nonlinear Theory & Application, 2013, 2, pp. 43-54.
http://dx.doi.org/10.4236/ijmnta.2013.21005 - 19. M.S. El Naschie: Nash embedding of Witten’s M-theory and the Hawking-Hartle quantum wave of dark energy. Journal of Modern Physics, 4(10), 2013, pp. 1417-1428.
http://dx.doi.org/10.4236/jmp.2013.410170 - 20. M.S. El Naschie: Cosserat-Cartan and de Sitter-Witten spacetime setting for dark energy. Quantum Matter, 5(1), 2016, pp. 1-4.
http://dx.doi.org/10.1166/qm.2016.1247 - 21. K. Becker, M. Becker and J.H. Schwarz: String theory and M-theory. Cambridge University Press, Cambridge, 2007.
- 22. P. West: Introduction to strings and branes. Cambridge University Press, Cambridge, UK, 2012.
http://dx.doi.org/10.1017/CBO9781139045926 - 23. M. Kaku: Strings, Conformal fields and M-theory. Springer, New York, USA, 2000.
http://dx.doi.org/10.1007/978-1-4612-0503-6 - 24. A. Connes: Noncommutative geometry. Academic Press, San Diego, 1994.
- 25. C. Rovelli: Quantum gravity. Cambridge Press, Cambridge, 2004.
http://dx.doi.org/10.1017/CBO9780511755804 - 26. D. Freedman and A. van Proeyen: Super gravity. Cambridge University Press, Cambridge, 2004.
- 27. C.V. Johnson: D-Branes. Cambridge University Press, Cambridge, UK, 2003.
- 28. M.S. El Naschie: Penrose universe and Cantorian spacetime as a model for noncommutative quantum geometry. Chaos, Solitons & Fractals, 9(6), 1998, pp. 931-933.
http://dx.doi.org/10.1016/S0960-0779(98)00077-0 - 29. M.S. El Naschie: Superstrings, knots and noncommutative geometry in E-infinity space. International Journal of Theoretical Physics, 37(12), 1998, pp. 2935-2951.
http://dx.doi.org/10.1023/A:1026679628582 - 30. L. Marek-Crnjac, J.-H. He: An invitation to El Naschie’s theory of Cantorian space-time and dark energy. International Journal of Astronomy and Astrophysics, 3(4), 2013, pp. 464-471.
http://dx.doi.org/10.4236/ijaa.2013.34053 - 31. J.-H. He, et al.: The importance of the empty set and noncommutative geometry in underpinning the foundations of quantum physics. Nonlinear Science B, 1(1), 2011, pp. 14-23.
- 32. M. Duff (Editor): The world in eleven dimensions. IOP Publishing, Bristol, UK, 1999.
- 33. M.S. El Naschie: A Rindler-KAM spacetime geometry and scaling the planck scale solves quantum relativity and explains dark energy. International Journal of Astronomy and Astrophysics, 3(4), 2013, pp. 483-493.
http://dx.doi.org/10.4236/ijaa.2013.34056 - 34. M. Gardner: Penrose tiles to trapdoor ciphers. W.H. Freeman, New York, USA, 1989. (See in particular Chapter 1, pp. 10).
- 35. E-Infinity Group: The exact renormalization equation for E-infinity unification of fundamental forces, quantum mechanics and the golden mean in theoretical physics. E-infinity-High Energy Communication No. 62, 15 December 2010. e-infinity.blogspot.co.uk/2010/exactrenormalization-equation-for-e.html
- 36. M.S. El Naschie: Elementary number theory in superstring loop quantum mechanics, twistors and E-infinity high energy physics. Chaos, Solitons & Fractals, 27(2), 2006, pp. 297-330.
http://dx.doi.org/10.1016/j.chaos.2005.04.116 - 37. M.S. El Naschie: String theory, exceptional Lie groups hierarchy and the structural constant of the universe. Chaos, Solitons & Fractals, 35(1), 2008, pp. 7-12.
http://dx.doi.org/10.1016/j.chaos.2007.06.023 - 38. L. Marek-Crnjac: Stein spaces in connection with El Naschie’s exceptional Lie groups hierarchies in high energy physics. Chaos, Solitons & Fractals, 38(2), 2008, pp. 309-315.
http://dx.doi.org/10.1016/j.chaos.2008.01.022 - 39. T. Zhong: From the numeric of dynamics to the dynamics of numeric and visa versa in high energy particle physics. Chaos, Solitons & Fractals, 42, 2009, pp. 1780-1783.
http://dx.doi.org/10.1016/j.chaos.2009.03.079 - 40. M.S. El Naschie: On a new elementary particle from the disintegration of the Symplectic ‘t Hooft-Veltman-Wilson fractal spacetime. World Journal of Nuclear Science and Technology, 4(4), 2014, pp. 216-221.
http://dx.doi.org/10.4236/wjnst.2014.44027 - 41. M.S. El Naschie: Mathematical models and methods in dark energy theory: Dvoretzky’s theorem, Casimir effect, Mobius geometry. Problems of Nonlinear Analysis in Engineering Systems, 2(44), 2015, pp. 1-16. University of Kazan Press, Russia. (Published in Russian and English language versions).
- 42. M.S. El Naschie: Dark energy and its cosmic density from Einstein’s relativity and gauge fields renormalization leading to the possibility of a new ‘tHooft quasi particle. The Open Journal of Astronomy, 8, 2015, pp. 1-17.
http://dx.doi.org/10.2174/1874381101508010001 - 43. M.S. El Naschie: Compactified dimensions as produced by quantum entanglement, the four dimensionality of Einstein’s smooth spacetime and ‘tHooft’s 4-ε fractal spacetime. American Journal of Astronomy & Astrophysics, 2(3), 2014, pp. 34-37.
http://dx.doi.org/10.11648/j.ajaa.20140203.12 - 44. M.S. El Naschie: The quantum gravity immirzi parameter—A general physical and topological interpretation. Gravity and Cosmology, 19(3), 2013, 151-153.
http://dx.doi.org/10.1134/S0202289313030031 - 45. J.-P. Auffray: E-infinity, the zero set, absolute space and the photon spin. Journal of Modern Physics, 6(5), 2015, pp. 536-545.
http://dx.doi.org/10.4236/jmp.2015.65058 - 46. M.-W. Ho, M.S. El Naschie and M.W. Giuseppe Vitello: Is spacetime fractal and quantum coherent in the golden mean? Global Journal of Science Frontier Research, 15(1), 2015, pp. 61-80.
- 47. J. Ambjorn, J. Jurkiewicz and R. Loll: Quantum gravity: The act of building spacetime. In “Quantum Gravity”, Edited by D. Oriti. Cambridge University Press, Cambridge, UK, 2009, pp. 342-359.
- 48. L. Amendola and S. Tsujikawa: Dark energy: Theory and observation. Cambridge University Press, Cambridge, UK, 2010.
http://dx.doi.org/10.1017/CBO9780511750823 - 49. M.S. El Naschie: On the unification of heterotic strings, M theory and E (∞) theory. Chaos, Solitons & Fractals, 11(14), 2000, pp. 2397-2408.
http://dx.doi.org/10.1016/S0960-0779(00)00108-9

