ABSTRACT

Based on Lyapunov stability theorem, a method is proposed for feedback syn- chronization with parameters perturbation and external disturbances. It is proved theoretically that if the perturbation and disturbances are bounded, the synchronization error can be ensured to approach to and stay within the pre-specified bound which can be arbitrarily small. Some typical chaotic systems with different types of nonlinearity, such as Lorenz system and the original Chua’s circuit, are used for detailed description. The simulation results show the feasibility of the method.

Keywords:

Lyapunov Stability Theorem, Feedback Synchronization, Parameters Perturbation, External Disturbances, Robustness

1. Introduction

In 1990, Pecora and Carroll presented the conception of “chaotic synchronization” and introduced a method to synchronize two identical chaotic systems with different initial conditions [1] [2] . Since chaos control and synchronization have great potential applications in many areas such as information science, medicine, biology and engineering, they have received a great deal of attention. Numerous researches have been done theoretically and experimentally [3] [4] [5] . Muradi and Kapitaniak expanded Corroll and Pecora’s work, presented a single unidirectional coupled synchronization scheme [6] [7] . Celka achieved chaos synchronization by using the time-delay feedback method [8] . Agiza et al. synchronized Rössler and Chen systems via active control method [9] and Impulsive control [10] . Guo et al. proposed a simple adaptive-feedback controller for chaos synchronization [11] . Agrawal et al. realized the synchronization of fractional order chaotic systems using active control method [12] . Norelys et al. presented the adaptive synchronization of fractional Lorenz systems using a reduced number of control signals and parameters [13] . Kajbaf et al. used sliding mode controller to obtain chaotic systems [14] . Wang et al. proposed a new feedback synchronization criterion based on the largest Lyapunov exponent [15] . However, most synchronization criterions were obtained under ideal circumstances. If parameters perturbation and external disturbance exist, this kind of criterions will take no effect. According to this practical problem, some solutions have been presented. For examples, Jiang et al. proposed a LMI criterion [16] for chaotic feedback synchronization. Although the simulations showed that it is robust to a random noise with zero mean, but no rigorous mathematical proof was provided and we can’t determine if their method is effective for other kinds of noise. In Ref. [17] , parameters perturbation was involved in their scheme. The theoretical proof and numerical simulations were given in their work, but external disturbance didn’t receive attention, which made their method unila- teral.

Above all, these methods are effective, but still lack generality or robustness. In this paper, we propose a practical synchronization scheme for chaotic synchronization with parameters perturbation and external disturbance. Rigorous mathematical proof is provided, and simulation results show the feasibility and robustness of our scheme.

2. Theory and Method

In the following scheme, a universal robust synchronization method is proposed. In the method, synchronization will be achieved with bounded parameter disturbances and noise.

Suppose a class of ideal chaotic systems as

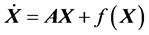
where 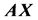is the linear part, 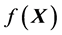is the nonlinear part, then the system can be described as

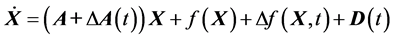(1)

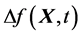where and are the parameters perturbation, 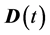is the external disturbance. Choose system (1) as the drive system, the relevant response system can be described as

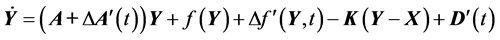(2)

where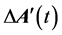, 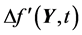and are the relevant disturbances in the response system. We choose 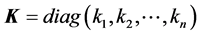(n is the dimension of the chaotic system). Let the error vector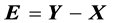, then the error is

(3)

Set a pre-defined bound 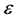for the synchronization error, suppose 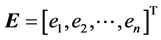, choose suitable 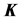to ensure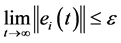 , then system (1) and system (2) achieve approximate synchronization, the precision is. When is very small, we can consider system (1) and system (2) have been synchronized.

Choose the following Lyapunov function, yield.

According to Equation (3), the derivative of can be described as

(4)

is the element of matrix, and is bounded, is bounded external disturbances, is feedback coefficients．When the errors go beyond, we have

(5)

(6)

If

(7)

we can obtain

(8)

That is to say, when the error is not within the bound, it will exponentially converge to zero. Hence system (1) and system (2) will achieve approximate synchronization, the precision is at least.

3. Numerical Simulations

Lorenz system and the original Chua’s circuit have different types of nonlinearity. Next we will adopt the two systems for detailed description.

3.1. Taking Lorenz System as Example

Lorenz system [18] is described as

(9)

In the paper choose, , so that system (9) exhibits a chaotic behavior [18] . The projections of Lorenz system’s attractor are shown in Figure 1. Obviously we have.

Choose the following Lorenz system with parameters perturbation and external disturbances

(10)

as drive system, then the relevant response system is

(11)

In system (10) and system (11), are parameters perturbation, are external disturbances, , , are feedback coefficients. Let

(12)

Then, ,. The error system is

(13)

Hence

(14)

where

(15)

Choose Lyapunov function

(16)

We have

(17)

Substitute Equation (14) into Equation (17), obtain

If

(18)

is satisfied, we will obtain. According to Lyapunov stability theorem, the error system (13) will converge to zero when the error is not within the bound, i.e. system (10) and system (11) will achieve approximate synchronization, the precision is at least．

When the parameters perturbation and external disturbances are small, we can consider the variables of system (10) and system (11) are bounded as shown in Figure 1. Suppose the upper bounds of these disturbances and perturbation are 0.5, choose, substitute Equation (15) into Equation (18), after calculating we obtain if

(19)

is satisfied, Equation (18) will be always true.

In the simulation, suppose, , , , , , are random from -0.5 to 0.5. A time step of size 0.0001 (sec.) is employed and fourth-order Runge-Kutta method is used to solve Equation (10) and Equation (11). Let, , , Figure 2 shows the history of, , in the error system (13) within 0.1 sec. From Figure 2, we can see that, , are steady near zero at last.

3.2. Taking the Original Chua’s Circuit as Example

The original Chua’s circuit [19] is described as

(20)

where. In this paper choose, , and so that system (20) exhibits a chaotic be- havior [19] . The projections of the original Chua’s circuit’s attractor are shown in Figure 3. Obviously we have.

Choose the following Chua’s circuit with parameters perturbation and external disturbances

(21)

As drive system, where , then relevant re- sponse system is

(22)

where. In system (21) and system (22), are parameters perturbation, are external disturbances, , , are feedback coefficients. Let

(23)

Then, ,. The error system is

(24)

when the parameters perturbation and external disturbances are small, we can consider the variables of system (21) and system (22) are bounded as shown in Figure 4. Next we will substitute directly to simplify the results, so we have

(25)

(26)

Because

we have

(27)

Hence

(28)

where

(29)

and.

Choose Lyapunov function

(30)

We have

(31)

Substitute Equation (28) into Equation (31), obtain

If

(32)

is satisfied, we will obtain. According to Lyapunov stability theorem, the error system (24) will converge to zero when the error is not within the bound, i.e. system (21) and system (22) will achieve approximate synchronization.

Suppose the upper bounds of these disturbances and perturbation are 0.2, choose, substitute Equation (29) into Equation (32), after calculating we obtain if

(33)

is satisfied, Equation (32) will be always true.

In the above simulation, let, , , , , , , , are random from -0.2 to 0.2. A time step of size 0.0001 (sec.) is employed and fourth-order Runge- Kutta method is used to solve Equation (21) and Equation (22). Let, , , Figure 4 shows the history of, , in the error system (24) within 0.5 sec. From Figure 4, we can see that, , are steady near zero at last.

4. Conclusion

In this paper, a practical scheme is proposed for feedback synchronization with parameters perturbation and external disturbances. Lorenz system and the original Chua’s circuit are used for detailed description. The simulation results show the feasibility of the method. According to Ref. [15] , if all the feedback coefficients are larger than the largest Lyapunov exponent, two identical systems will be synchronized under ideal circumstance. In the paper, our scheme proved that high feedback coefficients will ensure more robust synchronization theoretically. The practical feedback should be bounded in a proper limit, so we have to control the error within a proper bound to obtain suitable feedback. The feedback will be smaller when the error is smaller. It’s not hard for us to find a chance when the error between the drive system and the response system is small enough.

Acknowledgements

The work was supported by Natural Science Foundation of Liaoning Province (No. 201602034).

Cite this paper

Wang, M.J., Yu, W.B. and Zhao, J. (2017) Feedback Chaotic Synchronization with Disturbances. International Journal of Modern Nonlinear Theo- ry and Application, 6, 1-10. http://dx.doi.org/10.4236/ijmnta.2017.61001

References