ABSTRACT

We consider the defocusing mass-critical nonlinear Schrödinger equation in the exterior doma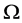in in 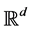(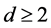). By analyzing Strichartz estimate and utilizing the inductive hypothesis method, we prove the stability for all initial data in.

Keywords:

Mass-Critical, Stability, Nonlinear Schrödinger Equation

1. Introduction

In this short note, we consider the defocusing mass-critical nonlinear Schrödinger equation in the exterior doma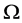in in 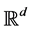() with Dirichlet boundary conditions:

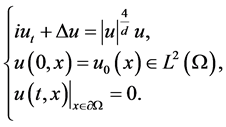(1)

Here 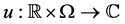and the initial data 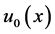will only be required to the 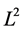space.

This equation has Hamiltonian

(2)

As (2) is preserved by (1), we shall refer to it as the mass and often write 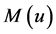or M for.

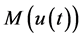H. Brezis and T. Gallouet [1] considered that 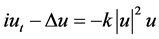in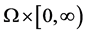, , 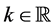the nonlinear Schrödinger equation in 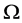of a bounded domain or an exterior domain of with Dirichlet boundary conditions. In [2] , N. Burq, P. Gérard and N. Tzvetkov described nonlinear Schrödinger equations in exterior domains. In [3] [4] , R. Killip, M. Visan and X. Zhang considered the defocusing energy-critical nonlinear Schrödinger equation and the focusing cubic nonlinear Schrödinger equation in the exterior domain of a smooth, compact, strictly convex obstacle in with Dirichlet boundary conditions, respectively.

In [5] , T. Tao and M. Visan established stability of energy-critical nonlinear Schrödinger equations in. However, we established stability of mass-critical nonlinear Schrödinger equations in the exterior domain in ().

Throughout this paper, we restrict ourselves to the following notion of solution.

Definition 1 (solution). Let I be a time interval containing zero, a function is called a solution to (1) if it lies in the class for any compact interval, and it satisfies the Duhamel formula

(3)

for all. The interval I is said to be maximal if the solution cannot be extended beyond I. We say u is a global solution if.

In this formulation, the Dirichlet boundary condition is enforced through the appearance of the linear propagator associated to the Dirichlet Laplacian.

Our stability theorem concerns mass-critical stability in for the initial-value problem associated to the Equation (1).

Theorem 2 (Stability theorem). Suppose, I is a compact interval and let be an approximate solution to

(4)

in the sense that

(5)

for some function e.

Assume that

(6)

(7)

for some positive constants M and L.

Let and obey

(8)

for some. Moreover, assume the smallness conditions

(9)

(10)

for some, where is a small constant.

Then, there exists a solution u to

(11)

on with initial data at time satisfying

(12)

(13)

. (14)

The rest of the paper is organized as follows. In Section 2, we introduce our notations and state some previous results. In Section 3, we finally prove Theorem 2, except for proving a lemma about approximate solutions.

2. Preliminaries and Notations

In this section we summarize some our notations and collect some lemmas that are used in the rest of the paper.

We write to signify that there is a constant such that. We use the notation whenever. If the constant C involved has some explicit dependency, we emphasize it by a subscript. Thus means that for some constant depending on u. We write for the nonlinearity in (1).

We define that for some,

We also define to be the space dual to with appropriate norm.

With these notations, the Strichartz estimates read as follows:

Theorem 3 (Strichartz estimates [3] [6] ). Let be a time interval and let, then the solution to

satisfies

Proposition 4 (Local well-posedness). Given, there exists such that if and

on some interval, , then there exists a unique solution of (1) satisfying. Besides,

The quantities defined in (2) are conserved on I.

3. Proof of Theorem 2

We need the following lemma to prove this theorem.

Lemma 1. Let I be a compact interval and let be an approximate solution to

(15)

in the sense that

(16)

for some function e.

Assume that

(17)

for some positive constant M.

Let and be such that

(18)

for some.

Assume also the smallness conditions

(19)

(20)

(21)

for some, where is a small constant.

Then, there exists a solution u to

(22)

on with initial data at satisfying

(23)

(24)

(25)

. (26)

Proof of Lemma 1. By symmetry, we may assume. Let, then w satisfies the following problem

where.

For, we define

By (19),

(27)

On the other hand, by Strichartz, (20), (21), we get

(28)

Combining (27) and (28), we obtain

By bootstrapping, we see if is taken sufficiently small,

which implies (26).

Using (26) and (28), we see (23).

Moreover, by Strichartz, (18), (21) and (26),

which establishes (24) for sufficiently small.

To show (25), we use Strichartz, (17), (18), (26), (19),

Choosing sufficiently small, this finishes the proof of the lemma. W

We now turn to the proof of stability theorem.

Proof of Theorem 2. We now subdivide I into subintervals

, , such that

where as in the lemma.

We need to replace by as the mass of the difference might grow slightly in time.

By choosing sufficiently small depending on J, M and, we can apply the lemma to obtain for each j and all,

provided we can show that analogues of (8) and (9) hold with replaced by.

In order to verify this, we use an inductive argument.

By Strichartz, (8), (10) and the inductive hypothesis,

Similarly, by Strichartz, (9), (10) and the inductive hypothesis, we see

so we see

Choosing sufficiently small depending on J, M and, we can guarantee that the hypotheses of the lemma continue to hold as j varies. W

4. Conclusion

In this paper, we consider a mass-critical stability of the defocusing mass-critical nonlinear Schrödinger equation. Then we prove two different types of perturbation to show the stability of nonlinear Schrödinger equation.

Acknowledgements

The research of Guangqing Zhang has been partially supported by the NSF grant of China (No. 51509073) and also “The Fundamental Research Funds for the Central Universities” (No. 2014B14214). The author would like to thank his tutor Zhen Hu for helpful conversations. The author also thanks the referees for their time and comments.

Cite this paper

Zhang, G.Q. (2016) On the Stability of the Defocusing Mass- Critical Nonlinear Schrödinger Equation. International Journal of Modern Nonlinear Theory and Application, 5, 115-121. http://dx.doi.org/10.4236/ijmnta.2016.53012

References