Journal of Textile Science and Technology
Vol.01 No.02(2015), Article ID:57797,19 pages
10.4236/jtst.2015.12006
A Combined Discrete Event―Agent Based Approach to Modeling Tensile Strength of One-Dimensional Fibrous Materials. Face Validation and Effect of the Basic Model Parameters
Arkady Cherkassky1*, Eugene Bumagin2
1Department of Industrial Engineering, Shenkar College of Engineering, Design and Art, Ramat-Gan, Israel
2MultiCorpora R&D Inc., Gatineau, Canada
Email: *cherk@shenkar.ac.il, cherk@netvision.net.il
Copyright © 2015 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 26 May 2015; accepted 5 July 2015; published 8 July 2015
ABSTRACT
A combined method of discrete event and agent based modelling has been applied to the computer modelling and simulation of the tensile strength of one-dimensional fibrous materials (ODFM). This combined method is based on the concept of discrete event simulation as being applied to the modeling of the structure of the fiber flow and on the concept of agent based modelling for modelling and simulation of the fiber interaction within the structure of the fibrous material. Frictional and traction forces arise as the result of this fiber interaction. A model of the ODFM tensile strength, which is based on the slippage effect, is created and studied in this research. Only frictional and traction forces determine the tensile strength in this kind of the model. The article examines the validation problem of the slippage effect based tensile strength model and questions regarding the strength potential estimation through variation in the parameters of the model.
Keywords:
Tensile Strength, Discrete Event Simulation, Agent Based Modeling, Face Validation, Fiber Exchange, Model Normalization, Strength Potential

1. Introduction
Tensile strength is one of the most important characteristics of fibrous materials. Various approaches and methods of tensile strength analysis and prediction have been developed and evaluated [1] - [4] over the last few years. The complexity of the strength research problems is determined by the fact that mechanical properties, including tensile strength, are the result of random and non-linear interactions of a huge number of fibers in the structure of the fibrous material [5] - [11] . Traditional methods that study the mechanical properties of fibrous materials are based on analytical modeling [12] - [17] or on regression models and models of artificial intelligence which in their turn are based on experimental data [3] [18] - [20] . These models do not take into account the characteristics of individual fibers and interactions between individual fibers. An analysis of the results obtained by analytical or regression models leads to the conclusion that these approaches have exhausted themselves. This leads to the development of a new approach to the study of the tensile strength problem and the creation of a new class of tensile strength models.
This new approach [21] is based on the idea of keeping track of each individual fiber in the structure of the one-dimensional fibrous materials (ODFM) and to analyze the interactions between separated fibers as tensile strength is a consequence of these fiber interactions. When developing a new approach it is extremely important to take into consideration that the individual fibers are structural elements of fibrous materials, and that the structure of a fibrous material is a flow of fibers which are oriented along the ODFM length. This circumstance has been taken into consideration in developing new models of ODFM within the concept of discrete event simulation (DES) [22] .
An interaction between separated fibers can be described and represented in the framework of agent based modeling [23] - [25] . The agent based approach allows one to consider each individual fiber as an active agent with its own specific location, random shape (crimped fibers), and its individual behavior in the fiber flow (fiber population). The fiber behavior can be described as a set of fiber activity parameters and the tensile strength of the fibrous material is the result of the fiber/agent interactions.
The logical sequence of the tensile strength study within the proposed approach can be represented as a series of sequential steps in which the parameters of the model are determined or the simulation algorithms are implemented: the fiber parameters―the fiber flow parameters―the fiber flow simulation―parameters of the fiber/ agent interaction―modification of the fiber flow structure due to the fiber interaction―the fiber contact analysis―the contact based zero-order model―the twist effect and the friction forces simulation and analysis―the friction forces based first order model.
Thus the model of the fibrous material’s strength is a combined two-layer model. The first level of this model is the sub-model of a random fibrous structure (fiber flow) which can be realized through the DES approach. In the framework of the first level sub-model, which is built on the idea of DES, fibers/agents are presented in the form of parallel line segments. The length of this line segment is the only characteristic of the fiber at this first level. The DES approach allows generating a flow of fibers with any given distribution of this characteristic. Each fiber is an agent in the framework of the agent based modelling concept. The random fibrous structure obtained as a result computer aided simulation is an environment in which the fibers interact.
The parameters of the flow of fibers affect the strength of the fibrous material and are taken into account in the first level model. The nonlinear dependence of the number of fibers in the cross section on the fibers front ends and on the fiber lengths distribution is implemented in the ODFM DES-model [26] . The number of fibers in the cross section, the fiber length and twist multiplier are the most important parameters that determine the number and the length of the contacts within the structure of the ODFM and hence its tensile strength [27] .
The second layer of the combined tensile strength model is a sub-model of fiber interactions. This sub-model is realized in this paper in the framework of agent-based modeling. When modeling tensile strength at the level of the interaction between separated fibers within the structure of the fibrous material, it is absolutely necessary to take into consideration the 3D shape of the fiber and the change of this shape as a result of fiber migration. In this case, in our view, it is useful to consider each individual fiber as an active agent (fiber/agent). Its ability to change location in the cross-sections of the one-dimensional fibrous materials along the length of the material is the new property of the fiber/agent in the framework of a tensile strength problem. However, it should be bear in mind that the agent-based part of the model is designed to play random contacts between the fibers, rather than studying the physical effects arising from the contact of the fibers. These effects are well studied and are not the object of this paper.
In this way, substitution of the passive fiber/agent in the model of the mass irregularity (first level model) by the active fiber/agent in the tensile strength model (second level model) is equivalent to the transition from a one-dimensional model to a three-dimensional model. This kind of three-dimensional model enables consideration of the properties and characteristics of each individual fiber in the structure of the fibrous material.
Algorithms and implementation of the combined discrete event and agent based model of the ODFM tensile strength are presented in [21] . This model describes the fiber/agent interaction depending on a generalized parameter―the activity within the exchange processes. The model considers several types of fiber/agent exchanges and allows modeling nonlinear effects in the fiber/agent exchange processes.
The nature of the effect which leads to the breaking of the ODFM is essential to the modeling and simulation of tensile strength. The relationship between the fiber strength and frictional forces allows determining three types of tensile strength models: (A)―a slippage effect model, (B)―a fiber break effect model, and (C)―a mixed slippage and fiber break effect model.
The images of the broken end structures, presented in [28] and shown in Figure 1, give a clear understanding of these three effects. The slipped broken end on Figure 1 corresponds to the slippage effect model; the sharp broken end corresponds to the fiber break effect model, and the tapered broken end corresponds to the mixed slippage and fiber break effect model.
A combined discrete event and agent based approach enables the construction of the tensile strength model for types A, B, and C; but the algorithms for simulation and prediction of the tensile strength are very different for each of these three types of models.
Due to the complexity of modeling and simulation in the framework of one generalized model of the above mentioned effects, it is entirely reasonable to study them separately. A model based on the slippage effect, is presented in this paper. This type of strength formation is peculiar to fibrous materials with a low value of twist, like roving, sliver etc. In such fibrous materials, the friction and traction forces between the fibers are less than the fiber strength, so the fibrous material tensile strength is determined only by these forces.
It should be noted that a separate study of the slippage effect and the fiber breakage effect, which is very effective and reasonable on the stages of modeling and simulation, is not realizable practically during the stage of experimental studies. Simultaneous development and study of the model based on both effects seems to be an almost impossible task. However, in nature both effects occur simultaneously and their separation is not feasible. Thereby, experimental testing and calibration will be possible only on the basis of the mixed model.
The combined discrete event and agent based model of the ODFM tensile strength examines three types of fiber/agent exchanges which take place in every cross-section of ODFM: exchange between the fibers (F/F exchange), exchange between the fiber and a hole within the cross-section (F/H exchange), and the substitution of the hole by a new fiber (NF/H exchange) [21] . The concept of the fiber/agent activity in the exchange process has been used to model these three types of exchanges. This activity can be interpreted as an individual exchange probability for the fiber or hole and can be represented as a specific characteristic with uniform distribution on interval (0, 1). The fiber exchange algorithm actually reproduces the migration process within the structure of the ODFM with the only difference that the initial fiber shape is represented as a line segment. The simulation of the migration of fibers is a side effect of the simulation of the fibers interaction in the framework of the agent-based model associated with the slippage effect. The model provides a calculation of the following indicators of migration: Mean Fiber Position, Mean Migration Intensity, Migration Frequency, and Migration Factor.
An application of the concept of the fiber/hole activity in conjunction with a number of supplementary

Figure 1. Classification of broken end structures: (a) sharp broken end, (b) tapered broken end, (c) slipped broken end [28] .
conditions is the basis for the modeling of all three types of exchange in the cross-section of a fibrous material.
The strength of the ODFM, in the framework of the slippage model, primary depends on the friction forces within the structure of the ODFM, which arise in turn due to contacts between the fibers. The length of contact, without taking into account the pressing force between the fibers, provides only a limited view of the strength of the ODFM. In this sense, the contact length can be interpreted as the output of zero-order models. A minimal value of the total contact length from both sides of the cross-section is the strength characteristic in the frame of this zero-order model. The first-order model specification is associated with the friction between the fibers. The slippage effect occurs when the tension force exceeds the force of static friction.
Such approach to the problem allows modeling not only cross-section tensile strength but also segment- strength tensile strength [16] that is the minimum value of the function of the cross-section strength over the length of the segment.
2. The Validation Problem of the Slippage Effect Based Model
The complex structure of the strength model and the large number of its parameters affecting the behavior and responses of the model, make it necessary to check the consistency of the model responses to variation of its parameters. This model consistency checking is known as the validation problem in the field of computer simulation [22] [29] . Though the model validation takes on many forms, the main goal of this stage is to make sure that the model has a property, which is called “face validity”. “If the simulation results are consistent with the perceived system behavior, then the model is said to have face validity” ( [22] , p. 281). Within the framework of the face validity test, it seems reasonable to freeze the initial state (working point), vary the model parameters alternately, and observe and analyze the model behavior. This model behavior, manifested in its responses to the change of the parameters (number of contacts between fibers, packing density, number of holes, dimension of cross-section etc.) should not contradict the ideas about the mechanism of the strength formation, which were laid down in the development of the model. All results obtained with these face validity tests should be explainable in terms of physical concepts about the fibrous material strength.
As noted above, calibration and adjusting of the slippage effect based strength model is extremely complicated because the slippage effect and the effects of fiber breaking exist simultaneously and are practically inseparable [21] . Furthermore, Standard Test Methods [30] do not allow distinguishing the slippage and fiber break effects.
The mixed C-model is an integration of these two types of strength models. The face validity testing is a sine qua non of such integration. This test should include checking of the integrated models with marginal (boundary) values of the parameters. In its turn, “marginal tests” allow estimating the potential of the strength model and the relative level of effectiveness of the structure of the fibrous material. In this connection, the problem arises of estimating the maximum allowable strength values (strength potential) of the zero and first order slippage effect based models [21] .
2.1. Marginal Values of the Model Parameters
The most significant parameters of the model, which determine the exchange processes of types F/F and F/H, are the critical value of the fiber exchange activity―CA, the fiber exchange threshold―CT, and the critical value of the hole activity―HA [21] . As follows from the description of the modeling algorithm, there are marginal values of the basic parameters that correspond to a specific exchange condition. The data describing the relationship between the rate of exchanges of types F/F and F/H, on one hand, and the marginal values of the basic parameters of the exchange processes, on the other hand, are presented in Table 1. In this table, the value “max” of the exchange intensity corresponds to the maximum possible exchange intensity when removing all restrictions from the fibers participating in the exchange processes. The value “0” means there is no exchange.
This table is based on the representations about the exchange mechanism, which were laid down in the development of the model, and was used as the basis for testing face validity of the tensile strength model [21] .
Prior to testing the strength model, simulation of the ODFM with the following parameter values was carried out: normal distribution of the fiber length with average fiber length (FLmean) of 30 mm and standard deviation (Fstd) of 3 mm; exponential distribution of the fiber front ends with the average distance between these ends (TB) of 1 mm. These parameters of the ODFM model have been used as a basic set of parameters for testing and validation of the ODFM model [26] . The same ODFM model was used for the tensile strength model validation. A file containing the coordinates of the front and back ends of 5000 fibers within a 5000 mm specimen length
Table 1. Exchange rate for marginal values of the model parameters.
has been obtained as a result of the ODFM simulation.
2.2. Effect of the Fiber Exchange Threshold
As noted above, inclusion of the fiber exchange mechanism into the ODFM model allows transforming the one- dimensional model of the fiber flow to a three-dimensional model of the tensile strength. This transformation is absolutely required because in the framework of the one-dimensional model it is absolutely impossible to describe and simulate various effects of fiber interaction. In the framework of the three-dimensional model the fiber has a random shape (crimp fiber). This random fiber shape is presented here as a result of the fiber exchange processes. The random fiber shape determines traction the forces between the fibers, which together with the frictional forces between the fibers in its turn determine the tensile strength. The behavior of the fibers/ agents in the exchange processes depends on its individual values of the activity parameters, critical values of the fiber exchange activity―CA and the hole exchange activity―HA (holes in the cross-section which are replaced by fibers are considered as agents), and the fiber exchange threshold―CT [21] . The fiber exchange occurs when a difference of the contacting fibers activities exceeds the fiber exchange threshold. This threshold in substance is the global parameter of the ODFM’s tensile strength model but not parameter of the individual fiber/agent.
A series of experiments on the model have been carried out in order to face validate the model behavior and its responses. It was assumed that every fiber and hole in each cross-section of ODFM can participate in the exchange processes. An excess of the fiber exchange threshold CT was a necessary condition of the fiber and holes exchanges. The fiber exchange threshold has been selected as the criterion for face validation, as it is a key parameter which determines the intensity of both types of exchange―the fiber/fiber exchange and the fiber/hole exchange. In the series of tests (Table 2) the threshold CT has been varied over the entire range of possible values (0 - 1) with increments of 0.1.The values of thresholds of the fibers and holes activity were constant (CA = 0 and HA = 0). The data shown in Table 2 was obtained by averaging the results of 10 consecutive computer simulations in accordance with the conclusions of the statistical analysis presented in [21] .
Table 2 contains the values of the total number of exchanges (TNE), average number of the exchanges per fiber―AEPF, relative average number of exchanges per fiber―RAEPF (RAEPF = AEPF/FLmean), total number of holes (TNH), and average number of holes per cross-section―AHPCS (AHPCS = TNH/NCS = TNH/4800). The transient processes in the “heating” and “cooling” phases of the ODFMs simulation model were excluded from the data. The length of the transition process was determined as a result of preliminary runs of the ODFMs model and was evaluated as100 mm (cross-sections). This duration of the transition process is on the order of three average fiber lengths and is characteristic for the linear dynamic systems of the first order. After exclusion of the transient processes from the simulation data, the active part of the simulated ODFMcontains4800 cross-sections.
The results shown in Table 2 are fully consistent with the expected model responses. When СТ = 0, there are no restrictions on the fiber and hole activities and the number of exchanges of the types F/F and F/H is at a maximum (TNE = 123848) and consequently the number of the holes is at a minimum (TNH = 12933).
With increase of the fiber exchange threshold, a percentage of fibers which participate in the exchange process decreases. The relative number of exchanges per fiber per cross-section (RAEPF = AEPF/(FLmean-1)) (Figure 2), and the total number of exchange of the both types decrease and, therefore, the total number of holes and the average number of holes per cross-section increase (Figure 3). This behavior of the simulation model responses
Figure 2. Decrease in the relative number of exchanges per fiber per cross- section (RAEPF) with increasing the fiber change threshold (CT).
Figure 3. Increase in the average number of holes per cross-section (AHPCS) on reducing the fiber activity by increasing in the fiber change threshold (CT).
Table 2. Impact of fiber exchange threshold (CA = HA = 0).
on variation of the fiber exchange threshold indicates consistency between the simulation algorithm and the simulation software implementation.
In addition to the model responses, Table 2 contains theoretical values of the exchange probability PrE for different values of CT. These values of the exchange probability were obtained by analyzing the distribution of the difference between the fiber activities 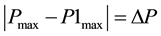 with the uniform distribution of the fiber exchange activity on the interval (0,1). It can be shown that this distribution is a special case of Simpson’s distribution [31] :
with the uniform distribution of the fiber exchange activity on the interval (0,1). It can be shown that this distribution is a special case of Simpson’s distribution [31] :
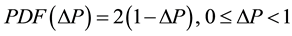 (1)
(1)
The probability of exchange at a given value of the fiber exchange threshold CT is:
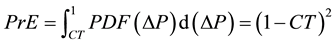 (2)
(2)
Over the whole range of the fiber exchange threshold the simulation results are less than the estimated theoretical values. This fact, in our view, it does not refute the validity of the model but it is associated with the following circumstances.
Fibers at the periphery of the cross-section have less than six fibers in their environment. Consequently, some of the peripheral fibers do not find a “partner” for the mutual exchange and are not involved in the exchange process seven if CA = HA = CT = 0. This leads to a reduction in the relative number of exchanges per fiber per cross-section―RAEPF value compared to the theoretical values of the exchange probability―PrE.
Besides, there may be a situation where some of the fibers” do not find a partner” for the mutual exchange within the internal layers of the cross-section. The probability of this situation increases with the increase in the exchange intensity, which arises with the decrease of the fiber exchange threshold. This explains the increase in the difference between the relative numbers of exchanges per fiber per cross-section and the exchange probability values for the low values of the fiber exchange threshold that were calculated according to Equation 2 (Figure 4).
Figures 5-7 demonstrate the impact that the fiber exchange threshold has on the transformation of the one- dimensional model of ODFM to the three-dimensional model of tensile strength. These figures present three- dimensional images of fibers in a randomly selected cross-section in the interval (100, 4800) of the tensile strength model (images are shown in Cartesian, not in hexagonal lattice, coordinates).
Figure 5 presents a situation when all restrictions on the fibers and holes activities were canceled but the limit value of the fiber exchange threshold (CT = 1) does not permit any type of exchanges. When we study the irregularity of the ODFM, such a model can be regarded as one-dimensional [26] . But, in the framework of the zero-order tensile strength model it is necessary to calculate the total number and length of contacts between fibers, and this model should be classified and studied as three dimensional.
Figure 4. Difference between the theoretical PrE and simulation values of the exchange probability (AEPCS) as a function of the fiber exchange threshold (CT).
Figure 5. Dependence of the total number of exchanges of all types (TNE) on the critical value of the fiber exchange activity (CA).
Figure 6. Total number of fiber exchanges TNE and the relative number of exchanges per fiber RAEPF vs. the fiber exchange threshold CT and the different critical values of the fiber exchange activity CA.
This situation changes significantly with the decrease in the value of the fiber exchange threshold (Figure 6). According to the simulation results, which are shown in Table 2, a statistical estimation of the exchange probability of both types equals 0.129. It is absolutely clear that, in addition to the frictional forces, traction forces arise between fibers in this case.
The limiting case, with no restrictions on the mutual F/F and F/H exchanges, occurs when the critical values of the fiber and hole exchange activities, and the fiber exchange threshold equals zero (Figure 7).
It should be noted that the values of the fiber exchange threshold cannot be obtained experimentally, as this parameter, as well as parameters CA and HA, are virtual parameters of the strength model which describe the behavior of the single fiber in the fiber population and its interaction with other fibers in its own surrounding. However, unlike the parameters of crimped fibers which are available for observation and measurement [32] [33] , these parameters allow us to implement a virtual probabilistic model of the flow of crimped fibers and ultimately allow simulation of the tensile strength.
Some estimation of the fiber exchange threshold value can be obtained during the model calibration by a visual comparison between a series of crimped fiber images and corresponding images in hexagonal coordinates obtained as a result of computer simulation. Here we need to note that the hexagonal coordinates for simulatingagent interactions was used in studying conflicts on the basis of agent based simulation [34] . This possibility to study conflicts on the level of individual agent interaction has been described previously [21] .
Figure 7. Dependence of the average number of holes per cross-section (AHPCS) on the critical value of the hole activity (CA = CT = 0.3).
2.3. Effect of the Critical Value of the Fiber Exchange Activity
Traction forces arise between fibers as a result of the exchange processes. These forces depend on that part of the fibers which potentially can be involved in the exchange processes. This part is determined by critical values of the fiber and hole exchange activity CA and HA, which have been excluded from the face validation process at the previous stage. The fiber exchange thresholdand the critical values of the fiber and holes exchange activity are associated with nonlinear effects in the tensile strength model (signum effect). However, it can be assumed that in the case when the number of F/F exchanges substantially exceeds the number of F/H exchanges, increasing the critical value of the fiber activity will cause a linear decrease of the total number of exchanges TNE. This assumption is based on the fact that variation of the critical value of the fiber exchange activity leads to a linear change in the number of fibers that can take a part in the exchange processes. A test of this assumption is one of the stages of the face validation of the tensile strength model.
Results of experiments in order to analyze the impact critical value of the fiber activity are shown in Table 3. The results which were obtained for the fixed values of the fiber exchange threshold (CT = 0.3) and the hole activity (HA = 0) demonstrate the impact of the critical value of the fiber exchange activity CA on both types of exchange. The number of holes TNH, as follows from Table 3, is independent of the critical value of the fiber exchange activity; and this is fully consistent with the type F/H exchange algorithm. The values of TNE, AEPF, and RAEPF for CA > 0.6 characterize only exchanges of type F/H, since they correspond to the case when the exchange of type F/F is forbidden by violating the condition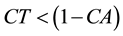 .
.
Figure 5 shows a graph of the total number of exchanges with increasing the critical value of the fiber exchange activity CA for a fixed level of the fiber exchange threshold (CT = 0.3). This graph confirms the assumption of linear dependence of the total number of exchanges TNE on the value of the fiber exchange activity in the area of permitted exchanges of type F/F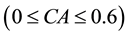 . The range of values
. The range of values 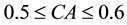 can be defined as the transition area, as the number of exchanges of type F/F in this area is comparable with the number of exchanges of type F/H.
can be defined as the transition area, as the number of exchanges of type F/F in this area is comparable with the number of exchanges of type F/H.
Table 3 also presents the theoretical values of the type F/F exchanges probability―PrE. In a general case, for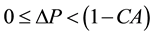 , the following expression for the distribution function of an absolute difference
, the following expression for the distribution function of an absolute difference 

In the special case of СА = 0, this expression leads to the distribution Equation (1).
The probability of the exchange of type F/F is:
Figure 8. Dependence of the fiber packing density (PDF) on holes per cross- section on the critical value of the hole activity (CA = CT = 0.3).
Table 3. Impact of critical value of the fiber exchange activity.

The values of PrE that are calculated according to Equation 4 exceed the values of the average number of exchanges of types F/F and F/H per cross-section A EPCS, which have been obtained by computer simulation. This difference between the theoretical and experimental values of the exchange probability (PrE > AEPCS) is explained with the above-described effect of “loss of exchange partner”, which arises by exchanges at the periphery of the cross-section.
2.4. Joint Effect of the Fiber and Holes Exchange Parameters
The results presented above give us reason to conclude that the tested tensile strength model meets the requirements of face validation and its responses are logical and explainable on the physical level. Virtual model parameters, such as CT and CA, in our view adequately characterize the mechanisms of strength formation associated with the slippage effect. However, these results were received with independent variation of each the model parameters. Joint parameter variation, in principle, can lead to inconsistent results, because the model is based on stochastic processes of the fiber and hole exchanges, which are complicated by nonlinear effects. To verify the consistency of the model under the joint variation of the key parameters of the exchange processes, and to obtain more comprehensive information about the properties of the tensile strength model, a series of computer simulation experiments has been carried out.
Two basic parameters of the fiber exchange process―the critical value of the fiber exchange activities CA and the fiber exchange threshold CT were varied in this series of experiments. The condition CT < (1 − CA) was imposed on all experiments; hence all three types of fiber exchange have been realized. When this condition does not hold, an exchange of type F/F is impossible. The critical value of the hole exchange activity was set equal to zero in all experiments; thus a maximum number of holes has been involved in the exchange processes. The number of simulated cross-sections was equal to 5000, with a step between cross-sections of 1mm. The parameters of the simulated fiber flow were: the fiber length distribution is normal, average fiber length equals 30 mm, standard deviation of the fiber length equals 3 mm, distribution of the distance between the fiber front ends is Poisson, and the average distance between the fiber front ends equals 1mm. So the total number of fibers simulated by the tensile strength model equals 5000 and the average number of fibers in the cross-section equals 30. In order to eliminate transient effects the first and the last 100 cross-sections of the simulated ODFM were excluded from consideration, and the effective number of the cross-sections was therefore 4800.
The dependence of the total number of all exchange types TNE, and the relative number of exchanges per fiber RAEPF, on the varied parameters CA and CT is shown in Figure 6.
As noted above, the effect of fiber traction, which is determined by the crimp coefficient kc, is very important for tensile strength modeling. The crimp coefficient is equal to the ratio of the number of the fiber exchanges to the fiber length, and coincides with the relative average number of exchanges per fiber―RAEPF. It is natural that the graphs of RAEPF obtained as a result of the simulation of fiber random shape and the graphs of the total number of fiber exchanges TNE are identical.
The fiber packing density (FPD) in the cross-section is another important characteristic significantly affecting tensile strength. The fiber packing density can be represented as the number of holes in the cross-section of ODFM. The simulation algorithm determines an unfilled node of the hexagonal lattice as a hole when the concentric hexagon layer of this node has more than two fibers. According to the simulation algorithm F/H exchange occurs when the condition PD > HA is satisfied. An increase in the critical value of the hole activity should lead to a decrease in the frequency of the F/H exchanges and consequently to an increase in the number of holes and a decrease in the fiber packing density. These conclusions are confirmed by the simulation results shown in Figure 7 and Figure 8.
On the other hand, the number of holes and the fiber packing density depends on parameters of the exchange process of type F/H. Figure 9 shows the dependence of the number of holes on the parameters of the fiber exchange activity for the fixed critical value of the hole activity (HA = 0).
The strength of the zero-order model―ST0has been calculated in accordance with the results of the computer simulation. The dependence of the normalized strength of the zero-order model on the parameters of the fiber exchange processes for a fixed critical value of the hole activity (HA = 0) is shown in Figure 10. The results demonstrate the decrease of the zero-order model tensile strength with the increase in the parameters of the exchange process CA and CT relative to the corresponding tensile strength of the completely filled closed-packed hexagonal cross-section.
3. Normalization Procedure and Potential of the Tensile Strength Model
As noted above, the tensile strength model, which is based on the slippage effect, can be calibrated only in conjunction with a model based on the fiber breakage effect. The lack of opportunity of separate calibration of the tensile strength model prevents both realizing a statistical validation [22] of the model, and also the use of natural variables for the model output. However, the model allows calculation of the maximum values of the output variables and in this way determination of the potential of the model. The normalization of the tensile strength model relative to the maximum values of the output variables makes it possible to evaluate the degree of utilization of this potential for various levels of the model parameters. Practically, the normalization procedure is realized by dividing the model outputs that are the total length of contacts-TCL and total frictional/traction forces―TFTF by the maximum possible TCLM and TFTFM for the given fiber structure.
Such estimations of the “relative strength” have been obtained for hexagonal fiber packing as result of structure analysis and computer simulation.
Figure 9. Dependence of the total number of holes (TNH) and the average number of holes per cross-section (AHPCS) on the parameters of the fiber exchange activity for a fixed critical value of the hole activity (HA = 0).
Figure 10. Dependence of the normalized strength of the zero-order model on the parameters of fiber exchange activity for the fixed critical value of the hole activity (HA = 0).
3.1. Maximum Value of the Total Length of Contacts
The maximum value of the total length of contacts in the cross-section (TCLM) can be calculated as a product of the maximum number of contacts in the cross-section of the close-packed hexagonal structure by the average fiber length. In a close-packed hexagonal structure, all fibers touch each other [35] . It can be shown that the number of fibers in the concentric hexagon layer of the order r (r-CHL) of the center of the cross-section is 

We separate two types of contact between fibers-external contacts with fibers in the CHL of order (r-1) andinternal contact sin the CHL of order r. A simple analysis of the hexagonal cross-section shows that the number of external contacts between the fibers of the r-CHL is:

The number of internal contacts between the fibers of the r-CHL is:

It follows that the total number of contacts in the close-packed hexagonal structure of order R is:

In the case where the external environment of order R (R-CHL) contains less than 6R fibers, we define an average number of the contacts per fiber in the CHL of order R:

The number of contacts created by 

Thus, the maximum number of contacts produced in the cross-section of order R if R-CHL is not completelyfilled is:

Table 4 shows the number of fibers and the number of contacts between fibers for the cross-sectionsup to 10 orders of magnitude.
The graphs in Figure 11 and Figure 12 demonstrate the dependence of the average number of contacts per fiber in the partially filled R-CHL

The maximum possible value of the total contact length in the cross-section―TCLM equals the product of the total number of contacts in the close-packed cross-section 

The normalized value of the total contact length in the cross-section is:

For example, if FLmean = 60 mm, Fstd = 3 mm, TB = 3 mm, CT = CA = 0.5, and HA = 0, then computer simulation produces the value of the total length of contacts in cross-section of TCL = 7445 mm. According to Table 4, this case corresponds to the four partially filled layers (lack of a single fiber in the fourth layer). It follows that
Table 4. The number of fibers and number of contacts vs. order of cross-section.
Figure 11. Dependence of the average number of contacts per fiber in the partially filled R-CHL (
Figure 12. Dependence of the total number of contacts 
the total number of contacts in the cross-section equals 153, and the maximal possible value of the total length of contacts is TCLM = 153 * 60 = 9180 mm. The normalized value 
3.2. Maximal Value of the Total Frictional/Traction Forces
As shown above, at every individual contact between the fibers, a force arises that prevents a mutual displacement of the fibers―

The distance coefficient―k does not depend on the number of contacts and their location within the cross- section. Therefore, in the analysis of the relationship between the actual and the maximum possible value 
When calculating the TFTFM in every CHL, only the external contacts with the underlying layer and the contacts within the layer need to be taken into account. In the external R-CHL only the contact with the (R-1) layer should be considered, as only these contacts of the exterior layer contribute to the total force off riction between the fibers in the cross-section.
Table 5 and Figure 13 show the values of the maximum of the friction/traction force (FTFMr) for the separated CHL which have been obtained by the analysis of the close-packed hexagonal cross-sections of order R

The last row of Table 4 presents the values of FTFMΣ which should be used for the normalization of the tensile strength model.
The graphs in Figure 13 reflect two different trends. On the one hand, it shows the increase in the friction/traction forces associated with increasing the number of contacts in the layer away from the center of the cross-section (linear effect). On the other hand, it shows the reduction in the tensile strength caused by approaching the periphery of the cross-sections (quadratic effect). The first tendency prevails near the center of the cross-section. However, as it approaches the periphery of the cross-section, the second trend compensates the effect of increasing the number of contacts in the peak point of the graph. In the last R-layer the forces opposing the mutual displacement of the fibers arise only as a consequence of internal contacts in the underlying (R − 1) CHL.
The dependence of the maximum of the frictional/traction forces-FTFMΣ on the close-packed hexagonal cross-section of order―R is shown in Figure 14.
Figure 13. Dependence of the maximal Friction/Traction Force (FTFMr) on the CHL order―r for close-packed hexagonal cross-sections of order-R.
Table 5. The maximal frictional/traction forces for a close-packed hexagonal cross-section.
Figure 14. Dependence of the maximum of the frictional/traction forces (FTFMΣ) on the cross-section order R.
The maximum possible value of the total frictional/traction forces for the fibers belonging to the cross-section equals the product of the maximum value of the frictional/traction forces (FTFMΣ) and the average fiber length:

The normalized values of the total frictional/traction forces in the cross-section of ODFM:


It is quite obvious that the relative estimates of the degree of utilization of the strength potential will be correct at any value of the strength model parameters, which can be obtained by the model calibration.
In the case where there is an additional, incomplete CHL of the order (R + 1), the value of FTFMΣ from Table 5 needs to be added to the friction forces that arise between the layers R and (R + 1). This additional component equals the product of the number of fibers 

The data shown in Table 6, allows us to determine the cross-section order and its basic parameters for values of the average number of fibers in the cross-section in the range (30 (10) 300).
The data presented in Table 6 allows us to determine the cross-section parameters and evaluate the potential of the first order tensile strength model considering the frictional and traction forces between the fibers.
4. Conclusion
The behavior and main responses of the fibrous material tensile strength model, which is based on the fiber slippage effect, are studied with the goal of face validation. Specific features of this model are the principles underlying in its foundation. The concept of discrete event simulation has been used for the modeling and simulation of the fiber flow. This approach in the framework of the given research allows taking into account the fiber length distribution and the random fiber location along a one-dimensional fibrous material. The three dimensional model of the tensile strength that is based on the concept of agent based modeling is the expansion of the fiber flow model. The specific technique of face validation, that is elaborated and widely used in the field of computer aided simulation, has been used to study the tensile strength model. The effects of the individual and joint variation of the fiber exchange parameters and the influence of these parameters on the contacts between fibers and frictional and traction forces which arise as a consequence of this contacts have been investigated in the framework of face validation. The face validation results show a consistent predictability of the model responses in the full range of the fiber exchange parameters. Furthermore, the possibility and efficiency of the crimp fiber simulation, based on the probability and nonlinear exchange mechanisms between fibers in the
Table 6. Cross-section order and basic parameters.
cross-sectional of the one-dimensional fibrous materials, is confirmed.
Estimations of the maximum possible values of the total length and frictional/traction forces for the close- packed hexagonal structure of the fibrous material cross-section have been obtained. These estimations are the basis for the normalization of the tensile strength model. The normalized model, in its turn, allows determining and calculating the tensile strength potential considering the total contact length and total frictional/traction forces.
The development of a tensile strength model, taking into account the effect of fiber breakage, and a generalized model based on the fiber slippage and fiber breakage effects are the aims of further research in the field of combined modeling and simulation of fibrous materials tensile strength. This combined model should be developed as an expansion of the slippage effect based tensile strength model presented and validated in this paper. Calibration and statistical validation based on experimental data will be carried out in the testing stage of the mixed slippage and fiber breaks model.
References
- Xie, Y.O. (1986) A Study of the Strength of Wrapped Yarns. Part III: The Relationship between Structural Parameters and Strength. Journal of the Textile Institute, 5, 314-326.
- Ghosh, A.I. (2005) Predictive Models for Strength of Spun Yarns: An Overview. Autex Research Journal, 5, 20-29.
- Furferi, R. and Gelli, M. (2010) Yarn Strength Prediction: A Practical Model Based on Artificial Neural Networks. Ad- vances in Mechanical Engineering, 2010, Article ID: 640103.
- Majumbar, A. (2008) Yarn Strength Modeling Using Fuzzy Expert System. Journal of Engineered Fibers and Fabrics, 3, 61-68.
- Hearle, J.G., Grosberg, P. and Backer, S. (1969) Structural Mechanics of Fibers, Yarns and Fabrics. Wiley-Interscience, New York, 482.
- Sullivan, R.R. (1942) A Theoretical Approach to the Problem of Yarn Strength. Journal of Applied Physics, 13, 157- 167. http://dx.doi.org/10.1063/1.1714849
- Grossberg, P. (1966) The Strength of Slivers of Relatively Low Twist. Journal of the Textile Institute Transactions, 57, T15-T23. http://dx.doi.org/10.1080/19447026608662332
- Koo, H.S. (2001) Variance Tolerancing and Decomposition in Short-Staple Spinning Process. Part 1: Modeling Spun Yarn Strength through Intrinsic Components. Textile Research Journal, 71, 1-7.
- Wang, Y.S. (2001) Digital-Element Simulation of Textile Processes. Composites Science and Technology, 61, 311-319. http://dx.doi.org/10.1016/S0266-3538(00)00223-2
- Vas, L.H. (1994) Modelling the Breaking Process of Twisted Fibre Bundles and Yarns. Periodica Polytechnica, Ser. Mechanical Engineering, 38, 325-350.
- Phoenix, S.L. (1979) Statistical Theory for the Strength of Twisted Fiber Bundles with Applications to Yarns and Cables. Textile Research Journal, 49, 407-423. http://dx.doi.org/10.1177/004051757904900708
- Djaja, R.M. (1992) Finite Element Modelling for an Oriented Assembly of Continuous Fibers. Textile Research Journal, 62, 445-457.
- Keefe, M. (1994) Solid Modeling Applied to Fibrous Assemblies. Part 1: Twisted Yarn. Textile Research Journal, 85, 338-349. http://dx.doi.org/10.1080/00405009408631278
- Langenhove, L. (1997) Simulating the Mechanical Properties of a Yarn Based on the Properties and Arrangement of Its Fibers. Part 1: The Finite Element Model. Textile Research Journal, 67, 263-268.
- Morris, P.M. (1999) Modelling of Yarn Properties from Fiber Properties. Journal of the Textile Institute, 90, 322-335.
- Porwal, P.B. (2007) Statistical Strength of Twisted Fiber Bundles with Load Sharing Controlled by Frictional Length Scales. Journal of Mechanics of Materials and Structures, 2, 773-791. http://dx.doi.org/10.2140/jomms.2007.2.773
- Siewe, F.G. (2009) An Application of Queuing Theory to Modeling of Melange Yarns. Part 1: A Queuing Model of Melange Yarn Structure. Textile Research Journal, 79, 1467-1485. http://dx.doi.org/10.1177/0040517508095593
- Pan, N. (1998) Investigation on the Strength-Size Relationship in Fibrous Structures Including Composites. Journal of Materials Science, 33, 2667-2672. http://dx.doi.org/10.1023/A:1004325907536
- Vishwanath, B.V., Vernekar, S. and Ghosh, B (1999) Effect of Fiber Friction on Yarn Properties. Man-Made Text India, 7, 267-271.
- Das, A., Ishtiaque, S.M. and Parida, J.R. (2005) Effect of Fiber Friction, Yarn Twist, and Splicing Air Pressure on Yarn Splicing Performance. Fibers and Polymers, 6, 72-78. http://dx.doi.org/10.1007/BF02875576
- Cherkassky, A. and Bumagin, E. (2014) A Combined Discrete Event―Agent Based Approach to Modeling Tensile Strength of One-Dimensional Fibrous Materials. Part 1: Simulation Algorithm on the Base of the Fiber Slippage Effect. Journal of Engineered Fibers and Fabrics, 9, 1-15.
- Law, A.K. and Kelton, W.D. (2000) Simulation Modeling and Analysis. 3rd Edition, McGraw-Hill, Singapore, 760.
- Borshchev, A. (2013) The Big Book of Simulation Modeling. AnyLogic, America, 612.
- Van Dam, K.H., Nikolic, I. and Lukszo, Z. (2013) Agent-Based Modelling of Socio-Technical Systems. Springer, Berlin, 267.
- Allen, T.T. (2011) Introduction to Discrete Event Simulation and Agent-Based Modeling: Voting Systems, Health Care, Military, and Manufacturing. Springer, London, 215. http://dx.doi.org/10.1007/978-0-85729-139-4
- Cherkassky, A. (2010) Neural Network Meta-Model of the Fibrous Materials Based on Discrete-Event Simulation. Part 1: Discrete-Event Simulation Model of the One-Dimensional Fibrous Material. Journal of The Textile Institute, 102, 442-454. http://dx.doi.org/10.1080/00405000.2010.488078
- Das, B.R., Ishtiaque, S.M. and Rengasamy, R.S. (2010) Failure Mechanism of Staple Yarns: A Critical Review. Journal of Textile and Apparel, Technology and Management, 6, 1-10.
- Ishtiaque, S.M.., Das, B.R.., Kumar, A. and Ramamoorthy, M. (2008) Static and Dynamic Failure Mechanisms of Cot- ton Yarns. Indian Journal of Fibre Textile Research, 33, 111-118.
- Chung, C.A. (2003) Simulation Modeling. CRC Press, Boca Raton, 608.
- American Society for Testing and Materials (ASTM) Standard (2006) Standard Test Method for Breaking Force and Elongation of Textile Fabrics. ASTM D, 5035-06, 274-279.
- Evans, M.H. (2010) Triangular Distribution. In: Forbes, C., Evans, M., Hasting, N., et al., Eds., Statistical Distributions, 4th Edition, Wiley, New York, 210.
- Brand, R.H. (1970) Measurement of Three-Dimensional Fiber Crimp. Textile Research Journal, 40, 169-177. http://dx.doi.org/10.1177/004051757004000210
- American Society for Testing and Materials (ASTM) Standard (2012) Standard Test Method for Crimp Frequency of Manufactured Staple Fibers, ASTM D, 3937-12, 256-259.
- Sabin, P. (2014) Simulating War. Bloomsbury, London, New Delhi, New York and Sydney, 363.
- Sreprateep, K.B. (2006) Computer Aids Modeling of Fiber Assemblies. Computer-Aided Design and Applications, 3, 367-376. http://dx.doi.org/10.1080/16864360.2006.10738475
Notation
AEPCS: Average number of exchanges of the types F/F and F/H per cross-section,
AEPF: Average number of exchanges of the types F/F and F/H per fiber,
AHPCS: Average number of holes per cross-section,
CA: Critical value of fiber exchange activity,
(CHL): Concentric hexagon layer,
CSS: Cross-section strength,
CT: Fiber exchange threshold,
CV: Coefficient of variation,
d: Diameter of fiber,

FLmean: Average fiber length,
HA: Critical value of hole exchange activity,
i: Cross-section number,
j: Fiber number,
: Distance coefficient,


N: Normal pressureforceper unitlength of the fiber,

NCS: Number of cross-sections (length of replication),
(ODFM): One-dimensional fibrous material,
(PDF): Probability density function,
PrE: Exchange probability,
r: Radius of contact (number of concentric hexagon layer),
R: Radius of cross-section (order of close-packed cross-section),
RAEPF: Relative average number of exchanges per fiber per cross-section,
RSE: Relative standard error,
s: Distance between adjacent cross-sections (simulation step),
SE: Standard error,

TCL: Total length of contacts in cross-section,


TCLM: Limit value (maximal possible value) of total length of contacts,
TFTF: Total frictional/traction forces for cross-section―i,



TFTFM: Limit value (maximal possible value) of total frictional/traction forces,
TNE: Total number of exchanges,
TNF: Total number of fibers,
TNH: Total number of holes,

(AAA): Acronym, abbreviation.
NOTES
*Corresponding author.
















