Received 16 March 2015; accepted 11 April 2016; published 14 April 2016

ABSTRACT

The flow of blood continues to arouse much interest among researchers in rheology, fluid mechanics and medicine. Existing models of the apparent viscosity versus shear stress are numerous, and we have chosen to discuss the Carreau-Gambaruto model and the Quemada model. The comparison between models and viscosity measurements shows discordance for shear rates below a few tenths of s⁻¹. The existence of an inflection point on the experimental curve is probably related to a system relaxation due to the rupture of red blood cells structure named rouleaux. This work suggests us to adapt these models for the weak shear rates.

Keywords:

Blood, Rheology, Viscosity, Model, Viscoelasticity

1. Introduction

Blood is a complex fluid as non-Newtonian. Its flow laws do not obey the laws of simple fluids described by the Navier-Stokes equations. The laws of the blood flow continue to be processed by different research on the subject. The complexity of blood fluid is due to a nontrivial coupling between red blood cells (RBCs, the majority of blood components) that move and deform continuously, and the carrier fluid. The movement of blood cells in turn acts on the global flow of blood, and so on. It is essential to understand the movement of cells under different flows and geometries. Understanding of blood flow is essential for the elucidation of cardiovascular disease, the leading causes of death worldwide. This paper presents an overview of the laws of the rheological behavior of blood.

2. Blood and Fluid Flow

Blood is a complex fluid in perpetual renewal, which provides a number of essential functions for maintaining life. It consists of cells suspended in the plasma and accounts for about 7% of the body weight for an adult. Suspended particles are red blood cells (the majority), white blood cells, platelets, and other substances such as proteins, vitamins etc. The suspending liquid is plasma, aqueous solution of electrolytes and organic substances, mostly proteins.

Blood flows continuously into the vasculature of the body, under the impulse of the heart beat. Since the discovery of the large circulation attributed by historians to William Harvey in 1628 (the small pulmonary circulation is higher in Harvey), the Blood research has continued, bringing together several scientific specialties (anatomy, medicine, physiology, rheology…).

From the standpoint of the rheology, the blood is considered as a concentrated colloidal suspension of erythrocytes. The relationship between shear rate and apparent viscosity of a suspension of erythrocytes in plasma was studied by Shu Dog [1] . It was proved that if the shear rates are inferior to 10² s⁻¹, the blood has a rheofluid behavior, whereas outside this range it has Newtonian behavior. The interpretation of this rheological behavior is based on two processes:

・ aggregation of red blood cells at low shear rate, leading to high viscosity values.

・ deformation and orientation of the red cells at high shear rate, lead to low values of viscosity.

These two processes making the viscosity depending on the flow rate, are closely related to the properties of plasma and red blood cells. In addition to the applied shear rate, the apparent viscosity of blood depends on other important factors which are: the volume concentration of red blood cells (hematocrit or H_t), mechanical properties and plasma viscosity (itself a function of the fibrinogen concentration and albumin).

The varied geometry of the circulatory system (arteries, veins, capillaries…) gives rise to various flow regimes, themselves responsible for the structuring of erythrocytes according to the strength of the shear. Thus, the blood has viscoelastic and thixotropic properties as the shear rate less than a few s⁻¹. This is explained by the aggregation of red blood cells in “rouleaux”, manifested in transient flow regimes.

3. The Dynamic Models and Blood Viscosity

The blood viscosity depends on suspension components (plasma, particles) but also the diameter of the vessel and the walls of its deformability. Thus the red blood cell stacks are important at the large vessels and induce an increase in viscosity. In the capillary, the force of flow disaggregates the “rouleaux”, so the viscosity decreases. Red blood cells have the ability to individually align, and pass through the capillary whose diameter is less than the average diameter cells.

It is also recognized that the blood has a yield stress τ₀, below which the blood does not flow.

In the following, we limit ourselves to the blood flow in large vessels with H_t = 45%.

3.1. Models of the Viscosity Function

Analysis of the rheological behavior of blood, as for any non-Newtonian fluid, is based on the dependence between shear stress τ and shear rate γ. The viscosity of blood is generally normalized by plasma viscosity, and is then called relative viscosity. The relative viscosity of blood depends on the hematocrit and the tendency of red blood cells to aggregate/deform/move. A mathematical formulation of the viscosity should consider these parameters to be appropriate. The rheological models (empirical or semi empirical) that we will study are able to adjust the results of experimental measurements over a wide range of shear rate with the same parameters.

To represent the dynamic viscosity of blood depending on the shear rate, we cite four models very present in the literature [2] [3] . It should be noted that these models already exist for non-Newtonian fluids in particular rheo-fluids but were either adapted or developed for blood.

Each model contains parameters identified as important to describe the rheological behavior of blood. We cite as examples of parameters: the yield stress, the Newtonian limiting viscosity and the molecular composition of the blood.

3.1.1. Cross Model

The relation 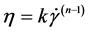or power model is valid only on a limited range of shear rates (without low and high shear rates). This led to a model with three parameters, proposed by Cross [4] and extending the power-law model to a wider range of shear rates:

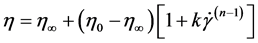(1)

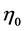and 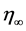and are respectively the viscosity of the blood when 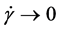and, k is a parameter dependent on blood components and n is the rheo-fluid exponent.

Walburn-Schneck Model

This power-law model with two parameters has been proposed to coincide with the experimental curve obtained from a blood sample for which hematocrit and chemical composition were known. There is a statistical correlation between the three most influential parameters on the fluid (shear rate, hematocrit, proteins concentration in plasma) [5] . The apparent viscosity is:

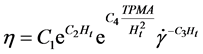(2)

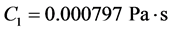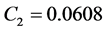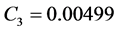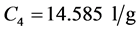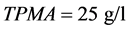, , , , (Protein concentration without albumin in a normal human blood)).

3.1.2. Carreau-Gambaruto Model

The following equation represents the Pierre Carreau Model, it gives the viscosity dependence on γ:

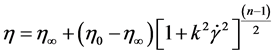(3)

k is the time relaxation constant.

If the shear rate is very low (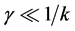), the fluid is Newtonian but if the shear rate is strong (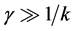), the fluid follows a power law. Suitable for blood, the Carreau model has several variants as the model of Carreau-Gambaruto [6] - [8] which has the parameters below:

3.1.3. Quemada Model

This model was developed by Quemada [9] to represent the apparent viscosity of the blood based on the shear rate and taking into account the hematocrit.

(4)

(plasma viscosity), k_∞ = 2.07, k₀ = 4.33, γ_c = 1.88 s⁻¹.

3.2. Comparison between Measured and Viscosity Models―Viscoelastic Behavior

If in the literature, many models are described or used, two models are cited as being more appropriate to describe the measured viscosity: Quemada model and Carreau-Gambaruto model.

Figure 1 shows a comparison between the two models and the measured viscosity [6] . The experimental values correspond to a blood of non-smoking healthy man of 56 years. The hematocrit is always considered equal to 45% for a healthy blood.

We note that the slopes do not coincide and the experimental curve has an inflection point.

Overall, the curve of the viscosity can be divided into three areas corresponding respectively to the low, the means and high shear rates. For the region of low (< 1 s⁻¹), normal RG form large aggregates. The term viscoelasticity is used for blood for the first time by GB Thurston [10] who explains that for this region, the viscoelastic properties are due to aggregation and not to deformability which is negligible. For medium as, the internal stress is sufficient to break the aggregates. With shear rate increasing, the disaggregated cells are gradually moving in the flow direction. In this region, the aggregation influence on the viscoelasticity decreases in favor of the deformability influence. The point of inflection between region 1 and region 2 is well explained by the phenomenon of relaxation of the blood (break of RG rouleaux)

When the shear rate reaches high levels, normal RG stretches or deforms and align with the flow. The blood forms RG layers stretched and sliding on plasma layers.

In terms of energy, viscosity is related to the dissipated energy in the flow due to the deformation and slip of

the RG and aggregates. Elasticity is due to the stored energy in the flow direction due to the deformation and the red blood cells.

Very recently, Brust et al. [11] proved by numerical simulation and by experience, the existence of a significant viscoelasticity of plasma to be taken into account.

4. Conclusions

The study of the blood rheological behavior is very important for understanding blood flow, which helps to detect and consequently to treat cardiovascular disease. Existing models of viscosity including models Carreau- Gambaruto and Quemada are approximate because it is difficult to introduce all the factors affecting the viscosity. Viscoelasticity of blood and the newly established viscoelasticity of plasma should bring more light on blood rheology.

The discontinuity (inflection point) on the experimental curve presents a notable difference with the models. We suggest that the models of the viscosity must consider the relaxation of the system as it is mentioned in the work of G.B. Thurston [10] . We also note that this study is based on one experimental measure of blood viscosity. We will work in the future on an averaged experimental viscosity.

Cite this paper

Zineb Mimouni, (2016) The Rheological Behavior of Human Blood—Comparison of Two Models. Open Journal of Biophysics,06,29-33. doi: 10.4236/ojbiphy.2016.62004

References