Open Journal of Inorganic Non-metallic Materials
Vol.06 No.04(2016), Article ID:70516,8 pages
10.4236/ojinm.2016.64005
Piezomodulation of Connection Conductance TlInTe2
Eldar M. Gojaev1, Sevda Kh. Agaeva2, Samire O. Kulieva1, Mahir T. Dadashov1
1Azerbaijan Technical University, Baku, Azerbaijan
2National Aviation Academy of Azerbaijan, Mardakan, Azerbaijan

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: May 27, 2016; Accepted: September 9, 2016; Published: September 12, 2016
ABSTRACT
This paper discusses the development method of strain sensors based on acicular single crystal compound TlInTe2 is grown by Bridgman-Stockbarger technique. Creating mechanically reliable ohmic contacts on said obtained single crystals was carried out directly by spot welding wires corresponding to the ends of the capacitor discharge heated in a stream of inert gas from the single crystal TlİnTe2. This method of creating contacts proved effective and reliable. Sensors made by this manner stuck to the calibrated beams of steel thickness of 1 mm, a length of 30 mm and a corresponding optimal regime got sensors with a maximum piezo-sensitivity. It was found that if enshrined at one end of a thin steel sheet with glued crystals TlİnTe2 initiate periodic mechanical vibrations to the same frequency, occurs conductivity modulation. The effect of piezo-modulation conductance depending on the degree of deformation, illumination in interval of deformation and lux illumination in room temperature were studied. The study showed that the more mechanical deformation and illumination are, the more modulation amplitude is. We studied the modulation of the conductivity of crystals during mechanical deformation values of 8, 14, 19, 23 and 27 × 10−5and illuminance 1000, 2750, 4750 and 6500 suites. Investigations the level piezo-signal depending on the amplitude of mechanical vibrations at frequency of 85 Hz, it is found that with increasing magnitude of the mechanical deformation of 7 × 10−5 and 26 × 10−5 amplitude increases six times. It is shown that the piezoresistive effect is clearly manifested in dynamic mode. Additional conductivity occurs during mechanical deformation. When the illuminated samples piezoconductivity magnitude increases linearly.
Keywords:
Electrical Conductivity, Compound TlInTe2, Piezosensitivity, Illumination, Mechanical Deformation, Piezomodulation

1. Introduction
The interest in semiconductor compound of АIIIВIIIХ2V types, being similar to АIIIВVI arises from the presence of unpaired number of valence electrons, sharp asymmetry of chemical bond, specific peculiarities of crystal lattice structure and the possibility to realize variation at wide range in chemical composition in the frame of lattice [1] [2] . Globally, a lot of attention is put on the research of fundamental properties of ternary compounds of above-mentioned types [3] - [11] . This is due to that receiver of near infrared radiation, switching devices, elements of electric and optical memory, laser modulation devices and other functional devices used in optoelectronics were developed on the basis of these materials and their solid solutions [12] - [14] .
The research of fundamental physical properties of semiconductors is important to obtain information on zone parameters, energy spectrum of charge carrier and their scattering mechanism in the crystal.
Since kinetic phenomena in semiconductors is heavily dependent on type, quantity and impurity distribution and is very sensitive to such external actions as electric, magnetic, acoustic and temperature fields, deformation and illumination, these processes are the physical basis of numerous transformers used in up-to-date electronic, optical, photoelectric, magnetic, acoustic, strain gauge and piezoelectric devices. Clearly, profound research of new properties of compounds and solid solutions results in the discovery of new effects and possibilities in an advanced instrumentation [15] .
However, there is no any information on piezomodulation of conductance in TlInTe2 crystals. This paper aims at studying the effects of piezo-modulation in TlInTe2 ternary compound.
2. Experimental Technique
Fresh cleavages easily splits from massive ingot of thin standard-gauge plates in TlInTe2 crystals with four opposed mirror edges of natural cleavage were used to produce strain sensors.
The similar TlInTe2 crystals used to produce strain sensors-gauges can easily be derived with a simple pressing in of a sharp knife (blade thickness―0.01 mm) on loose tip of a thin but wide and long TlInTe2 plate at an acute angle.
After choosing the further step, determining the width of workpieces, the plate along with the stages of micromanipulator is moved under a microscope across the line of spallation.
Obtained zone melting methods needle crystals TlInTe2 with mirrored facets without any additional treatments were ready for welding pins and landing them on a base substrate (Figure 1(a)).
Mechanically reliable ohmic contacts on the mentioned workpieces were produced by spot welding of wires by capacitor discharge to the ends of a workpiece heated in the flow of inert gas. This method was effective and reliable.
Steel plates with a thickness of 1 mm and a length of 30 mm were used like tared beams for glued transducers. The substrate surface corresponded to the 7th class of
Figure 1. (a) A needle crystals TlInTe2, (b) tensosensor (strain gauge transducer).
processing. Before applying the sublayer, the given substrates were treated in toluene to be defatted, being later washed in ethyl alcohol. The sublayer of epoxy-cresol varnish (EP-96), being a solution of epoxy resin (E-40) modified by equal acid with butanolizedresol “RB” and K-421-02 resin, was brushed on cleaned substrates (surface patch).
A thickness of sublayer is 16 micrometers. In the process applying of sublayer was ensured uniform coating by thicker.
After 2 hour exposure in room temperature, the substrate is moved to drying cabinet for high-temperature polymerization.
Slow rise of temperature to 480 K and 2 hour exposure in room temperature contributes to full polymerization, preventing from air bubbles. A second layer of varnish, exceeding the size of resistance strain gauge, is applied on substrate over sublayer.
TlInTe2 crystals with welded pins are landed on a layer of varnish and are slightly pressed, while, crystal surface is completely varnished. At the same time, crystal is properly positioned in substrate plane.
A device is covered with 1.5 mm thin width fluoroplastic (PTFE) tape for a tighter contact with transducer and maintenance of specified orientation of transducer in regard to substrate. A transducer is dried an hour at 300 K with post annealing and two hours at 460 K.
If necessary, after drying, fluoroplastic (PTFE) tape is easily removed from ready transducer. This drying mode proved to be optimal and the instruments showed the highest sensitivity (Figure 1(b)).
The following step is to study tensometric features of the strain sensor on the basis of TlInTe2 single crystals.
We found that if mechanical oscillations are excited on a thin steel plate fixed at one end with TlInTe2 glued crystals, modulation of conductance has the same frequency then.
The effect of piezo-modulation was recorded by the scheme in Figure 2.
υ frequency and ΔVon amplitude values of piezo-signal were recorded by a F510 selective amplifier. Depending on the task, piezo-signal in some cases was recorded on the tapes of 8LS type recorders. Similarly, C 1-29 type storage oscilloscopes proved to be convenient.
3. Experimental Results and Their Discussion
In the study of piezo-modulation in TlInTe2 crystals, a recorded piezo-signal on the display screen is shown in Figure 3. Under certain parameters of measuring circuit (V, RH and RK), determination of relative resistance change ΔRn/Rk used to estimate strain gauge factor is determined on the basis of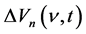 .
.
Experimental values on the study of piezo-modulation effects in different peaks of mechanical deformation and illumination are in Figure 3, while, dependence of signal level on amplitude of mechanical oscillations at = 85 Hz in the dark is shown in Figure 4. Under the influence of mechanical deformation, the effect of oscillation is strengthening.
The modulation of conductance and mechanical deformation of sample were considered to be at equal ( ) frequency to explain the identified effects in TlInTe2 single
) frequency to explain the identified effects in TlInTe2 single
Figure 2. The registration scheme of piezo-modulation conductance.
Figure 3. TlInTe2 oscillograms of piezo-signal at 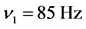 (а)
(а) 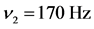 (b)
(b)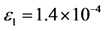 ,
,  (I unite. = IV).
(I unite. = IV).
Figure 4. The modulation of piezo-conductance of crystals depending on the degree of deformation (a) and illumination (b) (vertically  /div (1 c/div), horizontally 1 ms/div.)
/div (1 c/div), horizontally 1 ms/div.)
crystals.
The variable component of total conductance, i.e. piezo-conductance is determined by the formula
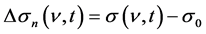 (1)
(1)
 is the total conductance,
is the total conductance,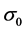 ―conductance with no external action. The desired signal, giving in formation features of external actions is determined by
―conductance with no external action. The desired signal, giving in formation features of external actions is determined by 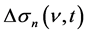 variable component. If along with the crystals with periodic stretching and contraction at u frequency, the battery is switched on with V constant voltage and RH load resistance, ΔVn (n, t) variable piezo-signal will be the last to emerge with its value in low resistance (
variable component. If along with the crystals with periodic stretching and contraction at u frequency, the battery is switched on with V constant voltage and RH load resistance, ΔVn (n, t) variable piezo-signal will be the last to emerge with its value in low resistance ( ―the resistance of the crystal) which is directly proportional to piezo-conductance:
―the resistance of the crystal) which is directly proportional to piezo-conductance:
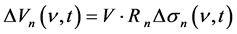 . (2)
. (2)
It should be noted that, the main practical and methodical tasks of semiconducting strain gauging aim at determining pure piezo-conductance:
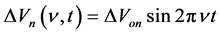
It is known that the latter is determined by a variable component of the current

According to Ohm, the absence of deformation:

When with deformation the resistance of crystal decreases in ΔRn(n, t), the current increases in In(n, t), then

After simple transformations in the last three equations [3] - [5] , it follows that:

Thus, strain gauge for the constant field (

Here, the relative deformation is determined by 

with 
It should be noted that, the presence of piezo-resistive effect is more observed in dynamic mode. In fact, if a crystal is undergoes to proportional deformation 



The amplitude values increases with an increasing degree of deformation.
However, when an optical illumination is laid, additional conductance 



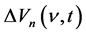


Consequently, piezo-photoconductance:

Thus it is possible to track changes of piezo-photoconductance with an increasing intensity of illumination, while measuring amplitude values at the same degree of deforma-
tion in the dark 

For this purpose, the recording was made (Figure 4(b)) at a constant amplitude of sine like deformation 
the amplitude of piezophoto-conductance 
According to oscillograms in Figure 4, piez-osignal at I = 6500 lux in tensity and 


According to experimental data, an observed piezo-photoresistive effect may significantly broaden the possibilities of semiconducting strain gauging to record dynamic processes (Figure 3 and Figure 4).
4. Conclusion
The investigation of the piezo-modulation effects and conductivity of single crystal TlInTe2 revealed that with the change of the value mechanical deformation and optical illumination can be obtained materials with high strain sensitivity for recording dynamic processes.
Cite this paper
Gojaev, E.M., Agaeva, S.Kh., Kulieva, S.O. and Dadashov, M.T. (2016) Piezomodulation of Connection Conductance TlInTe2. Open Journal of Inorganic Non-Metallic Materials, 6, 25-32. http://dx.doi.org/10.4236/ojinm.2016.64005
References
- 1. Müller, D., Eulenberger, G. und Hahn, H. (1973) über Ternare Thallium Chalkogenide Mit Thalliums-Selennid-Struktur. Zeitschrift für Anorganische und Allgemeine Chemie, 398, 207-220.
- 2. Müller, D. and Hahn, H. (1978) Zur Strukture des TlGaSe2. Zeitschrift für Anorganische und Allgemeine Chemie, 438, 259-271.
- 3. Gojayev, E.M. and Kafarova, D.M. (2004) Thermal Expansion and Isothermal Compressibility TlGaTe2. Materialy Russian Academy of Sciences, 40, 1060-1061 (Russia).
- 4. Gojayev, E.M., Gulmammadov, K.D., Khalilov, H.S. and Guliyev, S.O. (2011) Switching Effect in Thin Films TlInSe2. Electronic Materials Processing, 47, 18-22 (Russia).
- 5. Henkel, W., Hocheimer, H.D., Carlone, C., Werner, A., Ves, S. and Shnering, H.G.V. (1982) High-Pressure Raman Study of Ternary Chalcogenides TlGaSe2, TlGaS2, TlInS2 and TlInSe2. Physical Review B, 26, 3211-3222.
- 6. Orujov, G.S., Gojayev, E.M., Kerimov, R.A. and Allahyarov, E.M. (2006) Band Structure and Optical Properties of the Chain Connection TlInTe2. FTT, 48, 40-43(Russia).
- 7. Аbdullаevа, S., Mаmedov, N. and Orudzhev, G. (1983) Bаnd Structure of TlGаSe2. Physica Status Solidi (b), 119, 4845.
- 8. Аbdullаevа, S. and Mаmedov, N. (1986) Bаnd Structure of TlGаSe2 Ternary Layered Crystals. Physica Status Solidi (b), 133, 171-177.
- 9. Kilday, D., Niles, D., Margaritondo, G., et al. (1987) Electronic Structure of the “Chain” Chalkogenide TlInSe2. Physical Review B, 35, 660-663.
- 10. Orudzhev, G., Mamedov, N., Uchiki, H, Yamamoto, N., Iida, S., Toyota, H., Gojaev, E. and Hashimzade, F. (2003) Band Structure and Optical Functions of Ternary Chain TlInSe2. Journal of Physics and Chemistry of Solids, 64, 1703-1706.
- 11. Al Orainy R.H. (2012) Interpretation of Switching Properties of InGaSe2 Single Crystal. Acta Physica Polonica A, 121, 666-672.
http://dx.doi.org/10.12693/APhysPolA.121.666 - 12. Gojayev, E.M., Allahyarov, E.A. and Sadikhov, H.O. (1994) Tenzometric Properties of Single Crystals TlInTe2. Materialy Russian Academy of Sciences, “Inorganic Materials”, 30, 859-860 (Russia).
- 13. Mobarak, M., Berger, H., Lorusso, G.F., Capozzi, V., Perna, G., Ibrahim, M.M. and Margaritondo, G. (1998) The Growth and Properties of Single Crystals of GaInTe2, a Ternary Chalkogenide Semiconductor. Journal of Physics D: Applied Physics, 31, 1433-1437.
- 14. Yee, K.A. and Albright, T.A. (1991) Bonding and Structure of TlGaSe2 by Tight Binding Calculations. Journal of the American Chemical Society, 113, 6474.
- 15. Gojayev, E.M., Nazarov, A.M., Allahyarov, E.A., Gulmammadov, K.D., Khalilov, H.S. and Mammadov, E.M. (2007) Acoustic Photovoltaic Effect in Single Crystals TlInSe2, TlInTe2 and TlGaTe2. Inorganic Materials, 43, 1184-1189 (Russia).






