Journal of Power and Energy Engineering
Vol.05 No.03(2017), Article ID:74793,17 pages
10.4236/jpee.2017.53001
Transient Behavior in Water Distillation Tower for Tritium Separation and Its Long-Time Operation Test Results
Yoshiaki Miho1,2, Satoshi Fukada1, Mitsuki Arimoto2, Takaho Takeuchi2, Tomohiro Motomura2, Junji Mizutani2, Satoru Hirano2
1Department of Advanced Energy Engineering Science, Kyushu University, Kasuga, Japan
2Sasakura Engineering Co. Ltd., Osaka, Japan
Copyright © 2017 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: February 17, 2017; Accepted: March 18, 2017; Published: March 21, 2017
ABSTRACT
Transient separation behavior in tritiated water distillation tower packed with materials having ability to adsorb water is investigated analytically and experimentally for nuclear reactor safety. Analytical equations based on the stage model are set up for simulation of the transient behavior of tritium (T) separation from wastewater. It is found that a dimensionless time defined in terms of the inside vapor flow rate and the liquid holdups in tower, reboiler and condenser can correlate variations over time to achieve a steady-state T concentration. However, when mixing with different T concentrations at a feed point occurs, the transition time period becomes longer than expected. Effects of the reflux ratio, the stage separation factor and the total stage number on the transient and steady-state T concentrations are numerically calculated. Variations over time to achieve each steady-state value are compared with experimental data using a small-scale tower. Long time distillation experiment for one month has been completed, and it is confirmed that a distillation column packed with ceramic Raschig rings coated with zeolite 13X adsorbent is hardly affected by water corrosion.
Keywords:
Water Distillation, Tritium, Isotope Separation, Transient Analysis, Adsorption, Wastewater

1. Introduction
Tritium (T) is an evaporable radioisotope having half-decay time of 12.3 years. T is also a research target as a fuel for the future fusion reactor. Although the isotope emits only a weak beta ray, it is easily exchanged with H atom in natural water. Therefore, high concentration tritiated water can cause internal radiation exposure to human body. Consequently, it is necessary to separate or remove radioactive T from tritiated water less than the regulated value of 60 Bq/cm3 in wastewater.
Various methods to separate hydrogen isotopes were proposed and investigated previously [1] . D2O moderator for heavy water reactor is detritiated by the liquid phase chemical exchange (LPCE) method effectively. Although the isotopic chemical exchange system between H2 and HDO shows a comparatively larger isotope separation factor, it needs a special Pt catalyst when it is operated around at 70˚C. Therefore, it may not be suitable for large-scale detritiation of wastewater. Since the T concentration in reactor cooling water is very low, the apparatus for T recovery is estimated a huge one. Distillation is a possible method to separate evaporable component mixtures continuously [2] and is widely utilized to separate crude oil or hydrocarbon mixtures in petrochemical industry [3] . Separation of O-18 by cryogenic distillation is successfully performed in a Japanese company [4] .
There were some analytical or experimental investigations on distillation previously. The stage model is one of the several analytical methods to determine the transient behavior in a distillation tower [5] . The relation between the stage model and the mass-transfer coefficient one was understood to be complementary in the transfer process between condensate and vapor countercurrent flows in a distillation tower [6] [7] . Transient response in separation processes was also in good agreement with experiment [8] [9] . Different types of column arrangement for distillation [10] or utilization of heat pump [11] were also proposed for wider applications of distillation system for hydrocarbons.
We are performing experimental research aiming at large-scale separation system to detritiate wastewater generated in the Fukushima Daiichi nuclear power station lower than the regulated level of radioactivity. Not only distillation can separate components by simple operation but also large-scale separation process may become possible if evaporation heat is effectively recovered by a heat pump system. However, when it is applied to an actual water distillation system as it is, the volatility ratio between H2O and HTO is 1.028 at 100˚C or 1.055 even at 60˚C. Consequently, it needs a large-scale multi-column system to achieve high enrichment product or high decontamination factor. Since the isotope separation factor of water distillation is not so large, some trials to increase separation efficiency by the decrease of the height equivalent to a theoretical plate (HETP) were made [12] [13] . However, since operation power is still large if as it is, it is considered to be difficult to apply it to an actual large-scale operating system. On the other hand, when packing materials have ability to adsorb water, slightly larger isotope effect is observed in its adsorption or desorption process. It was found in our previous studies [14] [15] [16] that enhancement of the isotope separation factor by use of adsorptive packing is possible. Our group has clarified that mesoporous zeolite has shown the larger enhancement of the separation factor in water distillation system and the separation performance for
detritiation is improved significantly after various intensive trials. These results are applied for an international patent [17] . In addition, use of heat pump leads to significant reduction of evaporating heat necessary for distillation [18] . In the present study, initial transient behavior from the start of distillation operation and, on the contrary, long-time operation for one month is focused in order to apply it to actual detritiation processes for wastewater. Analytical study to clarify the transient behavior at startup is performed based on the plate model, and comparison is made between experiment and analysis using a small-scale apparatus. In addition, possibility of changes of distillation performance due to effects of water corrosion on packed materials is investigated for long-time operation experimentally. One month continuous operation of water distillation is tested using a distillation tower packed with ceramic Raschig ring coated with zeolite 13X.
2. Analysis
2.1. Material Balance Equations
A distillation system investigated analytically is shown in Figure 1, which is composed of a packed column, a condenser, a reboiler, one feed port and two extraction ones at the column top and bottom. Variations of the T concentration with time are analyzed based on a widely accepted stage model. The general transient mass-balance equations of T and liquid holdup in the number i stage inside a distillation tower are described as follows:
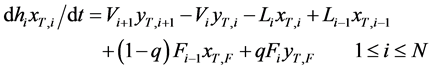 (1a)
(1a)
Figure 1. Arrangement of distillation tower with two reservoirs of reboiler and conden- ser.
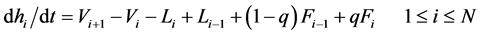 (1b)
(1b)
where Fi is a feed rate at the specified feed stage number of i and other marks and symbols are explained in Nomenclature. The distillation tower is assumed to be operated under the following feed condition:
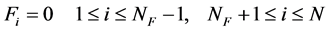 (2a)
(2a)
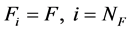 (2b)
(2b)
In addition, it is assumed that the T concentration in each stage 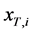 is uniform within one unit stage and the vapor holdup can be ignored compared with the liquid one. The mass-balance equations of T and liquid holdup in a condenser
is uniform within one unit stage and the vapor holdup can be ignored compared with the liquid one. The mass-balance equations of T and liquid holdup in a condenser 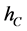 are described as follows:
are described as follows:
 in a condenser (3a)
in a condenser (3a)
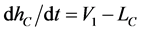 (3b)
(3b)
The mass-balance equations of T and liquid holdup in a reboiler hR are done similarly as follows:
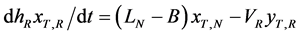 in a reboiler (4a)
in a reboiler (4a)
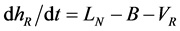 (4b)
(4b)
Since equilibrium between two isotopesis achieved within a stage i, the following stage separation factor between H2O and HTO is defined between the T molar fractions of vapor yT,i and condensate xT,i:
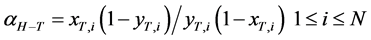 (5)
(5)
The stage separation factor αH-T is assumed to be constant throughout the column in the stage model, and the ratio of the height of the packed column H to the total stage number N is called the height equivalent to a theoretical plate HETP as follows:
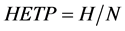 (6)
(6)
The value of HETP is a constant depending on the vaporization rate and packed materials. The relation between HETP and the mass-transfer coefficient is already described in previous researches [2] [3] .
The set of the above nine Equations (1a)-(5) can give variations over time of the T concentration in the whole stages, reboiler and condenser under proper initial conditions. Several assumptions without loss of generality are set here in a similar way to previous papers [5] . A typical but widely accepted distillation condition is applied to a column having one feed and two extractions from a reboiler and a condenser, and the tower is operated immediately after the start of reboiler heating and condenser cooling under no heat loss condition in the distillation column. Consequently, the liquid and vapor flow rates in each stage, Li and Vi, are assumed to be constant. In addition, the holdup hi in every stage in a distillation tower approach their respective steady-state values immediately. Then the inter-stage flow rates of Li and Vi and the feed, distillate and bottom flow rates of F, D and B approach the following values immediately after the start of vaporization and condensation:
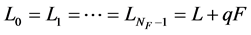 (7a)
(7a)
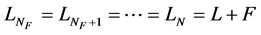 (7b)
(7b)
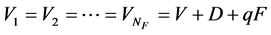 (8a)
(8a)
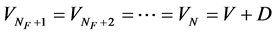 (8b)
(8b)
where L and V defined above are the constant parts of flow rates of liquid and vapor independent of feed and extraction conditions. The relation of 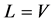 is held in an adiabatic distillation tower under steady-state condition. The conditions of the liquid and vapor flow rates inside tower are also shown in Figure 1.
is held in an adiabatic distillation tower under steady-state condition. The conditions of the liquid and vapor flow rates inside tower are also shown in Figure 1.
When the four conditions of Equations (7a)-(8b) are applied to the set of Equations (1a)-(2b), Equations (1a) and (2b) are reduced to the following equations:


Equation (1a) at 



The T mass balance of Equation (3a) in the condenser and Equation (4a) in the reboiler are modified to the following equation:


Since all liquid is present in any of the reboiler, column and condenser at first and the T concentration is uniform in the whole distillation tower initially, the following initial conditions are applied:

The transient T concentrations in the present distillation system are determined by solving Equations (9)-(14) under the initial condition of Equation (15).
2.2. Analytical Equation for Steady-State T Concentration in Distillation System
When all the left hand sides of Equations (9)-(14) become zero, the steady-state operation has been achieved in the distillation tower. Then the T atomic molar fraction in the stage i in the tower xT,i and the atomic molar fractions in the condenser and reboiler, xT,C and xT,R, are determined by analytical equations under specified conditions.
When the T molar fraction is very small in wastewater, Equation (5) to give the relation between xT,i and yT,i can be simplified to the following linear relation:

The stage separation factor αH-T is defined here so as to αH-T > 1. The steady- state solutions for Equations (9)-(14) under the condition where the T molar fraction is very low can be obtained as follows:


where the condensate reflux ratio RD and the bottom vapor reflux ratio RB are defined as follows:


The following relation is satisfied between RD and RB:

In addition, since the relations of 


Similarly, the following relation is held among the feed and two extraction rates:

Judging from Equation (17), it is necessary to operate the distillation column at the condition of 


A similar condition of 

When the condensate reflux ratio of RD is infinite, i.e., under the total reflux condition, the T abundance ratio between distillate and product flows is correlated by the following Fenske’s equation regardless of the T molar fraction:

When the reflux ratio is a finite value, the concentration ratios defined as the ratios of 

3. Calculation Results
3.1. Transient Concentration Changes in Distillation Tower with one Feed and Two Extractions
Figure 2 shows calculation examples of transient behavior of the T concentration in condenser, reboiler and feed point under the same conditions of the total stage number of N = 100, the product cut ratio of
Figure 2. Variations of T concentrations in reboiler, condenser and feed point with constant feed and extractions.
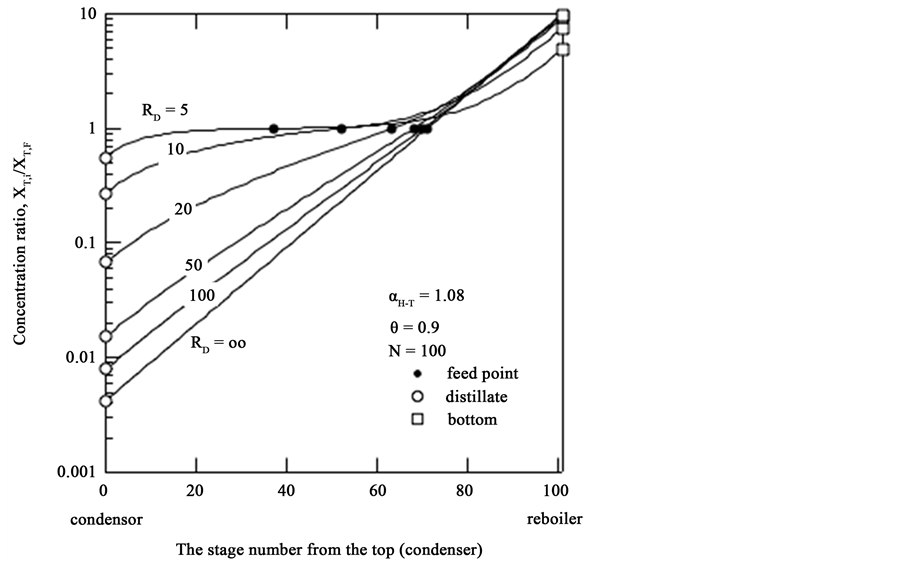
3.2. Steady-State T Concentration Profile as a Function of Reflux Ratio
When T distillation is operated with one feed and two extractions at the top and bottom of the column, a steady-state T concentration profile in the upper depleting and lower enriching sections is achieved as shown in Figure 3. The value of the H-T stage isotope separation factor of 

The values of the bottom/distillate T concentration ratio defined as 

Figure 3. Steady-state T concentration in a distillation column.
Figure 4. Steady-state T concentration ratio between distillate and bottom flow rate and T recovery ratio as a function of reflux ratio.
tion ratios between distillate and bottom flows are 28, 137 and 660 at the different values of the reflux ratio of RD = 10, 20 and 50 under the same product cut of 
3.3. Correlation of Transient T Concentration under Total Reflux Condition
Here it is focused on correlation of transient time among different conditions of the total stage number and the stage separation factor. Figure 5 shows calculation results of the transient T concentration changes with time under three different conditions of the total stage number. In order to avoid the effects of T mixing at the feed point, operation under the total reflux condition are compared for simplicity. The reason why the dimensionless time of 


As shown in the figure, the T concentrations in the condenser and reboiler approach their respective steady-state concentrations of 
Figure 5. Variations over time of T concentrations in reboiler and condenser under total reflux condition for different stage numbers. The values in the bracket show the ratio of 
when 

Judging from the transition behaviors of the T concentrations in reboiler or condenser, variations of the T concentration in the reboiler or condenser can be simulated by the following approximated equations:


Figure 6 shows correlations of the normalized T concentrations in the condenser and reboiler using Equation (28) and (29). The calculation is carried out in the ranges of the total stage number (N = 30, 50 and 100), the stage separation factor (αH-T = 1.05, 1.1 and 1.15) and the liquid holdup (
Figure 6. Correlation of transient concentration curves in condenser and reboiler.
4. Experiment
4.1. Apparatus and Methods
A small-scale H2O/HTO distillation system was set up in the radioisotope laboratory of Kyushu University. A schematic diagram of the experimental apparatus for distillation of tritiated water is shown in Figure 7. The distillation column has an outer diameter of 114.3 mm and a height of 1025 mm. The reboiler has an outer diameter of 318.5 mm and a length of 960 mm. The condenser has an outer diameter of 267.4 mm and height of 400 mm. All the tubes are made of SS-304 or partly SS-316, and the thickness of the three tubes is 3.0 mm. The inside of the column, reboiler and condenser is evacuated by a vacuum pump with the evacuation rate of 125 L/min, which makes it possible to operate distillation even at reduced pressure of 12 kPa. Two heaters to evaporate tritiated water have the maximum heating capacity of 10 kW for each, and the distillation column is sufficiently insulated from the outside air. The boiling temperature for distillation ranges from 50˚C to 90˚C under reduced pressure. Five thermocouples, two pre- ssure monitors, two level meters and two liquid flow meters are attached in the system, and the system pressure, temperature and flow rate are controlled at specified values within the demanded accuracy.
The following two types of packing are tested: (1) Sulzer regular structured packing of Katapak-lab enclosing zeolite 13× beads of 3 mm in diameter, and (2) ceramic Raschig ring as irregular packing coated with zeolite 13X of nominal diameter of 
Figure 7. A schematic diagram of the experimental apparatus for tritiated water distillation.
the experiment is 6.52 × 108 Bq/m3 and the total volume of tritiated water is 5.68 × 10−2 m3. Sample ports are attached at two locations of condenser outlet and reboiler inlet. The T activities in liquid samples taken out from the reboiler inlet and condensor outlet are detected by a liquid scintillation counter. A water sample of 0.5 cm3 is mixed with liquid scintillator cocktail of 5.0 cm3. The scintillator used here is a scintillation cocktail called the commercial name of Hydrofluor LS-111. The T activities in samples are detected after standing still in a darkbox for one day in order to decrease background scintillation.
In order to clarify the transient distillation performance using tritiated water distillation tower packed with the above adsorbents, the two types of experiment are performed: (1) initial start-up T separation test up to achieve a steady-state T concentration profile in a distillation column, reboiler and condensor, and (2) long-time performance change in order to find out deterioration of adsorbent packing materials in a distillation tower due to water corrosion. Run time of the experiment (1) is one day, and a small amount of T sample is extracted from the reboiler and condenser every 2 hour and until maximally 24 hours. Experiment (2) is performed for one-month continuous operation under the condition where all the experimental parameters are maintained at constant. Liquid samples from the two ports are taken out once a day. All the experiment is performed under the total reflux condition.
Before the T distillation experiment, heating rates where loading and flooding begin were determined using the distillation tower packed with normal Raschig rings. The loading heat rates (or loading vaporization rate) determined at three boiling temperatures are 1.07 MW/m2 (0.45 kg/m2s) at 50˚C, 1.44 MW/m2 (0.61 kg/m2s) at 65˚C and 2.08 MW/m2 (0.92 kg/m2s) at 100˚C, respectively. When the distillation tower is operated under lower heating rate than the loading point, it is confirmed that no loading or flooding occurs in it. The above two distillation experiments were always carried under the no loading and flooding conditions.
The number of stages for the Sulzer structured packing and Raschig ring packing, N, is 2.5 to 4 in the present experiment. The vapor flow rate per unit cross sectional area of the column is 0.14 - 0.69 kg/m2s, which is equal to V/A in the analysis. Although the column height or the number of stages is smaller than the actual separation tower, the vapor flow rate per unit cross sectional area is designed to be equal to the actual tower. The total volume of the distillation system is 5.68 × 10−2 m3, which is equal to 
4.2. Results of Transient Behavior between Experiment and Analysis
Figure 8 shows comparison between experiment and calculation for variations of the total separation factor 


Figure 8. Comparison of transient T concentration variations between experiment and analysis under the total reflux condition.
4.3. Long-Time Performance of Distillation Column Packed with Ceramic Raschig Ring Coated with Zeolite 13X
Long-time distillation experiment during one month has been performed using a distillation column packed with ceramic Raschig ring coated with zeolite 13× under the total reflux condition. The purpose of this experiment is to investigate how the packing materials are deteriorated with water corrosion or not. The experimental distillation conditions are as follows: (i) Tritiated water in reboiler is evaporated using an electric heater of 12 kW and the vaporization rate is always kept to be constant 0.56 kg/m2s. (ii) The boiling temperature is controlled to 65˚C throughout the experiment. (iii) Each sample water in the rebolier and condenser is taken out every one day, and its T activity is detected by a liquid scintillation counter.
Figure 9 shows variations of the total separation factor 


Figure 9. Results of long-time distillation of tritiated water using ceramic Raschig ring coated with zeolite 13X.
5. Conclusion
Variations of the transient T concentrations in tritiated water distillation column, reboiler and condenser with time were analytically and experimentally investigated. Calculation transient changes of the T concentrations in the reboiler and condenser were well correlated in terms of the dimensionless time of 
Acknowledgements
Part of the present research was supported by JSPS KAKENHI Grant Numbers 15K14281.
Cite this paper
Miho, Y., Fukada, S., Arimoto, M., Takeuchi, T., Motomura, T., Mizutani, J. and Hirano, S. (2017) Transient Behavior in Water Distillation Tower for Tritium Separation and Its Long-Time Operation Test Results. Journal of Power and Energy Engineering, 5, 1-17. https://doi.org/10.4236/jpee.2017.53001
References
- 1. Geniesse, D.J. and Stegen, G.E. (2009) Evaluation of Tritium Removal and Mitigation Technologies for Wastewater Treatment. DOE/RL-2009-18.
- 2. Stichlmair, J.G. and Fair, J.R. (1998) Distillation: Principles and Practice. Wiley-VCH, Weinheim.
- 3. McGreavy, C. (2014) Dynamics and Control of Chemical Reactors and Distillation Columns. Elsevier Science, Amsterdam.
- 4. Kihara, H., Kanbe, T., Hayashida, S. and Kawakami, H. (2004) Development of Oxygen-18 Separation Process by Oxygen Distillation. Taiyo Nissan Giho, 23, 14-19.
- 5. Sittel Jr., C.N. and Fisher, G.T. (1973) Transient Response of a Distillation Column Plate. Part I. Theory: Five Models and Their Fourier Transforms. Separation Science, 8, 419-444.
https://doi.org/10.1080/00372367308068445 - 6. Baber, M.F. and Gerster, J.A. (1962) Experimental Transient Response of a Pilot-Plant Distillation Column: Part II. Response to Liquid and Vapor Rate Perturbations. AIChE Journal, 8, 407-415.
https://doi.org/10.1002/aic.690080329 - 7. Tommasi, G. and Rice, P. (1970) Dynamics of Packed Tower Distillation. Industrial & Engineering Chemistry Process Design and Development, 9, 234-243.
https://doi.org/10.1021/i260034a012 - 8. Ugryumova, D., Vandersteen, G. and Huyck, B. (2012) Identification and Modeling of Distillation Columns from Transient Response Data. 2012 IEEE International Instrumentation and Measurement Technology Conference, Graz, 13-16 May 2012, 2098-2103.
https://doi.org/10.1109/i2mtc.2012.6229227 - 9. Wittgens, B. and Skogestad, S. (2000) Evaluation of Dynamic Models of Distillation Columns with Emphasis on the Initial Response. Modeling, Identification and Control, 21, 83-103.
https://doi.org/10.4173/mic.2000.2.2 - 10. Flodman, H.R. and Timm, D.C. (2012) Batch Distillation Employing Cyclic Rectification and Stripping Operations. ISA Transactions, 51, 454-460.
- 11. Salim, M.A., Sadasivam, M. and Balakrishnam, A.R. (1991) Transient Analysis of Heat Pump Assisted Distillation Systems. 2 Column and System Dynamics. International Journal of Energy Research, 15, 137-148.
https://doi.org/10.1002/er.4440150207 - 12. Kaba, A., Akai, R., Yamamoto, I. and Kanagawa, A. (1988) Measurement of HETP of SUS Dixon Ring and Porcelain Packing in Small-Scale Water Distillation Column for H2O-HTO Isotope Separation. Journal of Nuclear Science and Technology, 25, 825-830.
https://doi.org/10.1080/18811248.1988.9735930 - 13. Sugiyama, T., Enokida, Y. and Yamamoto, I. (1999) Effects of Partition Wall within Packings on Separative Performances of Water Distillation for H2O-HTO. Journal of Nuclear Science and Technology, 36, 691-697.
https://doi.org/10.1080/18811248.1999.9726256 - 14. Fukada, S. (2004) Tritium Isotope Separation by Water Distillation Column Packed with Silica-Gel Beads. Journal of Nuclear Science and Technology, 41, 619-623.
https://doi.org/10.1080/18811248.2004.9715525 - 15. Fukada, S. (2005) Tritium Isotope Separation Using Adsorption-Distillation Column. Fusion Science and Technology, 48, 140-143.
- 16. Fukada, S. (2006) Transient Behavior of Enrichment of Tritium Water in Adsorption-Distillation Column. Journal of Nuclear Science and Technology, 43, 423-426.
https://doi.org/10.1080/18811248.2006.9711116 - 17. Hirano, S., Yuasa, M., Motomura, T. and Fukada, S. (2016) International Patent PCT/JP2016/067016.
- 18. Miho, Y., Fukada, S., Motomura, T., Mizutani, J., Hirano, S., Arimoto, M. and Takeuchi, T. (2017) Tritium Water Distillation Assisted with Adsorption and Isotopic Exchange. Fusion Science and Technology, in Press.
Nomenclature
B: Bottom flow rate, mol/s
D: Distillate flow rate, mol/s
F: Feed rate, mol/s
h: Hold-up in one stage, mol
hC: Hold-up in condenser, mol
hR: Hold-up in reboiler, mol
L: Constant part of liquid molar flow rate under adiabatic distillation, mol/s
LC: Liquid molar flow rate from condenser (=D + L0), mol/s
Li: Liquid molar flow rate at i stage, mol/s
N: The total number of stages in a distillation tower,
NF: The number of feed point in a distillation tower,


q: Ratio of vapor supplied as feed,
RB: Vapor reflux ratio of bottom defined as
RD: Liquid reflux ratio of distillate defined as
t: Time, s
V: Constant part of vapor molar flow rate under adiabatic distillation, mol/s
Vi: Vapor molar flow rate at i stage, mol/s
VR: Vapor molar flow rate evaporated from bottom reboiler, mol/s
xT,B: T atomic molar fraction in bottom liquid flow (=xT,N),
xT,C: T atomic molar fraction in condenser,
xT,D: T atom molar fraction in distillate,
xT,F: T atomic molar fraction in feed,
xT,i: T atomic molar fraction at stage i in condensed phase,
xT,R: T atomic molar fraction in reboiler liquid phase,
yT,F: T atomic molar fraction in feed vapor,
yT,i: T atomic molar fraction at stage i in vapor phase,
yT,R: T atomic molar fraction in rebolier vapor phase,
αH-T: Stage isotope separation factor between H and T,
αH-T,tot: Total isotope separation factor between H and T,
θ: Product or bottom cut defined as