Received 28 May 2015; accepted 12 July 2015; published 16 July 2015

ABSTRACT

The topological index of molecular graph is a number that attributed to the molecular graph and is valid than graph isomorphism, this number can reflect the properties of the molecules. In this study, Harari index in family phenacenes was calculated with some electronic and optical properties desired for a number of elements of the family, a model for predicting the electronic and optical properties by Harari index was prepared. To offer this model using mathematical software, electronic and optical properties of phenacenes calculated and compared with the data sources.

Keywords:

Nanostructures, Harari Index, Electronic and Optical Properties, Gap Energy, Phenacenes

1. Introduction

Around mid-century theoretical chemists and physicists noted that various features of molecular structure of organic matter can be established by examining the appropriate structure to obtain the molecular graph. These graph constants are appropriate for the purposes of the physical and chemical and called topological indices. Topological indices are real numbers in terms of graph parameters (such as the degree of vertices, distances, etc.) in the study on molecular graphs presented in chemistry and physical and chemical properties of molecules can describe [1] . One of these topological indices, Harari index was introduced in 1991 by Professor Harari [2] .

Today, scientists are trying to design and provide nanoscale electronic components. Manufacture nanoscale faced with limitations in many cases virtually impossible, therefore, due to small parts in recent years has led to the creation of nanostructures branch in electronics [3] [4] .

Molecular electronic also called moltronic is a branch of nano-electronics that study the use of small groups of molecules in nano dimensions. Phenacenes are organic molecules that are highly regarded in molecular electronics and nanoscale. Because of the important electronic properties of the family, many research and studies have been done on them [5] . But in the molecules that the number of rings is more than six rings, measuring the electronic and optical properties needed to spend a long time and high cost. In these circumstances, there is a model for predicting the electronic and optical properties will be particularly important [6] .

Definitions

Graph in mathematic is non-empty set of objects called vertices (V) are the vertices by lines called edges (E) connected and it show as G = G(V, E). A molecular graph is simple graph, which is mainly composed of atoms of one molecule and the bonds between the atoms, are graph edges. In chemical graphs hydrogen atoms have been removed and will be ignored and the degree of each vertex is maximum 4 and all bonds between atoms are considered single.

Topological indexes are defined on the basis of graph theory [7] [8] .

One of these topological indices, Harari index is defined by:

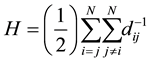(1)

where d_ij is elements of the distance matrix.

2. Methodology

The purpose of this paper is to obtain a simple model based on graph theory to predict optical and electronic properties of phenacenes.

Chemical formula phenacenes family is C_4n+2H_2n+4 with the n ≥ 2. For example, the Harari index for C₁₀H₈ molecules is calculated. In Figure 1. The simple graph of the molecule is drawn.

According to Figure 1, the inverse matrix of C₁₀H₈ calculated in Table 1. Is as follows:

Using the Equation (1) Harari index for molecules C₁₀H₈ number 23.9 obtained (H = 23.9).

Harary index molecules C_4n+2H_2n+4 to n = 7 as well as the calculated and results in Table 2 shown.

3. Results

Some electronic and physical properties of phenacenes family (C_4n+2H_2n+4), the Ionization Energy, Bind Energy, Gap Energy and Electron Affinity Energy using software (Gaussian 09) was calculated and experimental data in the valid literature was compared. The results in Table 3. Had shown [6] [9] [10] .

Using Table 2 and Table 3 data changes plot E_gap and E_bind in Harari index (H) in the Figure 2 and Figure 3 is drawn:

As the Figure 2 and Figure 3 can be predicted E_gap and E_bind in phenacenes family by the Harari index is possible and this prediction is very accurate, so that E_bind and E_gap carefully before R² = 1 predicted.

Figure 2 and Figure 3 can be expected to provide the following relationships:

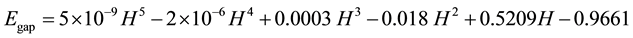(2)

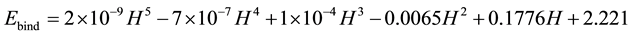(3)

Also in the Figures Figure 4 and Figure 5 changes diagram in both property Ionization Energy and Electron Affinity Energy of phenacenes family in term of Harari index (H) plotted.

Prediction of E_Ionization, E_Affinity by the Harari index Figures Figure 4 and Figure 5 is associated with very high accuracy, so that predicted E_Ionization and E_Affinity will carefully R² = 1 is possible in the family phenacenes. The Ionization Energy (E_Ionization) and Electron Affinity Energy (E_Affinity) C_4n+2H_2n+4 molecules can be predicted by the following relationship:

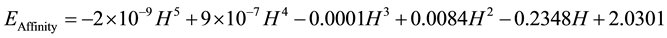(4)

(5)

4. Conclusions

As Figures 2-5 shown using the Harari topological index, some electronic and optical properties of phenacenes family are given by C_4n+2H_2n+4 predict with great accuracy.

The success of the predicted energy gap E_gap, bind energy E_bind, ionization energy E_Ionization and affinity electron energy E_Affinity with Relations (2)-(5) is possible.

Acknowledgements

We would like to thank Islamic Azad University Central Tehran Branch (IAUCTB) for helpful protections. Also we acknowledge all those who have helped us with their support and cooperation in conducting this study.

Cite this paper

Ali AsgharKhakpoor,Bahare AgahiKeshe, (2015) Electronic and Optical Properties of Nanostructures and Its Relationship with Harari Index. Journal of Materials Science and Chemical Engineering,03,1-5. doi: 10.4236/msce.2015.38001

References

NOTES

^*Corresponding author.